ASM Metals HandBook Vol. 8 - Mechanical Testing and Evaluation

Подождите немного. Документ загружается.

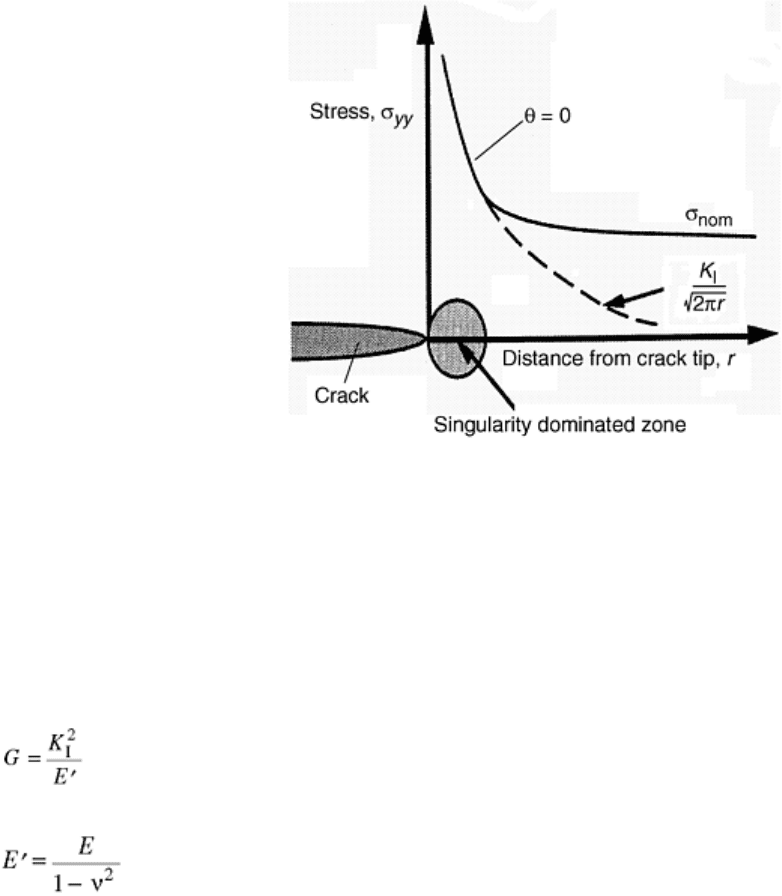
Fig. 3 Schematic plot of the stress field around a crack. Source: Ref 52

With Eq 4, it is possible to relate the magnitude of the single parameter, K, to the applied stress and crack size.

This is the basis for most common applications of LEFM. Through the use of published expressions for the

geometry factor, Y, many common loading conditions and structures can be analyzed. The published

expressions for K

that include the proper Y for specific loading and cracking conditions are commonly called

K-calibrations. The calculated stress intensity factor from the K-calibration and the loading level can be

compared to a critical stress intensity value to determine the safety of the structure (Ref 51, 52, and 53).

The energy release rate is related to the stress intensity factor by the equation:

(Eq 5)

where E′ = E (Young's modulus) for plane stress conditions, or where:

(Eq 6)

where ν is Poisson's ratio for plane strain conditions.

Critical Crack Growth. Crack extension can either be stable or unstable depending on material properties and

specimen geometry. Therefore, an important issue in LEFM is the definition of critical conditions that lead to

unstable crack growth. Critical conditions for unstable crack growth can be expressed as either a critical energy

release rate or a critical stress intensity factor. The critical value is the value of G or K at the instant when

unstable crack extension occurs, that is, when the crack propagates through the specimen and thus causes the

specimen to break in two. The critical energy release rate, G

, or the critical stress intensity factor, K

, are thus

defined as the values of G and K at the instant of unstable crack extension that leads to fracture. Under these

conditions, either parameter can be defined as the fracture toughness of the material. Usually K

is the chosen

parameter to express the fracture toughness. If the fracture toughness is not a function of specimen size or

geometry then this fracture toughness value can be considered a material property. Otherwise, the fracture

toughness result is only valid under the conditions it was measured (Ref 51, 52, 53, and 54).

Crack Growth Resistance and R-Curves. If stable crack growth occurs, a single value for fracture toughness is

difficult to define. In the case of stable crack growth, a plot of the experimentally measured fracture parameter,

in terms of either G or K, versus crack length is developed. This plot can be generated from the load and crack

length data taken from a material test. The stress intensity or the energy release rate is calculated from the

measured load and crack length data using expressions similar to those shown in Eq 2 and 4 (the correct

expression must be used for the conditions of the test). Fracture parameters calculated in this way are defined as

the resistance to crack growth parameters because they are calculated from a crack growing in a stable manner.

The resistance to crack growth, expressed in terms of the energy release rate, is given the symbol R, and the

resistance to crack growth, expressed in terms of the stress intensity factor, is given the symbol K

. The plot of

resistance to crack growth, either R or K

, versus crack length is called a crack growth resistance curve, or R-

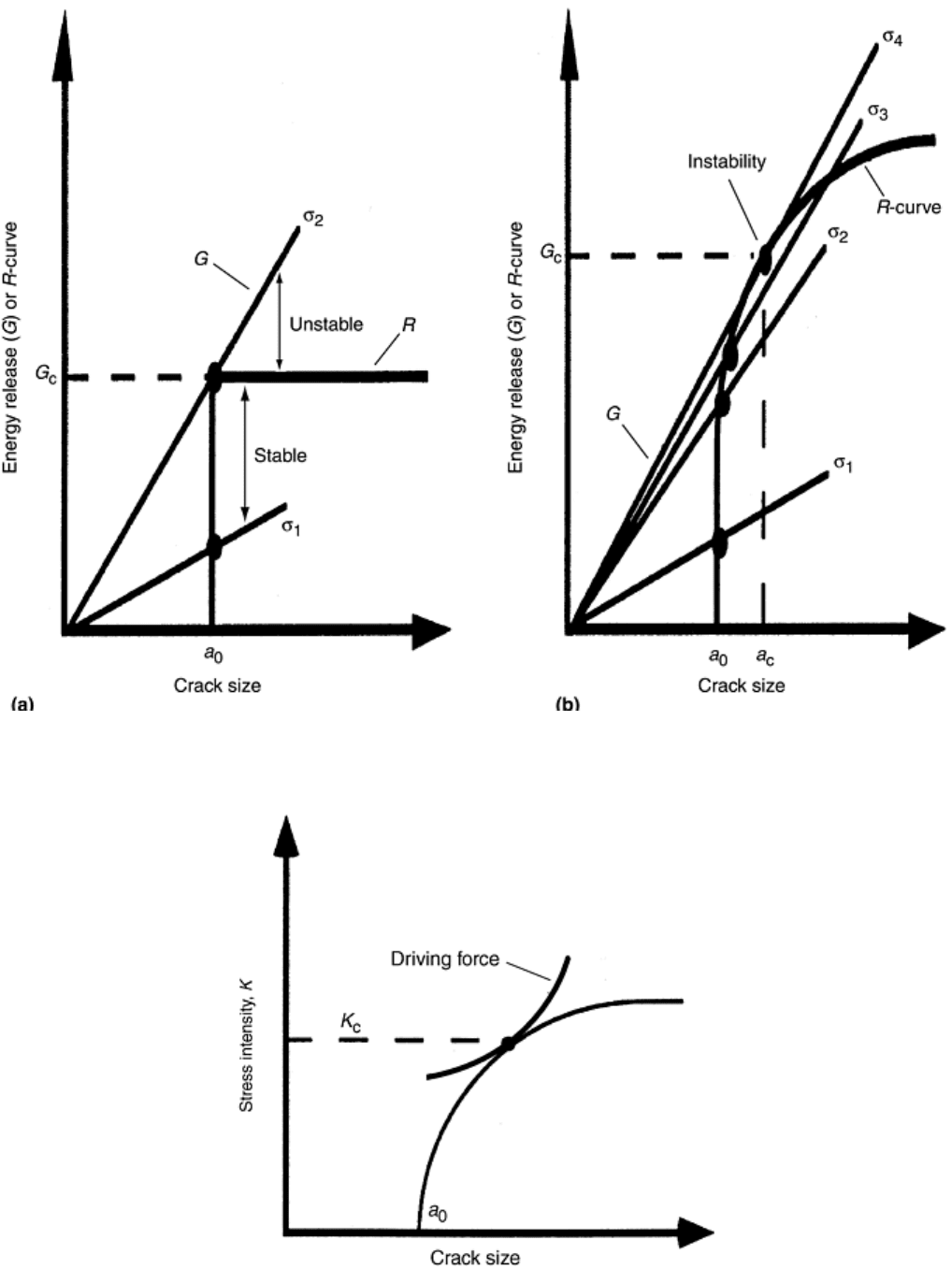
curve, and the entire curve becomes the measure of fracture toughness. Schematic examples of R-curves plotted

in terms of G and K are shown in Fig. 4 and 5, respectively (Ref 51, 52, andd 53).

Fig. 4 Schematic crack growth resistance curves and crack driving force curves, in terms of the energy

release rate, G. (a) Single-valued fracture toughness. (b) Rising R-curve behavior. Adapted from Ref 52

Fig. 5 Schematic crack growth resistance curve and crack driving force curve in terms of the stress

intensity factor, K. Adapted from Ref 52

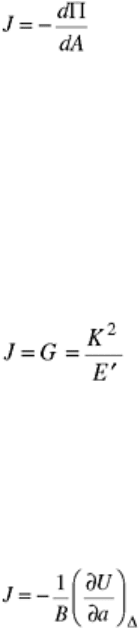
Figure 5(a) shows a flat R-curve, which is the result of unstable crack growth. The flat R-curve presents the

ease of defining fracture toughness as a critical value for unstable crack growth, in this case G

. Figure 5(b) is

an example of an R-curve that demonstrates stable crack growth prior to instability. Stable crack growth occurs

because the crack growth resistance increases with increasing crack length. This trend is called the rising R-

curve behavior. An R-curve plotted in terms of K rather than G is shown in Fig. 5 (Ref 51, 52, and 53).

The R-curve can be used to predict the conditions that will cause crack extension. To do this, it is necessary to

plot the crack driving force on the same axes as the crack growth resistance curve. The curve for crack driving

force is calculated using the same expressions of K and G that relate the geometry and loading conditions to

fracture. However, instead of using the data from a crack propagation test, the procedure is to calculate crack

driving force curves by holding the stress, or load, constant and increasing the crack length incrementally. The

point where the crack driving force curve crosses the resistance curve represents the condition under which

critical crack growth occurs (Ref 51, 52, and 53). The expected in-service stress levels of a cracked body can be

evaluated from this.

In summary, crack growth can either be stable or unstable. If the driving force curve crosses the resistance

curve at the tangency point, the crack growth will be unstable. If it crosses below this point, the crack growth

will be stable. To determine the point where the unstable crack growth occurs it is necessary to establish an

iterative scheme of generating driving force curves for different stress levels until tangency is achieved.

Schematic examples of driving force curves plotted with resistance to crack growth curves are also shown in

Fig. 4 and 5. Also evident in the figures are definitions of the critical energy release rate, G

Fig. ( 4) and

critical stress intensity factor K

(Fig. 5). These critical values are defined at the point of instability (Ref 51, 52,

and 53).

Elastic-Plastic Fracture Mechanics

EPFM does not have the requirement that the material behave in a linear-elastic manner. Instead, nonlinear or

plastic deformation is allowed in EPFM methods to a much greater extent than in LEFM methods. The primary

fracture parameter for EPFM is the J-integral. The J-integral, or more simply J, can be defined in two ways

(Ref 56, 57). The first is defined by a path-independent line integral around the crack tip. The second is an

energy definition similar to G, except that linear behavior is not required.

The energy definition of J states that J is a more general form of the energy release rate, G, where:

(Eq 7)

When permanent deformation occurs (as in the case of plastic deformation of metals), some of the stored

potential energy, Π, is dissipated and is therefore unavailable for crack extension. In this context, J can be

thought of as the potential energy absorbed by a cracked body prior to crack growth. In other words, J is a

measure of the intensity of the entire elastic-plastic stress-strain field around the crack tip, and J reaches a

critical value just prior to crack extension.

A special case of the energy definition of J is the value for G (when the energy is released as a crack grows in a

linear-elastic material). For the special case of linear-elastic behavior, there is little or no energy absorbed by

permanent deformation, and the only energy dissipation is due to the crack surface creation. Hence, under

linear-elastic conditions J = G:

(Eq 8)

where E′ is equal to E or is related to the elastic modulus per Eq 6.

Under nonlinear conditions beyond the elastic regime, calculating J can be much more difficult. Unlike the K

solutions or K-calibrations (which exist for many different crack and load configurations with K values for

many situations), expressions that relate J values to the applied load and crack configuration are very few. For

the simple situation of an edge-cracked specimen under elastic-plastic loading conditions, the energy definition

of J is as follows:

(Eq 9)

where B is the specimen thickness, Δ is the load-line displacement, and U is the area under the P-Δ curve up to

the initiation of crack growth (Ref 51, 52, and 53) where P is the applied load. Because a certain amount of the

deformation must always be elastic, the expression for J in Eq 9 can be separated into elastic and plastic

components and be rewritten as follows:

(Eq 10)

where η

is a dimensionless constant that depends on the specimen and loading configuration, U

is the

plastic area under the P-Δ curve, and b is the remaining ligament (W-a, where W is the specimen width).

Equation 11 is used in experimentally measuring J (Ref 51, 52, 53, and 57).

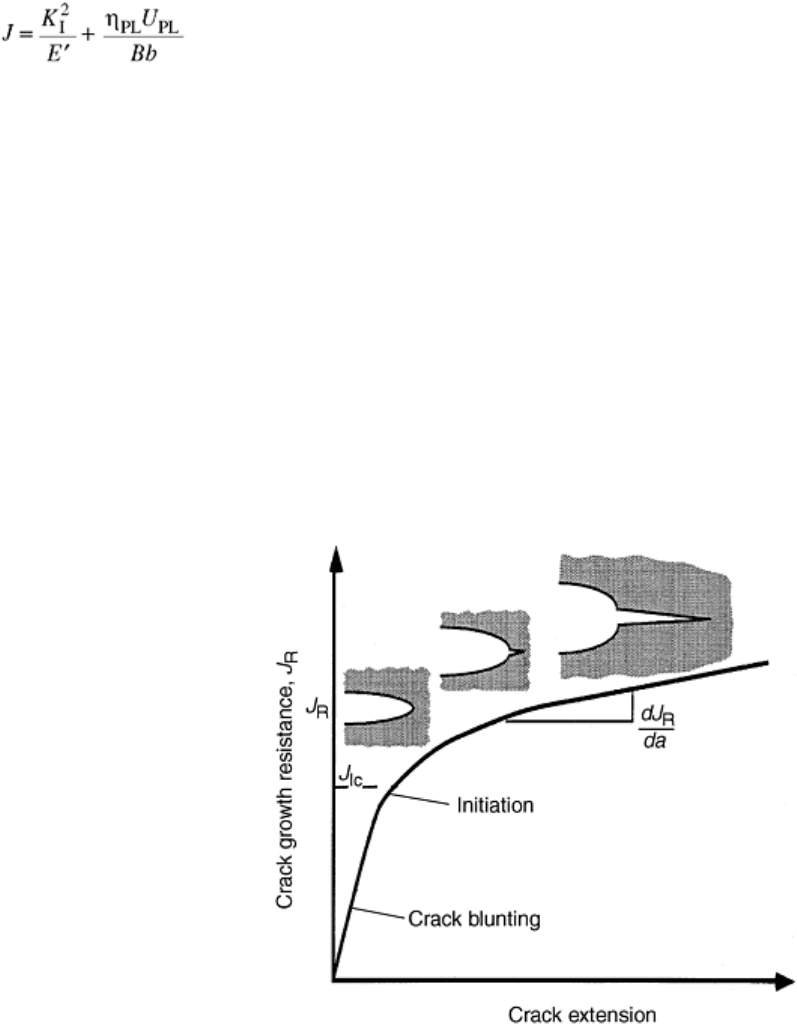
In the elastic-plastic regime, there is typically some amount of stable crack growth prior to an unstable crack

extension. The stable crack growth occurs because of energy dissipation and the crack blunting induced by

plastic deformation. As a result, when J is experimentally measured, an R-curve based on the J parameter is

generated. This J-based R-curve is a plot of the resistance to crack growth, J

, as a function of crack length or

extension (Fig. 4). The critical J, J

in the opening mode, is then taken from the J

curve at a point near the

initiation of crack growth (Ref 51, 52, 53, 54, 55, 56, and 57).

The basic procedure for experimentally measuring J involves testing a bend specimen or a compact tension

specimen with a deep crack and using the load, displacement, and crack length data to calculate J with Eq 11. J

is calculated for several different crack lengths, and the J

curve is generated. From the J

curve, the J

is taken

at the point where initial crack extension occurred, as shown in Fig. 6. The test can be done with one of two

goals. If the point of the test is to determine J

, J is calculated with less attention to the fact that some crack

extension occurred during the test. Ignoring the crack extension does not present much error because the crack

growth initiation is the important feature in the test. If the development of the full J

curve is the goal of the

test, more care is taken in the data analysis to take into account the growing crack (Ref 51, 52, 53, 54, and 57).

Fig. 6 Schematic crack growth resistance curve in terms of J

. Adapted from Ref 52

References cited in this section

51. R.W. Hertzberg, Deformation and Fracture Mechanics of Engineering Materials, 3rd ed., John Wiley &

Sons, 1989

52. T.L. Anderson, Fracture Mechanics, 2nd ed., CRC Press, 1995

53. J.M. Barsom and S.T. Rolfe, Fracture and Fatigue Control in Structures, Prentice-Hall, Inc., 1987

54. “Standard Test Method for Plane-Strain Fracture Toughness of Metallic Materials,” ASTM Standard E

399–90, ASTM Book of Standards, Vol 03.01, American Society for Testing and Materials, 1995

55. J.R. Rice, Journal of Applied Mechanics, Vol 35, 1968, p 379–386

56. J.A. Begley, G.A. Clark, and J.D. Landes, Results of an ASTM Cooperative Testing Procedure by

Round Robin Tests of HY130 Steel, JTEVA, Vol 10 (No. 5), 1980

57. “Standard Test Method for J

, A Measurement of Fracture,” ASTM Standard E 813-87, ASTM Book of

Standards, Vol 03.01, American Society for Testing and Materials, 1995

Fracture Toughness of Ceramics and Ceramic Matrix Composites

J.H. Miller, Oak Ridge National Laboratory P.K. Liaw, The University of Tennessee, Knoxville

Fracture Mechanics of Ceramics and CMCs

The concepts of both LEFM and EPFM methods, as previously described in the context of metallic materials,

provides a general basis for the fracture mechanics of monolithic ceramics and ceramic matrix composites.

Generally LEFM methods are applicable for monolithic ceramics while EPFM methods may be suitable for

CMCs.

Monolithic ceramics are inherently brittle due to their strong bonding and more complicated (less symmetric)

crystal structures. Compared to metallic materials, the mixed ionic and covalent atomic bonding and low-

symmetry crystal structure of ceramics severely limit the opportunity for plastic deformation mechanisms from

dislocation formation, movement, and slip. Monolithic ceramics thus have high strength and stiffness with

much less plastic deformation than metals. As a result, their behavior is primarily linear-elastic, which is one of

the required conditions for LEFM methods.

The other condition for LEFM analysis is the presence of a sharp crack or flaw with a crack tip radius

approaching zero. Monolithic ceramics meet this condition as well, and LEFM has been used successfully to

evaluate monolithic ceramic fracture behavior. Some additional work was necessary to augment LEFM

techniques to handle the difficulties of obtaining sharp starter cracks and maintaining stable crack growth that

occur in brittle ceramics. Nonetheless, the result of the application of LEFM to monolithic ceramics is a

relatively mature state of the art. Techniques exist to determine the single-valued critical fracture toughness and

to measure crack growth resistance behavior of monolithic ceramic materials. Current research is centered on

the further refinement of these techniques (see also the article“Fracture Resistance Testing of Brittle Solids” in

this Volume).

Ceramic matrix composites are being developed in an attempt to increase the toughness and damage tolerance

of ceramic materials. The toughness of CMCs is greater than the monolithic ceramics due to the toughening

(energy-absorbing) mechanisms of microcracking, crack bridging, and crack branching. These toughening

mechanisms enable the CMC to behave in a manner that closely resembles the elastic-plastic behavior of

metals. Although this fact may suggest EPFM as appropriate for the study of CMCs, there are differences in the

deformation mechanism of ductile metals and CMCs that can bring into question the appropriateness of metal-

based EPFM methods for use with CMCs.

Metals dissipate crack-tip energy by the plastic deformation mechanisms of slip, dislocation generation, and

dislocation movement. CMCs, on the other hand, dissipate crack tip energy through crack branching, fiber

bridging, and microcracking. In both cases, energy is dissipated by nonlinear deformation of the body prior to

crack extension. Fortunately, EPFM theory does not depend on the mechanism through which the energy is

dissipated during nonlinear deformation, only on the fact that it does occur. As a result, even though the

deformation mechanisms are very different, EPFM theory is valid for both metals and CMCs. Unfortunately,

the complex nature of the processes that cause the nonlinear fracture behavior in CMCs complicates the

experimental application of EPFM methods to CMCs. The following section describes toughness tests that are

used on monolithic ceramics and CMCs.

Fracture Toughness of Ceramics and Ceramic Matrix Composites

J.H. Miller, Oak Ridge National Laboratory P.K. Liaw, The University of Tennessee, Knoxville

Fracture Toughness Evaluation

This section describes some fracture toughness measurement techniques that are being used on ceramics and

CMCs. The descriptions are organized by specimen type, and they include advantages and disadvantages of

each specimen type as well as experimental control schemes that have been employed on each specimen type.

More detailed information on the fracture toughness testing of monolithic ceramics is also provide in the article

“Fracture Resistance Testing of Brittle Solids” in this Volume.

Single Edge Notch Bending (SENB). The SENB specimen, shown in Fig. 7(a) has a rectangular cross section

with a straight-through saw notch. It is loaded in either three- or four-point bending. The advantages of the

SENB include ease of machining due to simple geometry and ease of use due to simple three- or four-point

bend loading, which uses a fixture loaded in simple compression. The SENB, while easy to machine and test, is

not very stiff. This leads to problems in starting a sharp crack at the end of the saw notch and in achieving

stable crack extension (Ref 58).

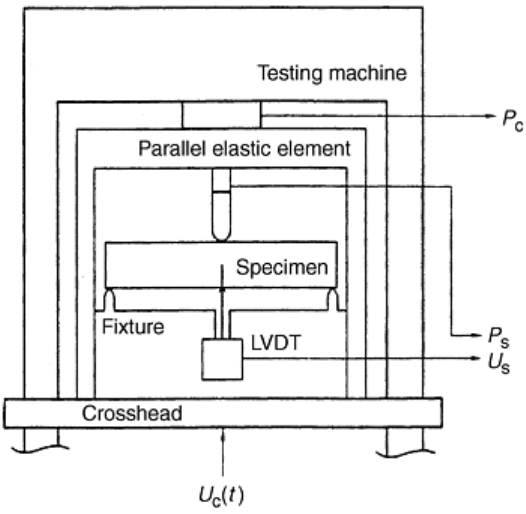
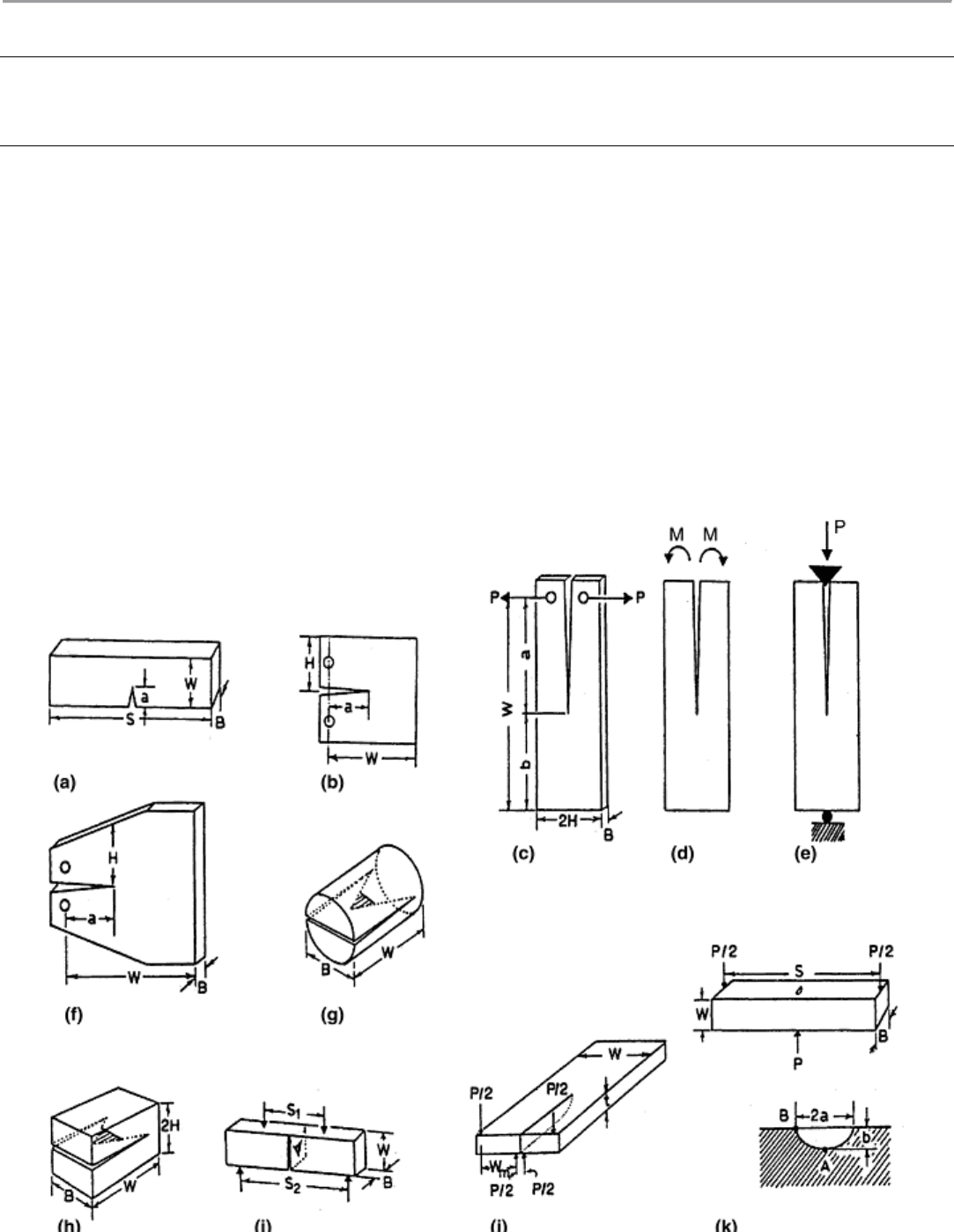
Fig. 7 Fracture toughness specimens. (a) Single edge notch bending (SENB). (b) Compact tension (CT)

or wedge open loaded (WOL). (c) Double cantilever beam (DCB) tensile loading. (d) DCB constant

bending moment loading. (e) DCB wedge loading. (f) Tapered DCB. (g) Chevron notch short rod. (h)

Chevron notch short bar. (i) Chevron notch bending. (j) Double torsion (DT). (k) Three-point bending

with controlled surface flaw (CSF). P, load. Adapted from Ref 58

One method of producing a sharp starter crack in a SENB specimen involves using a hardness indenter to

introduce a surface flaw and forcing the surface flaw to propagate to the outer edges and become a sharp edge

crack. This can be done by loading the indented specimen in compression between two rigid plates. One of the

plates has a single groove, and the indentation crack is positioned over the groove. During the compressive

loading, the surface flaw is subjected to tensile opening stresses due to the groove, while the bulk of the

material is in compression. This tension allows the crack to grow to become an edge crack, while the

compression prevents the crack from propagating in an unstable manner through the specimen (Ref 59).

SENB specimens have been used to measure the fracture toughness of a yttria-partially stabilized zirconia (Y-

PSZ) ceramic at ambient and elevated temperatures (Ref 59). The point of this work was to demonstrate the use

of a new technique of controlling the fracture test in such a way as to promote stable crack growth so that the R-

curve behavior of the ceramic could be measured. Crack mouth opening displacement (CMOD) was monitored

using a laser extensometer, and the CMOD signal was used in the control of the servohydraulic testing machine

in real time. The results of this type of control were positive, and stable crack growth was achieved (Ref 59).

The SENB method has also been improved by a scheme that employs both a method to provide for stable crack

growth and better real-time computer-aided data acquisition (Ref 60). The crack growth stability was

augmented by adjusting the stiffness of the test frame in such a way as to promote stable crack growth. The test

machine stiffness, or compliance, was adjusted by placing a “parallel elastic element” (PEL) supporting

structure between the crosshead and the top of the three-point bend loading fixture (Fig. 8). The optimum

compliance for stable crack growth was determined by theoretical analysis and experimentation. During testing,

the compliance of the specimen and the test frame were continuously monitored in real time through the use of

a computer. The real-time load and displacement data form various points in the test system, as presented in

Fig. 8. After the optimum PEL compliance was determined, and stable crack growth was achieved, the R-curves

of the test materials were measured. The real-time load and displacement (and, therefore, compliance)

monitoring, along with compliance versus crack length calibrations and the stress intensity calibrations, allowed

the crack length, crack velocity, and stress intensity level to be calculated in real time during the test (Ref 60).

Fig. 8 Schematic of the compliance-controlled three-point bending test with a parallel elastic element

(PEL). Adapted from Ref 60

Compact Tension (CT). The CT sample is a common specimen in fracture mechanics tests. It is loaded in

tension, but the primary stress is due to bending because the load line is offset from the crack front (Fig. 7b).

Relatively stable crack propagation is possible with the CT specimen if a stiff testing machine is used. Also, a

variant of the CT can be used in a wedge-opening mode to increase the stable crack growth capability (Ref 49).

The stable crack growth capability allows for the generation of R-curves using the CT specimen (Ref 61). The

CT specimen is complicated to machine in ceramic materials, and precracking the can be difficult (Ref 58).

In addition to R-curve measurements of monolithic ceramics (Ref 61), R-curves have been measured in CMCs

using the CT specimen. CT specimens have been employed to generate R-curves for CMCs in an attempt to

analyze the crack-face fiber-bridging stress field (the stress field in the wake of the crack that is due to fiber

bridging). The crack-face fiber-bridging stress is evaluated by comparing the experimentally measured

compliance versus crack length, which includes the contribution of the fiber bridging, to the compliance versus

crack length data calculated from the elastic properties of the CMC, assuming no fiber bridges are present (Ref

62).

The J-parameter toughness has also been measured using a CT specimen (Ref 63). A J-based testing technique

has been developed for CMCs using the CT specimen geometry. The concept of the J-parameter is used to

determine the contribution of the process zone (the contribution of, for instance, fiber bridging and crack

branching, analogous to the plastic zone in metals) to the toughness of the CMC. The J-parameter contribution

of the process zone is determined using Eq 12 (Ref 63):

∞

+ J

tip

= 0

(Eq 11)

where J

∞

is the far field J, J

is the process zone J (analogous to J

plastic

), and J

tip

is the crack tip J. Also, it is

assumed that elastic action takes place at the crack tip, so J

tip

can be calculated from K

tip

using Eq 9. The value

of J

∞

was experimentally calculated from measurements using CT specimens with two different crack lengths,

and a

. The value J

∞

is the far-field J, as the crack grows from a

to a

. Therefore, J

was calculated from Eq

12 (Ref 63).

The J-parameter toughness has also been measured with CT specimens in a study conducted to compare the

toughness values of woven fabric-reinforced CMCs with different interphases (Ref 64). The value of J,

calculated from the experimental load-displacement curves at the point of maximum load, corresponds to

macrocrack initiation in the process zone (Ref 64).

Double Cantilever Beam (DCB) Testing. The DCB specimen looks like a long CT specimen. It can be loaded in

three different configurations: direct tension, constant bending moment, and wedge opening. The tensile-loaded

DCB is very similar to the CT in all respects (Fig. 7c), but the DCB geometry is better than the CT for growing

stable cracks. The tapered DCB, shown in Fig. 7(f), provides a constant K

level with crack growth. Another

DCB variant that provides a constant K

level with crack growth is the moment-loaded DCB (Fig. 7d).

Unfortunately, the fixturing that applies the moment to the arms of the DCB can be difficult to deal with. The

final DCB variant is the wedge-loaded DCB (Fig. 7e). The wedge loading allows the specimen to be tested in

simple compression. The wedge-loaded DCB specimen was first used in ASTM method E 561. All the DCB

variants may need side grooves to keep the crack moving down the center of the specimen. The grooves

complicate the K-calibration, and machining damage in the groove can affect the crack extension (Ref 58).

Specimens very similar to the constant bending moment DCB, called the Browne/Chandler test specimen, have

been employed in the determination of R-curves for monolithic ceramics (Ref 65). The Browne/Chandler

specimen geometry, shown in Fig. 9, applies loads to the outside corners of a specimen, supported by a stiff

solid base, that has a rectangular cross section and a vertical edge crack. This loading induces a bending

moment around the center of the specimen, which causes the crack to open and extend. If the applied load were

held constant, the stress intensity at the crack tip would decrease as the crack grew. Therefore, the load must

increase in order for crack growth to continue, which prevents unstable fracture. Also, crack-guiding side

grooves are not necessary because the compressive stress parallel to the crack keeps the crack growing down

the center of the specimen. Unfortunately, the Browne/Chandler test geometry does not lend itself to an

analytical solution of K-calibrations for all crack lengths. As a result, stress intensities have to be estimated

using numerical methods at both very short and very long crack lengths (Ref 65).

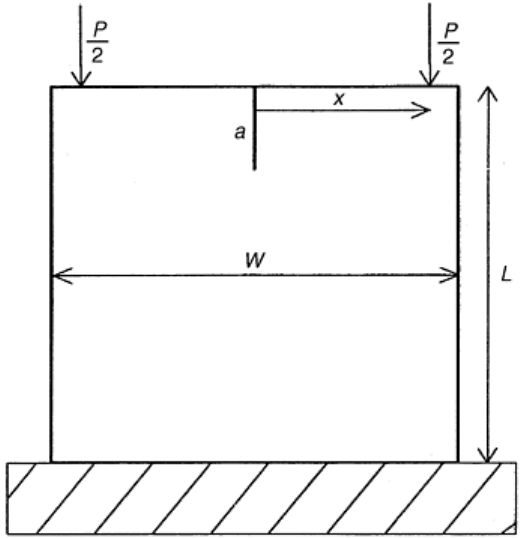
Fig. 9 Schematic sketch of the Browne/Chandler test geometry. Adapted from Ref 65

Chevron Notch Methods (CHV). The chevron notch is used in three specimens (Ref 58, 66). There is a short

rod CHV (Fig. 7g), a short rectangular bar CHV (Fig. 7h), and a bend bar with a rectangular cross section and a

chevron notch (Fig. 7i). The chevron notch geometry, due to the increase in the width of the crack surface

during crack extension, forces rising R-curve behavior in ideally brittle materials. This means that after crack

initiation, the crack front is stable and is always ideally sharp. Stable crack growth is not always found in

practice due to the fact that excess stored energy in the specimen can overcome the geometrical tendency to

force rising R-curve behavior. In these cases, the excess stored energy can cause catastrophic failure in the

specimen. As a result, a stiff specimen geometry and test machine are important (Ref 58, 66).

One of the main advantages of the chevron notch geometry is the fact that it is possible to calculate the fracture

toughness based on the maximum load and the specimen dimensions alone for ideally brittle materials. Due to

the shape of the growing crack, the geometry factor, Y, goes through a minimum as crack growth occurs. The

minimum in the geometry factor corresponds to the maximum load in the test. This unique fact allows the

fracture toughness to be calculated without knowledge of the crack length (Ref 58). However, calculation of

fracture toughness from the maximum load and specimen dimensions does not work for materials that exhibit

natural rising R-curve behavior. In these cases, the minimum in the geometry factor versus crack length does

not correspond with the maximum load, and the crack length must be known to calculate the fracture toughness

(Ref 58).

The chevron notch specimen has become almost a standard for measuring fracture toughness in ceramic

materials (Ref 58, 67, 68, and 69). In fact, the only ASTM standard test method for determining the fracture

toughness of ceramics, B 771-87, uses the short rod and short bar chevron notch specimen (Ref 69). In addition,

chevron notch samples are used in ASTM PS70, “Provisional Test Method For Determining The Fracture

Toughness of Advanced Ceramics at Ambient Temperatures” (Ref 66). Also, researchers with new fracture

tests use chevron notch results to benchmark the results of the new tests (Ref 61, 70, and 71).

Double Torsion (DT). The DT specimen, shown in Fig. 7(j), is a flat plate with a longitudinal through crack that

is loaded in torsion. The torsional loading applies a bending moment that opens the crack on the tensile surface

and closes the crack on the compressive surface. The applied moment causes the K

level to be constant over a

wide range of crack length. It also causes the crack front to be significantly curved between the top and bottom

surfaces. This curvature of the crack front opposes the straight-through crack assumptions used in the stress

intensity calculation, and causes errors in the toughness calculation (Ref 58).

Indentation Techniques. Indentation fracture toughness techniques utilize a sharp crack introduced in the

material by a Knoop or a Vickers indenter. The Knoop indenter causes a single crack, which makes it attractive

for use in fracture mechanics methods involving a surface flaw. The Vickers indenter creates two cracks that

are mutually perpendicular to each other. Both indentation methods can produce reproducible surface cracks,

but the residual stress field from the indentation is complicated and must be removed or accounted for in the

analysis (Ref 58, 72, 73, and 74)

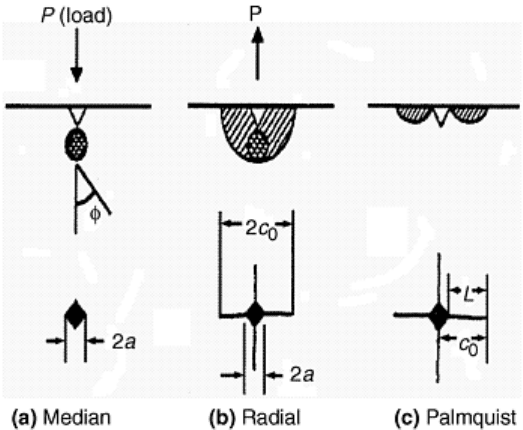
Indentation creates subsurface cracks, due to tensile stress formation, just below the contact point of the

indenter. These subsurface cracks are called median cracks. As the indenter is removed, the median cracks

become unstable and grow to the indented surface. The resulting crack is called a radial crack. In some high-

toughness brittle systems, small radial surface cracks can form prior to subsurface median crack formation.

These shallow surface cracks are called Palmqvist cracks. Schematic drawings of median, radial, and Palmqvist

cracks are shown in Fig. 10 (Ref 58, 72, 73, 74).

Fig. 10 Schematic diagram illustrating types of crack systems formed around an indentation. Adapted

from Ref 42, 71

There are three indentation-induced crack techniques for measuring fracture toughness in ceramics: the

controlled surface flaw (CSF) technique, the indentation microfracture (IM) technique, and the indentation

strength in bending (ISB) technique. The CSF method can provide a quantitative measure of the fracture

toughness because the stress intensity around the surface flaw is well known, but the IM and ISB provide only

estimates based on empirical expressions (Ref 58, 72, 73,and 74).

The CSF method involves testing a bending specimen with an elliptical surface crack induced by a Knoop

indenter (see Fig. 7k). The Knoop indenter is used to create a subsurface median crack. Then, the surface is

carefully polished to remove the indent and the associated residual stresses. The polishing also reveals the

median crack to the surface, and an elliptical surface flaw with no residual stress is the result. When the

specimen is tested, the surface flaw causes the initiation of fracture, and K

can be calculated based on the

applied loads and the crack size (Ref 58, 73).

The ISB is similar to the CSF technique (Ref 73). The ISB approach uses the Vickers indenter to induce

median-radial cracks on the tensile surface of a bending specimen. Through manipulation of the K-calibrations

for the crack system, which account for both the applied and residual stresses, it is possible to develop an

expression that relates the fracture stress in bending and the indentation load to the fracture toughness.

Therefore, the ISB method involves calculating the fracture toughness based on the indentation load and the

experimental fracture stress from a bending test. This method has the advantage of being relatively simple to

conduct, and there is no need to measure crack size. The disadvantage comes from the use of empirical factors

in the manipulation of the K-calibrations that are used to develop the expression relating the indentation load

and fracture stress to the fracture toughness. This causes the fracture toughness measured by this method to be

only an estimate (Ref 58, 73).

Another fracture toughness specimen that uses a Vickers hardness indenter to introduce sharp cracks into a

ceramic material is the miniature disk bend test (MDBT) specimen (Ref 70, 75). These specimens are 3 mm

(0.1 in.) in diameter and range in thickness from 200 to 700 μm. Vickers indentation cracks are introduced into

the center of the tensile side of the specimen. The disk specimen is loaded in a ring-on-ring bending mode,

schematically shown in Fig. 11. The MDBT is similar to the ISB test. In both experiments, the fracture stress