ASM Metals HandBook Vol. 17 - Nondestructive Evaluation and Quality Control
Подождите немного. Документ загружается.

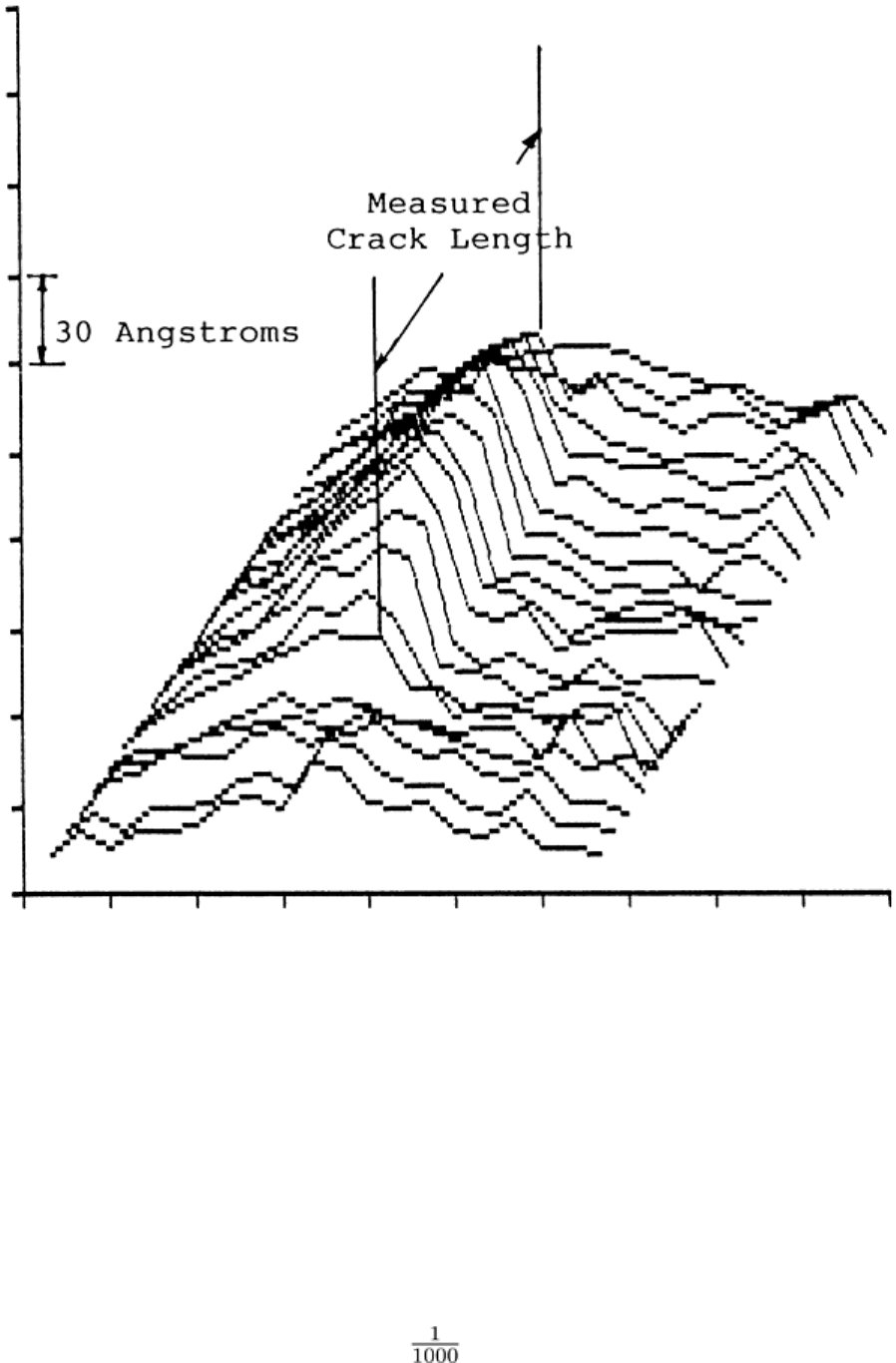
Fig. 18 Results of heterodyne holographic interferometry showing minute displacements adjacent to a surface-
breaking crack in a nickel-base superalloy.
A dual-reference, double-exposure recording technique was used where a bending stress was applied to the cracked
specimen between the holographic double exposures. The resulting hologram was reconstructed with a 100-kHz
frequency difference imposed between the two reconstructing beams so that the intensity of the image varied sinusoidally
at a 100-kHz rate.
Although each point on the image varies in intensity at 100 kHz, the relative phase of these oscillations varies from point
to point on the image, depending on the amount of displacement recorded between holographic exposures. Therefore,
when a small detector is scanned over the image, the phase of its output signal can be compared with some reference
phase from another (fixed) point on the object, as shown in Fig. 19. Because a phase difference of 360° corresponds to a
single interferometric fringe, the resulting map of phase difference is directly related to surface displacement. Electronic
phase measurement accuracy to 0.36° corresponds to of one fringe. Uncertainties resulting from the effects of
speckle and the environment do not permit meaningful measurements below this level.
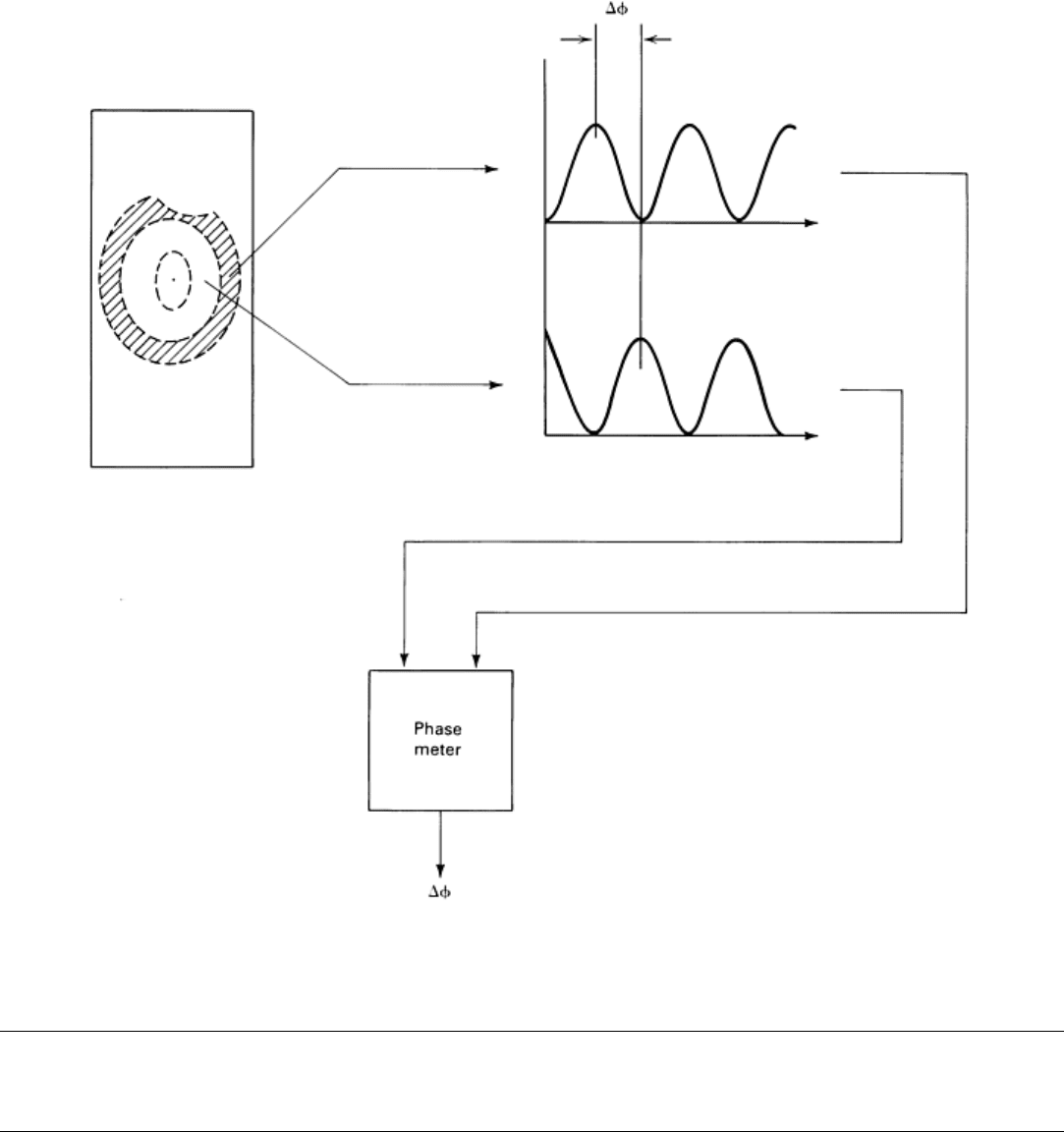
Fig. 19 Dual-detector readout for heterodyne holographic interferometry
Optical Holography
Revised by James W. Wagner, The Johns Hopkins University
Vibration Analysis of Turbine and Propeller Blades
Vibration analysis using routine optical holographic techniques can significantly contribute to the inspection and
evaluation of turbine and propeller blades in both the design and manufacturing stages (Ref 21, 22, 23). A recommended
approach to turbine blade evaluation is the simultaneous holographic recording of both sides of the blade as it is excited
into vibration with shaker tables (at frequencies generally limited to less than 50 kHz), air-horn vibrators, electromagnetic
drive systems (where blade materials permit), or piezoelectric transducers (bonded or clamped to the blade). By the use of
simultaneous recording, information over and above the straightforward recording of vibrational mode patterns can be
obtained. Differences in the mode patterns for the two sides of the blade will suggest an absence of structural integrity at
those points, while differences in vibrational amplitude (for example, in hollow blades) can offer a relative measure of
blade wall thickness (that is, a check on cooling passage alignment).
Holographic techniques are applicable to all types of blades, both solid and hollow and of almost any size and shape, as
well as entire turbine wheels or propeller assemblies. However, some experimental problems may be encountered in the
dual-sided simultaneous recording of large parts, thus necessitating a two-step procedure. (Caution must then be exercised
to ensure that the driving frequency and amplitude are identical for both of the holograms.)
For blades of reasonable size (up to perhaps 100 to 125 mm, or 4 to 5 in., chord by 460 to 610 mm, or 18 to 24 in.,
length), the simultaneous recording of both sides of the blade can be accomplished by a standard holographic system
through the use of mirrors (taking care to maintain the path lengths of the reference beam and the object beam within the
coherence length of the laser). An alternative method of simultaneous recording is to use a dual holographic system. A
reasonably compact (1.2 × 1.2 m, or 4 × 4 ft) setup of a dual system can be assembled by first splitting the incoming laser
beam into two beams (one for each hologram), each of which is subsequently split again into a reference beam and an
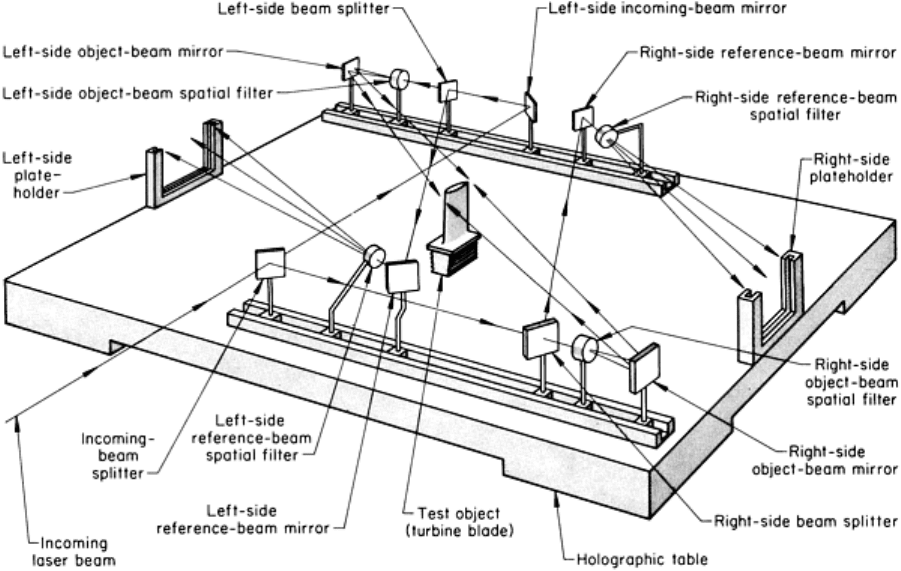
object beam by a symmetrical arrangement of the various optical components, as shown in Fig. 20.
Fig. 20
Schematic of a compact dual holographic system for the simultaneous recording of both sides of a
turbine blade
An example of the type of data that can be obtained by simultaneous recording is illustrated in Fig. 21, which shows
interferograms of both sides of a hollow blade whose pressure wall is about 250 to 375 μm (10 to 15 mil) thinner than its
suction wall. Although the two interferograms in Fig. 21 were not recorded simultaneously, identical ultrasonic
frequencies and amplitudes were used to excite the blade during recording. This produced fringe patterns in solid regions
(the leading and trailing edges) that are essentially the same on the front and back surfaces. However, over the hollow
region of the blade, the vibrational amplitude on the pressure side is measurably larger (each fringe represents
approximately 0.30 μm, or 12 μin., of out-of-plane displacement) than that on the suction side. In addition, the vibrational
amplitude increases from the root to the tip of the blade, indicating a decreasing wall thickness for both the pressure and
the suction sides. Pressure stressing is an alternative to vibrational excitation for the holographic inspection of hollow
turbine blades for detecting weakened structural characteristics.
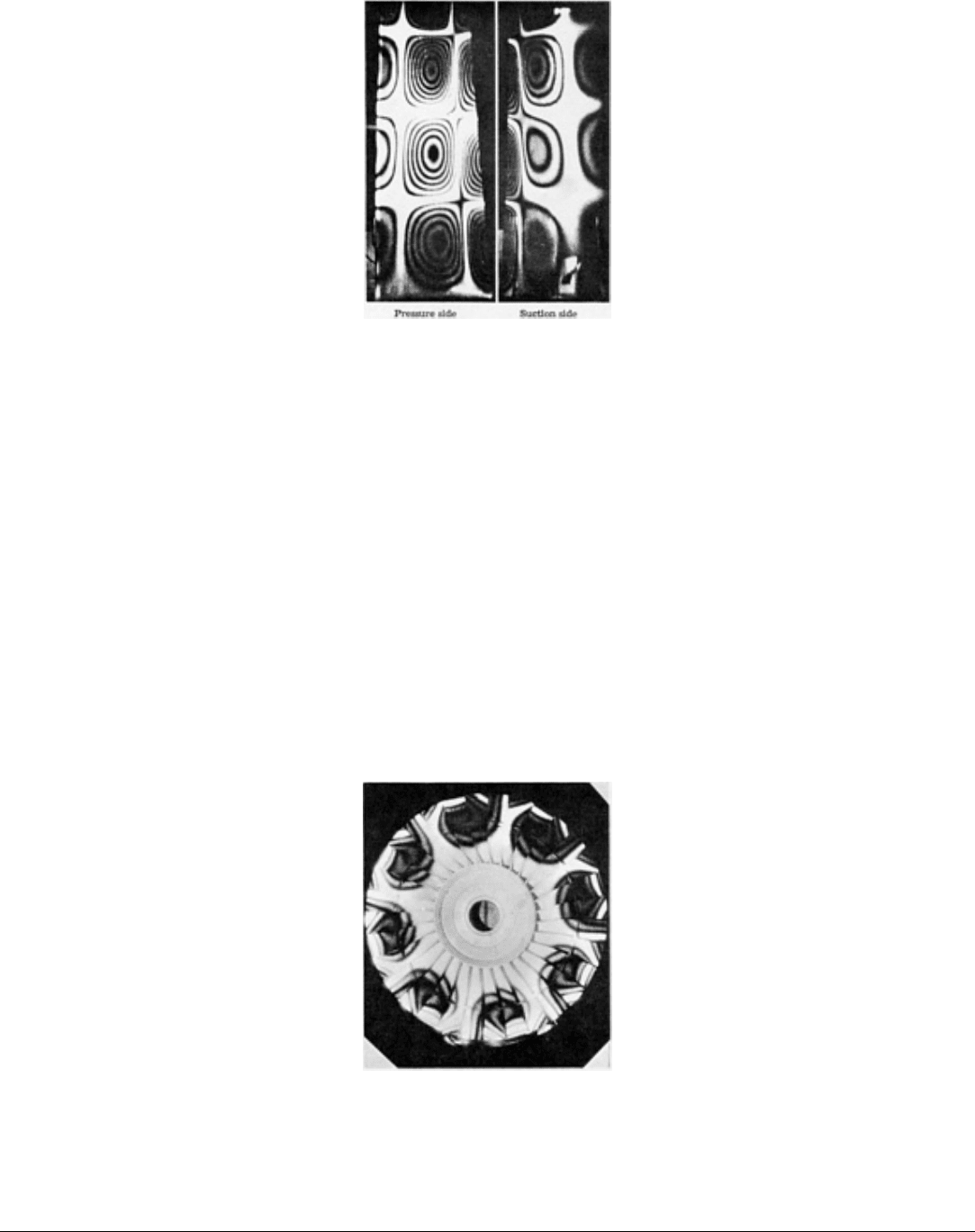
Fig. 21 Continuous-
exposure interferograms of both sides of a turbine blade that were recorded while the blade
was being vibrated. The interferograms were recorded at identical driving frequencies and amplitudes, such as
might be obtained with the compact dual holographic system shown in Fig. 20. The holocameras used helium-
neon lasers.
To establish the most favorable driving force and frequency for a particular blade, a somewhat extensive series of tests is
recommended (perhaps including sectioning of the blades) to correlate the results and to establish standards of
acceptance. Although such preliminary testing may be costly, for large production runs this inspection procedure could be
valuable for accurate wall thickness gaging in thin-wall structures, in which standard ultrasonic pulse-echo techniques are
the most difficult to effect.
The ability to observe one entire surface of a large test object at one time, rather than in a series of limited views, is one of
the most important benefits of using holography for nondestructive inspection. One entire surface of an 810 mm (32 in.)
diam jet engine fan assembly can be recorded in a single hologram (Fig. 22), considerably facilitating the performance of
a vibrational-mode analysis that alternatively would require transducers placed over the entire assembly.
Fig. 22 Continuous-ex
posure interferogram of one side of an 813 mm (32 in.) diam jet engine fan assembly
that was excited at a frequency of 670 Hz showing a 4-nodal-diam mode of vibration.
The holocamera used a
helium-neon laser.
References cited in this section
21.
R. Aprahamian et al.,
"An Analytical and Experimental Study of Stresses in Turbine Blades Using
Holographic Interferometry," Final Report AM 71-5 under NASC Contract N00019-70-C-0590, July 1971
22.
J. Waters et al., "Investigation of Applying Interferometric Holo
graphy to Turbine Blade Stress Analysis,"
Final Report J990798-13 under NASC Contract N00019-69-C-0271, Feb 1970 (available as AD 702 420)
23.
Proceedings of the Symposium on Engineering Applications of Holography, Society of Photo-
Optical
Instrumentation Engineers, 1972
Optical Holography
Revised by James W. Wagner, The Johns Hopkins University
Holographic Contouring
When evaluating the shape of an opaque object, interference fringes are generated on the object that represent depth or
elevation contours. These fringes are usually generated most readily by optically interacting two images of the object that
have been slightly displaced from one another, although one method simply requires projecting onto the object a set of
fringe surfaces whose normals are roughly parallel to the line of sight of the viewer. The three principal techniques for
holographic contouring are discussed below.
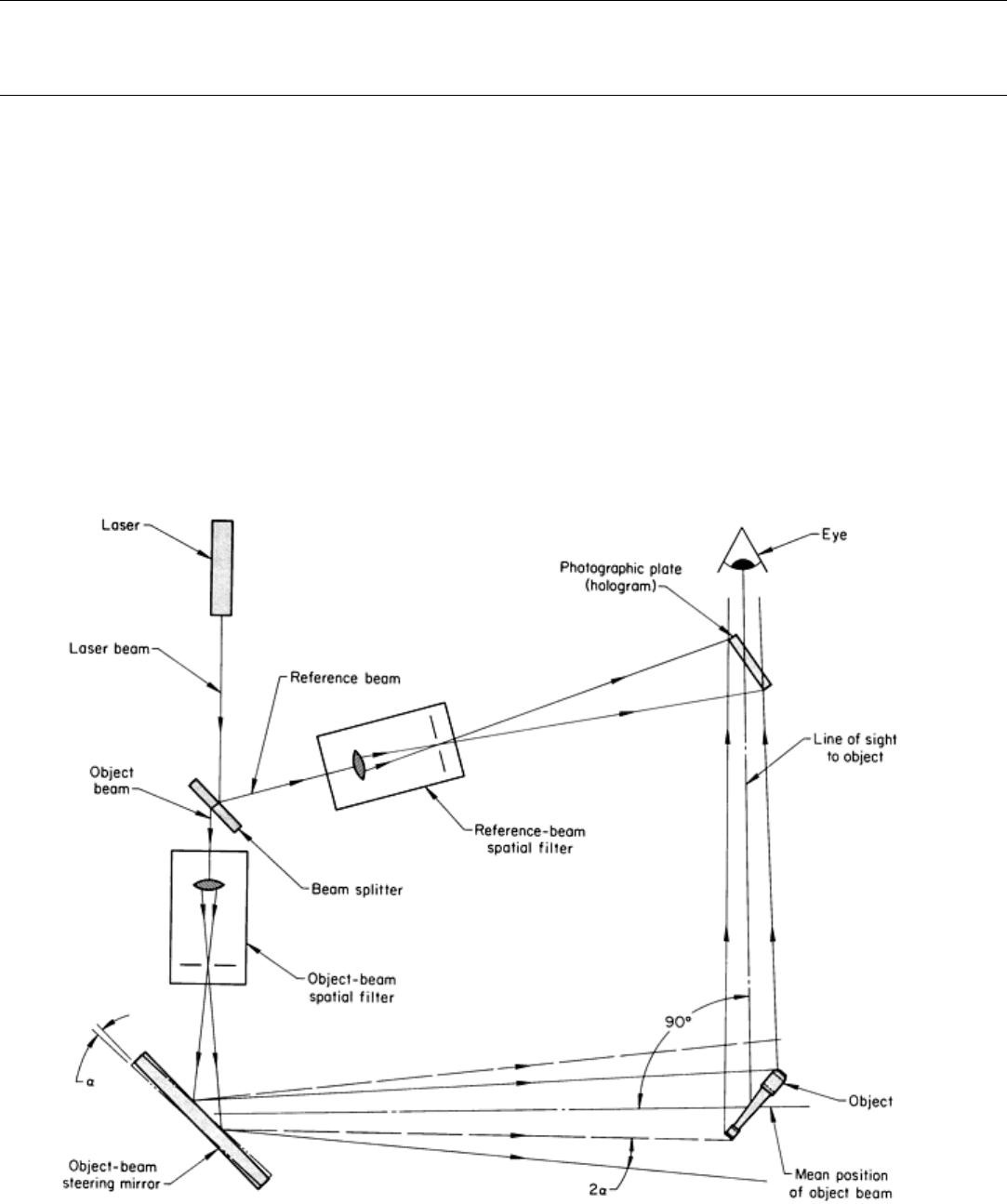
Multiple-Source Contouring. In the multiple-source technique (which is not really holographic contouring, but rather
holographic interferometry), an optical system is used that incorporates a rotatable mirror for steering the object beam
(Fig. 23). A fixed contour map of the object can be generated with this system by making two holographic exposures on
the photographic plate that differ only by a slight rotation of the object beam steering mirror, which shifts the virtual
image of the object-illumination source.
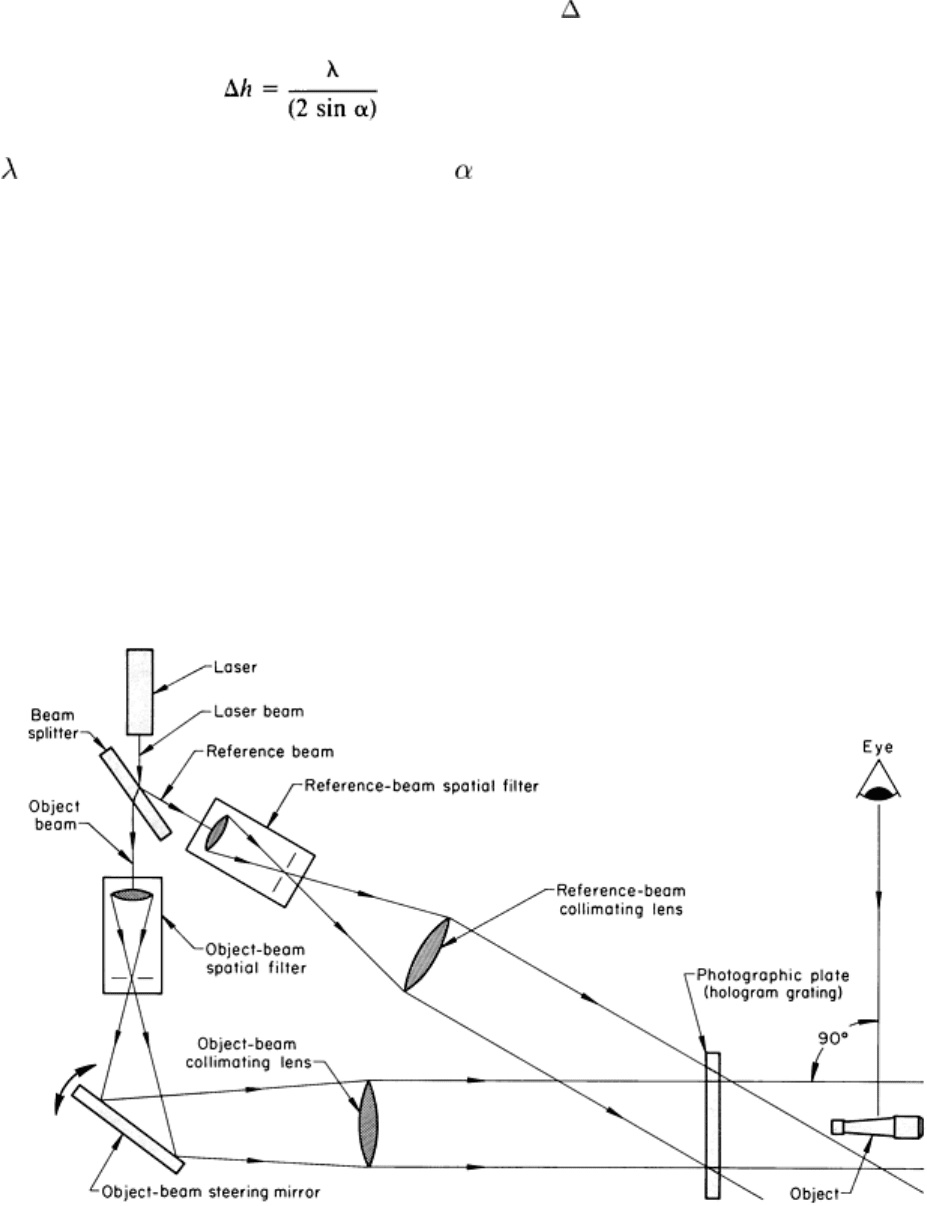
Fig. 23 Schematic of an optical system for multiple-source holographic contouring. Source: Ref 24
Alternatively, a single holographic exposure can be made with the optical system illustrated in Fig. 23, and after
processing and repositioning in the plate holder, a real-time contour map of the object can be generated by a similar slight
rotation of the object beam steering mirror. In either case, when the system is suitably arranged for proper viewing, the
altitude contours on the map of the surface have a separation distance, h, given by:
(Eq 10)
where is the wavelength of the laser light source and is the angle of mirror rotation. Suitable arrangement of the
system requires that the line of sight through the hologram to the object be in the same plane as the angle of rotation of
the object beam and be perpendicular to the mean position of the reflected object beam, as shown in Fig. 23. The
multiple-source technique has the advantage of providing for an almost unlimited range of contour separations. However,
because of the orthogonal illumination and observation directions, shadowing effects are a handicap, and no reentrant
surfaces can be contoured by this technique.
Real-Time Contouring. An optical system for the real-time generation of a contour map by the multiple-source
technique is shown in Fig. 24. This system uses collimated reference and object beams to generate hologram gratings by
means of two successive exposures at different settings of the object beam steering mirror. Subsequent illumination of a
hologram grating by only the collimated reference beam produces a simultaneous reconstruction of both the original and
the rotated object beams, which propagate and interfere beyond the hologram grating. The spacing of the resulting
vertical-standing-wave interference planes is given by Eq 10 and can be readily calibrated by direct measurement of the
fringe field on the hologram itself or by exposing a sheet of film oriented at right angles to the propagation direction of
the reconstructed object beams and measuring the fringes recorded there. Finally, suitable orientation of the object
anywhere in the reconstructed object beams yields a real-time contour map when viewed at right angles to the object
beams.
Fig. 24 Schematic of a real-time optical system for holographic contouring using the multiple-source
technique.
See text for discussion.
Example 8: Real-Time Contouring of Electroplated Copper Foil Using a Multiple-
Source Holographic System.
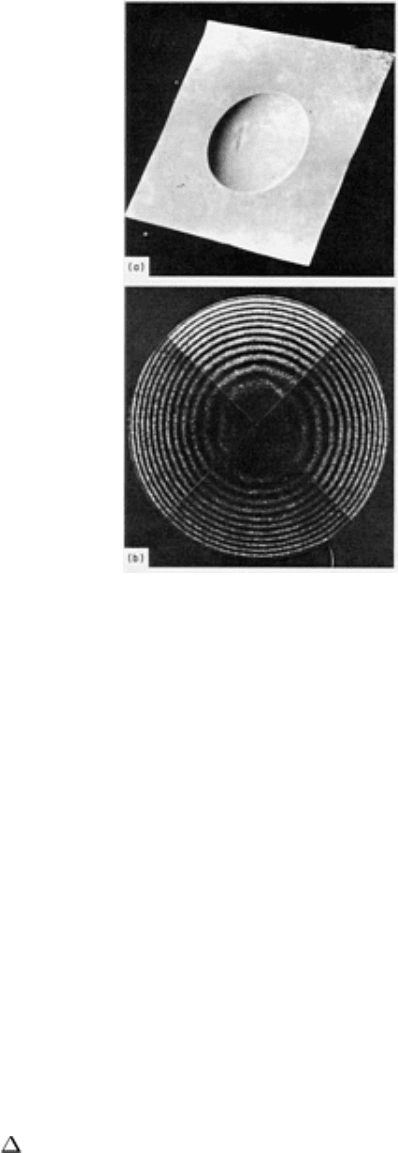
The multiple-source system shown in Fig. 24 was used in a study of the tensile properties of electrodeposited metal foils.
In the course of this study, the shapes of permanently bulged specimens (Fig. 25a) of copper foil 0.0889 mm (0.00350 in.)
thick were measured. The bulges, which were 29 mm (1.14 in.) in diameter, ranged from a few millimeters to half a
centimeter in height; therefore, a range of sensitivities was needed.
Fig. 25 Example of real-time contouring using the multiple-source holographic system illustrated in Fig. 24
. (a)
Photograph of bulged specimen of electrodeposited cop
per foil 0.0889 mm (0.00350 in.). The bulge was 29 mm
(1.14 in.) in diameter. (b) Composite of two contour maps. The specimen was rotated between recordings to
expose both sides of the bulge. The fringe separation in this map is 0.229 mm (0.00902 in.).
Because of shadowing, only one side or half of the specimen could be contoured at a time, but multiple recordings with
the specimen revolved to expose all sides were made and are shown combined in Fig. 25(b). For this contouring, the
fringe separation was 0.229 mm (0.00902 in.); other gratings were made for separations up to 5 mm (0.20 in.) and down
to 0.1 mm (0.004 in.). Because no high-precision repositioning is required and the reconstructed fringe field is permanent,
this represents a convenient and practical means of real-time contouring. However, if a single exposure is recorded in the
hologram and is accurately repositioned to provide an initially clear field with appropriately balanced beams, simple
adjustments of the object beam steering mirror provide the capability of a real-time variation of the sensitivity (fringe
separation) of the contour map when needed.
Multiple-Wavelength Contouring. Constant-range contours measured from an origin at the center of the hologram
can be generated with the multiple-wavelength technique (Ref 24). Because a change in wavelength produces changes in
position as well as in magnification and the phase of the object images, the multiple-wavelength technique is more
complicated than the multiple-source technique. One configuration has been demonstrated in which both the illumination
and viewing directions lie along a line through the center of the hologram and the object (Ref 25). This configuration
yields fringes with separations, r, given by:
(Eq 11)
where λ
1
and λ
2
are the wavelengths of the two laser light sources. Because λ
1
and λ
2
are not arbitrarily variable, only
discrete sensitivities are available, all of which are quite high. However, because of the more normal illumination
conditions, the problems of shadowing associated with the multiple-source technique are completely eliminated.
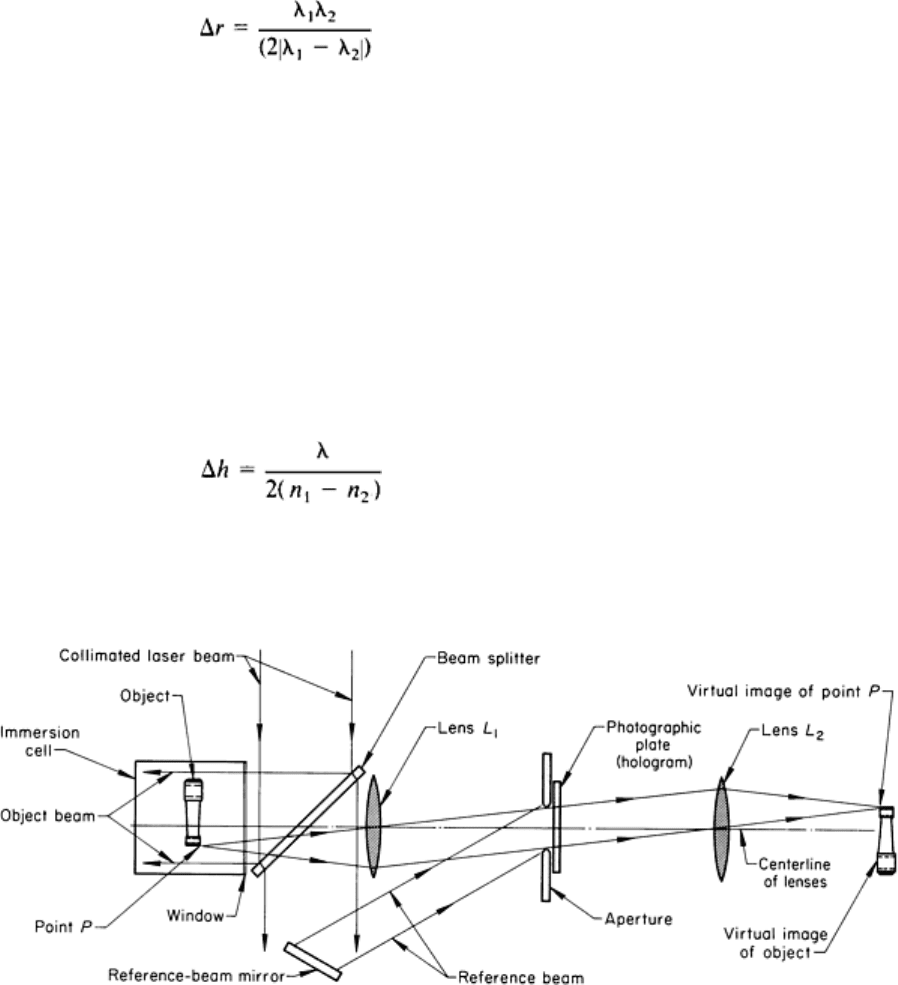
Multiple-Index Contouring. In this technique, the gaseous or liquid medium surrounding an object is changed
between successive exposures of the hologram (for double-exposure interferometry) (Ref 25, 26). This changes the
optical-path length by slightly altering the index of refraction of the medium and is most readily accomplished by the use
of an immersion cell with windows through which the object can be illuminated and viewed simultaneously. In the optical
system for the multiple-index technique (Fig. 26), lenses L
1
and L
2
act as a telescope with its viewing direction normal to
the cell window. The aperture between the lenses is used to limit aberrations. The hologram can be constructed at any
plane to the right of the beam splitter (for example, at the aperture, as shown in Fig. 26). When this system is used, the
contour separation distance, Δh, is given by:
(Eq 12)
where λ is the wavelength of the laser light source and n
1
and n
2
are the values of the index of refraction of the cell media
used for the two exposures.
Fig. 26 Schematic of the optical system for multiple-index holographic contouring
Example 9: Multiple-Index Holographic Contouring Used to Examine Wear of
Articulating Surfaces of an Artificial Knee Implant.
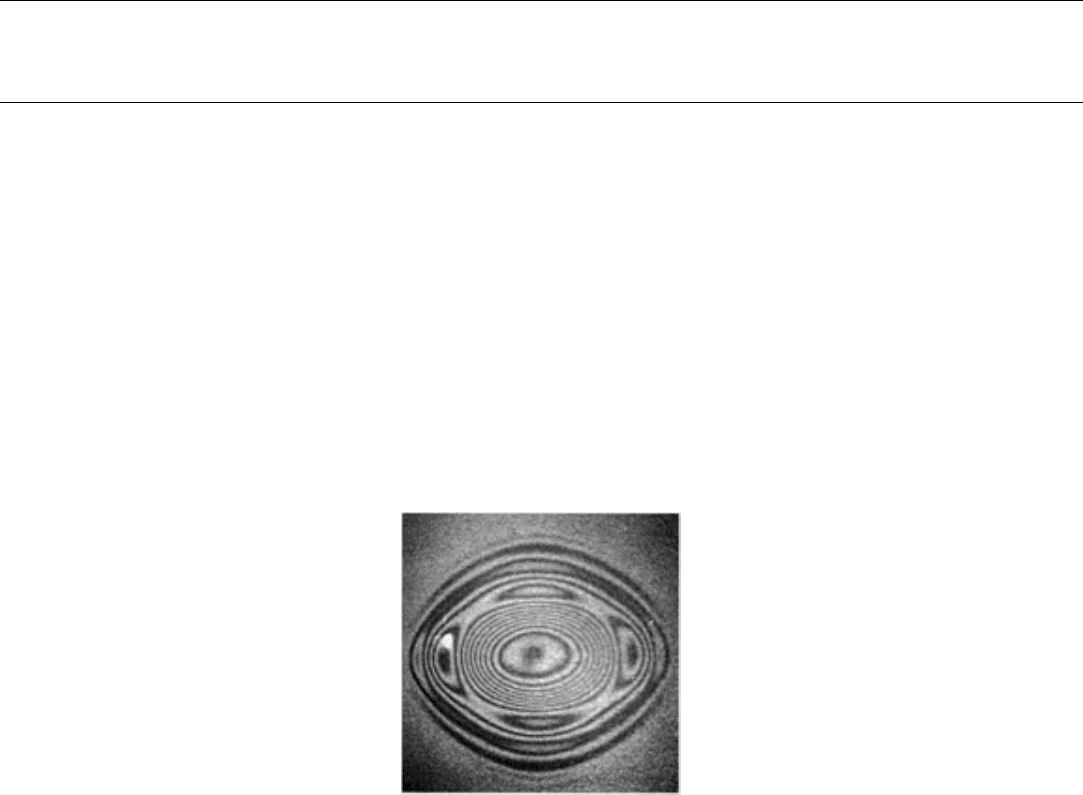
Figure 27 shows the results of a multiple-index holographic-contouring technique applied to the lower (tibial) component
of an artificial knee implant (Ref 27, 28). The material is a high-density polymer, and the intent of the holographic
contouring was to examine the articulating surfaces of these two sockets for wear resulting from in vitro testing. Because
wear tracks are not visible from the interferometric images, high-sensitivity heterodyne holographic techniques were
again applied. Figure 28 shows the results of the heterodyne analysis over a region of one of the knee sockets. From these
scans a gouge in the material is clearly observed; the details are revealed upon further amplification of the phase-
difference signal (Fig. 28b).
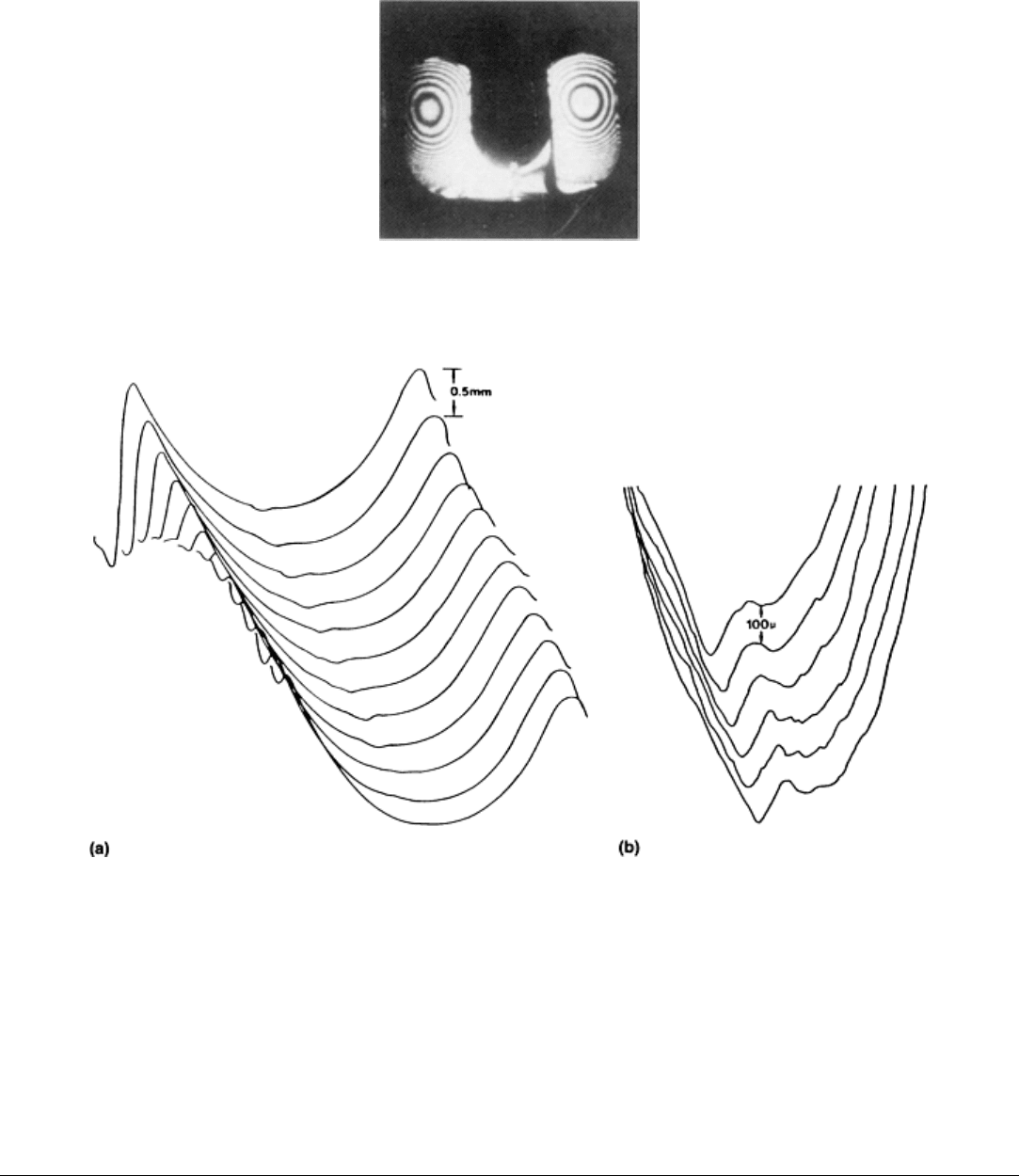
Fig. 27 Dual refractive index contour hologram of an artificial knee implant component
Fig. 28
Results of heterodyne holographic analysis. (a) Surface gouge in a portion of an artificial knee implant.
(b) Higher-resolution scanning that reveals details of the gouge
Multiple-Index Method Versus Multiple-Wavelength Method. The multiple-index technique offers results
equivalent to those of the multiple-wavelength technique. (Note that if the effective wavelengths in Eq 11 are defined as
λ
1
= λ/n
1
and λ
2
= λ/n
2
, Eq 11 becomes Eq 12.) The two techniques have the same types of restrictions and advantages
with regard to sensitivity, but the multiple-index optical system is easier to arrange. Both techniques can be performed in
either the double-exposure or the real-time mode.
References cited in this section
24.
B.P. Hildebrand and K.A. Haines, Multiple-Wavelength and Multiple-
Source Holography Applied to
Contour Generation, J. Opt. Soc. Am., Vol 57 (No. 2), 1967, p a155-162
25.
J.S. Zelenka and J.R. Varner, Multiple Index Holographic Contouring, Appl. Opt., Vol 8 (N
o. 7), 1969, p
1431-1434
26.
T. Tsuruta, N. Shiotake, J. Tsujuichi, and K. Matsuda, Holographic Generation of Contour Map of
Diffusely Reflecting Surface by Using Immersion Method, Jpn. J. Appl. Phys., Vol 6, 1967, p 661-662
27.
J.W. Wagner, High Resolut
ion Holographic Techniques for Visualization of Surface Acoustic Waves,
Mater. Eval., Vol 44 (No. 10), 1986, p 1238-1242
28.
J.W. Wagner, Examples of Holographic Versus State-of-the-
Art in the Medical Device Industry, in
Holographic Nondestructive Testing (NDT)--
Status and Comparison With Conventional Methods: Critical
Review of Technology, Vol 604, Conference Proceedings, Los Angeles, CA, Society of Photo-
Optical
Instrumentation Engineers, 1986
Optical Holography
Revised by James W. Wagner, The Johns Hopkins University
Characterization of Composite Materials
The high-speed pulsed holographic interferometry of transient acoustic waves is used to help determine material
properties and to identify certain types of defects in graphite-reinforced epoxy laminate sheets (Ref 29).
Example 10: Holographic Interferometry of Composite Sheet Material.
Large-amplitude acoustic waves were generated in the sheet materials by direct laser pulse excitation for thin sheets or by
laser detonation of a very small chemical explosive in thicker ones. Double-pulsed holographic exposures were made,
with the first exposure at the instant of excitation and the second exposure occurring after several microseconds of delay.
The resulting interferograms are shown in Fig. 29.
Fig. 29 Double-exposure pulsed hologram showing displacements associated with large-
amplitude acoustic
waves traveling in a centrally excited composite sheet
In this case, the composite sheet was six plies thick, with the fibers running in only one direction in each ply. The ply
orientation stacking sequence was 0
2
-90
4
-0
2
.
From the holographic reconstruction, one observes flexural waves (asymmetric Lamb waves) emanating from the point of
excitation. They are clearly least attenuated in the directions of the reinforcing fibers. From their velocities, one can
compute the flexural stiffness and estimate the effective Young's modulus as a function of direction in the material.
Again, in cases where features smaller than a single fringe must be resolved or when it is not practical to use high
excitation forces, phase stepping or heterodyne techniques can be applied (Ref 30).
Figure 30 shows the displacements associated with flexural wave propagation in a sheet where the peak displacement
amplitude is about one-third of an interferometric fringe. These displacement data were extracted using phase-stepping
methods on a hologram in which not even a single complete fringe was observed. With phase stepping, sensitivities to
