ASM Metals HandBook Vol. 17 - Nondestructive Evaluation and Quality Control
Подождите немного. Документ загружается.

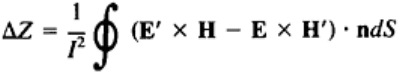
assist in design processes, for which state-of-the-art supercomputers may be available. In addition to progress in
hardware, researchers are applying certain new software techniques to eddy current problems. With new software running
on powerful computers, the difficulty in numerical analyses is becoming obsolete. Recent experimental advances have
also proved beneficial. Emphasis is placed, more than before, on making characterizable probes and on operating them in
broad frequency ranges. In fact, no fully quantitative eddy current methods can be established without these two
capabilities. Incidentally, all existing eddy current POD models discussed in the literature rely on some type of
experimental input for noise characterizations (Ref 30, 34).
Reciprocity Relation. On the theoretical side, the appearance of a reciprocity formula, proposed by B.A. Auld (Ref
31), has been regarded as a breakthrough in that it provides a formalism for interpreting measurements in realistic
experimental geometries. The formula gives the impedance change ΔZ due to the presence of a flaw in the form:
(Eq 6)
where E and H denote electric and magnetic fields in the absence of the flaw, and E' and H' denote the fields in the
presence of the flaw. The integration in Eq 6 is carried out over a surface enclosing the flaw, with n being the inner-
directed surface normal. The attractive feature of Eq 6 is that flaw signals can be evaluated as soon as electromagnetic
fields are obtained in the vicinity of a flaw region. Equation 6 has been proved effective in impedance evaluations and has
been used in many applications. Auld's theory, which was built around a combination of the reciprocity relation and the
Born approximation, has been playing a central role in the existing eddy current POD studies.
A word of caution is necessary concerning the reciprocity formula. Although its use is highly recommended, the
reciprocity formula is not the ultimate method for conducting impedance evaluations. In fact, the most versatile method is
the direct use of Faraday's law, which works under any circumstances. However, this method usually results in the most
computationally intensive efforts. A third possibility is to use the energy conservation law. This method, however, is the
least versatile because it is not always possible to estimate an amount of energy escaping from the system. There are no
general criteria for method selection. A case-by-case comparison is required, taking cost-effectiveness and the required
degree of accuracy into account.
Probe-Flaw Interaction Models. To make the eddy current method quantitative, one must be able to predict flaw
signals for any given system. Earlier, equivalent circuit methods were attempted (Ref 23). The present consensus is that
detailed evaluations of electromagnetic fields are needed to make sufficiently precise predictions.
One therefore needs to solve Maxwell's equations, assuming that all the system parameters (that is, a probe, a part, a flaw,
and the inspection geometry) are given. Such mathematical techniques as exact methods, integral equation methods,
perturbation theory, and variational methods are frequently used. Various approximation schemes can also be employed.
In the literature, the integral equation methods are used most frequently, where the basic differential equations are cast
into integral equations using appropriate boundary conditions. Particularly popular are the three-dimensional finite-
element method and the boundary integral equation (BIE) method.
Again, selection of the most suitable method for a given situation should be done on a case-by-case basis. The three-
dimensional finite-element method is the most versatile, but is also the most expensive. For crack problems, the BIE
method may be favored in several aspects. Typically, an eddy current system is piece. wise homogeneous, consisting of
several spatial regions separated by sharp boundaries. Moreover, the boundaries are rigid surfaces that are not subject to
change over time. Clearly, this is an ideal situation for applying the BIE method. Another favorable aspect is that the
aforementioned reciprocity formula uses only field values on boundaries. As long as it is applicable, the BIE approach
gives a faster algorithm than the three-dimensional finite-element method. There are, however, situations in which the
BIE method fails to work well. For example, it is not effective when dealing with flaws caused by corrosion where
conductivity may vary continuously in space. Presumably, this type of flaw is still confined in a local region. If so, it is
possible to solve the problem with a combination of the BIE and three-dimensional finite-element methods. To the best of
the authors' knowledge, such a combined approach has never appeared in the literature. Perturbation theory may be useful
if the variation is small. The perturbation method should certainly be used more frequently in dealing, for example, with a
conductivity fluctuation, which is an important noise source to consider in POD models.
Probability of Detection Models. In this section, definitions of probability of detection and probability of false alarm
(POFA) will be given in a form suitable for the eddy current inspection method. Various, inequivalent definitions are seen
in the literature, but the intention here is to illustrate the basic idea.
Suppose that an eddy current inspection system is specified and that a series of impedance measurements is carried out
over a flaw at a given frequency. By nature, the measured signals will scatter around a mean value in a complex
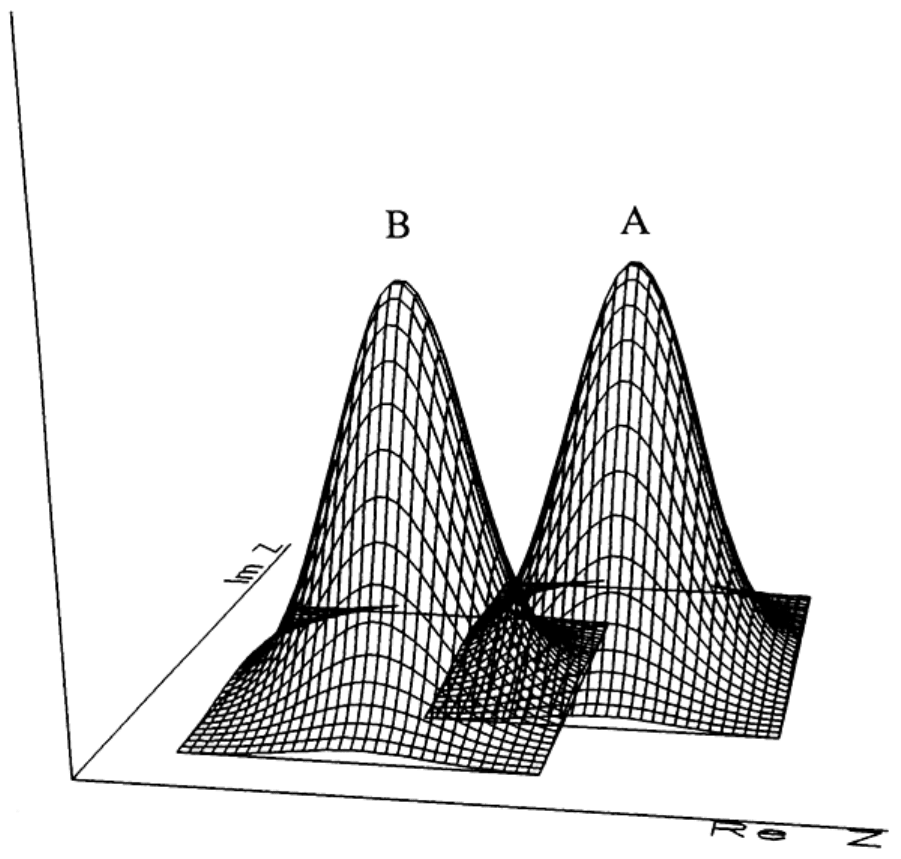
impedance plane. Distribution A shown in Fig. 7 illustrates a typical data distribution from a flawed area, while
distribution B represents a data set taken under the same controlled conditions but over an unflawed part. Strictly
speaking, a flaw signal is given by the difference between the on-flaw and off-flaw signals; the flaw signal corresponds
graphically to the shift of the two peak positions, A and B. The fluctuations around the peaks reflect the noise
characteristics of the given inspection system.
Fig. 7
Illustration of signal distributions. Measured impedance values will follow a certain distribution.
Distribution A illustrates an on-flaw signal distribution (signals measured on a flaw), while B illustrates an off-
flaw signal distribution.
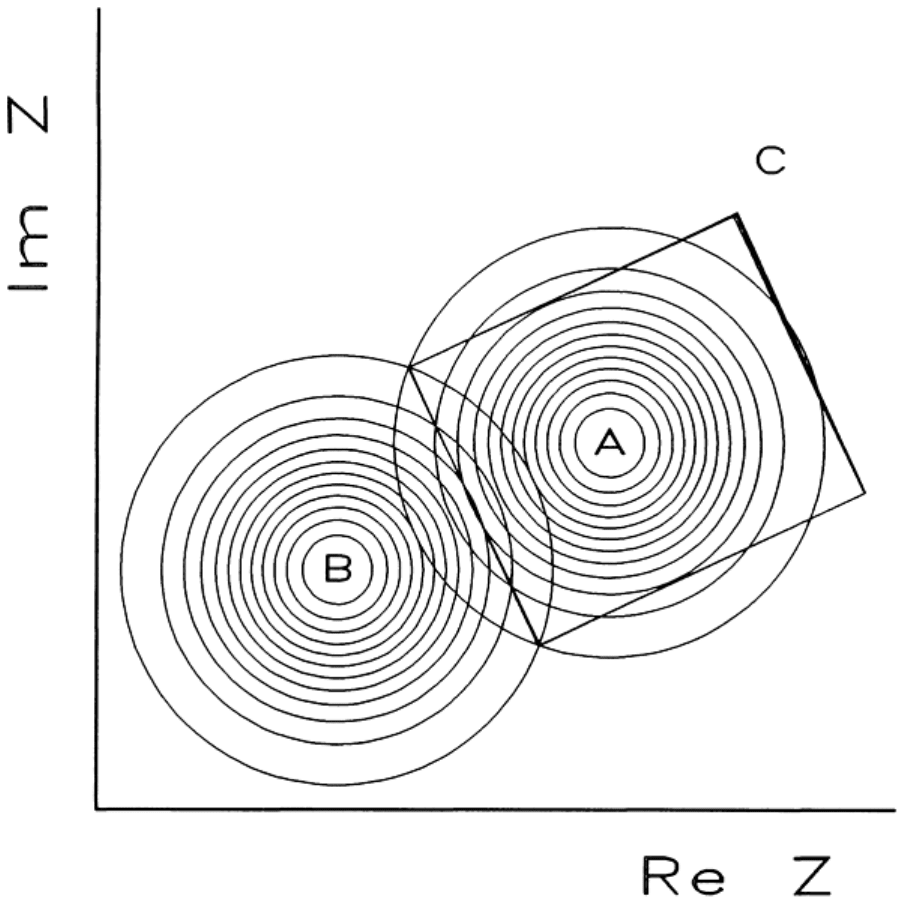
Once signal/noise distributions are given, one can begin to study the inspectability of the system, as follows. Consider a
window in the impedance plane (for example, C in Fig. 8), and make a hypothesis that any signal falling in this window is
actually a flaw signal. Then, POD is defined by the probability of this hypothesis being true, which is equal to the integral
of distribution A over window C (provided the total integral of A is normalized to unity). Similarly, POFA is given by the
integral of B over C, that is, the probability of off-flaw signals being mistaken as flaw signals. The POD and POFA
values thus evaluated are functions of C, and when evaluated for various windows, they will form a domain in a POD-
POFA plane. Such POD-POFA plots can be used as a measure of inspectability. Alternatively, representatives of POD
and POFA values can be used for specification, such as those in which the maximum POD/POFA ratio is achieved.
Fig. 8 The same distributions as in Fig. 7
viewed from above. Window C will be used to define POD and POFA as
integrals of the distributions over C.
Therefore, the fundamental task is to determine the on-flaw and off-flaw signal distributions for a given system. One
important theoretical task, for example, is to predict the peak positions A and B with model calculations. Noise
distributions are more difficult to predict because, by nature, any measurement system will contain certain unpredictable
noise sources. Nonetheless, to make eddy current POD packages an effective design aid, as much effort as possible should
be devoted to the complete identification and characterization of conceivable noise sources. References 30 and 34 contain
specific examples of POD calculations, and Fig. 9 illustrates an example of a POD-POFA plot.
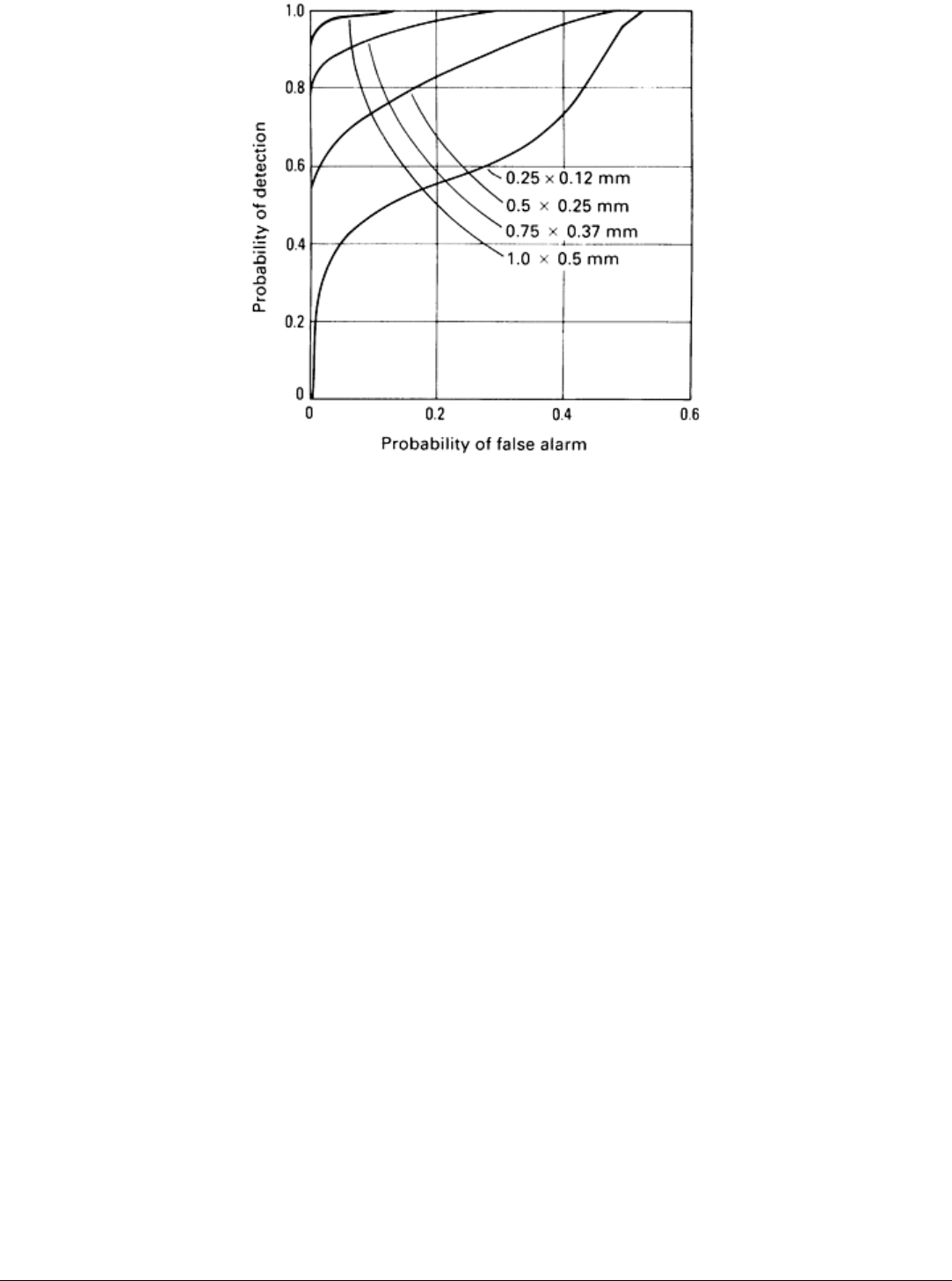
Fig. 9 Sample plot of POD-POFA curves for different flaw sizes. Source: Ref 12
Discussions and Future Directions. Comparing the goal just stated with the current developmental status in the
literature (Ref 30, 34) will lead one to expect improvements in eddy current POD models in the following areas. First,
theoretical models of limited capabilities have been used for impedance calculations. These limitations inevitably restrict
the applicability of the existing POD models. For example, the models have been probe specific, limited to work only for
certain measurement geometries, and so on. Any progress in forward calculations will provide more flexible POD models.
Second, noise characterizations have been accomplished almost exclusively by relying on experimental input. In view of
using POD models as a design aid, this is not an ideal situation, because one needs to build a prototype system before
calculating POD. Unfortunately, this trend will continue until sufficient data have been accumulated so that reliable
general formulas can be extracted empirically. Very little has been done to find purely theoretical noise simulations.
Finally, as a part of software development, a program shell of a POD package should be established, following the
general guideline of POD evaluations. Ideally, the package will be written based on a modular programming concept. It
may consist of three basic modules: impedance calculation, noise calibration, and POD calculation. Once the shell is
written, any progress in either forward calculation or noise characterization can be implemented quickly into the package
by replacing the appropriate modules.
There are also two technical recommendations. First, although POD itself can be calculated for a fixed frequency, it is of
crucial importance to enable the system to handle a broad range of frequencies. Without this capability, no precise
determinations of flaw sizes and shapes can be expected. This applies equally to hardware and software developments.
Second, few of the previous measurement techniques and associated POD models fully exploit the complex nature of
impedance. Both the real and imaginary parts should be treated on an equal footing as described above in order to make
full use of valuable information.
It should be emphasized that, so far, the concept of inspectability has been defined for systems as a whole, so that one can
discuss the POD of a system but not the POD of a single component. To validate the notion of the POD of a given probe,
it is necessary to integrate the probe into an inspection system in which all the other system components are strictly
controlled to meet certain specifications. In the future, such a standardization may become an important issue for
comparison purposes.
References cited in this section

12.
R.B. Thompson and E.F. Lopes, The Effects of Focusing and Refraction on Gaussian Ultrasonic Beams,
J.
Nondestr. Eval., Vol 4, 1984, p 107
23.
H.L. Libby, Introduction to Electromagnetic Nondestructive Test Methods, Wiley-Interscience, 1971
24.
M. Burrows, "A Theory of Eddy Current Flaw Detection," Ph.D. thesis, University of Michigan, 1964
25.
C. Dodd and W.E. Deeds, Analytical Solution to Eddy-Current Probe-Coil Problem, J. Appl. Phys.,
Vol 39,
1968, p 2829
26.
A.H. Kahn, R. Spal, and A. Feldman, Eddy-
Current Losses Due to a Surface Crack in a Conducting
Material, J. Appl. Phys., Vol 48, 1977, p 4454
27.
W.D. Dover, F.D.W. Charlesworth, K.A. Taylor, R. Collins, and D.H. Michael, in
The Measurement of
Crack Length and Shape during Fatigue and Fracture, C.J. Beevers, Ed., EMAS, 1980
28.
B.A. Auld, Theoretical Characterization and Comparison of Resonant-Probe Microwave Eddy-Curr
ent
Testing With Conventional Low-Frequency Eddy-Current Methods, in
Eddy Current Characterization of
Materials and Structures,
STP 722, G. Birnbaum and G. Free, Ed., American Society for Testing and
Materials, 1981
29.
T.G. Kincaid, A Theory of Eddy Current NDE for Cracks in Nonmagnetic Materials, in
Review of Progress
in Quantitative NDE, Vol 1, D.O. Thompson and D.E. Chimenti, Ed., Plenum Press, 1982
30.
A.J. Bahr, System Analysis of Eddy-Current Measurements, in Review of Progress in Quantitative NDE,
Vol 1, D.O. Thompson and D.E. Chimenti, Ed., Plenum Press, 1982; A.J. Bahr and D.W. Cooley, Analysis
and Design of Eddy-Current Systems, in Review of Progress in Quantitative NDE,
Vol 2A, D.O. Thompson
and D.E. Chimenti, Ed., Plenum Press, 1983; J.R. Mart
inez and A.J. Bahr, Statistical Detection Model, in
Review of Progress in Quantitative NDE,
Vol 3A, D.O. Thompson and D.E. Chimenti, Ed., Plenum Press,
1984
31.
B.A. Auld, F.G. Muennemann, and D.K. Winslow, J. Nondestr. Eval., Vol 2, 1982, p 1; B.A. Auld,
F.G.
Muennemann, and M. Riaziat, Quantitative Modeling of Flaw Responses in Eddy Current Testing, in
Research Techniques in Nondestructive Testing, Vol 7, R.S. Sharpe, Ed., Academic Press, 1984
32.
R.E. Beissner, Boundary Element Model of Eddy Current Flaw Detection in Three Dimensions,
J. Appl.
Phys., Vol 60, 1986, p 352-360; Analytical Green's Dyads for an Electrically Conducting Half-Space,
J.
Appl. Phys., Vol 60, 1986, p 855-858
33.
J.C. Moulder, P.J. Shull, and T.E. Capobianco, Uniform Field Probe:
Experiments and Inversion for
Realistic Flaws, in Review of Progress in Quantitative NDE,
Vol 7B, D.O. Thompson and D.E. Chimenti,
Ed., Plenum Press, 1987
34.
R.E. Beissner, K.A. Bartels, and J.L. Fisher, Prediction of the Probability of Eddy Current Flaw
Detection,
in Review of Progress in Quantitative NDE,
Vol 7B, D.O. Thompson and D.E. Chimenti, Ed., Plenum
Press, 1988
Models for Predicting NDE Reliability
J.N. Gray, T.A. Gray, N. Nakagawa, and R.B. Thompson, Center for NDE, Iowa State University
Radiographic Inspection Model
In radiographic modeling, one is essentially trying to predict how the two-dimensional projection of an irregularly shaped
object changes as the position of the object changes with respect to the x-ray beam and detector and, further, to study the
degree to which various parameters control image quality (Ref 35, 36). The requirements for a quantitative model of the
radiographic process consist of the ability to predict the output of an x-ray generator, the interaction of the x-ray beam
with the sample, and the detector characteristics. The capability to handle complex part geometries and non-trivial flaw
morphologies is required if the simulation is to have wide application.
Gray et al. have developed a full three-dimensional radiography model that generates a two-dimensional (x,y) film image
(Ref 37, 38) rather than the one-dimensional source-sample-detector dimension previously modeled (Ref 39). The full
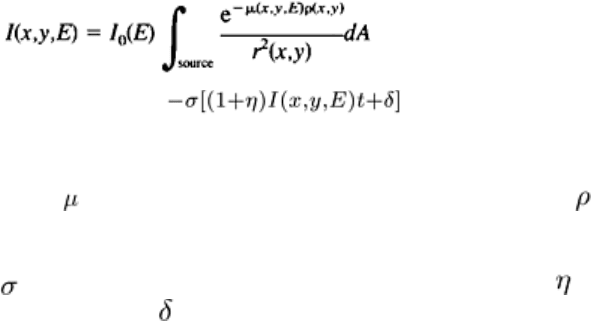
shadowing effects and the radial divergence of the x-ray beam in the projections are included, allowing a realistic
treatment of complex part geometries. The model consists of:
(Eq 7)
D(x,y,E) = D
0
(1 - e )
(Eq 8)
In Eq 7, E is the energy, I is the intensity immediately above an xy point on the detector, I
0
, is the initial intensity
produced from the x-ray generator, is the energy-dependent linear absorption coefficient, is the x-ray path length
through the sample, and r is the distance from the source to the detector.
In Eq 8, D is the film density, is the interaction cross section of an x-ray with a film grain, is the coefficient for the x-
ray scattering, t is the time of the film exposure, is the natural film fog density, and D
0
is the maximum film density. The
integration goes over the area of the source, thus including the affect of source properties on image quality. This model of
the x-ray radiographic process has the capability of modeling a very large number of parameters that affect the image
quality of an x-ray radiograph. As noted in Eq 7 and 8, the major elements of the model include an x-ray beam model (I
0
),
the interaction of the beam with a nontrivial sample, and a detector model. These must be combined with a detectability
criterion in order to complete a POD prediction.
Beam Model. The model of the initial x-ray beam involves a first-principles prediction of the energy-dependent x-ray
intensity spectrum calculated from the bremsstrahlung and characteristic interaction cross sections (Ref 40). These cross
sections are calculated from the interaction of the relativistic electron beam with the bound atomic electrons of the target
atom and are based on a one-photon production process. The angular dependence of the bremsstrahlung cross section is
integrated over all angles. This simplification implies that the calculated intensities will require a scaling factor to match
the experimentally measured values. The energy dependence of the spectrum is calculated assuming a thick target
attenuation of the electron beam. This beam model has features that allow variation in the beam focal-spot size, shape,
and uniformity. Further, the target material and angle of incidence to the electron beam are variables. The model accounts
for the inherent filtration of the generated x-ray beam by the target material and the vacuum window of the exit port.
Features that the beam model does not address are the generation of off-axis x-rays, the interaction cross sections that
involve the generation of more than one photon, the physical mechanics of electron beam focusing, and the variation of
the voltage from power supply. These features influence the intensity of the beam and at present are handled in the beam
model with an intensity scaling factor.
Sample Interaction Model. The interaction of the x-ray beam with the sample monitors the energy-dependent
attenuation through the sample (Ref 41). This portion of the model includes the effects of the sample shape, the flaw
morphology, and the configuration of the experimental setup. The sample shape currently used in the simulation is a flat
plate with surface roughness. The simple part shape allows the effects of many parameters to be isolated from those
involving the complex part shapes.
Two types of flaws are available for study in the simulation. The first is an ellipsoid with nine independent parameters
characterizing its shape, orientation, and position. The position of the origin, the three major axes, and three Euler angles
give complete, arbitrary control of the position and shape of the ellipsoid. The ellipsoid can be a void or an inclusion.
Further, the sample position relative to the source or detector, the thickness, and the material can be chosen as desired,
thus providing a wide range of setup geometries. The second flaw is a truncated cone whose apex angle, truncation
distance, composition material, and xyz position in the sample can all be varied. Additional features are the ability to
model density variations in the material and a wide choice of sample materials ranging from low atomic number carbon-
base materials to high atomic number materials such as tantalum. As noted above, the sample shape is limited. However,
future integration of the simulation into a CAD package will remedy the lack of a wide variety of shapes because any part
geometry that the CAD package can generate can be used in the simulation. A note on the introduction of complex CAD-
generated part shapes is that the calculation of the POD can take significant amounts of time, at least with present
computer capabilities.
Detector Model. If film is used as the detector, a model of the film simulates a number of film speeds, maximum
densities, and the energy-dependent effects of the x-ray interaction with the silver halide grains. The film model is derived
by considering the number of grains that have absorbed x-ray photons, which absorb an additional photon as a result of
the flux incident on the film. The number of photons required for a silver halide grain to be developed is energy
dependent. For very low energy (10 keV), several photons are required, while at high energy (1 MeV), one photon can
activate several grains. Unfortunately, the energy dependence of grain activation is also dependent on the likelihood of x-
ray absorption, a feature that is dependent on the size of the grain and the energy of the x-ray photon. These energy-
dependent features are reflected in the x-ray interaction cross section, . This cross section, together with the grain size,
gives the film model the capability to model the speed of the film (Ref 42). The total amount of silver halide grain
present, as represented by the weight of the emulsion, controls the maximum film density, D
0
. The fog density, , of the
unexposed film can be accounted for in the final density.
The film model tracks the random counting statistics noise and in a limited set of sample geometries tracks the noise due
to Compton scattering. For simple geometries, the Compton scattering is a uniform intensity at the film and is modeled
through the scattering coefficient, . This is a simplistic approximation to a complex problem. Indeed, the Compton
scattering for energies above several hundred kiloelectron volts is the dominant scattering mechanism and can contribute
more to the film density than the information-carrying portion of the beam. The additional complexities of part geometry
nullify the uniformity of the Compton scattering at the detector plane, making a detailed tracking of the Compton
component necessary. The addition of this important effect is the subject of ongoing research.
Detectability. The detectability criterion accounts for the physiological limitation of the human eye to detect, under
normal conditions, variations in gray film scale levels. The determination of the limits of gray-scale variation that the
human eye can perceive is based on studies of uniform gray background with a circle that has a different gray-scale level
(Ref 35). Although this is not the general condition under which radiographs are viewed, this is the criterion currently
incorporated into the model. Further modification involves a mechanism to account for degradation of the contrast due to
a poor signal-to-noise ratio. One of the features of the simulation is the capability of turning off the noise, thus allowing
examination of the noise degradation of the signal. The noise in the film image is, in most cases, dominated by the
random fluctuations of the number of photons impinging on a unit area. The result is that the noise magnitude is related to
the density of the film; higher noise is associated with low densities, while low noise corresponds to high density. This
has led to the incorporation of contrast degradation due to noise and therefore to the inclusion of its influence on flaw
signal detectability. This detectability model falls into the amplitude model class of detectability, and although a simple
model, it has the major features for adequately describing detectability (Ref 43).
Limitations. At present, there are a number of limitations to the model. They include a limited accounting for the effect
of Compton scattering, the need for an intensity scaling factor for the initial beam, and a simple detectability model.
Experimental verification of the major components is under way, and the preliminary results are very good. The initial
beam model gives results that only need scaling to accurately model the observed x-ray spectrum. The film model can
characterize the film response to a photon flux for a wide range of film types. For the full simulation, with only the
scaling for the initial beam intensity, the results, when compared to an actual radiograph of a machined cone-shaped void,
are in the worst case within a factor or two of the actual film densities. In the best cases, the model results are within 20%
of the observed results. The refinement of the model is work that is ongoing.
Applications. With this highly flexible model of the radiographic process, several factors affecting flaw detectability
can be studied in a quantitative manner. A sample of the types of effects studied can be seen in Fig. 10 and 11, in which
the loss of detectability was simulated for changes in beam hardness and flaw orientation, respectively. The simulation
predicts a range of kilovoltages for which adequate contrast and noise levels are present. To perform adequate x-ray
inspections, the data must be collected in these plateau regions. It should be noted that the sizes of these optimal regions
are also strongly controlled by the flaw shape and size. The loss of detectability was calculated quantitatively based on the
physiological limitation of human vision to discern variations in gray shades, the noise level present in the radiograph,
and the contrast available.
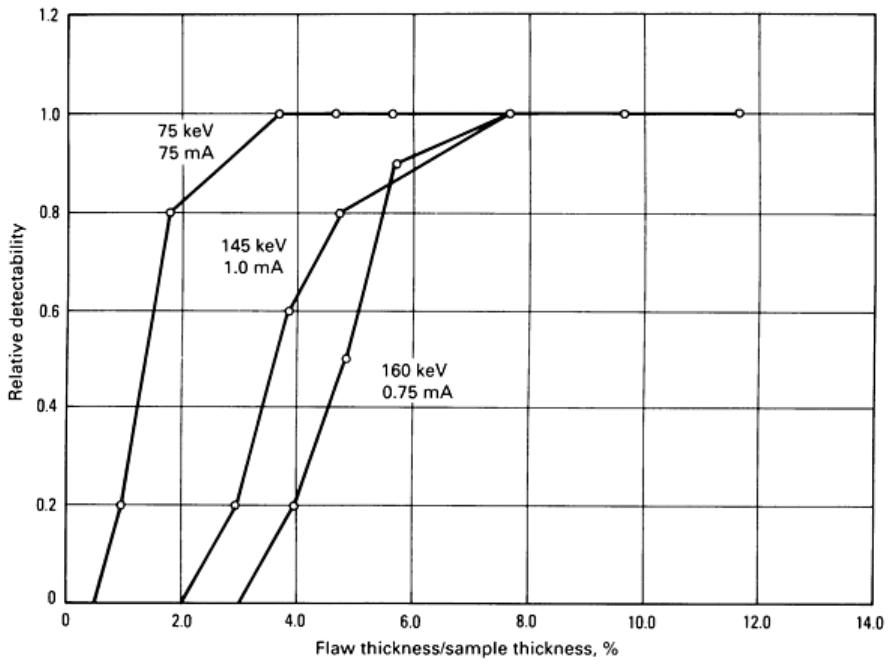
Fig. 10 Simulation results showing the effects on thickness sensitivity of the x-
ray beam hardness and the
relative size of the flaw to sample thickness
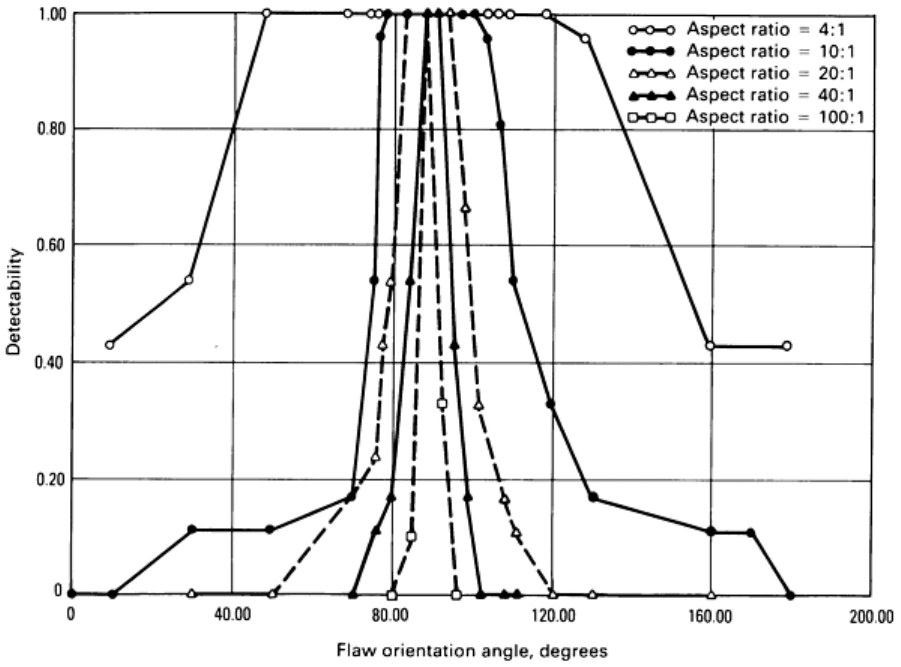
Fig. 11
Detectability of different ellipsoidal flaws as a function of their orientation and shape. The shape of the
ellipsoid i
s changed by varying the aspect ratio, where two of the major axes are kept equal and the third is
decreased. The ratio of the constant major axes to the smaller is the aspect ratio. As the aspect ratio increases,
the ellipsoid flattens and becomes crackli
ke. The scale for angular orientation is chosen such that a crack is
optimally oriented at 90°.
Figure 10 shows a plot of the effect of beam hardness (the white spectrums of a harder beam have a higher average
energy) on the detectability of a flaw where the film type, the sample composition, the x-ray beam shape, and the physical
geometric setup were kept the same. The time of the exposure was increased to attain the same film densities for each
simulated exposure. As can be seen, the harder beam has less sensitivity but a shorter exposure time, while the softer
beam yields greater sensitivity at the cost of longer exposure times. Thus, the trade-off between sensitivity and speed of
inspection can be studied.
Figure 11 shows the effect of flaw morphology on detectability. The flaws represented in Fig. 11 have widely varying
aspect ratios, thus simulating varying degrees of crack tightness. As the orientation of these cracks with respect to the
incident x-ray beam changes, their detectable signal drops abruptly.
There are a number of applications of the forward model of the x-ray radiographic process in the field of production
inspection. The first is the collapsing of the full three-dimensional aspect of the model to a one-dimensional line path.
This allows quick calculation of the kilovoltage, current, and time required for the exposure of a type of film to the
density required. Another is in the area of inspection validation studies. The use of seeded defects and image quality
indicators and the sensitivity testing of various parameters are time consuming and expensive, so much so that a full study
in which all parameters are varied is rarely done. The use of a simulation program coupled with a set of benchmark trials
can greatly expand the scope of this type of certification of an inspection procedure. Modest extensions of the model
would facilitate an even wider range of applications. The prediction of a full POD requires only the addition of a
probability distribution function describing the flaw distribution, as described in the section "Ultrasonic Inspection
Model" in this article. As can be seen from Fig. 10 and 11, the current model is very close to the generation of POD
curves for different system parameters. A second area for extension is the simulation of tomographic scans. The addition

of a detector model typical of those used in tomographic scans will allow the generation of the projection data required
for the reconstruction algorithms.
References cited in this section
35.
R. Halmshaw, Physics of Industrial Radiography, Applied Science, 1966
36.
P. DeMeester and W. Aerts, in Research Techniques in Nondestructive Testing,
Vol VI, R.S. Sharpe, Ed.,
Academic Press, 1982
37.
J.N. Gray, Three Dimensional Modeling of Projection Radiography, in
Review of Progress in Quantitative
Nondestructive Evaluation, Vol 7A, D.O. Thompson and D.E. Chimenti, Ed., Plenum Press, 1988, p 343-
348
38.
J.N. Gray, F. Inanc, and B.E. Shull, Three Dimensional Modeling of Projection Radiography, in
Review of
Progress in Quantitative Nondestructive Evaluation,
Vol 8A, D.O. Thompson and D.E. Chimenti, Ed.,
Plenum Press, 1989
39.
D.E. Rundquist, in Research Techniques in Nondestructive Testing, Vol IV, R.S. Sharpe, Ed., Aca
demic
Press, 1982
40.
C.M. Lee, L. Kissell, R.H. Pratt, and H.K. Tseng, Phys, Rev., Vol A13 (No. 5), 1976, p 1714-1727
41.
W.H. McMaster, N. Kerr Del Grande, J.H. Mallett, and J.H. Hubbell, "Compilation of X-
ray Cross
Sections," UCRL-50174 Section II Rev. 1, Los Alamos National Laboratory, 1974
42.
H.H. Barrett and W. Swindell, in
Radiological Imaging: The Theory of Image Formation, Detection, and
Processing, Vol 1, Academic Press, 1981, p 206
43.
L.D. Loo, K. Doi, and C.E. Metz, Phys. Med. Biol., Vol 29 (No. 7), 1984, p 837-856
Models for Predicting NDE Reliability
J.N. Gray, T.A. Gray, N. Nakagawa, and R.B. Thompson, Center for NDE, Iowa State University
Future Impact of Models
The three preceding sections describe computer models that can be used to generate quantitative predictions of
inspectability for ultrasonic, eddy current, and x-ray film methods. These are all physically based models that explicitly
consider the characteristics of the realistic flaws, the typical component geometries and materials, and the practical
inspection methods and practices. These or similar models may:
• Replace costly and time-
consuming experimental demonstration programs in the prediction of NDE
reliability
• Improve the validation and optimization of inspection procedures
• Improve component design and the definition of the life cycles
Another potential application of NDE reliability models may involve integration with CAD systems. This approach could
allow the assessment of inspectability during the design stage just as form, fit, and function are analyzed during design.
The integration of NDE reliability models with a CAD system may also allow quantitative estimates of inspection
reliability as a function of design changes. The following example, illustrated in Fig. 12 and 13, describes this application
of NDE/CAD integration for ultrasonic inspectability (Ref 8). The inspectability (POD) model for ultrasonic NDE has
achieved the highest state of technical maturity, and initial strides have been made toward its incorporation into CAD
systems. However, the general idea is applicable to NDE reliability models of inspection methods other than ultrasonics.
