Articolo G.A. Partial Differential Equations and Boundary Value Problems with Maple V
Подождите немного. Документ загружается.


468 Chapter 7
two-dimensional homogeneous wave partial differential equation with damping in rectangular
coordinates reads
∂
2
∂t
2
u(x, y, t) = c
2
∂
2
∂x
2
u(x, y, t) +
∂
2
∂y
2
u(x, y, t)
−γ
∂
∂t
u(x, y, t)
For the wave equation, we have to consider the two initial conditions
u(x, y, 0) = f(x, y) and u
t
(x, y, 0) = g(x, y)
In Exercises 7.1 to 7.15, we seek solutions to the preceding problem for various given
boundary and initial conditions. Evaluate the eigenvalues and corresponding two-dimensional
orthonormalized eigenfunctions, and write the general solution. Generate the animated
three-dimensional surface solution u(x, y, t), and plot the animated sequence for 0 <t<5.
7.1. Boundaries x = 0,x= 1,y= 0,y= 1 are held secure, c = 1/2,γ = 1/10:
u(0,y,t)= 0 and u(1,y,t)= 0
u(x, 0,t)= 0 and u(x, 1,t)= 0
Initial conditions:
f(x, y) = x(1 −x)y(1 −y) and g(x, y) = 0
7.2. Boundaries x = 0,y= 0 are held secure, boundaries x = 1,y= 1 are unsecure,
c = 1/2,γ = 1/10:
u(0,y,t)= 0 and u
x
(1,y,t)= 0
u(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial conditions:
f(x, y) = 0 and g(x, y) = x
1 −
x
2
y
1 −
y
2
7.3. Boundaries x = 0,y= 1 are held secure, boundaries x = 1,y= 0 are unsecure,
c = 1/2,γ = 1/10:
u(0,y,t)= 0 and u
x
(1,y,t)= 0
u
y
(x, 0,t)= 0 and u(x, 1,t)= 0
Initial conditions:
f(x, y) = x
1 −
x
2
(1 −y
2
) and g(x, y) = 0

The Wave Equation in Two Spatial Dimensions 469
7.4. Boundaries x = 0,x= 1 are held secure, boundaries y = 0,y= 1 are unsecure,
c = 1/2,γ = 1/10:
u(0,y,t)= 0 and u(1,y,t)= 0
u
y
(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial conditions:
f(x, y) = 0 and g(x, y) = x(1 −x)y
2
1 −
2y
3
7.5. Boundaries x = 0,x= 1,y= 1 are held secure, boundary y = 0 is unsecure,
c = 1/2,γ = 1/10:
u(0,y,t)= 0 and u(1,y,t)= 0
u
y
(x, 0,t)= 0 and u(x, 1,t)= 0
Initial conditions:
f(x, y) = x(1 −x)(1 −y
2
) and g(x, y) = 0
7.6. Boundaries x = 0,x= 1,y= 0 are unsecure, boundary y = 1 is held secure,
c = 1/2,γ = 1/10:
u
x
(0,y,t)= 0 and u
x
(1,y,t)= 0
u
y
(x, 0,t)= 0 and u(x, 1,t)= 0
Initial conditions:
f(x, y) = 0 and g(x, y) = x
2
1 −
2x
3
(1 −y
2
)
7.7. Boundaries x = 0,x= 1,y= 1 are held secure, boundary y = 0 is attached to an elastic
hinge, c = 1/2,γ = 1/10:
u(0,y,t)= 0 and u(1,y,t)= 0
u
y
(x, 0,t)−u(x, 0,t)= 0 and u(x, 1,t)= 0
Initial conditions:
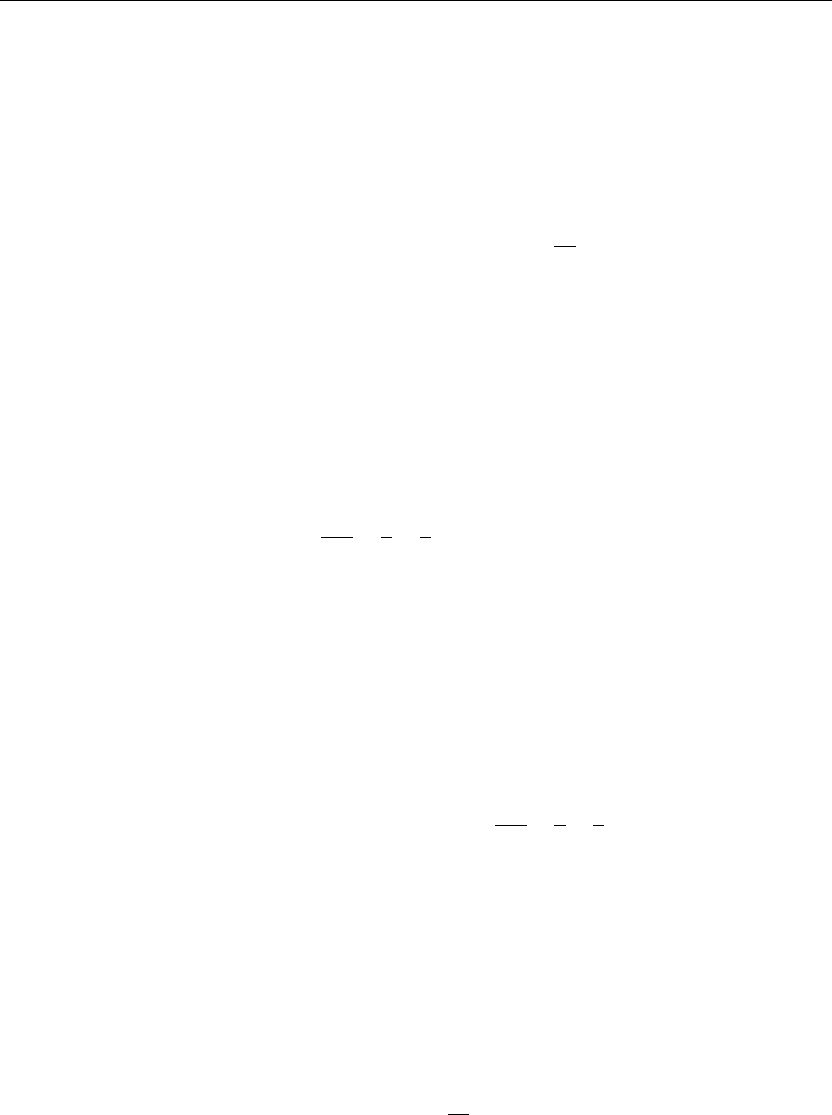
f(x, y) = x(1 −x)
−
2y
2
3
+
y
3
+
1
3
and g(x, y) = 0
470 Chapter 7
7.8. Boundaries x = 0,y= 0,y = 1 are held secure, boundary x = 1 is attached to an elastic
hinge, c = 1/2,γ = 1/10:
u(0,y,t)= 0 and u
x
(1,y,t)+u(1,y,0) = 0
u(x, 0,t)= 0 and u(x, 1,t)= 0
Initial conditions:
f(x, y) = 0 and g(x, y) = x
1 −
2x
3
y(1 −y)
7.9. Boundaries x = 1,y= 0,y = 1 are held secure, boundary x = 0 is attached to an elastic
hinge, c = 1/2,γ = 1/10:
u
x
(0,y,t)−u(0,y,t)= 0 and u(1,y,t)= 0
u(x, 0,t)= 0 and u(x, 1,t)= 0
Initial conditions:
f(x, y) =
−
2x
2
3
+
x
3
+
1
3
y(1 −y) and g(x, y) = 0
7.10. Boundaries x = 1,y= 1 are held secure, boundary y = 0 is unsecure, and boundary
x = 0 is attached to an elastic hinge, c = 1/2,γ = 1/10:
u
x
(0,y,t)−u(0,y,t)= 0 and u(1,y,t)= 0
u
y
(x, 0,t)= 0 and u(x, 1,t)= 0
Initial conditions:
f(x, y) = 0 and g(x, y) =
−
2x
2
3
+
x
3
+
1
3
(1 −y
2
)
7.11. Boundaries x = 0,x= 1 are held secure, boundary y = 0 is unsecure, and boundary
y = 1 is attached to an elastic hinge, c = 1/2,γ = 1/10:
u(0,y,t)= 0 and u(1,y,t)= 0
u
y
(x, 0,t)= 0 and u
y
(x, 1,t)+u(x, 1,t)= 0
Initial conditions:
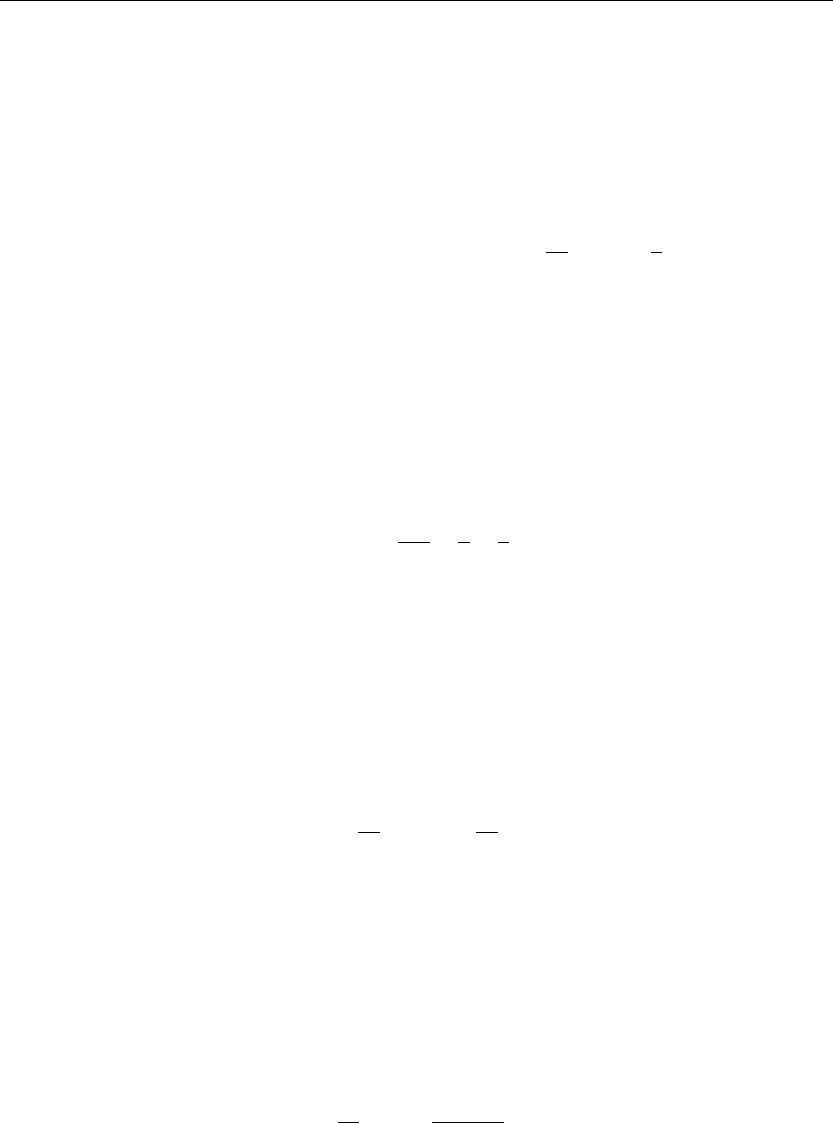
f(x, y) = x(1 −x)
1 −
y
2
3
and g(x, y) = 0
The Wave Equation in Two Spatial Dimensions 471
7.12. Boundaries x = 0,y= 0 are held secure, boundary y = 1 is unsecure, and boundary
x = 1 is attached to an elastic hinge, c = 1/2,γ = 1/10:
u(0,y,t)= 0 and u
x
(1,y,t)+u(1,y,t)= 0
u(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial conditions:
f(x, y) = 0 and g(x, y) = x
1 −
2x
3
y
1 −
y
2
7.13. Boundaries x = 1,y= 1 are held secure, boundary x = 0 is unsecure, and boundary
y = 0 is attached to an elastic hinge, c = 1/2,γ = 1/10:
u
x
(0,y,t)= 0 and u(1,y,t)= 0
u
y
(x, 0,t)−u(x, 0,t)= 0 and u(x, 1,t)= 0
Initial conditions:
f(x, y) =
1 −x
2
−
2y
2
3
+
y
3
+
1
3
and g(x, y) = 0
7.14. Boundaries y = 0,y = 1 are both unsecure, boundary x = 0 is secure, and boundary
x = 1 is attached to an elastic hinge, c = 1/2,γ = 1/10:
u(0,y,t)= 0 and u
x
(1,y,t)+u(1,y,t)= 0
u
y
(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial conditions:
f(x, y) = x
1 −
2x
3
y
2
1 −
2y
3
and g(x, y) = 1
7.15. Boundaries x = 0,y= 1 are both unsecure, boundaries x = 1,y= 0 are both attached to
an elastic hinge, c = 1/2,γ = 1/10:
u
x
(0,y,t)= 0 and u
x
(1,y,t)+u(1,y,t)= 0
u
y
(x, 0,t)−u(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial conditions:
f(x, y) =
1 −
x
2
3
1 −
(y −1)
2
3
and g(x, y) = 0

472 Chapter 7
Angular Frequency and Multiplicity
From Section 7.4, for small damping in the system, the damped angular frequency of vibration
for the (m, n) mode was given as
ω
m, n
=
4λ
m, n
c
2
−γ
2
2
where c is the propagation speed of the wave, γ is the damping coefficient, and λ
m, n
is given as
λ
m, n
= α
m
+β
n
Here, α
m
is the eigenvalue corresponding to the x-dependent eigenvalue problem, and β
n
is the
eigenvalue corresponding to the y-dependent eigenvalue problem. For a two-dimensional
membrane, the propagation speed of the wave is given as
c =
T
ρ
where T is the tension per unit length in the membrane and ρ is the area density (mass/area) of
the membrane.
For the special case of no damping in the system, the undamped angular frequency is given by
ω
m, n
= c
α
m
+β
n
From Example 7.6.1 in Section 7.6, for generalized coordinates x = a, y = b of the rectangular
membrane, the values for α
m
,β
n
, and ω
m, n
are given, respectively, as
α
m
=
m
2
π
2
a
2
β
n
=
n
2
π
2
b
2
and
ω
m, n
= c
m
2
π
2
a
2
+
n
2
π
2
b
2
for m = 1, 2, 3,..., and n = 1, 2, 3,....
The corresponding allowed eigenfunctions are
X
m
(x)Y
n
(y) = sin
mπx
a
sin
nπy
b
It is obvious from the preceding equations that two different values of m and n could give rise
to two different eigenfunctions corresponding to the same angular vibration frequency.

The Wave Equation in Two Spatial Dimensions 473
For example, from Exercise 7.1, a = 1,b= 1, we see that the ω(1, 2) mode angular frequency
is identical to that of the ω(2, 1); yet the eigenfunctions are different. Thus, we get a
“multiplicity” of the same eigenvalues corresponding to different eigenfunctions.
In Exercises 7.16 through 7.20, you are asked to evaluate the generalized expression for the
undamped (γ = 0) angular frequency ω
m, n
and to evaluate the “fundamental” angular
frequency corresponding to the “smallest” allowed values of m and n; generally, the
“fundamental” angular frequency is the ω(1, 1) mode.
7.16. Reconsider Exercise 7.1 for the case of no damping (γ = 0). (a) Evaluate α
m
, β
n
, and
ω
m, n
for the given constants. (b) Evaluate the “fundamental” frequency. (c) Find an
example of multiplicity in the system by seeking two different sets of values (m, n) that
give rise to the same angular frequency.
7.17. Reconsider Exercise 7.2 for the case of no damping (γ = 0). (a) Evaluate α
m
, β
n
, and
ω
m, n
for the given constants. (b) Evaluate the “fundamental” frequency. (c) Find an
example of multiplicity in the system by seeking two different sets of values (m, n) that
give rise to the same angular frequency.
7.18. Reconsider Exercise 7.4 for the case of no damping (γ = 0). (a) Evaluate α
m
, β
n
, and
ω
m, n
for the given constants. (b) Evaluate the “fundamental” frequency. (c) Find an
example of multiplicity in the system by seeking two different sets of values (m, n) that
give rise to the same angular frequency.
7.19. Reconsider Exercise 7.5 for the case of no damping (γ =0). (a) Evaluate α
m
, β
n
, and
ω
m, n
for the given constants. (b) Evaluate the “fundamental” frequency. (c) Find an
example of multiplicity in the system by seeking two different sets of values (m, n) that
give rise to the same angular frequency.
7.20. Reconsider Exercise 7.6 for the case of no damping (γ = 0). (a) Evaluate α
m
, β
n
, and
ω
m, n
for the given constants. (b) Evaluate the “fundamental” frequency. (c) Find an
example of multiplicity in the system by seeking two different sets of values (m, n) that
give rise to the same angular frequency.
Two Dimensions, Cylindrical Coordinates
We now consider the propagation of transverse waves in circular membranes over the
two-dimensional domain D ={(r, θ) |0 <r<a,0 <θ<b} with a small amount of damping.
The homogeneous partial differential equation reads as
∂
2
∂t
2
u(r,θ,t)= c
2
⎛
⎝
∂
∂r
u(r,θ,t)+r
∂
2
∂r
2
u(r,θ,t)
r
+
∂
2
∂θ
2
u(r,θ,t)
r
2
⎞
⎠
−γ
∂
∂t
u(r,θ,t)

474 Chapter 7
with the two initial conditions
u(r, θ, 0) = f(r, θ) and u
t
(r, θ, 0) = g(r, θ)
In Exercises 7.21 to 7.32, we seek solutions to the preceding problem for various given
boundary and initial conditions. Evaluate the eigenvalues and corresponding two-dimensional
orthonormalized eigenfunctions, and write out the general solution. Generate the animated
three-dimensional surface solution u(r,θ,t), and plot the animated sequence for 0 <t<5.
7.21. The center of the membrane has a finite displacement, and the periphery at r = 1 is held
secure. The boundaries θ = 0 and θ = π are held secure, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u(1,θ,t)= 0
u(r, 0,t)= 0 and u(r,π,t)= 0
Initial conditions:
f(r, θ) =
r −r
3
sin(θ) and g(r, θ) = 0
7.22. The center of the membrane has a finite displacement, and the periphery at r = 1 is held
secure. The boundaries θ = 0 and θ = π are unsecure, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u(1,θ,t)= 0
u
θ
(r, 0,t)= 0 and u
θ
(r,π,t)= 0
Initial conditions:
f(r, θ) = 0 and g(r, θ) =
r
2
−r
4
cos(2θ)
7.23. The center of the membrane has a finite displacement, and the periphery at r = 1 is held
secure. The boundaries θ = 0 and θ = π are held secure, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u(1,θ,t)= 0
u(r, 0,t)= 0 and u(r,π,t)= 0
Initial conditions:
f(r, θ) =
r
3
−r
5
sin(3θ) and g(r, θ) = 0
7.24. The center of the membrane has a finite displacement, and the periphery at r = 1 is held
secure. The boundaries θ = 0 and θ = π/2 are held secure, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u(1,θ,t)= 0
u(r, 0,t)= 0 and u
r,
π
2
,t
= 0

The Wave Equation in Two Spatial Dimensions 475
Initial conditions:
f(r, θ) = 0 and g(r, θ) =
r
2
−r
4
sin(2θ)
7.25. The center of the membrane has a finite displacement, and the periphery at r = 1 is held
secure. The boundaries θ = 0 and θ = π/2 are unsecure, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u(1,θ,t)= 0
u
θ
(r, 0,t)= 0 and u
θ
r,
π
2
,t
= 0
Initial conditions:
f(r, θ) =
r
4
−r
6
cos(4θ) and g(r, θ) = 0
7.26. The center of the membrane has a finite displacement, and the periphery at r = 1 is held
secure. The boundary conditions are such that we have periodic boundary conditions on
θ, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u(1,θ,t)= 0
u(r, −π, t) = u(r,π,t) and u
θ
(r, −π, t) = u
θ
(r,π,t)
Initial conditions:
f(r, θ) =
r −r
3
cos(θ) and g(r, θ) = 0
7.27. The center of the membrane has a finite displacement, and the periphery at r = 1is
unsecure. The boundaries θ = 0 and θ = π are held secure, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u
r
(1,θ,t)= 0
u(r, 0,t)= 0 and u(r,π,t)= 0
Initial conditions:
f(r, θ) =
3r −r
3
sin(θ) and g(r, θ) = 0
7.28. The center of the membrane has a finite displacement, and the periphery at r = 1is
unsecure. The boundaries θ = 0 and θ = π are unsecure, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u
r
(1,θ,t)= 0
u
θ
(r, 0,t)= 0 and u
θ
(r,π,t)= 0
Initial conditions:
f(r, θ) =
2r
2
−r
4
cos(2θ) and g(r, θ) = 0
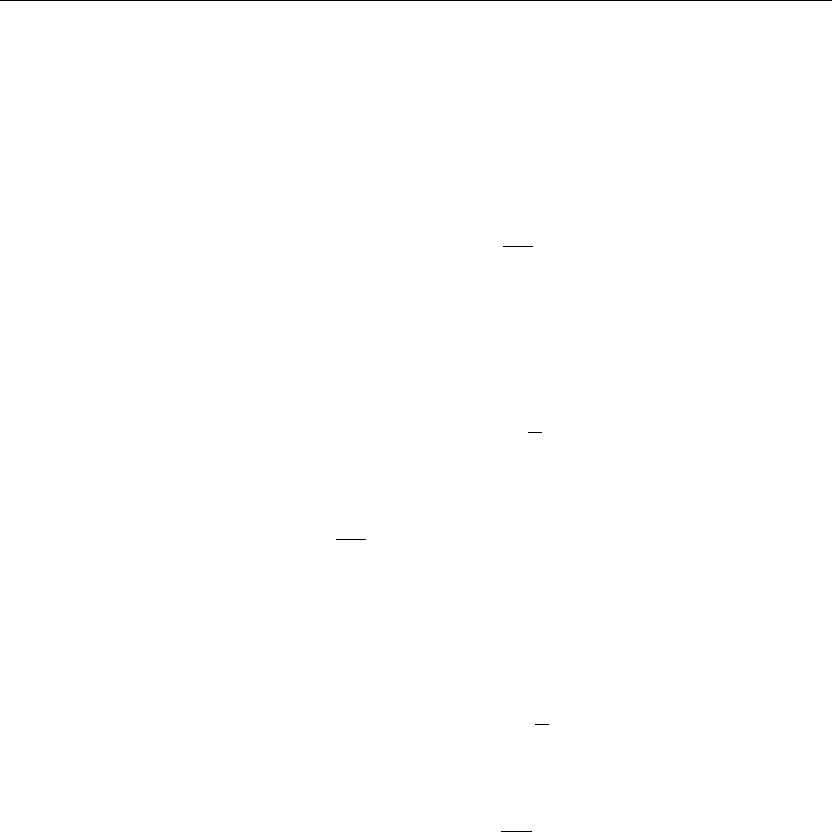
476 Chapter 7
7.29. The center of the membrane has a finite displacement, and the periphery at r = 1is
unsecure. The boundaries θ = 0 and θ = π are held secure, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u
r
(1,θ,t)= 0
u(r, 0,t)= 0 and u(r,π,t)= 0
Initial conditions:
f(r, θ) = 0 and g(r, θ) =
5r
3
3
−r
5
sin(3θ)
7.30. The center of the membrane has a finite displacement, and the periphery at r = 1is
unsecure. The boundaries θ = 0 and θ = π/2 are held secure, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u
r
(1,θ,t)= 0
u(r, 0,t)= 0 and u
r,
π
2
,t
= 0
Initial conditions:
f(r, θ) =
4r
6
3
−r
8
sin(6θ) and g(r, θ) = 0
7.31. The center of the membrane has a finite displacement, and the periphery at r = 1is
unsecure. The boundaries θ = 0 and θ = π/2 are unsecure, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u
r
(1,θ,t)= 0
u
θ
(r, 0,t)= 0 and u
θ
r,
π
2
,t
= 0
Initial conditions:
f(r, θ) = 0 and g(r, θ) =
3r
4
2
−r
6
cos(4θ)
7.32. The center of the membrane has a finite displacement, and the periphery at r = 1is
unsecure. The boundary conditions are such that we have periodic boundary conditions
on θ, c = 1/2,γ = 1/10:
|u(0,θ,t)| < ∞ and u
r
(1,θ,t)= 0
u(r, −π, t) = u(r,π,t) and u
θ
(r, −π, t) = u
θ
(r,π,t)
Initial conditions:
f(r, θ) =
3r −r
3
sin(θ) and g(r, θ) = 0

CHAPTER 8
Nonhomogeneous Partial
Differential Equations
8.1 Introduction
Here we consider the nonhomogeneous counterpart to those partial differential equations
discussed in Chapters 3 and 4. In Chapters 3 and 4, all internal heat sources or external driving
forces were assumed to have the value zero. In addition, all boundary conditions were
assumed to be homogeneous. If either of these two conditions is not present, then the problem
becomes nonhomogeneous.
We now consider nonhomogeneous versions of both the diffusion and wave partial differential
equations over finite intervals in one spatial dimension. We consider the very generalized
nonhomogeneous problems whereby we have both nonzero external driving forces or nonzero
internal heat sources in addition to nonhomogeneous boundary conditions.
8.2 Nonhomogeneous Diffusion or Heat Equation
The generalized nonhomogeneous diffusion or heat partial differential equation in one
dimension over a finite interval I ={x |0 <x<a} has the form
∂
∂t
u(x, t) = k
∂
2
∂x
2
u(x, t)
+h(x, t)
For heat phenomena, u(x, t) denotes the magnitude of the temperature, k is the thermal
diffusivity with dimensions of distance squared per time, and the function h(x, t), with
dimensions of temperature per time, accounts for any internal heat sources within the system.
We consider this partial differential equation with the time-dependent, nonhomogeneous
boundary conditions
κ
1
u(0,t)+κ
2
u
x
(0,t)= A(t)
and
κ
3
u(a, t) +κ
4
u
x
(a, t) = B(t)
© 2009, 2003, 1999 Elsevier Inc. 477
