Appel W. Mathematics for Physics and Physicists

Подождите немного. Документ загружается.

A book’s apology x x i

Remarks on the biographical summaries

The short biographies of mathematicians which are interspersed in the text are taken from

many sources:

• Bertrand Hauchecorne and Daniel Suratteau, Des mathématiciens de A à Z, Ellipses,

1996 (French).

• The web site of Saint-Andrews University (Scotland)

www-history.mcs.st-andrews.ac.uk/history/Mathematicians

and the web site of the University of Colorado at Boulder

www.colorado.edu/education/DMP

from which certain pictures are also taken.

• The lit erary structure of scientiﬁc argument, edited by Peter Dear, University of Pennsylvania

Press, 1991.

• Simon Gindikin, Histoires de mathématiciens et de physiciens, Cassini, 2000.

• Encyclopædia Univer s alis, Paris, 1990.

Translator’s foreword

I am a mathematici an and have now forgotten most of the little physics I learned in school

(although I’ve probably picked up a little bit again by translating this book). I would like to

mention here two more reasons to learn mathematics, and why this type of book is therefore

very important.

First, physicists beneﬁt from knowing mathematics (in addition to the reasons Walter

mentioned) because, provided they immerse themselves in mathematics sufﬁciently to become

ﬂuent in its language, t h ey will gai n access to ne w intuitions. Intuitions are very different from

any set of techniques, or tools, or methods, but th ey are just as indispensable for a researcher,

and they are the hardest to come by.

A mathematician’s intuitions are very different from

those of a physicist, and to h ave both available is an enormous advantage.

The second argument is different, and may be subjective: physics is hard, much harder

in some sense than mathematics. A very simple and fundamental physical problem may

be all but impossible to solve because o f the complexity (apparent or real) of Nature. But

mathematicians know that a simple, well-formulated, natural mathematical problem (in some

sense that is impossible to quantify!) has a “simple” solution. This solution may require inventing

entirely new concepts, and may have to wait for a few hundred years before t he idea comes to

a brilliant mind, but it is there. What th is means is that i f you manage to put the physical

problem in a very natural mathematical form, the guiding principles of mathematics may lead

you to the solution. Dirac was certainly a physicist with a strong sense of such possibilities;

this led him to discover antiparticles, for instance.

Often, nothing will let you understand th e intuitio n behind some important idea except,

essentially, rediscovering by yourself the most crucial part of it.

Notation

Symbol Meaning Page

\ without (example: A \B)

⊐ “has for Laplace transform” 333

〈·, ·〉 duality bracket: 〈a, x〉 = a(x)

(·|·) scalar product 251

|ψ〉, 〈ψ| ket, bra 379,380

⌊·⌋ integral part

X Dirac comb 186

1 constant function x 7→ 1

∗ convolution product 211

⊗ tensor product 210, 439

∧ exterior product 464

∂ boundary (example: ∂Ω, boundary of Ω)

△ laplacian

 d’Alembertian 414

∼ equivalence of sequences or functions 580

≃ isomorphic to (example: E ≃ F )

≈ approximate equality (in physics)

def

= equality deﬁning the left-hand side

[a, b] closed interval : {x ∈ R ; a ¶ x ¶ b}

]a, b[ open interval : {x ∈ R ; a < x < b}

[[1, n]] {1, .. . , n}

Index of notation x x i i i

Latin letter Meaning Page

B Borel σ-algebra 58

B(a ; r), B( a ; r) open (closed) ball centered at a with radius r

Bil(E × F ,G) s pace of bilinear maps from E × F to G

∁

Ω

A complement of A in Ω 512

C C ∪{∞} 146

C (I, R) vector space of continuous real-valued functions on I

C (a ; r ) circle centered at a with radius r

any constant

D vector space of test functions 182

′

vector space of distributions 183

′

, D

′

−

vector space of distributions with bounded support 236

on the left (right)

diag(λ

, . .. , λ

) square matrix with coefﬁcients m

i j

= λ

i j

e Neper’s constant (e: electric charge)

)

basis of a vector space 433

E(X ) expectation of the r.v. X 527

F [ f ],

f Fourier transform of f 278

f Laplace transform of f 333

f transpose of f ;

f (x) = f (−x) 189

(n)

n-th derivative of f

(K) group of invertible matrices of order n over K

Gℓ(E) group of automorphisms of the vector space E

H Heaviside distribution or function 193

K, K

′

R or C

ℓ

space of sequences (u

)

n∈N

such that

< +∞ 261

space of integrable functions 280

space of square-integrable functions 262

L (E, F ) space of linear maps from E to F

L Lebesgue σ-algebra 60

(K) algebra of square matrices of order n over K

P(A) set of subsets of A 57

P(A) probability of the event A 514

R R ∪{−∞, +∞}

Re(z), Im(z) real or imaginary part of z

S space of Schwartz functions 289

′

space of tempered distributions 300

S, S

group of permutations (of n elements) 26

 transpose of a matrix : (

i j

= A

u, v, w, x vectors in E 434

pv principal value 188

X , Y , Z random variables 521

x x i v Index of notation

Greek letter

(αα

αα

)

dual basis of the basis (e

)

436

Γ Euler function 154

δ, δ

′

Dirac distribution, its derivative 185, 196

, δ

i j

Kronecker symbol 445

ǫ(σ) signature of the permutation σ 46 5

∗k

space of exterior k-forms 464

Π rectangle function 213, 279

σ(X ) standard deviation of the r.v. X 528

characteristic function of A 63

ωω

ωω,σσ

σσ differential forms 436

Abbreviations

(n).v.s. (normed) vector space

r.v. random variable 521

i.r.v. independent random variables 537

Chapter

Reminders: convergence of

sequences and series

This ﬁrst chapter, which is quite elementary, is essentially a survey of the notion

of convergence of sequences and series. Readers who are very confortable with this

concept may start reading the next chapter.

However, although the mat h ematical objects we discuss are well known in princi-

ple, they have some unexpected properties. We will see in particular that the order

of summation may be crucial to the evaluation of the series, so that changing the

order of summation may well change its sum.

We start this chapter by discussing two physical problems in which a limit process

is hidden. Each leads to an apparent paradox, which can only be resolved whe n the

underlying limit is explicitly brought to light.

1.1

The problem of limits in physics

1.1.a Two paradoxes involving kinetic energy

First paradox

Consider a truck with mass m driving at constant speed v = 60 mph on a

perfectly straight stretch of highway (think of Montana). We assume that,

friction b eing negligib le, the truck uses no gas to remain at constant speed.

2 Reminders concerning convergence

On the other hand, to accelerate, it must use (or guzzle) ℓ gallons of gas in

order to increase its kinetic energy by an amount of 1 joule. This assumption,

although it is imperfect, is physically acceptable because each gallon of gas

yields the same amount of energy.

So, when the driver decides to increase its speed to reach v

′

= 80 mph , th e

quantity of gas required to do so is equal to the difference of kinetic energy,

namely, it is

ℓ(E

′

− E

) =

ℓm(v

′2

−v

) =

ℓm(6 400 −3 600) = 1 400 ×ℓm.

With ℓ · m =

10 000

J · mile

−2

· hour

, say, this amounts to 0.14 gallon. Jolly

good.

Now, let us watch the s ame scene of the truck accelerating, as observed by

a highway patrolman, initially d riving as fast as the truck w = v = 60 mph,

but with a motorcycle wh ich is unable to go faster.

The patrolman, having his college phys ics classes at his ﬁngertips, argues as

follows: “in my own Galilean reference frame, the relative speed of the truck

was previously v

∗

= 0 and is now v

∗′

= 20 mph. To do this, the amount of

gas it has guzzled is equal to the difference in kinetic energies:

ℓ(E

∗

′

− E

∗

) =

ℓm



∗′

)

−(v

∗

)



ℓm(400 −0) = 200 ×ℓm,

or around 0.02 gallons.”

There is here a clear p roblem, and one of the two observers must be wrong.

Indeed, the Galilean relativity principle states that all Galilean reference frames

are equivalent, and computing kinetic energy in the patrolman’s reference

frame is perfectly legitimate.

How is this paradox resolved?

We will come to the solution, but ﬁrst here is another problem. The reader,

before going on to read the solutions, is earnestly invited to t hink and try to

solve the problem by herself.

Second paradox

Consider a highly elastic rubber ball in free fall as we ﬁrst see it. At some

point, it hits the g round, and we assume that this is an elastic shock.

Most h igh-school level books will describe the following argument: “as-

sume that, at the instant t = 0 when the ball h its the ground, the s peed of

the ball is v

= −10 m·s

−1

. Since the shock is elastic, there is conservation of

total energy before and after. Hence the speed of the ball after the rebound is

= −v

, or simply +10 m·s

−1

going up.”

This looks convincing enough. But it is not so impressive if seen from

the point of view of an observer who is also moving down at constant speed

obs

= v

= −10 m·s

−1

. For this observer, the speed of the ball before the

shock is v

∗

= v

− v

obs

= 0 m·s

−1

, so it has zero kinetic energy. However,

The problem of limits in physics 3

after rebounding, the speed of the ball is v

∗

= v

− v

obs

= 20 m·s

−1

, and

therefore it has nonzero kinetic energy! With the analogue of t he reasoning

above, one sh ould still have found v

∗

= v

∗

= 0 (should the ball go through

the ground?)

So there is something ﬁshy in this argument also. It is important to

remember that the fact that the right answer is found in the ﬁrst case does not imply

that the argument that leads to the answer is itself correct.

Readers who have solved the ﬁrst paradox will ﬁnd no difﬁculty in this

second one.

Paradoxes resolved

Kinetic energy is of course not the same in every reference frame. But this

is not so much the kinetic energy we are interested in; rather, we want the

difference before and after the event described.

Let’s go back to elementary mechanics. What happens, in two distinct

reference frames, to a system of N solid bodies with initial speed v

(i =

1, . . . , N ) and ﬁnal speed v

′

after s ome shock?

In the ﬁrst reference frame, the difference of kinetic energy is given by

∆E

i=1

( v

′

− v

In a second reference frame, with relative speed w with respect to the ﬁrst,

the difference is equal to

∆E

∗

i=1



( v

′

− w)

−( v

− w)



i=1

( v

′

− v

) −2 w ·

i=1

( v

′

− v

)

= ∆E

−2 w ·∆ P ,

(we use ∗ as labels for any phys ical quantity expressed in the new reference

frame), so that ∆E

∗

= ∆E

as long as the total momentum is preserved during

the shock, in other words if ∆ P = 0.

In the case of the truck and the patrolman, we did not really take the

momentum into account. In fact, the truck can accelerate because it “pushes

back” the whole earth behind it!

So, let us take up the computation with a terrestrial mass M , which is

large but not inﬁnite. We will take the limit [M → ∞] at the very end of the

computation, and more precisely, we w ill let [M /m → ∞].

At the beginning of the “experiment,” in the terrestrial reference frame,

the speed of the truck is v. At the end of the experiment, th e speed is v

′

Earth, on the other hand, has original speed V = 0, and if one remains

in the same Galilean reference frame, ﬁnal speed V

′

( v − v

′

) (because

4 Reminders concerning convergence

of conservation of total momentum).

The kinetic energy of the system at

the beginning is then

m v

and at the end it is

m v

′2

M V

′

. So, the

difference is given by

∆E

m( v

′2

− v

) +

( v − v

′

)

m( v

′2

− v

)

1 + O



i

This is the amount of gas involved! So we see that, up to negligible terms, the

ﬁrst argument gives the right answer, namely, 0.14 gallons.

We now come back to the patrolman’s frame, moving with speed w with

respect to the terrestrial frame. The initial s peed of the truck is v

∗

= v − w,

and the ﬁnal speed is v

′∗

= v

′

− w. The Earth has initial speed V

∗

= −w

and ﬁnal speed V

′

∗

= −w +

′

( v − v

′

). T he difference is now:

∆E

∗



′∗2

− v

∗2





′

∗2

− V

∗



m( v

′

− w)

−

m( v − w)

( v − v

′

) − w

−

M w

m v

′2

−

m v

+ m( v − v

′

) · w +

( v − v

′

)

−m( v − v

′

) · w,

∆E

∗

= ∆E

Hence the difference of kinetic energy is preserved, as we expected. So even

in this oth er reference frame, a correct computation shows tha t th e quantity

of gas involved is the same as before.

The pat rolman’s mistake was to forget the positive term −m( v − v

′

) · w,

corresponding to the difference of kinetic energy of the Earth in its Galilean

frame. This term does not tend to 0 as [M/m → ∞] !

From the point of view of the patrolman’s frame, 0.02 gallons are needed to

accelerate the truck, and the remaining 0.12 gallons are needed to ac celerate the Earth!

We can summarize this as a table, where T is the truck and E is the Earth.

Initial

speed

Final

speed

init. E

ﬁnal ∆E

T v v

′

m v

′2

( v

′2

− v

)

( v − v

′

)

E 0

( v − v

′

) 0

( v − v

′

)

∗

v− w v

′

− w

m( v− w)

m( v

′

− w)

( v

′2

− v

)

( v − v

′

)

∗

−w

( v − v

′

) − w

M w



( v− v

′

) − w



Note that the terrestrial reference frame is then not Galilean, since the Ear th started

“moving” under the truck’s impulsion.

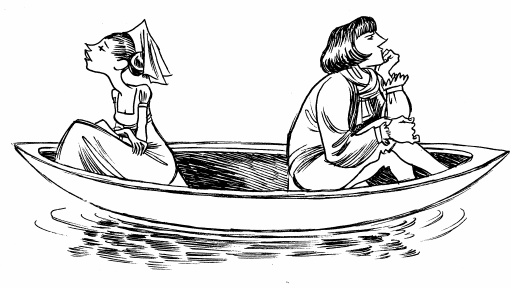
The problem of limits in physics 5

(Illustration c Craig Thompson 2001)

Fig. 1.1 — Romeo, Juliet, and the boat on a lake.

The second paradox is resolved in the same manner: the Earth’s rebound

energy must be taken into account after the sh ock with the ball.

The interested reader will ﬁnd another paradox, relating to optics, in Exer-

cise 1.3 on page 43.

1.1.b Romeo, Juliet, and viscous ﬂuids

Here is an exa mple in mechanics where a function f (x) is deﬁned on [0, +∞[,

but lim

x→0

f (x) 6= f (0).

Let us think of a summer afternoon, which Romeo and Juliet have dedi-

cated to a pleasant boat outing on a bea ut iful lake. They are sitting on each

side of their small boat, immobile over the waters. Since the atmosphere is

conducive to charming murmurs, Romeo decides to go sit by Juliet.

Denote by M the mass of the boat and Juliet together, m that of Romeo,

and L the length of th e walk from one side of the boat to the other (see

Figures 1.1 and 1.2).

Two ca ses may be considered: one where the friction of the boat on the

lake is negligible (a perfect ﬂuid), and one where it is given by the formula

f = −ηv, where f is the force exerted by the lake on the boat, v the speed of

the boat on the water, and η a viscosity coefﬁcient. We consider the problem

only on the horizontal axis, so it is one-dimensional.

We want to compute how far the boat moves

1. in the case η = 0;

2. in the case η 6= 0.

Let ℓ be this distance.

The ﬁrst case is very easy. Since no force is exerted in the horizontal plane

on the system “boat + Romeo + Juliet,” the center of gravity of this system

6 Reminders concerning convergence

(Illustration c Craig Thompson 2001)

Fig. 1.2 — Romeo moved c lo ser.

does not move during the experiment. Since Romeo travels the dist ance L

relative to th e boat, it is ea sy to deduce that the boat must cover, in the

opposite direction, the distance

ℓ =

m + M

L .

In the second case, let x(t) d enote the position of the boat and y(t) that

of Romeo, relative to the Earth, not to the boat. The equation of movement

for the center of gravity of the system is

M ¨x + m¨y = −η˙x.

We now integrate on bot h sides between t = 0 (before Romeo starts moving)

and t = +∞. Because of the f riction, we know that as [t → +∞], the speed

of the boat goes to 0 (hence also the speed of Romeo, since he will have been

long immobile with respect to the boat). Hence we have

(M ˙x + m˙y)



+∞

= 0 = −η



x(+∞) − x(0)



or ηℓ = 0. Since η 6= 0, we have ℓ = 0, which ever way Romeo moved to the

other side. In particular, if we take the limit when η → 0, h oping to obtain

the nonviscous case, we have:

lim

η→0

η>0

ℓ(η) = 0 hence lim

η→0

η>0

ℓ(η) 6= ℓ(0).