Antontsev S.N., Kazhikhov A.V., Monakhov V.N. Boundary Value Problems in Mechanics of Nonhomogeneous Fluids
Подождите немного. Документ загружается.


268
Chapter
5
degenerated on the sought solution
in
the general case
nZ
2
an
arbitrary function degenerated for ome values of the sought
solu-
tion and
so
on)
has been left open
f83,
84,
1501.
In
the present
paragraph integral estimates
of
higher derivatives, entering the
system are established under the assumption on convexity of the
P(X,
1;)
in the case
of
n
=
2
Hglder continuity
is
proved. In
paragraph
4
the theorem of uniqueness of
a
some class of solutions
is
proved. Paragraph
5
establishes some qualitative solution pro-
perties, such as the finite velocity of saturation disturbance
apreading
s(x,
t)
from initial data, and also the bounded time of
stabilization of nome boundary problems. Preliminary statements
si-
milarly to
L
9,
ll,
12
J
are established for single multidimensional
parabolic degenerating equation with integrable coefficients.
Further, in the system
(8.1),
(8.2)
p(x,
1)
E
LY(%)
is
conside-
red as
a
given function and
arising
conditions on the system coef-
ficients are expressed in terms of model functional parameters.
One should notice that
a
great number of works
67,
7l]has
been
devoted to the proof of existence of the boundek velocity of dis-
turbance spreading for one parabolic equation,
as
a
rule with
smooth
coefficients, and one space variable.
2.
Estimates for higher derivatives
Stronger estimates then those
oPi,
3
for sequences
of
smooth solu-
tions
of
regularized solutions
aE
=
a
+
E,
E
>
0
will
be estab-
lished here and in
Y
3.
These estimates remain valid for
a
limiting
solution. The limiting transition has been described in detail
in
p.
48
3
is
not being mentioned here again. For convenience index
will
be omitted further.
Note, that everywhere in this paragraph the conditions (i)
-
(iv)
s2 of
the existence of a generalized solution
of
the equation
system
(8.11,
(8.2)
are considered to be satisfied.
Consider the equation
(1.7)
(
(8.1
respectively) giving
it
the
form
-
L
1
(s)~
-
ust
+
iLi.J(i.;oao(S)
7s)
+
h,
=
0.
(9.1)
where accounting
(1.8)
and formulas
(1.19)
h
=
b1(x,
S)
7s
+
be(:<,
8)
~p
-
vs
+
b3(x1
s),
1
-+
4
+
b(s)
=
Ii,K-l,
b
=
div
P
=
div.,(i
-
bf),
x
A0
Theorem
9.1.
Let
(5,
)
E
C2+a1
(2fCL)/2
(%I)
be
a
solution of the
system
(1.71,
(1.81,
p(8.l
1,
(8.2)
respectively) and
in
addition

Filtration
of
Immiscible Liquids
269
Then there exists
a
positive number
p,
0
<
p(i.1)
<
p,
for
n5
7
as
estimate takes place
such that
J'
=
I
5'd.i~
+
I
(nosix
+
st
242
+
sx
+
+
p
-2a
px)5'dirdt
4
5
CI!~,
X
"LO
where
p
is
a diameter
of
a
carrier
U
of
the function
<(.,
L,)
in
Q
and a constant
C
depends only on
p,l,.
and the distance
from
52'
to
the boundary
I?.
Note that to satisfy the assumption
(9.2)
it
is
enough
to
require
that
9
Proof. Consider in
'to
=
Q
x
ti;,
,
G
J
an operator
-
without
loss
of
generality regarding that
u(xoj
=
1,
K0(s
)
=
I.
Integration by parts yields an equality
0
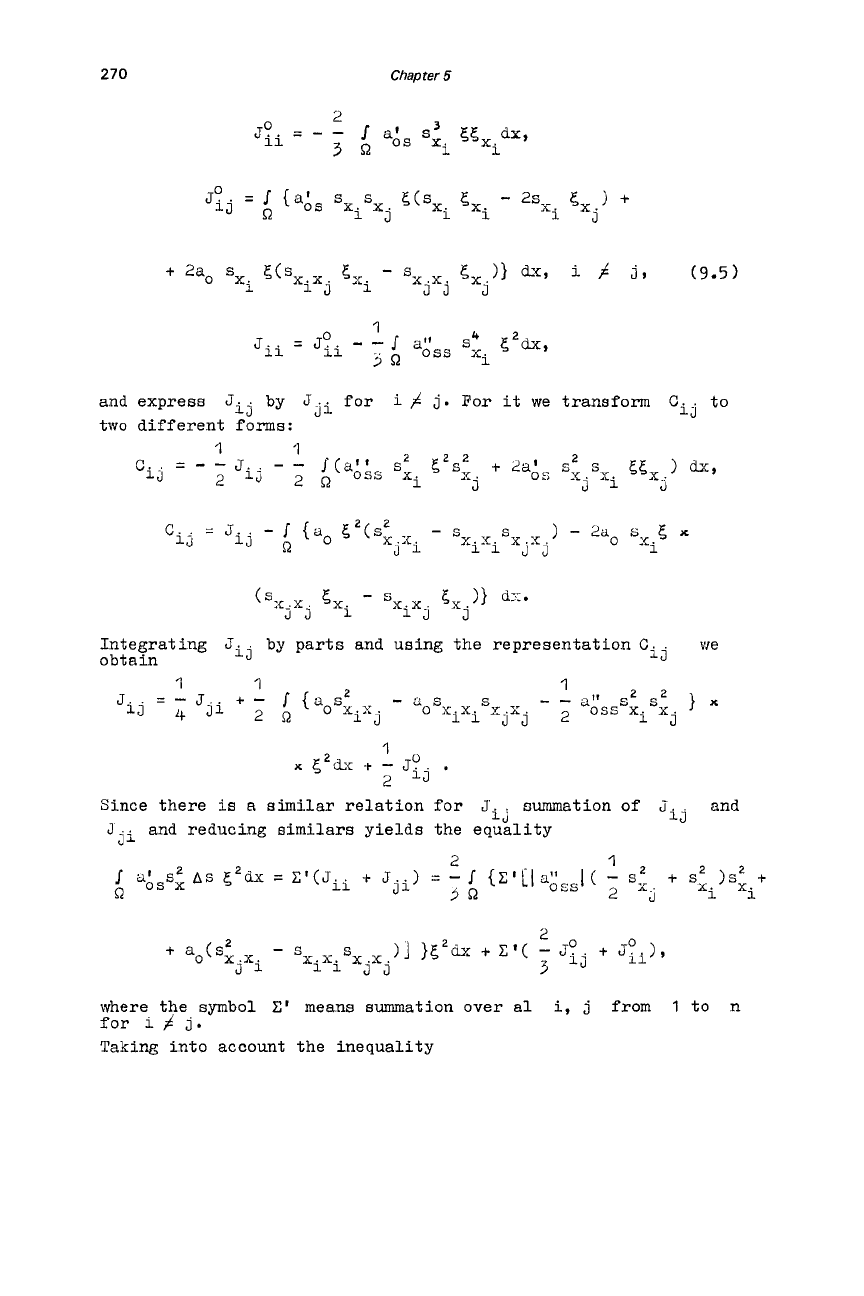
270
ChaDter
5
and express
two
different forms:
J.
.
by
Jji
for
i
#
j.
For
it we transform
C.
.
to
1J
1J
(sx,x,
Zx,
-
3
5
I}
dh*
J
J
1
x'x'
1
j
"j
obtain
IJ
1J
Integrating
J.
.
by parts and using the representation
C.
.
we
Since there
is
R
similar relation for
J.
.
summation of
j.
.
and
12
1J
J
..
and reducing similars yields the equality
J=
where the symbol
C'
means summation over a1 i,
j
from
1
to
n
for
i
#
j.
Taking into account the inequality

Filtration
of
Immiscible
Liquids
27
1
which
is
easily verified we find
that
N
Estimation the
last
addend from above by the
Young
inequality re-
sults in
V
-
.f
(LI.~S~~
+
s:)
S'dxdt
-
C
8
gA;<-'dsdt.
2
N
J
2
q0
Come back
to
(9.41, and expressing
again the
Young
inequality.
It
results in the final evaluation
s1;
from equation (9.1*) we use
J2
5
C
{I
8
Dut to (9.6) from
the
equality
Los
-
As
.
g2uiiiltI
+
K(5))
.
(9.6)
%O
we have that
(9.7)
Note that here the main terms of the
first
addend contain lower
multipliers
ni
-
ffi(xo),
IT
-
g(x
)
am
for
a
small
enough radius
p
of
the carrierd are estimated by respective positive addends
in
3'.
The most difficult nonlinear term containing pressure
gra-
dient
is
estimated by the Cauchy inequality
(IS1
<
p
1.11
0
P

272
Chapter
5
Consequently, with inequality
(8.13)
in
view for
v=
2
J,=
I
I
b:
lipxsx
AS
g2dxatl
5
€5'
+
C(E,
L,)
J'
pi
5'dXdt
5
'$0 "0
5
EJ~
+
c(p*O"
1
S;
S2dxdt
-1-
~(5)).
"0
Integrals from the rest of the addends
in
h,As
g'
are transformed
in
the following way:
and
are evaluated by the
Young
inequality
IJ
2)
<-
E
I
S.*,
1.
c2dSdb
+
c17,(<).
%O
Choosing
E
then
p
as
small enough,
we
finally come to the esti-
mate
(9.3).
The theorem
9.1
has been proved.
Theorem
9.2.
Let
in
addition
to
the assumntions of the theorem
9.
the condition be satisfied
Then there exists a positive number
p,
0
<
~(1,)
<
po
such that
for
11
=
2
an estimate takes place
(9.9)

Filtration
of
Immiscible
Liquids
273
Note that for the validity
of
the assumptions
(9.2)
and
(9.8)
of
the theorem
it
is
sufficient to satisfy the inequalities
(9.2*).
Proof. Integreting by parts and using
(9.1)
for
evaluatin, of
yields the equality
%
and the
surn
over
i'jfron
1
to
2
for
i
#
;is
designiltcrl
by
C'.
As
in
the
previous
lem.;?,
';/e
transform the term
.:to
::
all
terns
entering
Iio
exceTt
present in tvio different
form:
(9.1
1)

274
Chapter
5
n
NOVI
it
is
easy
to get that
I1
5
g2d<dt
+
Ro
=
R,
+
R,
+
Ri
+
(1
-
U)X3
+
UR!,
=
(5U-
-
)W
+
1
4
+
I
axss
C'(
-
s4
+
(
-
-
U)S;
>s2
5
xi
3
j
xi
%O
Here
meat
ioned
further.
Find
the expression
for
w.
To
do
thi8
we
preaent
w,
in
the
form
I),
=
H:
+
R:
+
E2
+
(1
-
.)I?;
+
UT,
and
the number
CL
will
be

Filtration
of
Immiscible Liquids
275
1
+
;
I
(aosx,x,~x2
-
a'
0sSx1~x2
5
XI
>s3
x2
5
bat.
'0
Addend
B
=
I
aOsx
equality (taking into account (9.111, (9.12)):
s3
g2d;cdt
is
expressed from the following
11
2x2
20
a
1
a4
L~s
-
(s3
c2)d.xdt
=
-
.f
5'
-
s
&Cdt
f
Ail
-
x
-
do
ax2
x2
4
yo
at
x2
Having introduced
B
and reducing
similars
we obtain using the
symmetry
w1
and
w2
that
Here
Consider similarly
to
the theorem 9.1 the equality
(9.15)
,[
Lys
+
(L,s
-
L:s)]
AslVsl
2<2ci~dt
=
0
4,
Substituting (9.131,
(9.14)
and (9.10)
in
(9.15)
reduce the
simi-
lam.
Regarding
CL
such that the squares
of
the second derivati-
vea,
as
well
as
the sixth powers
of
the
first
derivativee have
po-
sitive coefficients, we leave all known positive terms in the left
side and the rest are put into the
right
aide. Then
we
come
to
the
equality

276
Chapter
5
Y,
=
(2
+
1%1/~4;
Y,
=
'Y5y1
i
/2
>
0.
Eetimate the first two addenda in the right side
(9.16)
by
the
Cauchy inequality
in
the following
way:
Regard
Fng
re spec
t
ive
1
y
and reducing the similars we get that
I-P/
6~61>0,('l+~~--'E~bZ>I)
+
As1
V~1~)<~d:rdtI
+
IDII,
where
p(a)
=
15/2a
-
2/2
-
P2/4(1
-
6,)
-
4,/(3a
+
1
+
b2j
>
4
*lo-'
for
6,
=
6
=
IG-'
and
CL
=
L/>.
Since due
to
(9.2)
and
(9.8)

Filtration
of
Immiscible Liquids
277
the right side
of
the initial ine uzlity
is
evaluated by,,the con-
ventional method using smalnesslin?x>
-
dx0)l,
Iho(d
-
~~~(x~)l
and estimate
(8.14)
in which
P
is
choaen
in
the required way
is
it
has been done before. Finally we come
to
(9.9). The theorem
2
ha0 been proved.
3.
Continuity
of
solutions by Hslder
We demonstrate that the estimate
(9.9)
for
n
=
2
provides the
Hb'l-
der continuity
s(x,
b),
Ij(x,
b),
v
3
(x,
t)
in
Q'
over variables
xyb.
The proof of this fact
will
essentially use the following
result
of
S.N.
Kruzhkov [83].
Lemma
9.1.
Let a function
u(i,
L)
be continuous in
9
and be the
solution of a parabolic equation
x-
Assume that
for
a certain
to
E
[G,
'2~
and arbitrary
(xl,X2)
E
D
where
r
is
a distance from the point
x
to the boundary domaina
and
Otherwise,
in
accordance to lemma
(9.1
continuity
of
the solution
of
the degenerating equation (9.17) over
a
variable
t
results from
continuity
of
its
solutionu(x,
t)
over
a
variable x
=
(x,,
...,
xn).
Theorem
9.3.
Let
in
addition
to
the conditions
of
theorems 9.1,9.2
for
n
=
2
C,
=
Cl(i~~,
n,
Lo,
