Antontsev S.N., Kazhikhov A.V., Monakhov V.N. Boundary Value Problems in Mechanics of Nonhomogeneous Fluids

178

Chapter

4

Then, from

(3.16)

we have

UEing formulas

(3.10)

and

(3.11),

we conclude:

Besides, we shall need the estimates of HGlder's continuity

of

the

derivativea

ayi/ax,

and

L~Y~/~x~].

Let

ay/ax

and

au/ay

be the

matrices with the components

ayi/ax

.,

axi/ay

respectively.System

J

3

(3.5)

in

a

matrix form

is

as follows:

d

ay

au ay

ds

ax

ay ax

=

&,

--=-.-

(4.21)

where

E

is

a unit matrix. Let

ofQ

of

the Cauchy problem

(3.8)

with the initial data

x1

and

x2

be two arbitrary points

be solutions

and, accordingly,

yi

=

y(s,

xi,

t),

i

=

1,

2

yils=t

=

xi, i

=

1,

2.

For the difference

system

z

=

ay,/ax

-

ay,/ax

we derive from

(4.21)

the

with the zero data at

s

=

t.

Integrating with respect to

s

we

conclude

:

Let

UE

now make use of the estimates

(4.2)

and

(4.17):

x

\XI

-

x2p.

It

means that

ax

(4.22)

H:

(

-

)

5

NdT.

ax

Now let estimate the continuity

of

ay/ax

to the variable

t.

Let

LO,

T1

such that

tance from

I"

y,

=

y(s, x,

t,)

by HSlder with respect

tl

and

tp

be two arbitrary points on

-*

.

li;,

-

t,l

<

d

where

d

>

0

is

a dis-

For the sake

of

definiteness,

t,

<

t,.Let to

r2

be the solution

of

the Cauchy problem

(3.8)

with

Correctness

of

the

Problem

of

Flow

179

the initial condition y,

=

x

of the same problem with the condition

y,

=

x

ingly, let us set

sible:

z2

5

t,

If

t,

5

t,

is

defined at

s

=

t, .

Let us set

xo

=

y2(tl,

x,

t2)

y,(s>

at

s

=

t,

while

y,

be the solution

at

s

=

t,

.Accord-

zi

=

~(x,

ti),

i

=

I,

2.

Here two cases are

pos-

and

t,

<

t,

5

t,.

as a solution

of

the

Cauchy problem

the function

y,(~>

and coneider

dY

-+

ds

-

=

U(Y,

s),

Y(

=x

with the initial condition

of

the preceding estimate

s

=

t,.

In this case,

On the other hand, from the integral equation

for

Y,(S,

I,

t2>

=

x

-

s

=

t

1

we have, setting

J

S

use can be made

Y,(d

In the second case, when

z2

>

Iulo,k

*

It,

-

t21

<

d

only for

s

E[tl,

tl]

but also at

s

E

[t,,

z,].

Indeed, the solu-

tion

y,(s)

that at the moment

s

=

s,

the point

yl(sl)

E

r2

and that

t,

<

S1

<

t2

during the time

(t,

-

t,)

than

It,

-

tll

t,

that the solution

it

results from the condition

y,(s)

is

defined not

is

continued at

s

>

t,

until

y,(s)

E

R.

If

it

appears

it

means that the point

x

reaches the boundary

l?'

I"

during the

time

(Sl

-

t,).The total time

(t,

-

z,)

+

(s,

-

t,)

is

strictly

less

x

is

not less than the distance

d

between

r1

and

I"

.

Hence,

and the boundary

if

s,

<

t2

and the-distance covered by the point

s1

2

z2.

Let us set

Then

y,(s)

Cauchy problem

(3.8)

with the initial conditions

at

s

=

2,

x

1

=

Y,(t,(X,

t,),

x,

t,),

x,

=

Y,(t,(X,

t,),

x,

t,).

and

y,(s)

can be considered to be the solution to the

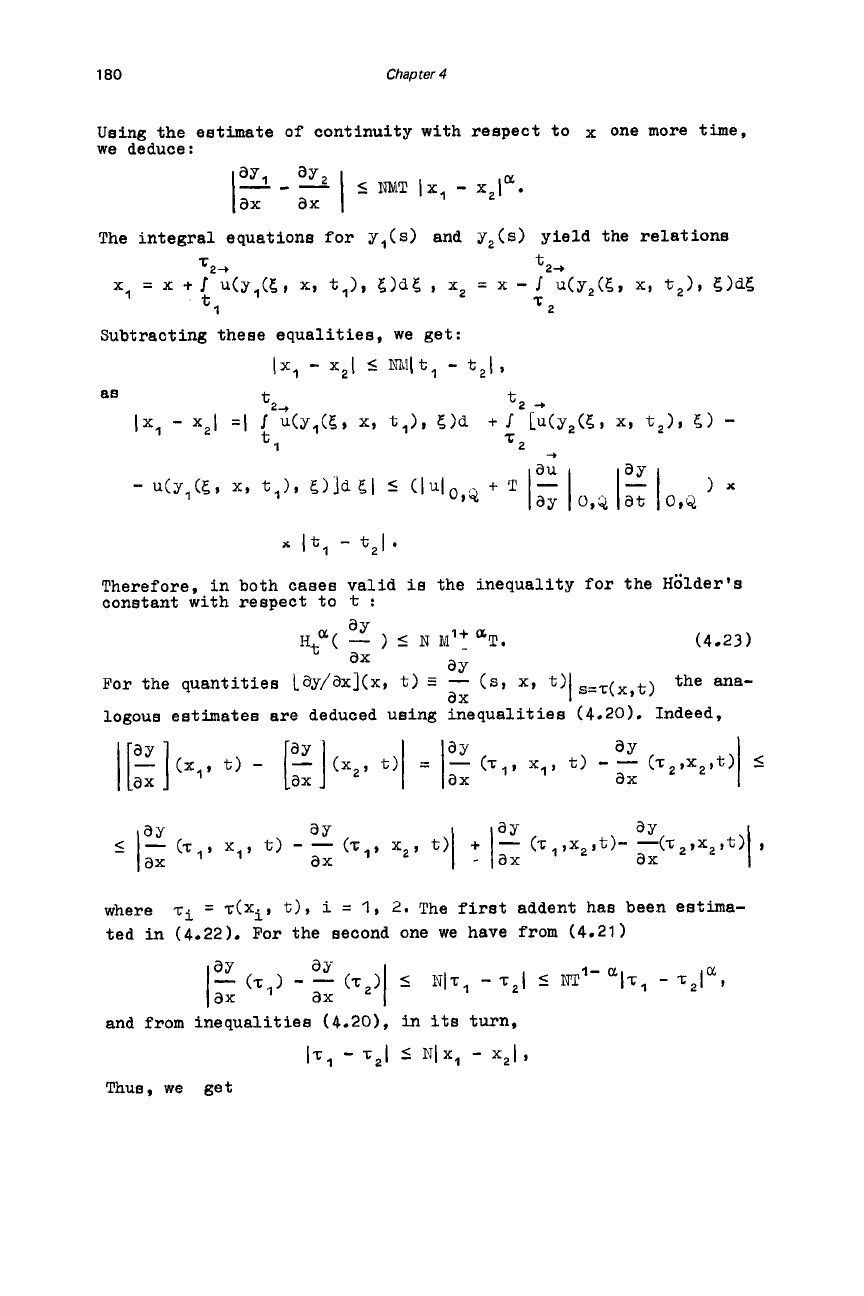
180

Chapter

4

Using the eetimate

of

continuity with respect to

x

one more time,

we deduce:

Subtracting these equalities, we get:

Ix,

-

XJ

5

J!TTl(tl

-

t21,

Therefore, in both cases valid ie the inequalit

constant with respect to

t

:

for the Holder's

(4.23)

aY

ax

Ht"(

-

)

5

N

MI:

aT.

1

aY

ax

For

the quantities

taY/ax](x,

t>

f

-

(s,

x,

t>

S=T(X,t)

the ana-

logoue estimates are deduced using inequalities (4.20). Indeed,

where

ted in (4.22).

For

the eecond one we have from (4.21)

T~

=

dXi,

$1,

i

=

1,

2.

The

first

addent has been estima-

and from inequalities (4.20),

in

its

turn,

ITl

-

T21

5

NIX,

-

X,l

9

Thus, we get

Correctness

of

the Problem

of

Flow

181

(4.24)

ax

HC([

-

1)

5

N(MT

+

TI-")

C

NT'-".

In

the same way, pn the basis-of (4.23) and (4.20) we get the

estimate of the Holder's continuity with respect to

t

:

(4.25)

aY

ax

H

:([

-

1)

5

NTI-".

4. Vortex estimates

To estimate the vortex, let

us

first

of

all

find in (3.20)

the

ad-

dents which present the greatest difficulty for estimation and

those having

a

small

parameter, i.e. which are subordinate.To begin

with, subordinate

is

z2

in (3.20). Therefore, from (3.18)

-

(3.20) we have

Here use

is

made of the estimate of the norm in

C"

for the product

If,

.

f21a

5

If&

P2I0

i.

lfll" If&-

Adding to and subtracting from

for relations (4.18), (4.241, (4.25), we get the inequality

ay/ax

the unit matrix

and

allowing

(4.26)

is

con-

+

bI",Q,

<c

I

boll",&

+

ITo*

It

should be again emphasized here that the denotation

ITo

ditional in

the

sense that

if

for the

first

addent (which

is

in-

luded

with

the

small

parameter

T)

we shall get the esthate from

above through

M,

then the second addent

No

through

M,

in which case at

T

+

0

thie value tends to

a

finite

M-independent

limit.

Then from the definition for

[$0]

given

in (3.51, (3.6)

and

(3.13)

we derive:

will

be also estimated

Here through

Q

(3.61, which are given on

S'

w

and the right-hand parts Fi and

g

in (3.6) belong to

this

set.

The second and the

third

addents in the right-hand part of (4.27).

are subordinate. IndEed, for instance, in the third addent for the

initial vorticity

w

we, due to (4.181, have

we denote all the functions employed

in

(3.5) and

or at

t,=

0.

The initial data

for

+

In an analogous way we shall operate

with

the rest of the terms

forming

[a]

For the second addent

23

+

one can use relations (4.2)

182

Chapter

4

In this

case

for the Hb'lder's constant with respect to

x

we

have

Here we use

a

simple inequality

-t

From equation (4.5)

Iu~~~,~

is

estimated through

M,

i.e.

Iu~~~,~

5

C(1

+

biz).

Hence, employing

(4.18)

and (4.20) again,

we

obtain:

a+

-i

H,

([.I>

5

EIWI~,~

+

C,N

M2(T

+

T1-a).

Hta([ul)

5

EIw~~,~

f

CEN

lul

(T

+

T

-

01+

Ta).

Analogously,

+

-t

Choosing

E

>

0

sufficiently

small,

after such-simplifying trans-

formations we deduce from inequality (4.27):

-t

14u,u

5

CI

t

v

Plla,Q

)To.

(4.28)

To

conclude deducing the

a

priori estimates,

let

us

demonstrate

that

[V

p]

pending on

M.

It

is

the central moment in the procedure

of

proving

the

a

priori estimates. The peculiarity lies in the

fact

that the

boundary values of

p

on

I"

are included

in

(3.18),

and, hence,

in

(4.281, without the

sma

1

parameter. Let

us

make use of the

procedure suggested in

[

64j. We shall

limit

ourselves by consider-

ing only

the

Holder's constants with respect to

x

and

t

since

the lower norm in C(Q)

is

estimated in an analogous way.

So,

let

x1

and

x2

be two arbitrary points in

Q.

Let

us

again

set

In this case valid

is

the obvious equality

in the

norm

C

"(c;)is estimated by the quantities de-

ti

=

z(xi,

t),

yi

=

y(ti,

xi,

t),

i

=

7,

2.

[

VPl(X,,

t)

-

[v

P1(Xl,

t)

vP(Y2,

tz)-

WY,,

z,)

=

Correctness

of

the Problem

of

Flow

183

Here

Let

us

estimate each of

Ji

separately.

In

J,

the second argument

(time) equals zero

and,

hence,

it

is

a known from the initial data

function of

Y*

Therefore,

Ji,

i

=

1,

2,

3,

are the expressions in braces.

(4.30)

a-c

ax

o,g

in which case

Itl-

t2

1-

<

T"-'~I-

1"

(4.201,

we get

1x1-x2

la

.

using (4.181,

15,

1

5

N

lxl-

x,

I"?

.

For

the second addent

J,

we have

IJ,

I

5

I

v

P(

-c2

1

-

VP(0)

la,

r'

lY1-

Y,

I"

-

Using

inequalities (4.16) and allowing for the fact

that

I

1'

we deduce:

05

2,

5

If

for the difference

I

jl-y2

1

we use relation

(4.301,

we get

I

J,

I

IT

Ixl-

x2

1"

.

Therefore,

it's

now turn to estimate the addent

First,

J,

in

(4.29).

1

J,

15

1

VP(

2,

)

-

vY(

tl)

I

0,ll

'

With the inequality

of

imbedding one can estimate

where

Q

'

is

an

arbitrary subdomain

Q

whose boundary belongs

to

r1

Uaing

(4.15)

and (4.16) and taking into account that

/T

-

T

~

I

5

1

5

$11

x1

I

we get:

IJ,

15

IT(l+

I.,

+

KT

a(l+)/(5*)

)P

T

P

Jxl-

xz

(a

,

where

p=

5+~

.

3+

a

,

p

=

5

5+

~

cI

.

Finally, we deduce

from

(4.29)

:

The same relation

is

valid

for

the Hb'lder's

constant with res-

pect

to

t

.

Therefore, finally, (4.28)

yields

the inequality

€1:

<[

81.'

j

5

;:

.

I

G

ITl

9

184

Chapter

4

where the quantity

N,

depends

on

hi

and

T

in

a

continuous way,

and at

T

-0

has a

limit,

which

is

M-independent. This inequality

is

an a priori estimate for the vortex, obtained

at

the k-th itera-

tion: through the norm in

C

a(q)

of

the preceding approximation

that at sufficiently small values

T

5

To

there

is

such

!do

(T)

that

N1(Mo,

T)

5

Moo

in

C

"(Q)

is

self-mapped by the transformation

wk-'

-+

uk

following uniform estimate

is

valid:

.

It

reaulta from the properties of the quantity

N,

=

N1(IVl,T)

;k-

1

It

denotes that a sphere

2f

the fadius

Mo

i.e. the

(4.31)

-'k

1.

)a,61

5

Ido,

k

=

1,

2,

...

.

5.

PROOF

OF

THE

EXISTENCE

AND

TKE

UNIQUENESS

OF

THE

SOLUTION

On the basis of the a priori estimates deduced above, the proof

of

the solvability

of

problem (1.1)

-

(1.6)

can be carried out in

two

weys. The shortest way

is

related to the Shauder

-

Tikhonov theo-

rem on a fixed point. Namely, in a Banach space

let

us

take the set

~

CB(Q),

0

<

B

<

CL

and define the

A

mapping on

K

according to the dame rule that

is

used for the mapping of

Gk-'

+

;k.

ined ins

4

that the operator

A

maps

K

into

K,

being continuous

in the norm

C

@(d),

0

<

p<cf.Jn this case the Shauder

-

Tikhonov

theorem affords the existence of at least one fixed point of the

operator on the set

K.

It

should be noted that this method

of

pro-

ving solvabi ity in the Holder's spaces

Ca

has been employed by

T.

Kato [173f in the problem with the boundary conditions of non-

-flowing

u,

=O

throughout the whole boundary

r.

The second method, which

is

a

constructive one,

is

based on the

application of the method of successive approximations, described

in

93.

When using this method, however, one must not forget that

there

is

a peculiarity associated with the fact that in the norm

of

the space

C

the operator

A

is

not a Lipahitz's one even

in the

small

relative to time. Indeed, at one

of

the stages of con-

structing the operator

A

i.e. when the solving problem (3.5),one

has to solve the Cauchy problem (3.8). In this case the trajectory

y(s)

=

y(s,

x,

t)

is

to be considered a result

of

the action

of

a

certain operator

A,

on

the right-hand part

3.

Let

d,

and

d,

be

two different vector fields, and

y,(s),

y,(s) be two solutions of

the Cauchy problem (3.8):

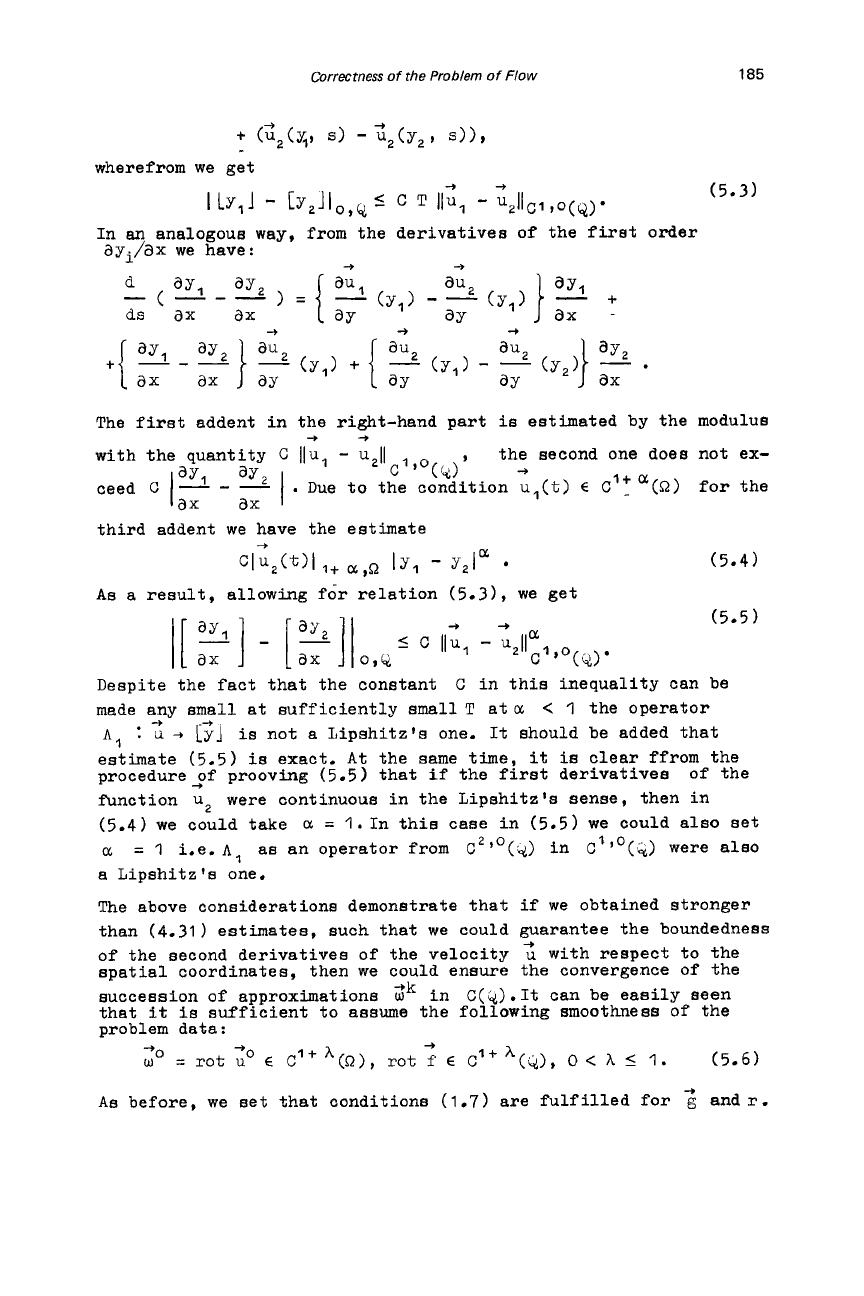
It

results from estimates obta-

Then for the difference y,

-

y,

we, obviously, have

d

-I

-3

-+

ds

-

(Y,

-

Y,)

=

U,(Y,,

9)

-

U2(Y2,

9)

=

(U,(Y,,S>

-

U,(Y,,S>>+

Correctness

of

the

Problem

of

Flow

185

wherefrom we get

(5.3)

In

an

analogous way, from the derivatives

of

the

first

order

a

yi/3

x

we have

:

The

first

addent in the right-hand part

-3-3

is

estimated by the modulus

the second one does not ex-

with the quantity

c

llu,

-

upll

ceed

C

-

1.

Due to the condition

u,(t)

E

C’y

CL(Q)

for

the

ay, ay,

c1

,O(Q)’

-f

lax

ax

third addent we have the

-+

Clu,(t)l

,+

estimate

CL

@,Q

IY,

-

Y21

AS

a result, allowing for relation

(5.31,

we get

(5.4)

Despite the fact that the constant

made any small at sufficiently

small

T

at

CL

<

1

the operator

A,

estimate

(5.5)

is

exact.

At

the same time,

it

is

clear ffrom the

procedure2f prooving

(5.5)

that

if

the

first

derivatives

function

up

were continuous in the Lipshitz’s sense, then in

(5.4)

we could take

CL

=

1.

In this case in

(5.5)

we could also set

cL

=

I

i.e.

A,

as an operator from

c2*O(4)

in

C‘,O(~)

were also

a Lipshitz’s one.

The above considerations demonstrate that

if

we obtained stronger

than

(4.31)

estimates, such that we could guarantee the boundedneas

of the second derivatives of the velocity

“u

with respect to the

spatial coordinates, then we could ensure the convergence of the

succession of approximations

Gk

in

C(q).

It

can be easily seen

that

it

is

sufficient to assume the following smoothness of the

problem data:

C

in this inequality can be

-+-+

u

4

Lyj

is

not a Lipshitz’s one.

It

should be added that

of the

-3

Go

=

rot

uo

E

C1+A(Q),

rot

f

E

~“’(q),

O<

A

5

1.

(5.6)

As

before, we set that conditions

(1.7)

are fulfilled

for

”g

and

r.

186

Char,

ter

4

In this case formula

(3.20)

yields that

Jk(t)

E

C1+*(S2)

hence,

u

(t>

E

C2(Q)

i.e. the second derivatives

of

the velocity

are uniformly bounded with respect to modulus over all

k.

In

this connection it should

be

stressed that under the conditions

of

Theorem

1.1

on the Bmoothness

of

the initial data and the right-

-hand part, the formula

of

type

(3.20)

for a vortex gives a genera-

lized solution of the Helmholtz equations

(2.1).

The initial Euler

equations are, however, fulfilled in a classical sense. The genera-

lized solution

(2.1)

obeys the integral identity

and,

-rk

-r+

+

-r -r

++

+--t

/

{(w,

Qb

+

(u

*v

)a)

-

((w

.v

)Q,

u)

-

(rot

f,

jP)}dx

dt

=

0

Q

-+

for any smooth and finite in

Q

vector-function

a.

And, since

+

w

=

rot

2

integration by parts results in the equality

which implies the fulfilment

of

equations

(1.1)

in a classical

form

due

to

the smoothness properties

of

the velocity

“u

By way of conclusion, let us consider the problem

of

uniqueness

of

the solution

of

problem

(1.1)

-

(1.6).

Let us demonstrate that uni-

queness takes place in a wider class, i.e. under the condition

of

boundedness

of

the first velocity derivatives with respect

to

the

variables

x.

Let

(c,,

p,)

and

G,,

p,)

be

two

possible different

solutions to problem

(1.1)

-

(1.6).

Then the differences

3

=

z,,-”u2,

=

PI

-

P,

form

the solution

of

the system of equations

a%

+

-+-r

-r -r

at

-

+

(ul

v)w

+

(w

V)u?

+

q

=

0,

div

w

=

0

with the zero initial

and

boundary conditions. Multiplying in a

scalar way in

L2(Q)

by

“w

we get the equality

-9

t+

-r-r

t

-9

Ilw(t>l12

=

-

.f((w

v

)u,,

w)dz

-/

ylwl

dT

(5.7)

0

or

On the part

r’

and at

t

=

0

we have

%

=

0

on

r0

and

y

>

Oon

I?‘

thus, the last integral is non-negative.

Since the first derivatives

of

3,

are bounded, the preceding for-

mula affords

and, hence,

y

=

c)

wherefrom we have

4

0.

This is the end

of

the proof

of

Theorem

1.1.

Correctness

of

the Problem

of

Flow

6.

SOm

GENERALIZATIONS

OF

THE

BASIC

THEOREM

187

1.

The

Let us

bed by

case of non-homogenous fluid

consider the flow of a non-homogenous ideal liquid, descri-

the systp

The problem of the liquid flowing through the domain

Q

is

posed in

the following way.

At

the

initial

moment

t

=

0

we set that the ve-

locity field and density distribution are known:

in

which case

po(x>

is

a strictly positive bounded function:

o

<

m

5

po(x>

5

M

<

m

,

x

c

Q.

(6.3)

As

before, on the impermiable part

of

the boundary

r0

the condition of non-flowing through

On the part of the flowing in

r1

density

we formulate

-3

(6.4)

un

=

(3

.

n)

=

0,

(x,

tj

E

SO.

we set the velocity vector and

+-#

u

=

g(x,

ti,

p=

pl(x, t),(x, t)

E

S',

gn<

collst

<

0,

(6.5)

in which case

p1

is

also a positive and bounded function:

1

o

<

m

5

p

(x,

t>

<:

IN

<

m,

(x,

t)

E

s'.

Besides, the functions

Po

or

p'

constant, since

if

they are, then

p(x,

t)

const, i.e. the

liquid

is

homogenous.

Then, on the part of flowing out, as before,

we

set the normal

component

of

the velocity

are not identically equal to the

(6.6)

un

=

r(x,

t>

>

0,

(x,

t>

E

s',

and, finally, the condition of the pressure normalization

s1

Therefore, in the model

of

a non-homogenous fluid the formulation

of the problem

is

modified with the

initial

data and the boundary

conditions

on

S1

for density

p.

Account taken of the fluid non-homogeneity does not prove to ham-

per significantly the investigation of the local solvability of

the problem

of

flowing through.

It

can attributed to the fact that,

first,

the equations

of

imcompreseibility afford

Antontsev S.N., Kazhikhov A.V., Monakhov V.N. Boundary Value Problems in Mechanics of Nonhomogeneous Fluids

Подождите немного. Документ загружается.