Antontsev S.N., Kazhikhov A.V., Monakhov V.N. Boundary Value Problems in Mechanics of Nonhomogeneous Fluids
Подождите немного. Документ загружается.


158
Chapter
4
+-+
In the second addent
-4-D
12WJ
cp
((g,
-
v,
1.
v,g)
dr
1
r”
of the Cauchy inequality
TE
let us make use of the boundedness
and of kthe imbedding
W;(Q)
in
L,(r)
II
CP
ll2,yl
5
C
II
V
CP
II
(2.22)
As
a result, we get
1
4
1,
5
-
II
v
cpl12
+
c
I
Ig
II
ZT
4,
I2
.
r’
The laet, fourth, addent
is
estimated in an analogous way:
Now
let us deduce the same stimate for the third
term
-+-+
3
3
1,s
J”
c;(($
-v,>*
VT(
1%
I
2
-
Iv,
I2>)d
I’
r’
For
this
purpose we should transform
it
in
such a way a8
to
ex-
clude the derivativee from the difference
-
v,
preserving
only the derivatives from the
Bum
&
+
3,
addents in the expression for
I,
respect to
(&
Then
-4
and as to have all the
only in the quadratic order
with
*
For simplicity, lat
us
denote
-go4
‘V,
-9
,%=.,+%,
.
3
-b
-+-b
-b
3-B
16,
I2-1vT
I
=
(aob),
(a.
V,(a
b
))
=
3
-9-3
-9
-b+
=
((a
*
0,
)b
a
)+((a
V,
)a*
b
).
In view of the boundedness
of
3
and
its
first
derivatives, we
have
Let us now estimate the last expression
((3.
v,)
can distinguish two groups of terms, containing the derivatives
a-
b
where we
from the components
of
2-
The
first
groups
is
a
ai
aa;
ai-b.z-
1
-
bi
,
i
=
1,2
.
a
‘li
2
a¶l

Correctness
of
the Problem
of
Flow
159
The second group
is
presented
as
ai
aa.
2
bj
,
i,
j
=
1,2,
i
#
j
.
a
si
In the
first
group, after integration by parts, we obtain
a
quadra-
tic form of
ai
with bounded coefficients. In the second group
let
use equality (2..17) which can be written in the
following
way
I
aHi
aH.
,
ifj
aa.
H
aai
-11=2
-
+ai-
--a.d
asi
H.
as.
-
H.
aq
asi
JJ
~j
a
ai
,,
aai
asj
J
2
asj
As
a
result, we get
a
combination
of
the terms with the derivatives
from a.
b.
i#j.
J'
a.
-
b.E
-
-
After integration by parts, transferring differentiation to
b.
and
Hk
we get
a
quadratic fom of
ai.
Thus, we obtain the required
J
estimate
++
I,
5
c/
~g
j
~g,
-
vT
1'
dr.
r1
From (2.20), using the obtained estimates for
we get the inequality
Ik,
k=l,..
.,
4
+
I
EJjt-
vt
(2.23)
1
dI'
At
t
=
0,
from the conditions of agreement between the initial and
boundary data we have
equality
and
+
%
=
$,
on
1"
Then from the preceding in-
(2.18) by the Gronewall
lemma
we conclude:
+
1
cp(x,t)
=
0,
(x,t;)
E
q;
vt
(x,t>
=&
(x,t),
(x,t>
E
s
.
It
means that
in problem (2.1)
-
(2.11) the boundary conditions
on
for the tangential velocity components are fulfilled automa-
tically, and that the function
p
determined from equation (2.2)
and boundary conditions (2.4)
-
(2.6)
is
the pressure
in
the Euler
equations (1.1). Thus, Lemma 2.1
is
proven.
The formulation of the problem of flowing through (1.1)
-
(1.6) in
the form (2.1)
-
(2.11
)
is
convenient since
it
ennables one to re-
duce the problem
to
an
operator equation with
a
continuous opera-
tor.
It
can be carried out, for instance, in such a way. Having
chosen an arbitrary field of the vortex
W,
let us
first
find the
velocity field
z1
from problem (2.31,
(2.8).
Then, from the Neu-
mann problem (2.21, (2.4)
-
(2.6) we can determine the pressure,
and, hence, from formulas (2.9).
(2.10) we can find the boundary
values of the vortex on
I'
.Then
we
constsuct
a
new field of the
1
-#
1

160
Chapter
4
vortex
z,
from the linear Helmholz equations (2.1) (on the given
velocity vector) by the known values on
easily see that the operator
A
:
3,
-t
d,
is
continuous in
P(u>,
given the solution of the problem. When constructing the
A
map-
ping, however, one should not forget that neither the Neumann prob-
lem
for
pressure no the problem
of
finding the velocity field by
the vortex are absolutely solvable. To ensure their solvability,
we have to make some additional transformations in the formulation
of problem (2.1)
-
(2.111,
the changes referring only to the lower
terms
in
the boundary conditions, since the property of the opera-
tor continuity must be preserved.
Namely, instead
of
conditions (2.4)
-
(2.111,
let us consider the
following boundary problem for system (2.1)
-
(2.3).
Let
us
take
the boundary data for pressure in euch
a
form:
r'
and
at
t
=
0.
We can
0
<
ct
(1
and that the fized point
of
this operator,
z=
6
3
ap
2
I
aHi
-
=
c
v;--
,
(x,
t)
E
so
,
an
i=i
H,H~
as,
(2.24)
(2.26)
I
P(
x,t)
dx
=
o
,
t
E
10,
tr
1.
a
Comparing (2.4)
-
(2.6) and (2.24)
-
(2.261,
we
see that the condi-
tions
on
vector
but only in the term where
these quantities
are
included without their derivatives. In a par-
ticular case, when
g
=
g(t)
and
I'lis
a
plane-cross-aection,
(2.5) and (2.25) coincide.
Then, conditions (2.8) for system (2.23) remain unchanged:
(2.27)
I"
have been changed, the tangential components of the
have been replaced with
%z
0,
x
E
1'O
6,
x
r,
x
E
I>*
(2.28)
E
PI,
t
E
[O,T].
un=y=

Correctness
of
the Problem
of
Flow
161
Finally, let us write the boundary conditions for the vortex on
in such
a
way:
Compared with (2.101, in the right-hand parts
of
(2.301, the
com-
ponents
g,
and
g,
are replaced with
v1,v2
and again only in
the derivativeless terms.
Besides, there remain the initial :,condition
=
Jo(x>
=
rot
uo(x)
,
x
E
Q
(2.31)
Formulation (2.1)
-
(2.31,
(2.24)
-
(2.31) also proves to be equi-
valent to the initial problem.
Lemma 2.2.
If
(
J,
p,
3)
is
a
classical
solution of system (2.1)
-
(2.3) with condition8 (2.24)
-
(2,31), then
6
,
p)
is
the solu-
tion to problem (1.1)
-
(1-6).
Proof.
As
in
Lemma
2.1, we assume that equations (1.1 with cer-
tain
n
are fulfilled. For the difference, again, there
arises
an
equation
with the boundary conditions on
1"
u
I"
+
(t=o
A'p=O,
xEQ(cpZp-
X),
acp
-
=o,
x
era
UP.
an
and on
I"
Then we repeat
in
detail the deduction of equalities (2.19),
(2.201, make use of the Cauchy inequalities and (2.22)
result, get
a
relation
of
type (2.231, which affords
cp
5
0,
(2.24)
-
(2.31) and conditions (2.4)
-
(2.11) result
in
a conti-
and,
as
a
Vz
5
g,.
Thus,
the lemma
is
proven. Both the boundary Conditions
+

162
Chapter
4
nuous operator
the conditions
of
solvability are fulfilled at every etage of con-
struction. This statement
will
be verified below when describing
the method of successive approximations and when obtaining a priori
eat hate
8.
3.
THE
METHOD
OF
SUCCESSIVE
APPROXIMATIONS
The solution
of
problem (1.1)
-
(1.6!
will
be sought by the method
of
iterations, making use
of
the equivalent formulation (2.1)
-
(2.31, (2.24)
-
(2.31). Let us take the initial data at
t
=
0
as
the zero approximation.
If
(
W
9
P
t
is
the approxima-
tion numbered
k-1
A:
3,
-9
G2.
Their advantage lies in the fact that
-9
k-I
IS-I
;k-I
then
Fn
order to find the next approximation
k
-‘k
(
ik,
p
,u
)
one must, one by one, solve the three problems:
+
-9
a) to determine the velocity field
b)
to
find the pressure
pk
son equation; in this case the
ri
ht-hand part
of
equation (2.3)
and boundary conditions (2.24)
-
$2.26) are calculated by the
obtained u
,
c) to construct a vortex field from the Helmgolz equation (2.1) at
a given velocity field
u
with the additional boundary conditions
on rlof type (2.29), (2.30). where the
pk
and
bk
values have
been substituted.
It
should be noted that the condition
of
solvability of the
first
problem on finding
Gk
by
Gk-’
lies in the fact that the equali-
ties
uk
by the vortex
wk-’,
from the Neumann problem for the Pois-
-‘k
-‘k
-D
+ +
div
wk-’
=
0
,
x
E
SZ
,
1
(
uk-’.
n
)d
C
=
0.
C,
must hold for any component
of
the connectedness
dary
r.
Therefore, in order to
go
over to the next approximation,
one must ensure the fulfilment of the above equalities
in
the third
problem on conetructing the field
the condition of solvability
of
the Neumann problem must be guaran-
k
teed in the second problem for
P
too.
So,
let us consider each of the three stages of finding the k-th
approximat ion.
Problem
1.
Reconstruction of the solenoidal velocity field by the
vortex and the normal component on the boundary:
C,
of
the boun-
zk
Besides, the fulfilment
of
(3.1)
-b
C:k
n
I=
y(x,t>,
x
E
r,
t
E
Lo,TJ.

Correctness
of
the Problem
of
Flow
163
This roblem has been thorou ly studied in theoretical hydrodyna-
mica ?see
182,
part
I,
Ch.
Ve
as
well
as
LEO]).
The solution
is
c3rried out in several stages. First, we expand the vortex field
wk-'
onto the whole
of
the space
and solenoidal outside the domain
Q
R2
aasuming
it
to be potential
-D
Zk-l
(x,t)
=
V
Ek"(x,t),
div
uk-'
=
0,
x
E
R2
\
?i
,
and preserving the continuity
of
the normal component
(
Zk-l
."n)
when crossing .the boundary
r
The fulfilment of the condition of solvability of this problem
is
ensured by the equality
*?I
Id
C
=
0
which
will
be veri-
+
k-
1
(
w
%l
vied below when constructing the vortex field.
The solution to the problem can be expressed through the Green
function of the Neumann problem
[
131
1
Then we can find the
rot
iit
=
tok-'
+
-*
velocity field
u:
set in
R'
and such that
"k
cliv
u1
=
0
.
,x
ER'
\Q
p-1
-t.
For
this purpose we shall introduce the vector potential
YK,
+k
'k
u
=
rot
Y
,
+,
in which case, without violating generality, we can set
div
YK=
9.
Indeed,
if
div
Y
#,O
then we can choose pch a scalar function
Then the field
yL=
'k
Y
+
Vqk
will"
in
all
probability, have
the
properties div
yk
=O,rot
$:
=
rot
Y
,
because
of
rot
(
vq
)
50
.
"k
nkthat
hvk
=
-div
yk.
k
k
+
Thus, we shall seek
div
3:
=
0
:k
=
rot
Gk,div
yk=O.
The condition
"k
is
automatically fulfilled, since
div(rot
Y
)=
0
while -'for
*k
i.e.
AY
space
R'
Newtonian
+-
determining
yk
we have the
"k
"k-I
rot
u1
=
w1
'
=
-
wk-l
as far as
rot
(rot
the solution
of
the Poisson
potential
1131
J
second equation
"
Gk)=
-
nyk.
Throughout the
equation
is
given by the

164
Chapter
4
'k
u,=
rot
Gk
in the domain
Q
obeys the
Therefore, the vector field
system of equations
rot
'k
u1
=
3'-1
,
diva!
=
0
.
'k
'
(u,*
n>
Let us denote the boundary values
of'
the normal component
k
k
through
y,
and then find the potential solenoidal field
V(P,
from the Neumann problem
Then, obviously, the
sum
ia
the solution of the initial problem
1.
It
should be noted that
the condition of the problem solvability for
+cp,is
fulfilled due
to the assumptions on
y
and the property
div
u':
=
0.
vector
Zk-'
through the solution of the Neu-
mann problems
for
the Laplace equation and through the volume po-
tential. The properties of the solutions of such problems are well
known (see,
for
instance,
[42,
43,
13111,
and aome of them
will
be
made uae
of
below.
The second stage of constructing the k-th approximation
is
solving
the Neumann problem
for
the pressure.
Problem
2.
This
is
the definition of the pressure
k
Thus, the
is
determined by
pk
:
kz
"-ffi,(X,
t)
E
s'
,
+
c
UVi)
+E2)--
2
i=l
H,H~
as,

Correctness
of
the Problem
of
Flow
165
Here, analogouely with Problem
1
,<,
ponents of the vector
tk
solvability of the problem under discussion
is
as
follows
i
P
1,
2,
3,
-
are the com-
q.
The condition of
in the coordinates
Let
us
show that
it
is
fulfilled. For this purpose we present the
right-hand
part
of equation
(3.2)
in
a divergent form and
then in-
tegrate
it,
using the Gauss-Ostrogradsky formula:
-9:
-+
If
we write the expression
((Zk
V)u
;-)=i;
the coordinates
q
and
take into ac$ount tlde pfoperty
div
u
the value of
(6
.
V)U
a
n)
on
r
equals
then we see that
where
y
is
defined with relation
(2.28).
Hence, the expression
k
-
((ck-
V>zk.”n>
on
P
is,
accordingly, equal to
dP
/
an
on
1’’
Thus, in
(3.3)
we have
a6
ar
k
a+
1
div,
tg(vk
-g
)jd
r
k
I
AP
dx
=
.f
ap
dr+J
-
d”+J
-
52
ran
riat
r2at
‘Gz
(3.4)
In line with
the Gause-Ostrogradsky formula, the last integral
equals
zero.
From the condition of agreement between the functiona

166
Chapter
4
g
and
r
and the equation
divbk
=
0
it
follows
that
the sum of
the
second and third addents in the right-hand part of
(3.4)
is
also equal to zero.
Thus,
we have proved that Problem
2
is
unambi-
guously solvable.
Problem
3.
Here
is
the vortex field
zk
+k
-9
-9
w-
+
<TP*
o);k
-
(
Zk.
v>
uk
=
rot
f,
(x,t>
e
k,
at
Zk
=
;;
(x,t>, (x, t)
ES1
u
Q,
.
(3.5)
-b
Here
a,={
(;c,t)lx
E
52
9
t
=
0
}
and the vector
uk
which
is
set
on
with
go
,
-9
On the surface
S1
=
PI
x(O,T)
the vector
Z"
is
given in the
projections
the formulas
s1
n
Q,,
is
determined in the following way: on
8,
it
coicides
-b
uk
=
uo
(x>
z
rot
uo(x>,
(x,~;)
E
Q,
.
0
0
<Eon the axes of the curvilinear coordinates
c1
by
As
has been noted earlier, when solving
this
problem,
it
is
neces-
sary to ensure the property of the vector
Efk
solenoidality.
Lemma
3.1.
Valid
is
the equality
div$k
(x,
t)
f
0,
(x,t)
E
%.
Qk
5
divZk
obeys
a
linear equation
Proof. Applying to equations
(3.5)
the operation div, we get that
the scalar function
At
t
=
0,
we have
--*
-D
ek
I
=
div
uo
=
div
(rot
uo
)
=
0
.
1
Hence,
if
Ok
f
0
on
r
then we have
Ok
I
0
in
4.
Thus, the
proof of the Lemma
is
reduced to the verification of the equality
div
EfK
=
0
on
1''
Since on
r'
all
the vector
3"
components are
given, then the derivatives
with
respect to the tangential direc-
tions from the tangential components
known.
As
to the derivative
with
respect to the normal from the
G:,
5;
are also
known.As
to
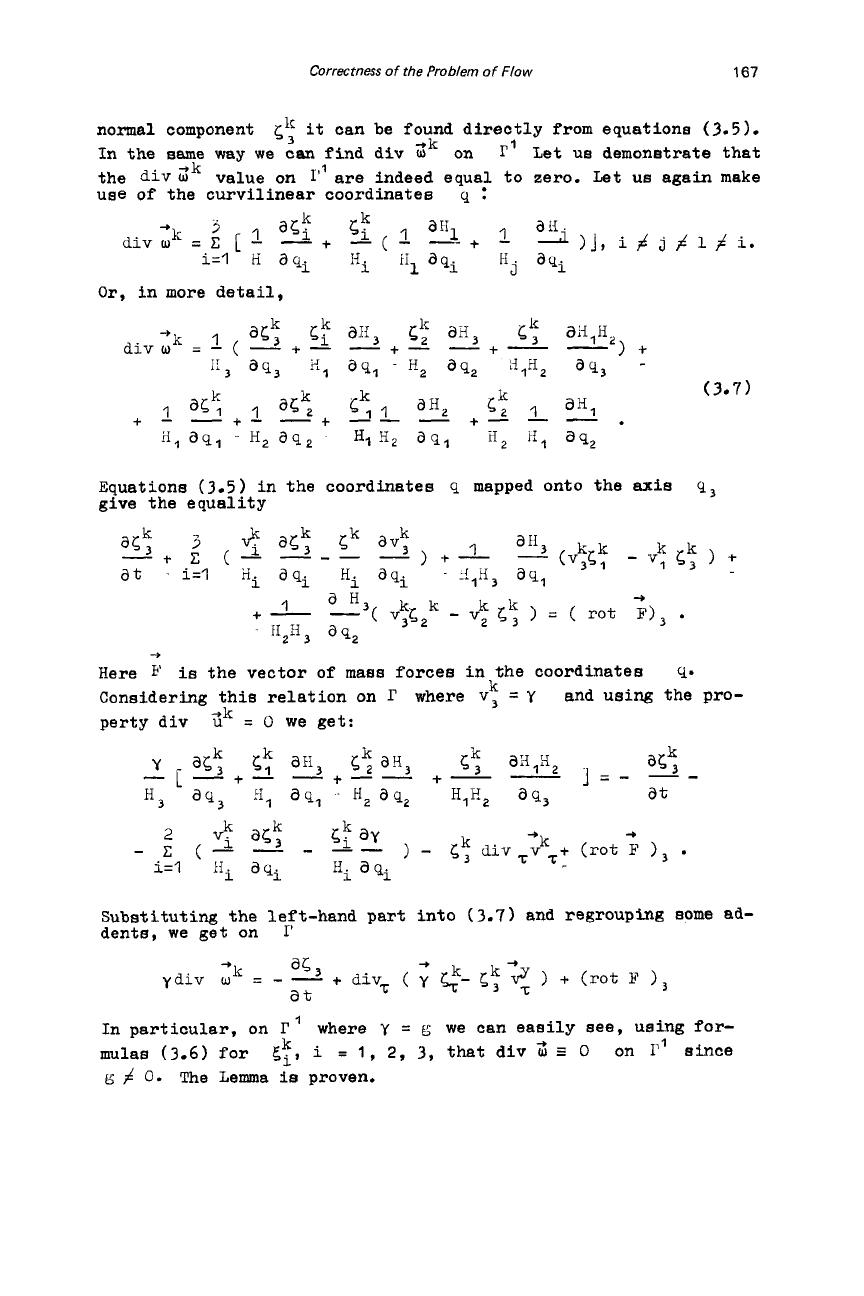
Correctness
of
the
Problem
of
Flow
167
normal component
<:
it
can be found directly from equations
(3.5).
In the same way we
can
find div
Gk
on
r1
Let
us
demonstrate that
the
div
zk
value on
I'
are indeed equa; to zero.
Let
us again make
uae
of
the curvilinear coordinates
y
.
1
(3.7)
Equations
(3.5)
in the coordinates
9
mapped onto the
axis
give the equality
q,
-3
Here
is
the vector of
mass
forces in the coordinates
ci-
Considering this relation on
r
where
v,
=
Y
and using the
pro-
perty div
k
"uk
=
0
we get:
y
as:
5,
k
ail,
<2
k
aH,
5;
a:{,x,
as:
]=-
--
-[-+-
-
+--
+-
-
H,
aq,
9,
as,
-
H,
as,
H,H,
aq,
at
Substituting the left-hand part into
(3.7)
and regrouping some ad-
dents, we get on
I'
-3
ydiv
wk
=
-
5
+
divz
(
$-
ctT
)
+
(rot
P
),
at
In particular,
on
r'
where
y
=
g
we can easily see, using
for-
mulas
(3.6)
for
on
I"
Since
g
#
0.
The Lemma
ie
proven.
k
gi,
i
=
1,
2,
3,
that
div
3
e
0
