Ames W.F., Roger C. Nonlinear equations in the applied sciences. Volume 185
Подождите немного. Документ загружается.


440
R.
W.
Ogden
where
A;
>
0,i
E
{1,2,3},
are the
principal stretches
and
~('1,
the
(unit) eigenvectors
of
U,
are
called the
Lugrangean
principal
aces.
Similarly,
V
has the spectral decomposition
where
v(')
=
Ru('),i
E
{1,2,3}.
It follows from
(3)-(5)
that
Let
dA
=
NdA
denote
a
vector surfa.ce area element
on
dB,,
where
N
is
the unit outward normal to the surface, aiid
da
nda
the
corresponding area element on
at?.
Then, the area elements are
connected according to
Nanson's fornzvla
nda
=
JA-~N~A,
(8)
where
A-T
=
(A-')T
and
denotes the transpose.
If
dV
and
dv
denote volume elemeiits in
B,
and
f?
respectively then we also have
dv
=
JdV.
(9)
For
an
isochora'c
(volume-preserving) deformation we have
At this point we
also
note that the
mass
conservation equation
may
be written in the form
Pr
=
PJ,
(11)
where
pz
and
p
are the
mass
densities
in
8,
aiid
B
respectively.
2.2
Stress and Balance Laws
We use the notation
t
for tlie (surface) force per unit area
on
the
vector area element
da.
Then
t
depends on
n
according to the
for-
mula
t=T
n,
(12)
T
Nonlinear Elasticity
441
where
T,
a
second-order tensor independent of
n,
is called the
Cauchy
stress tensor,
while
t
is
also
referred to
as
the
stress vector.
Using
(8)
we may write the force on
da
as
tda
=
STNdA,
(13)
where the
nominal stress tensor
S
is related to T by
S
=
JA-'T.
Let
b
denote the body force per unit mass. Then, if the body
B
is held in equilibrium by the combination
of
body forces in
f?
and
surface forces on
dB,
we obtain
or,
equivalently,
STNdA
+
ir
prbdV
=
0.
(15)
We require
(14)
and
(15)
to hold
for
every subbody.
deduce from the divergence theorem that
Hence, we
divT
+
pb
=
0,
(
16)
or,
equivalently,
DivS
+
prb
=
0,
assuming that the left-hand sides of
(16)
and
(17)
are continuous,
where div and Div denote the divergence operators in
B
and
0,
respectively. Equations
(16)
and
(17)
are alternative forms of the
equilibrium equations
for
B.
In components
(17)
has the form
and similarly for
(lG),
where
Sa;
are the components of
S.
simply
which may also be expressed
its
STAT
=
AS.
based on the
use
of
S
with
X
as the independent variable.
Balance of the moments of the forces acting on the body yields
TT
=
T,
(19)
Henceforth, we confine attention to the Lagrangean formulation
442
R.
W.
Ogden
2.3
Elasticity
We motivate the definition of elasticity by considering the work done
by the surface a.nd body forces in
a
virtual displacement
6x
from
the current configuration
f3.
By use of the divergence theorem and
equation (17) we obtain the
virtual
work
equation
where the left-hand side of
(20)
represents the virtual work and tr
denotes the trace of
a
second-order tensor. This work is converted
into stored energy
if
there exists
a
scalar function,
IV
say, defined on
the set of deformation gradients, such that
614'
M
tr(SGA). It follows
that
dW
s=-
dA
'
or, in components,
S,i
=
BW/BAi,.
This is the
stress-defomaution
relation
or
constitutive equation
for ail elastic material which
pos-
sesses
a
struin-energy function
IY,
W
being defined per unit volume
Thus, for purposes of this article, an elastic material is character-
ized by the existence of
a
strain-energy function such that (21) holds.
At this point there is
no
restriction on the form of the function
IY,
but
there are
a
number
of
factors limiting the class
of
functions that are
acceptable for the description of the elastic behaviour of materials,
as we see shortly. First, however, we examine how (21) is modified
if
the material is subject to internal constraints.
We illustrate this
by considering the
incompressibility constraint,
so
that
(
10) holds at
each point
X
E
f?,.
Using the fact that
BJ/BA
=
A-'
we see that
(21) is replaced by
in
f?,.
PA-'
detA
=
1,
s=--
dl,Y
dA
where
p
is
a
Lagrange multiplier associated with the constraint:
p
is
often referred to
as
an arbitrary hydrostatic pressure. Thus, (22) is
the stress-deformation rela.tion for an
incompressible elastic
material.

Nonlinear Elasticity
443
The corresponding Cauchy stress tensor is given by
dW
T
=
A-
-
pl
dA
det
A
=
1,
where
I
is the identity tensor.
Now consider
a
rigid deformation superimposed
on
the deforma-
tion
(1);
this is defined by the transformation
x
H
x'
=
Qx
+
c,
where
Q
is
a
rotation tensor and
c
is
a
translation (both indepen-
dent of
x).
The resulting deformation gradient (relative to
a,.)
is
A'
=
QA.
We require the stored elastic energy to be independent of
such superimposed deformations and it therefore follows that
for
all
rotations
Q.
A
strain-energy function satisfying this require-
ment is said to be
objective.
Use
of
the polar decomposition
(4)
and the choice
Q
=
RT
in
(23)
shows that
W(A)
=
W(U).
(24)
Thus,
W
depends on
A
only through the stretch tensor
U
and is
therefore defined on the class of positive definite symmetric tensors.
This motivates the introduction of the symmetric stress tensor
T'
defined by
1
dlY
T
=-
BU
for an unconstrained material and by
pu-'
det
U
=
1
1
dW
T
=--
dU
for
an
incompressible material. This is called the
Biot
stress tensor.
Material Symmetry
Further restrictions on the form of
W
are obtained if the material
possesses symmetries in the reference configuration.
A
material pos-
sesses
a
symmetry
if
its response is unchanged after
a
non-trivial
4.44
R.
W.
Ogden
change of reference configuration. Suppose that the reference config-
uration
0,
is changed to
Bg
with X changing to X'; then, we denote
by
P
the deformakion gradient GradX' of
Bc
relative to
B,
and by
A'
the deformation gradient of
B
relative to
BL
so
that
A
=
A'P.
In
general, the strain-energy function
of
the material relative to
Bi
is
different from that relative to
B,.
If, however, the change of reference
configuration just described does not affect the material response
then we write
W
also for the strain-energy function relative to
Bi,
and hence
W(A'P)
=
W(A)
=
W(A')
(27)
for all deformation gradients
A'.
group as the
symmetry group
of
the material relative to
B,.
The set
of
P
for which
(27)
holds forms
a
group; we refer to this
hot
ropy
To
be specific
we
confine attention to
isotropic elastic materials,
for
which the symmetry group is the
proper orthogonal group.
Then, we
have
for
all
rotations
Q.
The combination of
(23)
and
(28)
then yields
W(AQ)
=
W(A)
(28)
W(QUQ~)
=
~(u)
(29)
for
all rotations
Q;
note that the rotations
in
(23) and
(28)
are inde-
pendent. Equation
(29)
states that
lV
is
an
isotropic function
of
U.
It follows from the spectral decomposition
(5)
that
W
depends on
U
only through the principal stretches
XI,
Xz,
X3.
To
avoid introducing
additional notation we express this dependence
as
W(X1,
X2,
X3);
by
selecting appropriate values for
Q
in
(29)
we deduce that
W
depends
symmetrically on
XI,
X2,
X3.
Thus,
W(X1,
X2J3)
=
W(Xl,X3,
AZ)
=
W(A2,h,X3).
(30)
A
consequence of isotropy
is
that
T'
is
coazial
with
U
and hence,
paralleling
(5),
we have
3
T1
=
t;lU(')
@
u(i),
(31)
i=l
Nonlinear Elasticity
445
where, for an unconstrained material,
dW
ax;
t;
=
-.
For
an
incompressible material the latter is replaced
by
pxf'
x1x2x3
=
1.
tf=--
aw
ax;
It is worth noting in this case that
(33)
the first of which is analogous to
(4)1.
2.4
If the body force in (20) is conservative then we may write
Bo
u
nd
ar
y-valu
e
P
rob
1
ems
b
=
-grad$,
(35)
where
$
is
a
scalar field defined on points
x
E
B.
Given that the
material is elastic with strain-energy function
TV,
it
follows
that
(20)
can be expressed in the form
At this point we consider the equilibrium equation
(17)
together
with the stress-deformation relation (21)
for
an unconstrained mate-
rial, and the deformation gradient
(2)
coupled with
(1):
Div
(g)
+
p,b
=
0,
A
=
Gradx
X
E
Br.
(37)
We supplement
(37)
with boundary conditions, typical of those aris-
ing in problems of nonlinear elasticity, in which
x
is specified on part

446
R.
W.
Ogden
of the boundary,
al?:
c
dl?,
say, and the stress vector on the re-
mainder,
al?:,
so
that
al?:
U
aL3:
=
al?,
and
al?:
n
al?:
=
8.
We
write
x
=
((X)
on
as:,
(38)
STN=u(X)
on
aB;,
(39)
where
(
and
u
are specified functions. More genera.lly,
u
can be
allowed to depend on the deformation but, for simplicity, we restrict
attention here to the dependence expressed in
(39).
The surface
stress defined by
(39)
is referred to as
a
dead-load
traction.
The
basic boundary-value problem of nonlinear elasticity is characterized
by
(37)-(39).
In order
to
analyse this problem additional information
about the nature
of
the function W is needed; this important aspect
of the theory is not addressed to any great extent
in
this a.rticle.
Reference can be made to,
for
example,
[GI
and [14] for discussion of
this matter.
In view
of
the boundary condition
(39)
we now have
Sx
=
0
on
a&,
and
(36)
becomes
Since
u
is independent
of
the deformation, we write
u
=
grad(u
-x),
and
(40)
then becomes
If
we interpret
Sx
as
a
variation of the function
x,
then (41)
provides
a
variational formulation
of
the boundary-value problem
(37)-(39)
and can be written
SE
=
0,
where
E
is the functional
defined by
In
(42)
x
is taken to be in some appropriate class
of
mappings, which
need not be specified here, and similarly for admissible variations 6x

Nonlinear Elasticity
447
subject
to
6x
=
0
on
8s:.
A
similar variational statement can be
formulated if the boundary traction in
(39)
is replaced by
a
hydro-
static pressure,
Tn
=
-Pn
say, in which case
u
depends on the
deformation in the form
U=-JPA-~N
on
~UZ.
(43)
As we shall
see
in Section
3,
the energy functional plays on important
role in the analysis of stability and bifurcation. Detailed discussion
of variational principles in the contest of nonlinear elasticity ca.n be
found in
[14];
for the more technical mathematical aspects the reader
is referred to
[6,11]
and the papers by Ball
[1,2].
For an incompressible material, the functional
E
is modified by
the addition
of
a
constraint term -p{det(GradX)
-
1).
In components, equation
(37)
can be written as
for
i
E
{1,2,3}.
When coupled with suitable boundary conditions,
this forms
a
system of quasi-linear partial differential equations for
3;.
The coefficients
d2W/dAi,dAjp
are, in general, nonlinear func-
tions
of
the components of the deformation gradient.
Only
a
very
limited number
of
boundary-value problems have been solved
for
un-
constrained materials, and only then for very special choices of the
form
of
MI;
some
of
these are discussed in
[14],
for example.
For
incompressible materials the corresponding equations, obtained by
substituting
(22)
into
(17),
have yielded more success, and again we
refer to
[14]
for pointers to the literature.
It is not
our
intention
in this article to discuss such solutions, rather to examine certain
aspects of the structure of the equations.
Equations
(44)
are
strongly
elliptic
if
for
all
non-zero vectors
m
and
N,
where we have introduced the
notation

448
R.
W.
Ogden
3
Incremental Deformations
Suppose that
a
solution
x
to the boundary-value problem
(37)-(39)
is known and consider the problem
of
finding solutions near to
x
when the boundary conditions are perturbed. Let
x'
be
a
solution
for
the perturbed problem and write
x'
=
x'(X).
Also, we write
Then
Grad2
=
Gradx'
-
Gradx
=
A.
In the above and henceforth
a
superposed dot represents the differ-
ence
(-I=
(
1'4
1,
while
6(.)
represents
a
variation
of
(a).
Note that
A
is
linear
in
2.
The nominal stress difference is
aW
BW
aA
aA
S
=
S'
-
S
=
-(A')
-
-(A).
When
A
is small (in some appropriate sense) this can be approxi-
mated as
(49)
1
2
s
=
A'A
+
-A2[A,A]
-t
.
.
.
,
where
In
components, the first
of
the expressioiis
(50)
is given by
(46)
while
the second can be written
and similarly for higher-order terms
if
required (given that
W
is
sufficiently regular). The component
form
of
(49)
is
...
(51)
Sai
=
A,;pjAjp
+
~d~;pj,~Aj~Ak,
+
1'
1
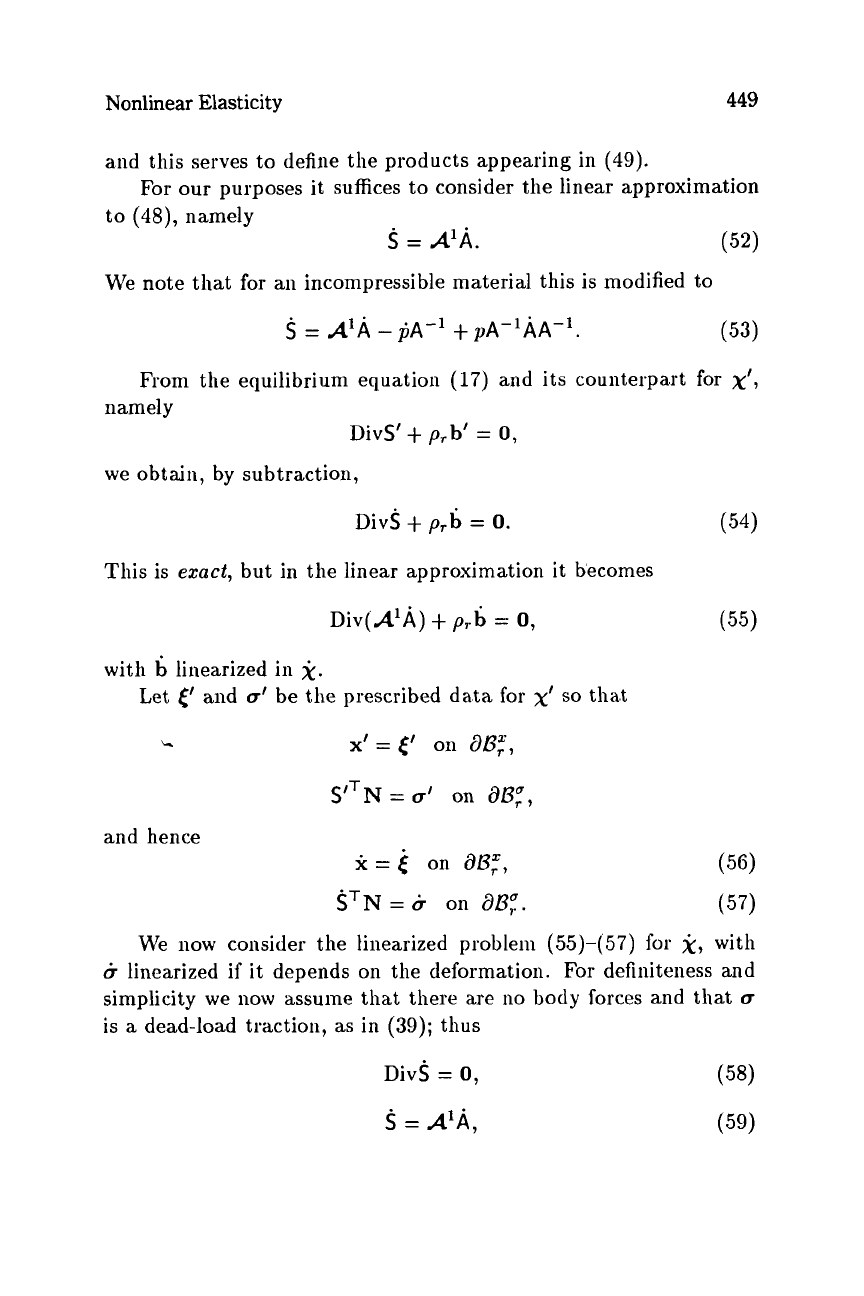
Nonlinear Elasticity
449
and this serves to define the products appearing in (49).
to (48), namely
We note that for an incompressible material this is modified
to
For
our purposes it suffices to consider the linear approximation
S
=
A'A.
(52)
From the equilibrium equation (17) and its couiiterpa.rt for
x',
namely
DivS'
+
p,b'
=
0,
we obtain,
by
subtraction,
DivS
+
prb
=
0.
(54)
This is
exact,
but in the linear approximation it becomes
Div(AIA)
+
p,b
=
0,
(55)
with
b
linearized in
x.
Let
<'
and
u1
be
the
prescribed data for
XI
so
tlmt
4
XI
=
on
at?:,
SI~N
=
0'
on
at?;,
and hence
k=i
on
at?:,
ST~=b
on
at?;.
(57)
We now consider the linearized probleiii (55)-(57)
for
jl,
with
b
linearized if it depends on the deformation.
For
definiteness and
simplicity we now assume that there are no body forces and that
u
is
a
dead-load traction, as in (39); thus
DivS
=
0,
(58)
S
=
A'A,
(59)
