Ames W.F., Harrel E.M., Herod J.V. (editors). Differential Equations with Applications to Mathematical Physics
Подождите немного. Документ загружается.


110
F.
Gesztesy
&
R.
Weikard
and joining the upper and lower rims
of
the cuts
pj
crosswise. This
leads to the compact hyperelliptic curve
I<,
consisting of points
P
=
(z,
&.+I
(z)'/~),
z
€a
and
P,
(3.9)
(P"
the point at infinity obtained by one-point compactification)
with branch points
(Em,O),
OSm52n,
P,.
(3.10)
We also need the projection
I<,
-a
u
n
:
{
P
=
(Z,k2,+1
(z)q
-
2
(3.11)
P"
-w
and the involution (sheet exchange map)
I<,
-
I(,
P
=
(2,
&,+I
(z)'/2)
-
P*
=
(z,
-R2,+1
(z)'/2).
*:{
(3.12)
The upper sheet
n+
of
K,
is then declared as follows. Define
lim
&,+'(A
-t
ie)'I2
=
-I&,+l(A
+
iO)1/217
A
<
EO
L
10
(3.13)
on
II+
and analytically continue with respect to
A.
Local coordinates
f,
near
PO
=
(zo,
k2n+l
(z~)'/~),
P"
then read
(.
-
%),
to
Ea\{Em)2=0
f,
=
(z
-
Em)'/2,
zo
=
Em,
0
5
m
5
2n
(3.14)
i
*-w,
20
=
00.
A
convenient homology basis
{aj,
bj}y=l
on
I<,,
n
E
N
is then chosen
as follows: the cycle
aj
surrounds the cut
pj
clockwise on
n+
while
bj
starts
at
the lower rim
of
pj
on
II+,
intersects
aj,
then encircles
Eo
clockwise thereby changing into the lower sheet
rI-
,
and returns
on
n-
to its initial point. The cycles are chosen in such
a
way that
their intersection matrix reads
aj
o
bl
=
6j,i7
1
5
j,l
5
n.
(3.15)

Spectral Deforrna tions and Soliton Equations
111
A basis for the holomorphic differentials (Abelian differentials
of
the
first kind, DFK) on
li,
is given by
qj
=
R2n+l
(z)-1/2
2j-l
dz,
1
5
j
5
n.
(3.16)
We choose the standard normalization
and define the b-periods
of
w[
by
(3.18)
Riemann’s period relations and
(€1.3.2)
then imply
Tj,l
=
T1j,
T
=
i
T, T
=
(Tj,l)
>
0.
(3.19)
Abelian differentials
of
the second kind
(DSK)
w(~)
are characterized
by vanishing residues and conveniently normalized by
I,
w(2)
=
0,
11
j
5
n.
(3.20)
The Riemann theta-function
tl
and Jacobi variety
J(K,)
associated
with
Ii,
are then defined
as
qZ)
=
C
e24m,z)t4m,7m)
9-
2
can
(3.21)
-
menn
and
J(lin)
=an/L,,
where
L,
denotes the period lattice
(3.22)
Divisors
2)
on
K,
are defined as integer-valued maps
2)
:
Ii,
-
z
(3.24)
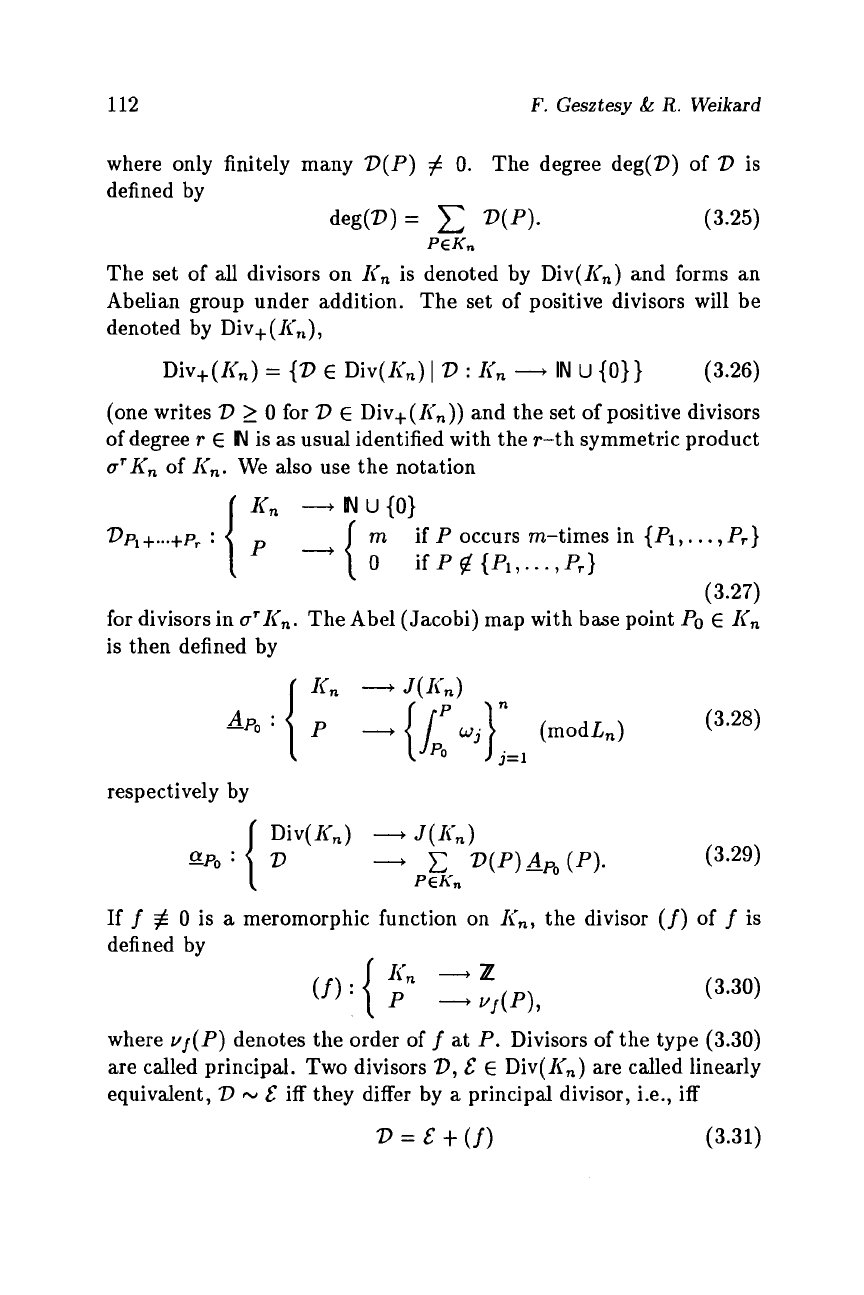
112
F.
Gesztesy
&
R.
Weikard
where only finitely many
D(P)
#
0.
The degree deg(2)) of
2)
is
defined by
deg(2))
=
D(P).
(3.25)
The set of
all
divisors on
lin
is denoted by Div(Kn) and forms an
Abelian group under addition. The set of positive divisors will be
denoted by Div+(K,,),
P€
Kn
Div+(Kn)
=
{D
E
DiV(Kn)
I
2)
:
Kn
+
IN
U
(0))
(3.26)
(one writes
2,
2
0
for
2)
E
Div+(Kn)) and the set of positive divisors
of degree
r
E
N
is as usual identified with the r-th symmetric product
arKn
of
Kn.
We also use the notation
K,
-
N
u
(0)
0
if
P
4
{PI,
...,
Pr}
(3.27)
for divisors in
grKn.
The Abel (Jacobi) map with base point
Po
E
Kn
is then defined by
respectively by
Div(K,)
-
J(Iin)
(3.29)
If
f
f
0
is
a
meromorphic function on
Kn,
the divisor
(f)
of
f
is
defined by
GPO:
2)
-
c
W)APO(P)*
{
P€Kn
(3.30)
where
vj(P)
denotes the order off
at
P.
Divisors of the type
(3.30)
are called principal. Two divisors
D,
&
E
Div(Kn) are called linearly
equivalent,
Z,
N
&
iff they differ by
a
principal divisor, i.e.,
iff
-z
(f)
:
(
Iin
P
-
q(P),
2)
=
E
t
(f)
(3.31)

Spectral Deformations and Soliton Equations
113
for some meromorphic
f
$
0
on
Kn.
The equivalence class of
D
is denoted by
[V]
(if
V
2
0,
ID1
usually denotes the set of positive
divisors linearly equivalent to
’0).
By Abel’s theorem,
deg(V)
=
deg(&)
APo(W
=
Lip,(&)*
V-&iff
(3.32)
The Jacobi inversion theorem states
-
apo
(onKn)
=
J(K,).
(3.33)
Finally,
a
positive divisor
2)
E
onKn
is called nonspecial iff the equiv-
alence class
ID1
of positive divisors of
D
only consists
of
D
itself, i.e.,
iff
PI
=
PI.
(3.34)
Otherwise
2,
2
0
is called special. One can show that
Vp,+ ...+p,
E
onKn
is special
iff
there exists
at
least one pair
(P,P*)
such that
(P,P*)
E
{Pl,...,Pn}.
(3.35)
After these preliminaries we can describe in detail the Its-Matveev
formula
[34]
for real-valued finite-gap potentials
V
satisfying
(3.1).
It reads
2n
n
V(X)=
C
Em-2CXj
m=O
j=1
(3.36)
n
n
Here
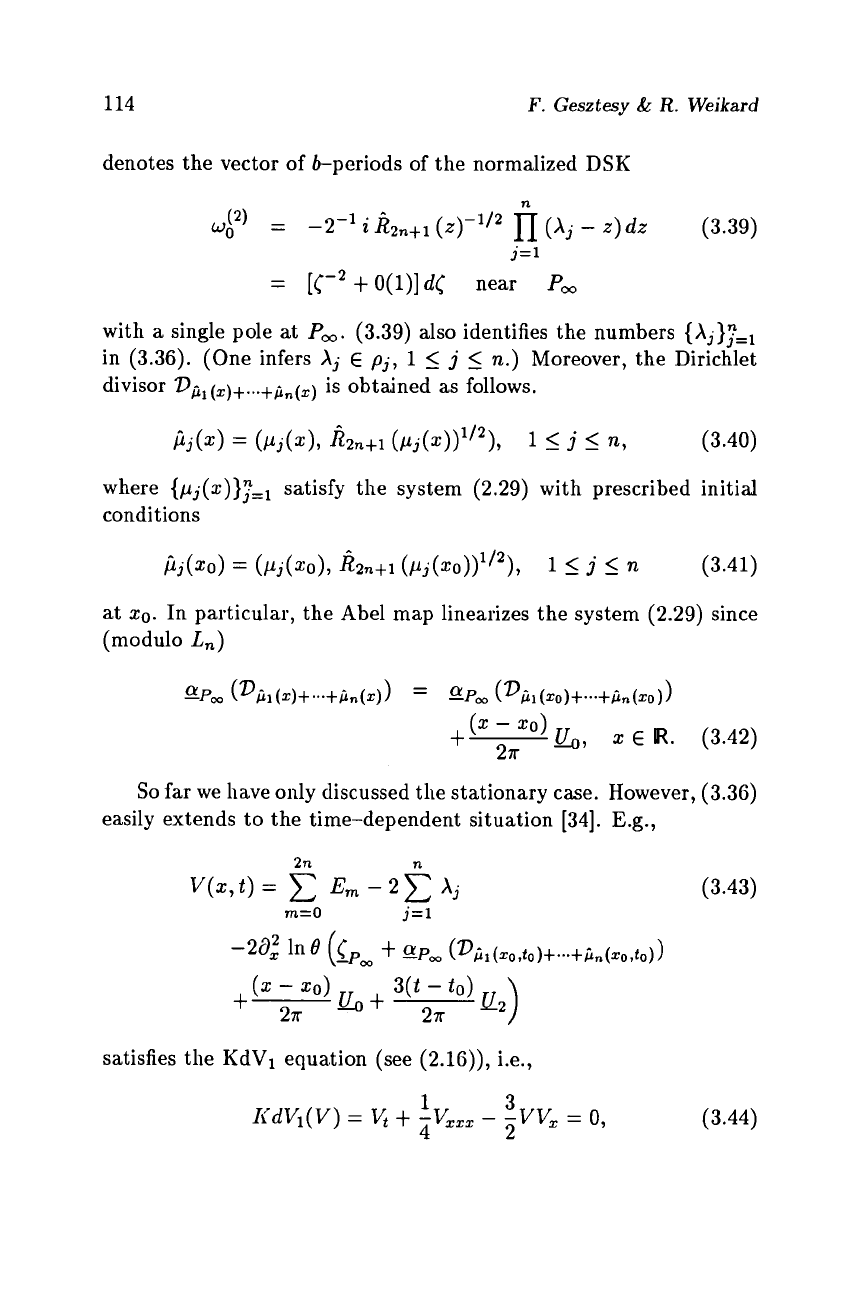
(3.37)
denotes the vector of Riemann constants,
&,
given by
114
F.
Gesztesy
&
R.
Weikard
denotes the vector of bperiods of the normalized DSK
with
a
single pole
at
P,.
(3.39)
also identifies the numbers
{Xj}y=l
in
(3.36).
(One infers
Xj
E
pj,
1
5
j
5
n.)
Moreover, the Dirichlet
divisor
Dfi,
(,I+
...+fin(,)
is
obtained as follows.
bj(x>
=
(pj(X),
fizn+l
(pj(X))”’),
1
I
j
I
12,
(3.40)
where
{pj(~))j”=~
satisfy the system
(2.29)
with prescribed initial
conditions
at
20.
In particular, the Abel map linearizes the system
(2.29)
since
(modulo Ln)
So
far we have oiily discussed the stationary case. However,
(3.36)
easily extends to the time-dependent situation
[34].
E.g.,
2n
n
V(x,t)
=
c
Em
-2c
xj
m=O
j=1
satisfies the KdVl equa.tion (see
(2.1G)),
i.e.,
1
3
4 2
lidVl(V)
=
v,
t
-v,,,
-
-vv,
=
0,
(3.43)
(3.44)

Spectral Deformations and Soh ton Equations
115
where
U2
is the vector of &periods
of
the normalized DSK
wp)
with
a
single pole
at
P,
of the type
up)
=
[c-‘
+
0(1)14
near
P,,
(3.45)
J
1=1
l#j
15
j
I
n
(3.48)
with prescribed initial conditions
Pj(zo,to>
=
(pj(zo,to),
&2n+1
(pj(zo,to))’/2),
1
I
j
I
n
(3.49)
at
(z0,to).
Again
the Abel map linearizes the system
(3.48)
since
(modulo
Ln).
4
Spectral Deformations, Commutation
Techniques
Since virtually all explicitly known solutions of the KdV hierarchy,
such as soliton solutions, rational solutions, and solitons on the back-
ground
of
quasi-periodic finite-gap solutions, can be obtained from

116
F.
Gesztesy
&
R.
Weikard
the Its-Matveev formula upon suitable deformations (singulariza-
tions) of the underlying hyperelliptic curve
K,
(see e.g.
[17]-[20],
[26],
[48], [49], [64]
and the references therein), we propose
a
system-
atic study of such deformations in this section. Our main strategy
will be to exploit single and double commutation techniques to be
explained below.
We illustrate the main idea by the following simple example. Con-
sider again the potential
(2.19)
v(5)
=
2p(x
+
w‘;
92793)
+
p(w’;
92793)
(4.1)
y2
=
(-el
+
e3
-
z)(-e2
+
e3
-
z)(-.t.),
(44
associated with the nonsingular elliptic curve (see
(2.34))
el
=
p(u;
92,93),
e2
=
p(u
+
u‘;
92793)~
e3
=
p(w’;
92,931.
(For
convenience we added
P(w‘)
in
(4.1)
in order to guarantee
E2
=
0.)
Then
H
=
--
+
V
has spectrum (see
(3.3))
d2
dx2
a(EI)
=
[-el
+
e3,
-e2
+
e3]
u
[O,m).
(4.3)
Fix
K
>
0
and deform
(4.4)
2
[-el
+
e3,
-2
+
e3]
-
-n
by taking
w
+
00,
w‘
=
(i7r/26).
Then
V
in
(4.1)
converges to the
one-soliton potential
1’1
v(x)
=
2p(,
+w’;
92793)
+
p(w’;
92793)
-
Vl(5)
=
-262[cosh(K2)]-2
(4.5)
and the associated elliptic curve
(4.2)
degenerates into
a
singular
curve
y2
=
(-el+es-z)(-e2+e3-z)(-~)
-
y2
=
(-K~-z)~(-z).
(4.6)
d2
da:
The corresponding operator
H1
=
-2
+
V,
then has the spectrum
a(&)
=
{-K2}
u
[O,w).
(4.7)

Spectral Deformations and
Soliton
Equations
117
A
further degeneration
K
+
0
finally yields
V(z)
=
0
and
y2
=
(-z)~.
(4.8)
This point of view has been adopted in [48] and [49] and the general
n-soliton potentials have been derived from the Its-Matveev formula
by
a
singularization of
K,
where all compact spectral bands degen-
erate into
a
single point
2
[E2(j4),
E2j-11
-
-Kj,
1
I
j
5
12,
K1
>
K2
>
*-.
>
K,,
E2n
=
0
(4.9)
(see (3.3)).
Here we shall
in
a
sense reverse the above point of view. Instead
of starting with
a
finite-gap potential such as (4.1) and degenerating
compact spectral bands into single points (such
as
in (4.4) with the
result (4.5)-(4.7)), we shall start with
a
finite-gap potential
VO
and
insert eigenvalues into its spectral gaps. In the context of the above
example this amounts to starting with
V&)
=
0,
y2
=
--z
(4.10)
and inserting the eigenvalue
-tc2
into the spectral gap
po
=
(-oo,O)
of
Vo
to arrive at
VI(X)
=
-2K~[COSh(Kx)]-2,
y2
=
(-62
-
-z)+).
(4.11)
The spectral deformations described
so
far were clearly non-isospec-
tral. In addition we will also discuss various isospectral deformations
of potentials below.
In
short, these isospectral deformations either
“insert eigenvalues” at points where there were already eigenvalues
or
they formally insert eigenvalues with certain “defects” such as
zero
or
infinite norming constants. In either case no new eigenvalue
is actually inserted and the deformation is isospectral.
A
systematic
and detailed approach to these ideas can be found in (251-[27].
We start with the single commutation method
or
Crum-Darboux
method [11]-[14],
[18],
[19], [3G],
[Gl].
Assume that
VO
E
Lioc
(IR)
is
real-valued and that the differential expression
(4.12)

118
F.
Gesztesy
&
R.
Weikard
is nonoscillatory and in the limit point case
at
foo.
Consider the
self-adjoint realization
Ho
of
TO
in L2(IR)
d2
dx2
(4.13)
Ho
=
--+v
0,
’WHO)
=
(9
E
L2(IR)
19,
9’
E
ACloc(lR),
Tog
E
L2(IR)}
(here ACloc(-) denotes the set of locally absolutely continuous func-
tions) with
Eo
=
inf[a(Ho)]
>
-m.
(4.14)
The basic idea behind the single commutation method is the follow-
ing: choose
A1
E
Po
=
(-00,
Eo)
(4.15)
and factor
(4.16)
d2
dx2
110
=
AA*
+
A1
=
--
+
Vo
with
for some real-valued distributional solution
$o(A1,
z).
Commuting
A and A* yields
d2
dx2
Vl(x)
=
Vo(x)
-
2-
In
$‘~(Al,x).
(4.18)
(4.19)
d2
dx2
We note that
TI
=
--
+
V~(X)
is in the limit point case at
Aoo
and that
a(H1)\{A1}
=
a(H0).
(4.20)
Depending on the choice of
$‘o(A1,x), A1
either belongs to
a(H1)
and one has inserted an eigenvalue
A1
into
po
=
(-oo,Eo)
which
represents the non-isospectral case,
or
A1
@
HI),
i.e.,
a(H1)
=

Spec
t
rd
Deformations an
d
Soliton Equations
119
a(H0)
which is the isospectral case. The above procedure can easily
be iterated and we only summarize the final results.
Consider weak solutions
$o,*
(XI,
x)
such that
(4.21)
0
<
$o,k
(A,
.)
E
L2((R,f~)),
R
E
IR,
A
<
Eo,
Ho
$o,k(x)
=
wo,*
(A),
<
Eo.
Pick
and define in
L2(
IR)
<
A2
< <
AN
<
Eo
(4.22)
d2
dx2
H(Ai,ci,.
.
.
,XN,EN)
=
--
+
V(xi,ci,.
. .
,
XN,CN),
(4.23)
V(Xl,El,
- - -
7
AN,
EN,
x)
=
vO(x)
d2
-2~1nW($O,cl(~1),-.
*
,'$'0,c~(AN))(x),
€1
E
{+,-},
15
15
N.
(4.24)
d2
dx2
Then
TN
=
--
+
V(X1,
€1,.
. .
,
AN,
EN,
x)
is in the limit point case
at
f-00
and
H(A1,€1,.
. .
,
AN,
EN)
and
HO
are isospectral, i.e.,
B(H(xl,El,...,XN,EN))
=
o(H0)
(4.25)
(in fact, one can show that they are unitarily equivalent
[13]).
If on
the other hand one replaces
$o,cl(X~,z)
in
(4.24)
by
a
genuine linear
combination
of
$o,+(&,
x)
and
$o,-(A[,z)
$O,€&X)
-
@O,+(h,X)
+
P$O,-(Xl,Z),
>
0,
P
>
0
(4.26)
then
XI
E
po
=
(--00,
Eo)
becomes actually an eigenvalue of the
resulting operator. Since we are going to use the single commutation
method only in the isospectral context in Section
5
we shall not give
any further details on the non-isospectral case.
In the special case of
VO
in
(4.13)
being
a
finite-gap potential
of
the type
(3.36),
2n
n
m=O
j=1
(4.27)
