Ambrosio L., Caffarelli L., Crandall M.G., Evans L.C., Fusco N. Calculus of Variations and Nonlinear Partial Differential Equations
Подождите немного. Документ загружается.


170 N. Fusco
Let us assume that (H
1
) and (H
2
) hold in some open set U ⊂ IR
n−1
.Then,
for every connected open subset U
α
of U, E ∩(U
α
×IR ) is equivalent to E
s
∩
(U
α
× IR ) , up to a translation in the y direction.
In particular, if (H
1
) and (H
2
) hold in a connected open set U such that
L
n−1
(π(E)
+
\ U)=0, then E is equivalent to E
s
, up to a translation in the
y direction.
As far as I know, this result was known in the literature only for convex sets,
where it can be proved with a simple argument. In fact, let us assume that E
is an open convex set such that P (E)=P (E
s
) < ∞. Then, π(E)isalsoan
open convex set and there exist two functions y
1
,y
2
: π(E) → IR , y
1
convex,
and y
2
concave, such that
E = {(x
,y): x
∈ π(E),y
1
(x
) <y<y
2
(x
)}.
Let us now fix an open set U ⊂⊂ π(E). From assumption (2.10) and from (2.1)
we have that P (E
s
; U × IR ) = P (E; U × IR). Since y
1
and y
2
are Lipschitz
continuous in U , we can write this equality as
2
U
,
1+
|∇(y
2
− y
1
)|
2
4
dx
=
U
1+|∇y
1
|
2
dx
+
U
1+|∇y
1
|
2
dx
.
From this equality, the strict convexity of the function t →
√
1+t
2
and the
arbitrariness of U,wegetthat∇y
2
= −∇y
1
in π(E) and thus y
2
= −y
1
+
const.. This shows that E coincides with E
s
, up to a translation in y direction.
The proof of Theorem 2.3, for which we refer to [9], uses delicate tools from
Geometric Measure theory. However, in the special case considered below it
can be greatly simplified.
Proof of Theorem 2.3 in a Special Case. Let us assume that E is an
open set, that π(E) is connected and that E is bounded in y direction.
Notice that since E is open, then µ is a lower semicontinuous function and
for any open set U ⊂⊂ π(E) there exists a constant c(U) > 0 such that
µ(x
) ≥ c(U ) for all x
∈ U. (2.11)
Moreover, since E is bounded in the y direction, the function
x
∈ π(E) → m(x
)=
E
x
ydy
is bounded in π(E). Then, the same arguments used in the proofs of Lemmas
1.1 and 1.3 yield that m ∈ BV
loc
(π(E)) and that for L
n−1
-a.e. x
∈ π(E),
i =1,...,n−1,
∂m
∂x
i
(x
)=
y∈∂
∗
E
x
yν
E
i
(x
,y)
|ν
E
n
(x
,y)|
, (2.12)
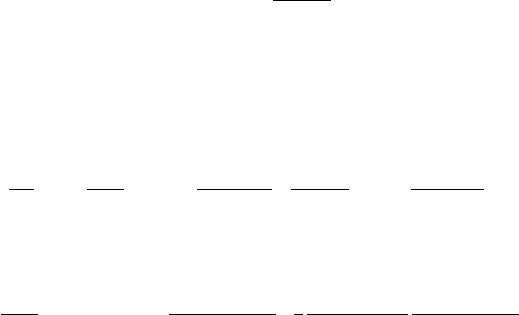
Geometrical Aspects of Symmetrization 171
wherewehavedenotedby∂m/∂x
i
the absolutely continuous part of the
derivative D
i
m.
By Proposition 2.1 we have that (H
1
) implies that the distribution
function µ is a Sobolev function. The same assumption implies also that
m ∈ W
1,1
loc
(π(E)). In fact, the argument used to prove (1.14) shows that if
B ⊂ π(E) is a Borel set, then |Dm|(B) ≤ MP(E; B × IR), where M is a
constant such that E ⊂ IR
n−1
× (−M, M). Therefore, if L
n−1
(B) = 0, from
(2.1) and Proposition 2.1 we have that P (E; B × IR ) = P (E
s
; B × IR ) = 0 ,
hence Dm is absolutely continuous with respect to L
n−1
.
Let us now denote, for any x
∈ π(E)byb(x
) the baricenter of the section
E
x
, i.e.,
b(x
)=
E
x
ydy
µ(x
)
.
From (2.11) and Proposition 2.1 we have that b too belongs to the space
W
1,1
loc
(π(E)). Thus, to prove the assertion, since π(E) is a connected open set,
it is enough to show that ∇b ≡ 0, hence b is constant on π(E). To this aim,
let us evaluate the partial derivatives of b, using the representation formulas
(2.12) and (1.21). We have, for any i =1,...,n−1 and for L
n−1
-a.e. x
∈ π(E),
∂b
∂x
i
(x
)=
1
µ(x
)
y∈∂
∗
E
x
yν
E
i
(x
,y)
|ν
E
n
(x
,y)|
−
E
x
ydy
µ(x
)
y∈∂
∗
E
x
ν
E
i
(x
,y)
|ν
E
n
(x
,y)|
. (2.13)
Since for L
n−1
-a.e. x
∈ π(E) (2.4) and (2.5) hold, the right hand side of
(2.13) is equal to
1
µ(x
)
y
2
(x
)+y
1
(x
)
ν
E
i
(x
,y
2
(x
))
|ν
E
n
(x
,y
2
(x
))|
−
1
2
y
2
2
(x
)−y
2
1
(x
)
y
2
(x
)−y
1
(x
)
2ν
E
i
(x
,y
2
(x
))
|ν
E
n
(x
,y
2
(x
))|
=0.
Hence, the assertion follows. !"
3TheP`olya–Szeg¨o Inequality
We are going to present the classical P`olya–Szeg¨o inequality for the spherical
rearrangement of a Sobolev function u and discuss what can be said about
the function u when the equality holds. In order to simplify the exposition,
we shall assume that u is a nonnegative measurable function from IR
n
, with
compact support. However, most of the results presented here, like Theorem
3.1, still hold with no restrictions on the support or on the sign of u.
Given u, we set, for any t ≥ 0,
µ
u
(t)=L
n
({x ∈ IR
n
: u(x) >t}) .
172 N. Fusco
The function µ
u
is called the distribution function of u. Clearly µ
u
is a de-
creasing, right-continuous function such that
µ
u
(0)= L
n
(suppu),µ
u
(esssup u)=0,µ
u
(t−)=L
n
({u ≥ t}) for all t>0 .
(3.1)
Notice that from the last equality we have that when t>0
µ
u
is continuous in t iff L
n
({u = t})=0.
Let us now introduce the decreasing rearrangement of u, that is the function
u
∗
:[0, +∞) → [0, +∞) defined, for any s ≥ 0, by setting
u
∗
(s)=sup{t ≥ 0: µ
u
(t) >s}.
Clearly, u
∗
is a decreasing, right-continuous function. The following elemen-
tary properties of u
∗
are easily checked:
(j) u
∗
(µ
u
(t)) ≤ t ≤ u
∗
(µ
u
(t)−) for all 0 ≤ t<esssup u ;
(jj) µ
u
(u
∗
(s)) ≤ s ≤ µ
u
(u
∗
(s)−) for all 0 ≤ s<L
n
(suppu);
(jjj) L
1
({s : u
∗
(s) >t} = µ
u
(t) for all t ≥ 0 .
Notice that (jjj) states that the functions u and u
∗
are equi-distributed, i.e.,
µ
u
= µ
u
∗
. Let us now define the spherical symmetric rearrangement of u,that
is the function u
:IR
n
→ [0, +∞), such that for all x ∈ IR
n
u
(x)=u
∗
(ω
n
|x|
n
) . (3.2)
By definition and by (jjj) we have
L
n
({u
>t})=L
n
({u>t}) for all t ≥ 0 ,
i.e., µ
u
= µ
u
. Thus also u and u
are equi-distributed. As a simple conse-
quence of this equality and Fubini’s theorem we have, for all p ≥ 1,
IR
n
|u
(x)|
p
dx =
IR
n
|u(x)|
p
dx ,
and, letting p → +∞, esssup u = esssup u
.
If u is a smooth function, in general its symmetric rearrangement will be
no longer smooth (actually the best we may expect from Theorem 3.1 below
is that u
is Lipschitz continuous). However, the symmetric rearrangement
behaves nicely on Sobolev functions, as shown by the next result.
Theorem 3.1 (P`olya–Szeg¨o Inequality). Let u ∈ W
1,p
(IR
n
), p ≥ 1,bea
nonnegative function with compact support. Then u
∈ W
1,p
(IR
n
) and
IR
n
|∇u
(x)|
p
dx ≤
IR
n
|∇u(x)|
p
dx . (3.3)

Geometrical Aspects of Symmetrization 173
The proof of this result relies upon two main ingredients, the isoperimetric
inequality and the coarea formula for BV functions. Let us start by recalling
the latter.
Let u be a BV (Ω) function. Then, for L
1
-a.e. t ∈ IR, the set {u>t} has
finite perimeter in Ω. Moreover, for any Borel function g : Ω → [0, +∞], the
following formula holds (see [1, Theorem 3.40]).
Ω
g(x) d|Du| =
∞
−∞
dt
∂
∗
{u>t}
g(x) dH
n−1
. (3.4)
In the special case u ∈ W
1,1
(Ω), it can be shown that for L
1
-a.e. t the reduced
boundary ∂
∗
{u>t} coincides, modulo a set of H
n−1
-measure zero, with the
level set {+u = t},where+u denotes the precise representative of u,whichis
defined H
n−1
-a.e. in Ω as in (2.9). Therefore, if u ∈ W
1,1
(Ω), (3.4) becomes
Ω
g(x)|∇u(x)|dx =
∞
−∞
dt
{˜u=t}
g(x) dH
n−1
(x) . (3.5)
The isoperimetric inequality states that if E be a set of finite perimeter, then
min {L
n
(E), L
n
(IR
n
\ E)}
n−1
n
≤
1
nω
1/n
n
P (E) . (3.6)
Moreover, the equality holds if and only if E is (equivalent to) a ball.
Next lemma shows that if u is a Sobolev function, then the same is also
true for u
.
Lemma 3.1. Let u be a nonnegative function with compact support from the
space W
1,1
(IR
n
). Then u
belongs to W
1,1
(IR
n
) and
IR
n
|∇u
|dx ≤
IR
n
|∇u|dx . (3.7)
Proof. Letusfirstprovethatforany0<a<b, the function u
∗
is absolutely
continuous in (a, b)and
b
a
|u
∗
(s)|ds ≤
1
nω
1/n
n
a
n−1
n
{u
∗
(b)<u<u
∗
(a)}
|∇u|dx . (3.8)
To this aim, we start by observing that from the third equality in (3.1) and
from inequality (jj) we have
L
n
({x ∈ IR
n
: u
∗
(b) <u(x) <u
∗
(a)})=µ
u
(u
∗
(b)) − µ
u
(u
∗
(a)−) ≤ b − a.
(3.9)
Let us denote by ω :[0, ∞) → [0, +∞) the modulus of continuity of the
integral of |∇u|, i.e., a continuous function, vanishing at zero and such that
for any set of finite measure E
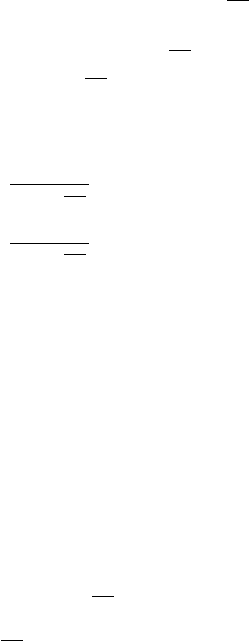
174 N. Fusco
E
|∇u|dx ≤ ω(L
n
(E)) .
Using the coarea formula (3.5), the isoperimetric inequality (3.6) and (jj)
again, we obtain the following estimate for the integral of |∇u| between two
level sets,
{u
∗
(b)<u<u
∗
(a)}
|∇u|dx =
u
∗
(a)
u
∗
(b)
P ({u>t}) dt
≥ nω
1/n
n
u
∗
(a)
u
∗
(b)
L
n
({u>t}
n−1
n
dt
≥ nω
1/n
n
[µ
u
(u
∗
(a)−)]
n−1
n
(u
∗
(a) − u
∗
(b)) (3.10)
≥ nω
1/n
n
a
n−1
n
(u
∗
(a) − u
∗
(b)) .
Let us now take a finite number of pairwise disjoint intervals (a
i
,b
i
) ⊂ (a, b),
i =1,...,N. By applying (3.9) and (3.10) to each interval (a
i
,b
i
), we get
N
i=1
|u
∗
(b
i
) − u
∗
(a
i
)|≤
1
nω
1/n
n
a
n−1
n
N
i=1
{u
∗
(b
i
)<u<u
∗
(a
i
)}
|∇u|dx
≤
1
nω
1/n
n
a
n−1
n
ω
N
i=1
(b
i
− a
i
)
. (3.11)
From this inequality it follows immediately that u is absolutely continuous in
(a, b), since the left hand side is smaller than a given ε>0assoonasthesum
of the lengths of the intervals (a
i
,b
i
) is sufficiently small. Moreover, by taking
the supremum of the left hand side of (3.11) over all possible partitions of the
interval (a, b), from the first inequality in (3.11) we get immediately (3.8).
Notice that from (3.8) it follows that u
is in W
1,1
loc
(IR
n
\{0}). To prove the
assertion, we fix σ>1 and estimate the integral of |∇u| in the annuli A
k,σ
=
{x ∈ IR
n
: ω
−1/n
n
σ
k/n
< |x| <ω
−1/n
n
σ
(k+1)/n
},fork ∈ ZZ. Using (3.8) again,
and recalling the definition (3.2), we get, for any k ∈ ZZ ,
A
k,σ
|∇u
|dx = nω
n
A
k,σ
|x|
n−1
|u
∗
(ω
n
|x|
n
)|dx
= n
2
ω
2
n
ω
−1/n
n
σ
(k+1)/n
ω
−1/n
n
σ
k/n
r
2n−2
|u
∗
(ω
n
r
n
)|dr
= nω
1/n
n
σ
k+1
σ
k
s
n−1
n
|u
∗
(s)|ds
≤ σ
n−1
n
{u
∗
(σ
k
)<u<u
∗
(σ
k+1
)}
|∇u|dx .
Then the assertion immediately follows by summing up both sides of this
inequality over all k ∈ ZZ and then letting σ → 1
+
. !"

Geometrical Aspects of Symmetrization 175
Notice that the lemma we have just proved provides the P´olya–Szeg¨oin-
equality for p = 1. However, for the general case p ≥ 1 we present a different
proof which has the advantage of giving better information when the inequal-
ity becomes an equality.
To this aim let us introduce a few quantities that will be useful later. If
u ∈ W
1,1
loc
(IR
n
), we set
D
+
u
= {x ∈ IR
n
: ∇u(x) =0}, D
0
u
=IR
n
\D
+
u
.
We can now give a representation formula for the derivative of µ
u
. Notice that
the formula stated in (3.12) uses the fact that the |∇u
| is H
n−1
-a.e. constant
on L
1
-a.e. level set {u
= t}.
Lemma 3.2. Let u ∈ W
1,1
(IR
n
) be a nonnegative function with compact sup-
port. Then, for L
1
-a.e. t>0,
µ
u
(t)=−
H
n−1
({u
= t})
|∇u
|
|{u
=t}
≤−
{˜u=t}
1
|∇u|
dH
n−1
. (3.12)
Proof. First of all let us evaluate µ
u
(t) using the coarea formula (3.5). We
get, for all t ≥ 0,
µ
u
(t)=L
n
{u>t}∩D
0
u
+ L
n
{u>t}∩D
+
u
= L
n
{u>t}∩D
0
u
+
D
+
u
χ
{u>t}
(x) dx (3.13)
= L
n
{u>t}∩D
0
u
+
+∞
t
ds
{˜u=s}
χ
D
+
u
|∇u|
dH
n−1
= L
n
{u>t}∩D
0
u
+
+∞
t
ds
{˜u=s}
1
|∇u|
dH
n−1
,
where the last equality follows by observing that coarea formula (3.5) implies
that H
n−1
({˜u = t}∩D
0
u
)=0forL
1
-a.e. t ≥ 0. By applying (3.13) to u
,we
get also that for all t ≥ 0
µ
u
(t)=L
n
{u
>t}∩D
0
u
+
+∞
t
H
n−1
({u
= t})
|∇u
|
|{u
=t}
(3.14)
Let us now recall a nice property of absolutely continuous functions (see for
instance [10, Lemma 2.4]).
If g is an absolutely continuous function in a bounded open interval I and,
for all t ∈ IR, we set φ
g
(t)=L
1
({g>t}∩D
0
g
), then φ
g
is a nondecreasing
function such that φ
g
(t)=0forL
1
-a.e. t.
By applying this result with g = u
∗
and observing that L
n
{u
>t}∩D
0
u
=
L
1
{u
∗
>t}∩D
0
u
∗
, for all t>0, from (3.14) we get immediately the equality
in (3.12). On the other hand, the inequality

176 N. Fusco
µ
u
(t) ≤−
{˜u=t}
1
|∇u|
dH
n−1
follows immediately from (3.13). !"
We are now ready to prove the P´olya–Szeg¨o inequality (3.3).
Proof of Theorem 3.1. Let us fix a nonnegative function u ∈ W
1,p
(IR
n
)
with compact support and let us assume, without loss of generality, that u
coincides with its precise representative +u. From Lemma 3.1 we know already
that u
belongs to the space W
1,1
(IR
n
). Thus, using the coarea formula (3.5)
with u replaced by u
and recalling that |∇u
| is constant on the level sets of
u
,weget
IR
n
|∇u
|
p
dx =
+∞
0
dt
{u
=t}
|∇u
|
p−1
dH
n−1
=
+∞
0
H
n−1
({u
= t})|∇u
|
p−1
|{u
=t}
dt .
From this equation, using twice (3.12), the isoperimetric inequality (3.6),
H¨older’s inequality and coarea formula again, we get
IR
n
|∇u
|
p
dx =
+∞
0
-
H
n−1
({u
= t})
.
p
[−µ
u
(t)]
p−1
dt
≤
+∞
0
-
H
n−1
({u
= t})
.
p
{u
=t}
dH
n−1
|∇u|
p−1
dt (3.15)
≤
+∞
0
-
H
n−1
({u = t})
.
p
{u=t}
dH
n−1
|∇u|
p−1
dt
≤
+∞
0
dt
{u=t}
|∇u|
p−1
dH
n−1
=
IR
n
|∇u|
p
dx .
Hence (3.3) follows. !"
Let us now discuss the equality case in (3.3). First, notice that if this is
the case, then all inequalities in (3.15) are in fact equalities. In particular, if
the second inequality in (3.15) holds as an equality, then we can conclude that
the set {u>t} is (equivalent to) a ball for L
1
-a.e. t ≥ 0. Moreover, if the
equality holds in the third inequality (where we have used H¨older inequality),
the conclusion is that for L
1
-a.e. t ≥ 0, |∇u| is H
n−1
-a.e. constant on the level
set {u = t}.
These are the immediate consequences of the equality case. However, with
some extra work, one can prove the following, more precise, result (see [5] or
[11, Theorem 2.3]).

Geometrical Aspects of Symmetrization 177
Proposition 3.1. Let u ∈ W
1,p
(IR
n
), p ≥ 1, a nonnegative function with
compact support such that
IR
n
|∇u
|
p
dx =
IR
n
|∇u|
p
dx . (3.16)
Then there exist a function v, equivalent to u, i.e. such that v(x)=u(x) for
L
n
-a.e. x ∈ IR
n
,andafamilyofopenballs{U
t
}
t≥0
such that:
(i) {v>t} = U
t
for t ∈ [0, esssup u);
(ii) {v = esssup u} =
/
0≤t<esssup u
U
t
, and is a closed ball (possibly a point);
(iii) v is lower semicontinuous in {v<esssup u};
(iv) if v(x) ∈ (0, esssup u) and L
n
({u = v(x)})=0, then x ∈ ∂U
v(x)
;
(v) for every t ∈ (0, esssup u) there exists at most one point x ∈ ∂U
t
such
that v(x) = t;
(vi) the coarea formula (3.5) holds with +u replaced by v;
(vii) for L
1
-a.e. t ∈ (0, esssup u), |∇v(x)| = |∇u
|
|{u
=t}
for H
n−1
-a.e.
x ∈ ∂U
t
.
This proposition contains all the information that we can extract from equality
(3.16). However it is not true in general that (3.16) implies that u coincides
with u
, up to a translation in x. This can be easily seen by considering any
spherically symmetric nonnegative function w, such that L
n
({w = t
0
}) > 0
for some t
0
∈ (0, esssup w) and another function u whose graph agrees with
the graph of w where u<t
0
and with a slight translated of the graph of
w where u>t
0
.Thenu
= w and (3.16) holds, but u is not spherically
symmetric. What goes wrong in this example is the fact that the gradient of
w (and of u) vanishes in a set of positive measure. Thus, this example suggests
to introduce the following assumption,
(H) L
n
({0 <u
< esssup u}∩D
0
u
)=0.
Notice that we are in a situation similar to the one we were in the previous
lecture when dealing with the assumption (H
1
). In fact, it can be proved (see
for instance [10, Lemma 3.3]) that (H) is implied by the stronger assumption
(H
) L
n
({0 <u<esssup u}∩D
0
u
)=0.
Moreover, (H) is equivalent to the absolute continuity in (0, +∞) of the dis-
tribution function µ
u
and the two conditions (H)and(H
) are equivalent if
(3.16) holds (see [10, Lemma 3.3] again).
The following result was proved for the first time in the Sobolev setting
by Brothers and Ziemer ([5]). It shows that when equality holds in (3.3),
assumption (H) guarantees that u and u
agree.
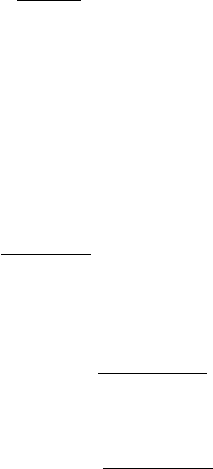
178 N. Fusco
Theorem 3.2. Let u ∈ W
1,p
(IR
n
), p>1, be a nonnegative function with
compact support such that (3.16) and (H) hold. Then u
= u,uptoatrans-
lation in x.
Notice that the above result is in general false if p = 1, even in one dimension.
To see this it is enough to take a function which is increasing in the interval
(−∞,a) and decreasing in (a, +∞).
The starting point of the proof of Brothers and Ziemer is to observe, as we have
done before, that the equality (3.1) yields that L
n
-a.e. set {u>t} is a ball and
that |∇u| is constant on the corresponding boundary. Then the difficult part
of the proof consists in exploiting assumption (H) to deduce that all these
balls are concentric, i.e. u is spherically symmetric. To prove this, we shall not
follow the original argument contained in ([5]), but a somewhat simpler one
used in [11], which is in turn inspired to an alternative proof of Theorem 3.2
given in [17].
To this aim from now on we shall assume, without loss of generality, that u
agrees with the representative v provided by Proposition 3.1 and that U
0
=
{u>0} is a ball centered at the origin. Then, for all 0 <t<esssup u we
denote by R
t
the radius of the ball U
t
and set, for all x ∈ U
0
,
Φ(x)=
µ
u
(u(x))
ω
n
1/n
. (3.17)
To understand the role of the function Φ, observe that if x ∈ U
0
is a point
such that u(x)=t,thenµ
u
(u(x)) = L
n
(U
t
). Therefore, Φ(x) is equal to the
radius of the ball U
t
.
The following lemma is a crucial step toward the proof of Theorem 3.2.
Lemma 3.3. Under the assumptions of Theorem 3.2, Φ ∈ W
1,∞
(U
0
) and
|∇Φ(x)| =1 forL
n
-a.e. x ∈ U
0
\{u = esssup u}. (3.18)
Proof. We claim that µ
u
◦ u ∈ W
1,∞
(U
0
)andthat
∇(µ
u
◦u)(x)=−H
n−1
({u= u(x)})
∇u(x)
|∇u|
|{u=u(x)}
χ
D
+
u
(x)forL
n
-a.e. x ∈ U
0
.
(3.19)
From assumption (H), which is equivalent by (3.16) to (H
), using (3.13) and
Proposition 3.1 (vii), we get that for all 0 ≤ t ≤ esssup u,
µ
u
(t)=L
n
({u = esssup u})+
+∞
t
H
n−1
({u = s})
|∇u|
|{u=s}
ds .
For ε>0, we set
µ
u,ε
(t)=L
n
({u = esssup u})+
+∞
t
H
n−1
({u = s})
|∇u|
|{u=s}
+ ε
ds .

Geometrical Aspects of Symmetrization 179
Clearly, µ
u,ε
(t) ↑ µ
u
(t) for every t ≥ 0asε ↓ 0. Moreover, µ
u,ε
is Lipschitz
continuous in [0, +∞), and
µ
u,ε
(t)=−
H
n−1
({u= t})
|∇u|
|{u=t}
+ ε
for L
1
-a.e. t ≥ 0 ,
whence
|µ
u,ε
(t) − µ
u
(t)|≤
εH
n−1
({u= t})
|∇u|
|{u=t}
|∇u|
|{u=t}
+ ε
for L
1
-a.e. t ≥ 0 .
Thus, µ
u,ε
(t) → µ
u
(t) L
1
-a.e. in [0, +∞)asε → 0, since |∇u|
|{u=t}
= 0 for
L
1
-a.e. t ≥ 0, in as much as
H
n−1
({u= t})
|∇u|
|{u=t}
∈ L
1
(0, ∞). This membership and
the fact that
|µ
u,ε
(t) − µ
u
(t)|≤
H
n−1
({u= t})
|∇u|
|{u=t}
for L
1
-a.e. t ≥ 0
entail that µ
u,ε
→ µ
u
in L
1
(0, ∞). Hence, µ
u,ε
→ µ
u
uniformly in (0, ∞).
Consequently, the functions µ
u,ε
◦u converge uniformly to µ
u
◦u. Furthermore,
by the chain rule for Sobolev functions (see e.g. [1, Theorem 3.96]),
∇(µ
u,ε
◦ u)(x)=µ
u,ε
(u(x))∇u(x)=−H
n−1
({u = u(x)})
∇u(x)
|∇u|
|{u=u(x)}
+ ε
for L
n
-a.e. x ∈ U
0
.
The last expression clearly converges to the right-hand side of (3.19). More-
over, from Theorem 3.1, we have that H
n−1
({u = t})=H
n−1
(∂U
t
) ≤
nω
n
R
n−1
0
for L
1
-a.e. t ∈ (0, ess sup u). Thus,
|∇(µ
u,ε
◦ u)(x)|≤nω
n
R
n−1
0
for L
n
-a.e. x ∈ U
0
.
By dominated convergence, ∇(µ
u,ε
◦ u) converges to the right-hand side of
(3.19) in L
1
(U
0
). Hence, the claim follows.
To conclude the proof let us now observe that for all t ∈ (0, esssup u),
µ
u
(u(x)) ≥ µ
u
(t) > 0 for all x ∈ U
0
\ U
t
. Therefore, we can compute the
derivatives of Φ in U
0
\ U
t
by the usual chain rule formula for Sobolev func-
tions, thus getting, from (3.19), that for L
n
-a.e. x ∈ U
0
\ U
t
∇Φ(x)=−
1
nω
1/n
n
(µ
u
(u(x)))
1−n
n
H
n−1
({u= u(x)})
∇u(x)
|∇u|
|{u=u(x)}
χ
D
+
u
(x)
= −
∇u(x)
|∇u|
|{u=u(x)}
χ
D
+
u
(x). (3.20)
Then, (3.18) follows immediately from Proposition 3.1 (ii). In particular, this
proves that Φ is a W
1,∞
function in the open set U
0
\{u = esssup u} (which
