Alexandrov A.S.,Theory of Superconductivity - From Weak to Strong Coupling
Подождите немного. Документ загружается.

136
Strong-coupling theory
and
b
†
m
b
m
=
1
2
− S
z
m
(4.170)
with the pseudospin
1
2
operators S
x,y,z
=
1
2
τ
1,2,3
. S
z
m
=
1
2
corresponds
to an empty site m and S
z
m
=−
1
2
to a site occupied by the bipolaron.
Spin operators preserve the bosonic nature of bipolarons, when they are on
different sites and their fermionic internal structure. Replacing bipolarons by
spin operators, we transform the bipolaronic Hamiltonian into the anisotropic
Heisenberg Hamiltonian:
H
b
=
m=m
[
1
2
¯v
mm
S
z
m
S
z
m
+ t
mm
(S
x
m
S
x
m
+ S
y
m
S
y
m
)]. (4.171)
This Hamiltonian has been investigated in detail as a relevant form for magnetism
and also for quantum solids like a lattice model of
4
He. However, while in those
cases the magnetic field is an independent thermodynamic variable, in our case
the total ‘magnetization’ is fixed,
1
N
m
S
z
m
=
1
2
− n
b
(4.172)
if the bipolaron density n
b
is conserved. The spin-
1
2
Heisenberg Hamiltonian
(4.171) cannot be solved analytically. The complicated commutation rules for
bipolaron operators (equations (4.167) and (4.168)) make the problem hard but
not in the limit of low atomic density of bipolarons, n
b
1 (for a complete phase
diagram of bipolarons on a lattice see [10, 109]). In this limit we can reduce the
problem to a charged Bose gas on a lattice [110]. Let us transform the bipolaronic
Hamiltonian to a representation containing only the Bose operators a
m
and a
†
m
defined as
b
m
=
∞
k=0
β
k
(a
†
m
)
k
a
k+1
m
(4.173)
b
†
m
=
∞
k=0
β
k
(a
†
m
)
k+1
a
k
m
(4.174)
where
a
m
a
†
m
− a
†
m
a
m
= δ
m,m
. (4.175)
The first few coefficients β
k
are found by substituting equations (4.173) and
(4.174) into equations (4.167) and (4.168):
β
0
= 1 β
1
=−1 β
2
=
1
2
+
√
3
6
. (4.176)

Bipolaronic superconductivity
137
We also introduce bipolaron and boson -operators as
(r) =
1
√
N
m
δ(r − m)b
m
(4.177)
(r) =
1
√
N
m
δ(r − m)a
m
. (4.178)
The transformation of the field operators takes the form:
(r) =
1 −
†
(r)(r)
N
+
(1/2 +
√
3/6)
†
(r)(r)(r)
N
2
+···
(r).
(4.179)
Then we write the bipolaronic Hamiltonian as
H
b
=
dr
dr
†
(r)t (r − r
)(r
) + H
d
+ H
K
+ H
(3)
(4.180)
where
H
d
=
1
2
dr
dr
¯v(r − r
)
†
(r)
†
(r
)(r
)(r) (4.181)
is the dynamic part,
H
K
=
2
N
dr
dr
t (r − r
)
×[
†
(r)
†
(r
)(r
)(r
) +
†
(r)
†
(r)(r)(r
)]. (4.182)
is the kinematic (hard-core) part due to the ‘imperfect’ commutation rules, and
H
(3)
includes three- and higher-body collisions. Here
t (r − r
) =
k
∗∗
k
e
ik·(r−r
)
¯v(r − r
) =
1
N
k
¯v
k
e
ik·(r−r
)
¯v
k
=
m=0
¯v(m) exp(ik·m) is the Fourier component of the dynamic interaction
and
∗∗
k
=
m=0
t (m) exp(−ik · m) (4.183)
is the bipolaron band dispersion. H
(3)
contains powers of the field operator higher
than four. In the dilute limit (n
b
1) only two-particle interactions are essential
which include the short-range kinematic and direct density–density repulsions.
Because ¯v already has a short-range part v
(2)
(equation (4.129)), the kinematic
138
Strong-coupling theory
contribution can be included in the definition of ¯v.AsaresultH
b
is reduced to
the Hamiltonian of interacting hard-core charged bosons tunnelling in a narrow
band.
To describe the electrodynamics of bipolarons, we introduce the vector
potential A(r) using the so-called Peierls substitution [111]:
t (m − m
) → t (m − m
)e
i2e A(m)·(m−m
)
which is a fair approximation when the magnetic field is weak compared with the
atomic field, eHa
2
1 (see also section 2.16). It has the following form:
t (r −r
) → t (r, r
) =
k
∗∗
k−2e A
e
ik·(r−r
)
(4.184)
in real space. If the magnetic field is weak, we can expand
∗∗
k
in the vicinity of
k = 0toobtain
t (r, r
) ≈−
[∇ + 2ie A(r)]
2
2m
∗∗
δ(r − r
) (4.185)
where
1
m
∗∗
=
d
2
∗∗
k
dk
2
k→0
(4.186)
is the inverse bipolaron mass. Here we assume a parabolic dispersion near the
bottom of the band,
∗∗
k
k
2
. Finally, we arrive at
H
b
≈−
dr
†
(r)
[∇ + 2ie A(r)]
2
2m
∗∗
+ µ
(r)
+
1
2
dr dr
¯v(r − r
)
†
(r)
†
(r
)(r)(r
) (4.187)
where we include the bipolaron chemical potential µ. We note that the bipolaron–
bipolaron interaction is the Coulomb repulsion, ¯v(r) ∼ 1/(
0
r) at large distances
(section 4.4) and the hard-core repulsion is irrelevant in the dilute limit. Hence,
equation (4.187) describes a charged Bose gas (CBG) with the effective boson
mass m
∗∗
and charge 2e.
4.7.2 Bogoliubov equations in the strong-coupling regime
Let us derive equations describing the order parameter and excitations of CBG by
the use of the Bogoliubov transformation similar to that in chapter 2. The equation
of motion for the field operator, ψ(r, t), is derived using the Hamiltonian (4.187)
as
i
d
dt
ψ(r, t) =[H,ψ(r, t)]=
−
(∇ − i2e A)
2
2m
∗∗
− µ
ψ(r, t)
+
dr
¯v(r − r
)ψ
†
(r
, t)ψ(r
, t)ψ(r, t). (4.188)
Bipolaronic superconductivity
139
A large value for the static dielectric constant (
0
1) in ionic solids makes the
Coulomb repulsion between bipolarons rather weak. If the interaction is weak, we
expect that some properties of CBG to be similar to the properties of an ideal Bose
gas (appendix B). In particular, the state with zero momentum (k = 0) remains
macroscopically occupied and the corresponding Fourier component of the field
operator ψ(r, t) has an anomalously large matrix element between the states with
N + 1andN bosons. Introducing the chemical potential µ we consider each
quantum state as a superposition of states with a slightly different total number
of bosons. The weight of each state in the superposition is a smooth function of
N which is practically unchanged in the window ±
√
¯
N near the average number
¯
N . Hence, because ψ changes the number of particles only by one, its diagonal
matrix element is practically the same as the off-diagonalone, calculated for states
with fixed N =
¯
N + 1andN =
¯
N . Following Bogoliubov [4] we separate the
large diagonal matrix element ψ
s
from ψ treating ψ
s
as a number and the rest,
˜
ψ,
as a small fluctuation
ψ(r, t) = ψ
s
(r, t) +
˜
ψ(r, t). (4.189)
Substituting equation (4.189) into equation (4.188) and collecting c-number terms
of ψ
s
we obtain a set of Bogoliubov-type equations for the CBG [112]. The
macroscopic condensate wavefunction ψ
s
(r, t), which plays the role of an order
parameter, obeys the following equation:
i
d
dt
ψ
s
(r, t) =
−
(∇ + i2e A)
2
2m
∗∗
− µ
ψ
s
(r, t) +
dr
¯v(r − r
)n
s
(r
, t)ψ
s
(r, t)
(4.190)
which is a generalization of the so-called Gross–Pitaevskii (GP) [113]
equation applied to neutral bosons. Taking into account the interaction
of ‘supracondensate’ bosons (described by
˜
ψ(r, t)) with the condensate,
and neglecting the interaction between the supra-condensate bosons, from
equation (4.188) we also obtain
i
d
dt
˜
ψ(r, t) =
−
(∇ + i2e A)
2
2m
∗∗
− µ
˜
ψ(r, t)
+
dr
¯v(r − r
)[n
s
(r, t) + ψ
∗
s
(r
, t
)ψ
s
(r, t)]
˜
ψ(r
, t)
+
dr
¯v(r − r
)ψ
s
(r
, t)ψ
s
(r, t)
˜
ψ
†
(r
, t). (4.191)
Here
n
s
(r, t) =|ψ
s
(r, t)|
2
(4.192)
is the condensate density. If the Coulomb repulsion of bosons is not very large,
r
s
=
4m
∗∗
e
2
0
(4πn
b
/3)
1/3
1 (4.193)

140
Strong-coupling theory
the number of bosons ˜n pushed up from the condensate by the repulsion is
small (see later). Therefore, the contribution of the terms nonlinear in
˜
ψ is
negligible in equation (4.191). Applying the linear Bogoliubov transformation
of
˜
ψ (chapter 2),
˜
ψ(r, t) =
n
[u
n
(r, t)α
n
+ v
∗
n
(r, t)α
†
n
] (4.194)
where now α
n
and α
†
n
are Bose operators, we obtain two coupled Schr¨odinger
equations for the quasi-particle wavefunctions, u(r, t) and v(r, t),as
i
d
dt
u(r, t) =
−
(∇ + i2e A)
2
2m
∗∗
− µ
u(r, t)
+
dr
¯v(r − r
)[|ψ
s
(r
, t)|
2
u(r, t) + ψ
∗
s
(r
, t)ψ
s
(r, t)u(r
, t)]
+
dr
¯v(r − r
)ψ
0
(r
, t)ψ
0
(r, t)v(r
, t) (4.195)
and
−i
d
dt
v(r, t) =
−
(∇ − i2e A)
2
2m
∗∗
− µ
v(r, t)
+
dr
¯v(r − r
)[|ψ
s
(r
, t)|
2
v(r, t) + ψ
s
(r
, t)ψ
∗
s
(r, t)]v(r
, t)
+
dr
¯v(r − r
)ψ
∗
s
(r
, t)ψ
∗
s
(r, t)u(r
, t). (4.196)
There is also a sum rule,
n
[u
n
(r, t)u
∗
n
(r
, t) − v
n
(r, t)v
∗
n
(r
, t)]=δ(r − r
) (4.197)
which retains the Bose commutation relations for new operators. The set of
equations (4.190), (4.195) and (4.196) plays the same role in the strong-coupling
theory as the Bogoliubov equations for the BCS superconductors (section 2.11).
4.7.3 Excitation spectrum and ground-state energy
For a homogeneous case with A(r) = 0, the quasi-particle wavefunctions are
plane waves,
u
k
(r, t) = u
k
e
ik·r−i
k
t
(4.198)
v
k
(r, t) = v
k
e
ik·r−i
k
t
Bipolaronic superconductivity
141
and the condensate wavefunction is (r, t)-independent, ψ
s
(r, t) =
√
n
s
=
constant. Then a solution of equation (4.190) is
µ = 0 (4.199)
because the volume average of ¯v(r) is zero due to the interaction with the ion
background (i.e. because of the global electroneutrality). Then the Bogoliubov
equations yield
k
u
k
=
k
2
2m
∗∗
u
k
+ n
s
¯v
k
[u
k
+ v
k
] (4.200)
−
k
v
k
=
k
2
2m
∗∗
v
k
+ n
s
¯v
k
[u
k
+ v
k
] (4.201)
and
|u
k
|
2
−|v
k
|
2
= 1. (4.202)
Here ¯v
k
is the Fourier transform of ¯v(r). As a result, we find
u
2
k
=
1
2
1 +
ξ
k
k
(4.203)
v
2
k
=−
1
2
1 −
ξ
k
k
(4.204)
u
k
v
k
=−
¯v
k
n
s
2
k
(4.205)
where ξ
k
= k
2
/2m
∗∗
+¯v
k
n
s
. The quasi-particle energy is
k
=
k
4
4(m
∗∗
)
2
+
k
2
¯v
k
n
s
m
∗∗
. (4.206)
Using the Fourier component of the Coulomb interaction yields [114]
k
=
k
4
4(m
∗∗
)
2
+ ω
2
ps
(4.207)
with a gap
ω
ps
=
16πe
2
n
s
0
m
∗∗
(4.208)
which is the plasma frequency. The quasi-particle spectrum (equation (4.207))
differs qualitatively from the BCS excitation spectrum (section 2.2). The BCS
quasi-particles are fermions and their energy is of the order of the BCS gap,
k
,
which is well below the electron plasma frequency,
k
ω
e
. The quasi-particles
in CBG are bosons and their energy is about the (renormalized) plasma frequency
142
Strong-coupling theory
ω
ps
. The density of bosons pushed up from the condensate by the Coulomb
repulsion at T = 0is
˜n =
˜
ψ
†
(r)
˜
ψ(r)=
k
v
2
k
(4.209)
which is small compared with the total density n
b
as
˜n
n
b
≈ 0.2r
3/4
s
. (4.210)
The ground state |0 is a vacuum of quasi-particles, α
k
|0=0. The ground-
state energy E
0
is obtained by substituting equation (4.194) into the Hamiltonian
(4.187) and neglecting terms of higher order than quadratic in α
k
,
E
0
≡0|H |0=
1
2
k
(
k
− ξ
k
). (4.211)
This can be written per particle as
E
0
n
b
=
2
3/2
3
1/4
π
ω
ps
r
3/4
s
∞
0
dkk
2
k
4
+ 1 − k
2
− 1/(2k
2
)
≈−0.23ω
ps
r
3/4
s
.
(4.212)
The negative value of the ground-state energy is due to the opposite charge
background. The value of |E
0
| is considered as the gain in the total energy
due to the condensation of interacting bosons with respect to the ground-state
energy (= 0) of an ideal Bose gas. Therefore, |E
0
| plays the same role as the
condensation energy in the BCS superconductor.
These results for three-dimensional charged bosons are readily generalized
for any bosons on a lattice beyond the effective mass approximation. For example,
the quasi-particle spectrum is given by
k
=
(
∗∗
k
)
2
+ 2
∗∗
k
¯v
k
n
s
. (4.213)
Hence, if the free-boson dispersion
∗∗
k
is anisotropic, the plasma gap is
anisotropic as well. In an extreme case of (quasi-)two-dimensional bosons
with a parabolic dispersion and a two-dimensional Coulomb repulsion, ¯v
k
=
8πe
2
/(
0
k), the Bogoliubov spectrum is gapless,
k
= E
s
k/q
s
+ k
4
/q
4
s
. (4.214)
Here E
s
= q
2
s
/2m
∗∗
and q
s
= (32πe
2
n
s
/
0
)
1/3
is a two-dimensional screening
wave-number, n
s
is the number of condensed bosons per unit area.
4.7.4 Mixture of two Bose condensates
The quasi-particle spectrum (equation (4.207)) satisfies the Landau criterion of
superfluidity (chapter 1), therefore the CBG is a superconductor. The actual
Bipolaronic superconductivity
143
spectrum of bipolarons on a lattice is more complicated, because inter-site
bipolarons have ‘internal’ quantum numbers like the spin (section 4.6), orbital
momentum and different symmetries of the one-particle Wannier orbitals bound
into the bipolaron. These internal degrees of freedom can affect the collective
excitations of a bipolaronic superconductor. Here we extend the Bogoliubov-type
equations of the previous subsection to the multi-component Bose condensate of
non-converting charged bosons [115]. Let us consider a two-component (1 and 2)
mixture of bosons described by
H =
j =1,2
dr
†
j
(r)
−
∇
2
2m
j
− µ
j
j
(r)
+
1
2
j, j
dr
dr
¯v
jj
(r − r
)
†
j
(r)
j
(r)
†
j
(r
)
j
(r
)
where m
j
is the mass of the boson j .
Using the displacement transformation (equation (4.189)) and the equations
of motion for the Heisenberg ψ-operators, the condensate wavefunctions are
found from two coupled GP equations:
i
∂
∂t
ψ
sj
(r, t) =
−
∇
2
2m
j
− µ
j
ψ
sj
(r, t)
+
j
dr
V
jj
(r − r
)|ψ
sj
(r
, t)|
2
ψ
sj
(r, t). (4.215)
The supracondensate wavefunctions satisfy four Bogoliubov-type equations:
i
∂
∂t
u
j
(r, t) =
−
∇
2
2m
j
− µ
j
u
j
(r, t) +
j
dr
V
jj
(r − r
)
×[|ψ
sj
(r
, t)|
2
u
j
(r, t) + ψ
∗
sj
(r
, t)ψ
sj
(r, t)u
j
(r
, t)
+ ψ
sj
(r
, t)ψ
sj
(r, t)v
j
(r
, t)]. (4.216)
and
−i
∂
∂t
v
j
(r, t) =
−
∇
2
2m
j
− µ
j
v
j
(r, t) +
j
dr
V
jj
(r − r
)
×[|ψ
sj
(r
, t)|
2
v
j
(r, t) + ψ
sj
(r
, t)ψ
∗
sj
(r, t)v
j
(r
, t)
+ ψ
∗
sj
(r
, t)ψ
∗
sj
(r, t)u
j
(r
, t)]. (4.217)
Here we applied the linear transformation of
˜
ψ,
˜
ψ
j
(r, t) =
n
u
nj
(r, t)(α
n
+ β
n
) + v
∗
nj
(r, t)(α
†
n
+ β
†
n
)
144
Strong-coupling theory
where α
n
,β
n
are bosonic operators annihilating quasi-particles in the quantum
state n. Solving two GP equations (4.215), we obtain the chemical potentials of a
homogeneous system as
µ
1
=¯vn
1
+¯wn
2
µ
2
=¯un
2
+¯wn
1
and solving the four Bogoliubov equations, we determine the excitation spectrum,
E(k), from
Det
ξ
1
(k) − E(k) ¯v
k
ψ
2
s1
¯w
k
ψ
s1
ψ
∗
s2
¯w
k
ψ
s1
ψ
s2
¯v
k
ψ
∗2
s1
ξ
1
(k) + E(k) ¯w
k
ψ
∗
s1
ψ
∗
s2
¯w
k
ψ
∗
s1
ψ
s2
¯w
k
ψ
∗
s1
ψ
s2
¯w
k
ψ
s1
ψ
s2
ξ
2
(k) − E(k) ¯u
k
ψ
2
s2
¯w
k
ψ
∗
s1
ψ
∗
s2
¯w
k
ψ
s1
ψ
∗
s2
¯u
k
ψ
∗2
s2
ξ
2
(k) + E(k)
= 0.
(4.218)
Here ξ
1
(k) = k
2
/2m
1
+¯v
k
n
1
, ξ
2
(k) = k
2
/2m
2
+¯u
k
n
2
and ¯v
k
, ¯u
k
, ¯w
k
are the
Fourier components of ¯v
11
(r), ¯v
22
(r) and ¯v
12
(r), respectively, ¯v ≡¯v
0
, ¯u ≡¯u
0
,
¯w ≡¯w
0
and n
j
=|ψ
sj
|
2
are the condensate densities. There are two branches of
excitations with dispersion
E(k)
1,2
= 2
−1/2
2
1
(k) +
2
2
(k) ±
[
2
1
(k) −
2
2
(k)]
2
+
4k
4
m
1
m
2
¯w
2
k
n
1
n
2
1/2
(4.219)
where
1
(k) =[k
4
/(4m
2
1
) + k
2
¯v
k
n
1
/m
1
]
1/2
and
2
(k) =[k
4
/(4m
2
2
) +
k
2
¯u
k
n
2
/m
2
]
1/2
are Bogoliubov modes of two components. If the interaction
is purely the Coulomb repulsion, ¯v
k
= 4π q
2
1
/k
2
, ¯u
k
= 4π q
2
2
/k
2
and ¯w
k
=
4πq
1
q
2
/k
2
, the upper branch is the geometric sum of familiar plasmon modes
for k → 0,
E
1
(k) =
4πq
2
1
n
1
m
1
+
4πq
2
2
n
2
m
2
(4.220)
while the lowest branch is gapless,
E
2
(k) =
k
2
2(m
1
m
2
)
1/2
q
2
1
n
1
m
1
+ q
2
2
n
2
m
2
q
2
1
n
1
m
2
+q
2
2
n
2
m
1
. (4.221)
Remarkably, this mode does not depend on the interaction at any charges of
the components, if m
1
= m
2
. It corresponds to a low-frequency oscillation in
which two condensates move in anti-phase with one another, in contrast to the
usual optical high-frequency plasmon (equation (4.220)) in which the components
oscillate inphase. The mode is similar to the acoustic plasmon (AP) mode in the
electron–ion [116] and electron–hole [117] plasmas. However, differing from
these normal-state APs with a linear dispersion, the AP of Bose mixtures has a
quadratic dispersion in the long-wavelength limit. We conclude that while the
Bipolaronic superconductivity
145
0.0 0.5 1.0
0
1
2
E(k)/
ω
p
k/q
s
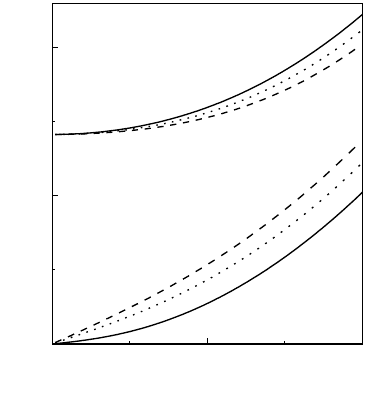
Figure 4.10. Excitation energy spectrum of the two-component Bose–Einstein condensate
with the long-range repulsive and hard-core interactions. In this plot n
1
= n
2
= n,
m
1
= m
2
and ¯u =¯v = ω
p
, q
1
= q
2
= e. Different curves correspond to the different
values of ¯w/¯v = 0.1(dashes),0.5 (dots), and 0.95 (full line), respectively. Excitation
energy is measured in units of the plasma frequency ω
p
= 4πne
2
/m and momentum k is
measured in inverse screening length q
s
= (16πe
2
nm)
1/4
.
CBG is a superfluid (according to the Landau criterion), their mixture is not.
In the case of bipolarons, the interaction includes both the long-range repulsion
and the hard-core interaction (section 4.7.1). Combining both interactions, i.e.
taking ¯v
k
, ¯u
k
, ¯w
k
∝ constant + 1/k
2
, transforms the lowest AP mode into the
Bogoliubov sound with a linear dispersion at k → 0, figure 4.10. Hence, the
two-component condensate of bipolarons is a superconductor.
4.7.5 Critical temperature and isotope effect
Polarons and bipolarons differ from electrons in many ways. One of the
differences is their effective mass. Electrons change their mass in solids due to
interactions with ions, spins and between themselves. Because λ does not depend
on the ion mass, the renormalized mass of electrons is independent of the ion
mass M in ordinary metals, where the Migdal adiabatic approximation is believed
to be valid (chapter 3). However, if the interaction between electrons and ion
vibrations is strong and/or the adiabatic approximation is not applied, electrons
form polarons and their effective mass m
∗
depends on M as m
∗
= m exp(A/ω
0
)
[82] (section 4.3.1), where m is the band mass in the absence of the electron–
phonon interaction, A is a constant independent of M and ω
0
is the characteristic
