Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics

This page intentionally left blankThis page intentionally left blank

Quantum

Bio-Informatics

IV

eds. L.

Accardi,

W.

Freud

enbe

rg

and

M.

Ohya

World

Scientific

Publishing

Co.

(pp.

91

- 99)

NON-MARKOVIAN

DYNAMICS

OF

QUANTUM

SYSTEMS

DARIUSZ

CHRU8CIl\[SKI

and

ANDRZEJ

KOSSAKOWSKI

Institute

of

Physics,

Nicolaus

Copernicus

University,

Grudzig,dzka

5/7,

87-100

Toru'Ti,

Poland

We

analyze

a local

approach

to

the

non-Markovian

evolution

of

open

quantum

systems.

It

turns

out

that

any

dynamical

map

repres

e

nting

evolution

of

such

a

system

may

be

described

either

by

non-loc

al

master

equation

with

memory

kernel

or

equival

e

ntly

by

equation

which

is

local

in

time.

The

price

one

pays

for

the

local

approach

is

that

the

corresponding

generator

might

be

highly

singular

and

it

keeps

the

memory

about

th

e

starting

point

'to'.

Remarkably,

singularities

of

generator

may

lead

to

interesting

physical

phenomen

a like

revival

of

coherence

or

sudden

death

and

revival

of

entanglement.

Keywords:

Open

systems;

quantum

dynamics;

non-Markovian

evolution.

1.

Introduction

The

non-Markovian dynamics of

open

quantum

systems

attracts

nowadays

increasing

attention.

1

It

is very

much

connected

to

the

growing

interest

in

controlling

quantum

systems

and

applications in

modern

quantum

tech-

nologies such as

quantum

communication,

cryptography

and

computation.

2

It

turns

out

that

the

popular

Markovian

approximation

which does

not

take

into account

memory

effects is

not

sufficient for

modern

applications

and

to

days technology calls for

truly

non-Markovian approach. Non-Markovian

dynamics was recently

studied

in Refs. 3- 18.

The

usual

approach

to

the

dynamics

of

an

open

quantum

syst

em

consists

in

applying

the

Markovian approximation,

that

leads

to

the

following local

master

equation

d

dtP(t) = .eMP(t) , p(to) = Po,

(1)

where p(t) is

the

density

matrix

ofthe

system

investigated

and.e

M

the

time-

independent

generator

of

the

dynamical

semigroup possessing

the

following

91

92

well known

representation

19

,

20,21

LMP= -i[H,p] + L (VaPVd -

~{VdVa,p})

'"

(2)

The

above

structure

of

LM

guaranties

that

dynamical

map

A(t, to), defined

by

p(t) = A(t,

to)Po,

is completely positive

and

trace

preserving for t

2:

to·

Note

that

A(t,

to)

itself satisfies Markovian

master

equation

d

dt A(t,

to)

=

LM

A(t,

to),

A(to,to) = ] ,

(3)

and

the

solution for A(t,

to)

is given by

A(t,

to)

=

e(t

-

tO)LM

,

(4)

which implies

that

A(t,

to)

depends

only

upon

the

difference 't - to'

and

hence A(t)

:=

A(t,O) defines a

I-parameter

semi

group

(t

2:

0) satisfying

homogeneous composition law

(5)

for t1, t2

2:

O.

In general

the

external

conditions which influence

the

dynam-

ics

of

an

open

system

may

very

in

time.

The

natural

generalization

of

the

Markovian

master

equation

(1)

involves

time-dependent

generator

LM(t)

which

has

exactly

the

same

representation

as in (2)

with

time-dependent

Hamiltonian

H(t)

and

time-dependent

Lindblad

operators

V",(t). Therefore

one gets

the

following

master

equation

for

the

dynamical

map

A(t,

to)

d

dt

A(t, to) = LM(t) A(t,

to),

A(to, to) = ] ,

(6)

which leads

to

the

following solution

A(t,

to)

= T exp

(1:

LM(T)dT) ,

(7)

where T

stands

for

the

chronological

operator.

Clearly, A(t,

to)

no longer

depends

upon

't - to'

but

it

still satisfies inhomogeneous composition law

A(t,s)·A(s,to)

= A(t,

to)

,

(8)

for t

2:

s

2:

to.

We stress

that

(6)

although

time-dependent

is perfectly

Markovian. Note,

that

the

solution (7)

has

only a formal

meaning

since

the

evaluation

of

T-product

is

in

general

not

feasible.

In

this

paper

we analyze a non-Markovian dynamics A(t,

to)

which does

not

satisfy

the

composition law -

this

is

the

essence

of

non-Markovianity.

93

In

the

we

present a general

strategy

to

the

description

of

non-

Markovian evolution

based

on

the

local

in

time

generator,

and

in

section 3

we

present

simple examples

to

illustrate

a general approach.

Final

remarks

are collected in section 4.

2.

Local

vs.

non-local

approach

The

standard

approach

to

the

dynamics

of

open

system

uses

the

Nakajima-

Zwanzig

projection

operator

technique

22

(see also Refs.

1,

21) which shows

that

under

fairly general conditions,

the

master

equation

for

the

reduced

density

matrix

pet)

takes

the

form

of

the

following non-local

equation

d

it

-d

pet)

= K(t - u)p(u) du ,

t

to

p(to) =

Po

,

(9)

in

which

quantum

memory

effects

are

taken

into

account

through

the

in-

troduction

of

the

memory

kernel K(t):

this

simply

means

that

the

rate

of

change

of

the

state

pet)

at

time

t

depends

on

its

history

(starting

at

t = to).

Equivalently,

one

has

the

following nonlocal

equation

for

the

dynamical

map

d

it

-d

A(t,

to)

= Ket - u)A(u,

to)

du ,

t to

A(to,

to)

= n .

(10)

Let

us observe

that

homogeneity

of

K (it

depends

upon

the

difference

't-u')

implies

that

the

corresponding

solution

A(t,

to)

is homogeneous as well.

This

property

enables one

to

rewrite

(10)

as follows

d

t

dt A(t) =

Jo

Ket - u)A(u) du ,

A(O)

= n ,

(11)

where

A(t)

:=

A(t +

to,

to).

This

form is usually

studied

in

the

literature

1

.

Note, however,

that

there

is no need

to

provide

the

initial

condition

at

to

=

o.

Equation

(10)

allows

to

take

completely

arbitrary

to.

Unfortunately, we

do

not

know

condition

for

the

memory

kernel K(t)

which

guarantee

that

the

corresponding

dynamical

map

A(t,

to)

is a legiti-

mate

quantum

evolution, i.e.

it

is completely positive

and

trace

preserving.

Therefore,

instead

of

non-local

approach

we propose

to

analyze

much

sim-

pler

approach

which is

based

on

the

local

in

time

Master

Equation

(this

approach

is usually called time-convolutionless (TCL)1,23). Note,

that

each

solution A(t)

of

(11) satisfies

the

following local in

time

equation

18

d

dt A(t,

to)

=

L(t

- to)A(t,

to),

A(to,

to)

= n ,

(12)

94

where

the

time-dependent

generator

£(t)

is defined

by

the

following loga-

rithmic

derivative of

the

dynamical

map

(13)

and

A(t)

:=

A(t

+

to,

to).

Let

us observe

that

Eq. (12) is local

in

time

but

its

generator

does

remember

about

the

starting

point

'to'.

This

is

the

most

important

difference

with

the

time-dependent

Markovian

equation

(6).

The

appearance

of 'to'

in

the

generator

£(t-t

o

)

implies

that

£ is effectively non-

local in

time,

that

is,

it

contains

a memory. Therefore,

the

local

equation

(12) is non-Markovian

contrary

to

the

local

equation

(6) which does does

not

keep

any

memory

about

to.

Note,

that

solution

to

(12) is given by

A(t,

to)

= T

exp

(fat-to

£(T) dT) .

(14)

It

shows

that

A(t,

to)

is indeed homogeneous in

time

(depends

on

't - to').

However,

contrary

to

(7),

it

does

not

satisfy

the

composition

law. Again,

this

is a clear sign for

the

memory

effect.

Now comes

the

natural

question: how

to

construct

non-Markovian

generator

£(t)

which does

guarantee

legitimate

quantum

dynamics.

The

general answer is

not

known

but

one

may

easily propose special con-

structions.

Let

£M

be

a

Markovian

generator

defined

by

(2)

and

de-

fine

£(t)

= a(t)£M'

It

is clear

that

if

J~

a(u)du

:2:

0 for t

:2:

0,

then

A(t) =

exp(J~

a(u)du£M)

defines completely positive non-Markovian dy-

namics.

This

construction

may

be

generalized as follows: consider N

mutu-

ally

commuting

Markovian

generators

£~),

...

,£r/)

and

N real functions

ak(t) satisfying

J~

ak(u)du

:2:

O.

Then

r(

) ( )

r(1)

( )

rCN)

J..-

t =

a1

t

J..-M

+ ... +

aN

t

J..-M

,

(15)

serves

as

a

generator

of

non-Markovian evolution.

Let

£(t)

(t

:2:

0)

be

a

commuting

family

of

superoperators,

i.e. [£(t),£(s)] =

O.

If

the

integral

J~

£(u)du

defines for each t

:2:

0 a

legitimate

Lindblad

generator,

then

£(t)

generates

legitimate

non-Markovian evolution.

If

the

family

£(t)

(t

:2:

0)

is

non-commuting

then

the

problem

of necessary

and

sufficient

condition

for

£

(t)

is still open.

3.

Examples

To

illustrate

our

approach

let us consider

the

following simple examples.

Throughout

this

section

we

put

A(t)

:=

A(t, 0)

and

define

the

initial value

problem

at

to.

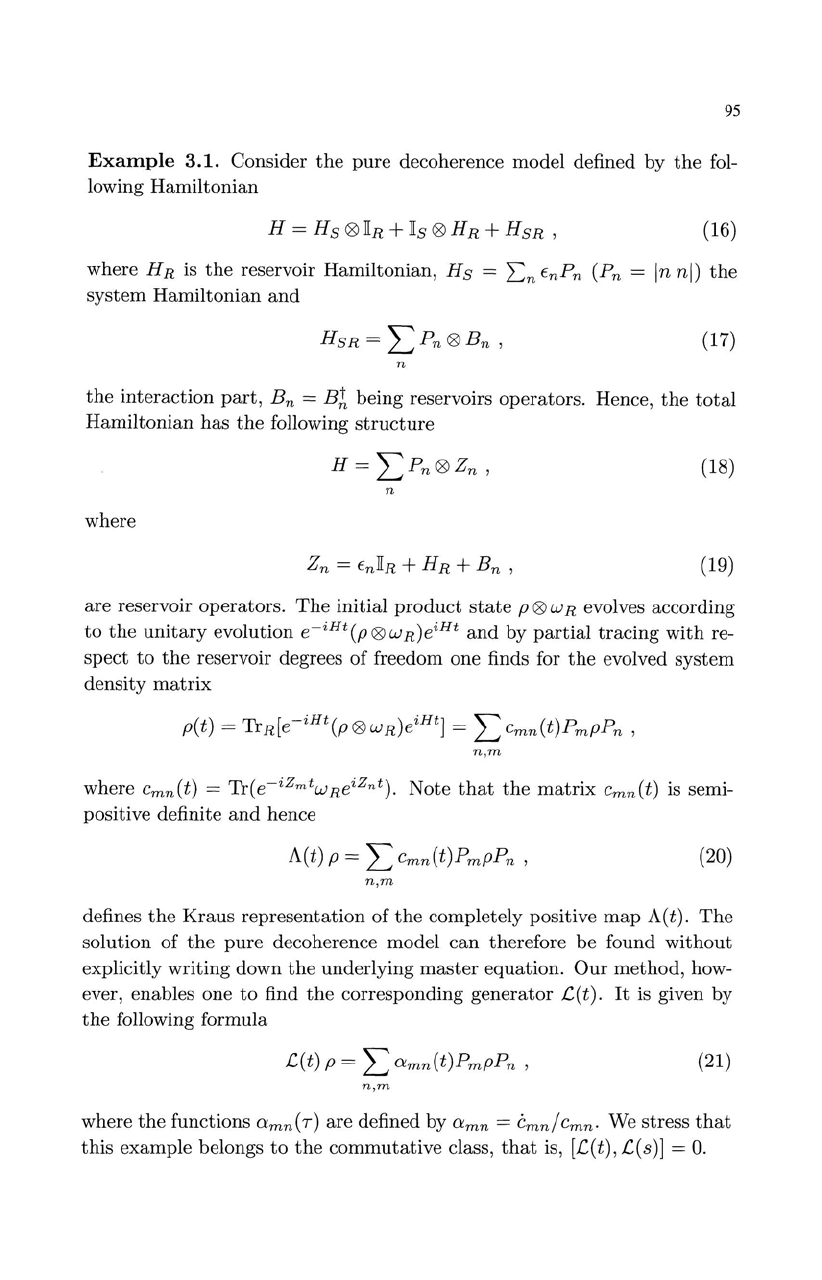
95

Example

3.1.

Consider

the

pure

decoherence

model

defined

by

the

fol-

lowing

Hamiltonian

(16)

where HR is

the

reservoir

Hamiltonian,

Hs

=

Ln

EnP

n

(P

n

=

In

nl)

the

system

Hamiltonian

and

(17)

the

interaction

part,

Bn

=

B~

being reservoirs

operators.

Hence,

the

total

Hamiltonian

has

the

following

structure

(18)

n

where

(19)

are

reservoir

operators.

The

initial

product

state

p ® W R evolves according

to

the

unitary

evolution

e-

iHt

(p

® W

R)e

iHt

and

by

partial

tracing

with

re-

spect

to

the

reservoir degrees

of

freedom one finds for

the

evolved

system

density

matrix

n,m

where cmn(t)

=

Tr(e-iz=twReiZnt).

Note

that

the

matrix

cmn(t) is semi-

positive definite

and

hence

(20)

n,m

defines

the

Kraus

representation

of

the

completely positive

map

A(t).

The

solution

of

the

pure

de coherence

model

can

therefore

be

found

without

explicitly

writing

down

the

underlying

master

equation.

Our

method,

how-

ever, enables one

to

find

the

corresponding

generator

L(t).

It

is given

by

the

following formula

(21)

n,m

where

the

functions

amn(T)

are

defined

by

a

mn

=

cmn/c

mn

. We

stress

that

this

example

belongs

to

the

commutative

class,

that

is, [L(t), L(s)] =

O.

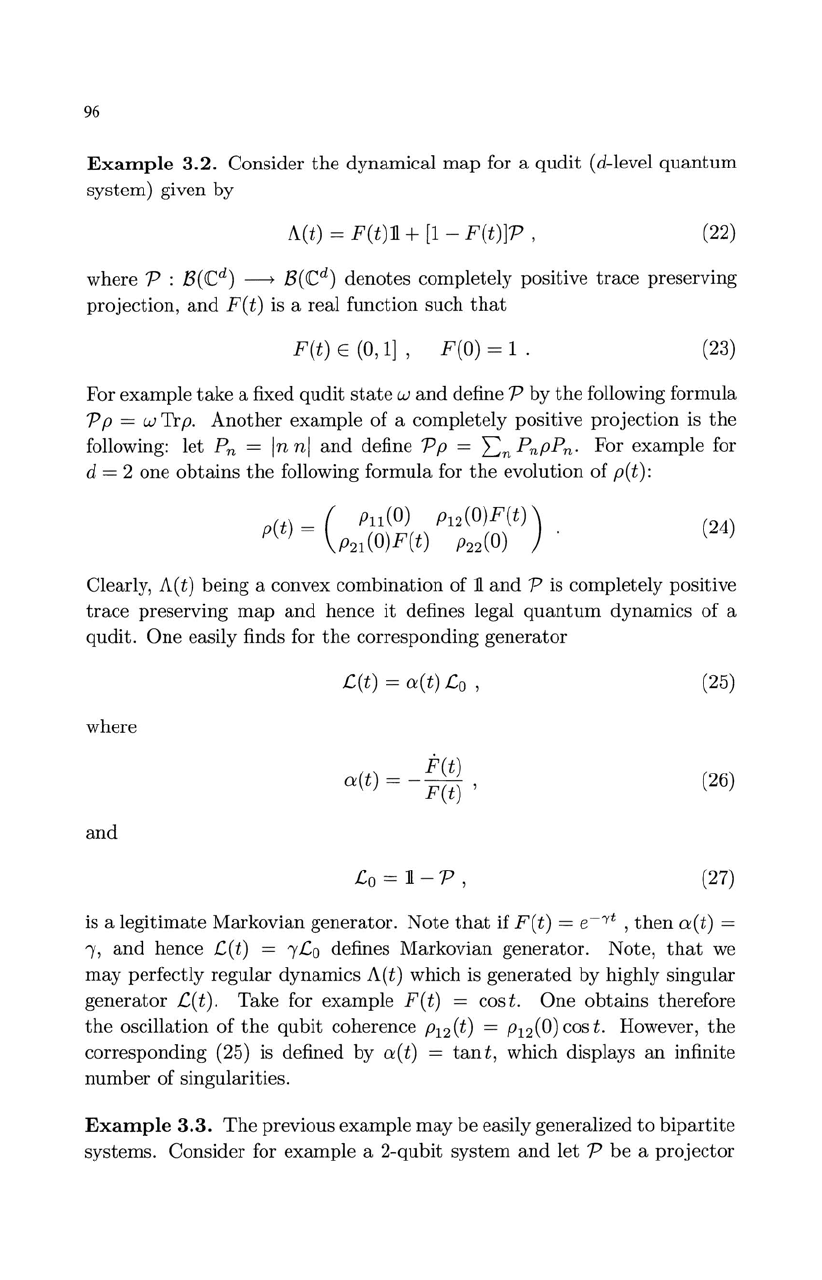
96

Example

3.2.

Consider

the

dynamical

map

for a

qudit

(d-Ievel

quantum

system) given by

A(t) = F(t)ll +

[1

- F(t)]1' ,

(22)

where

l'

: B(C

d

)

-------

B(C

d

)

denotes completely positive

trace

preserving

projection,

and

F(t) is a real function such

that

F(t) E

(0,1],

F(O)

= 1 .

(23)

For example

take

a fixed

qudit

state

wand

define

l'

by

the

following formula

l'

P = w Trp.

Another

example

of

a completely positive

projection

is

the

following: let P

n

=

In

nl

and

define

1'p

=

2:n

PnpP

n

. For example for

d = 2 one

obtains

the

following formula for

the

evolution

of

p(t):

P(t)-(

Pu(O) P12(0)F(t))

-

P21

(O)F(

t)

P22

(0)

.

(24)

Clearly, A(t) being a convex

combination

of

II

and

l'

is completely positive

trace

preserving

map

and

hence

it

defines legal

quantum

dynamics

of

a

qudit

.

One

easily finds for

the

corresponding

generator

where

and

.c(t) =

a(t).c

o

,

F(t)

a(t)

= - F(t) ,

.co

=

II

-1'

,

(25)

(26)

(27)

is a legitimate Markovian generator.

Note

that

if F(t) =

e-,t

,

then

a(t) =

"

and

hence .c(t) = ,.co defines Markovian generator. Note,

that

we

may

perfectly regular dynamics A(t) which is

generated

by highly singular

generator

.c(t). Take for example F(t) = cost. One

obtains

therefore

the

oscillation

of

the

qubit

coherence

P12(t)

=

P12(0)

cos t. However,

the

corresponding (25) is defined by a(t) =

tan

t, which displays

an

infinite

number

of singularities.

Example

3.3.

The

previous example

may

be

easily generalized

to

bipartite

systems. Consider for example a 2-qubit

system

and

let

l'

be

a

projector

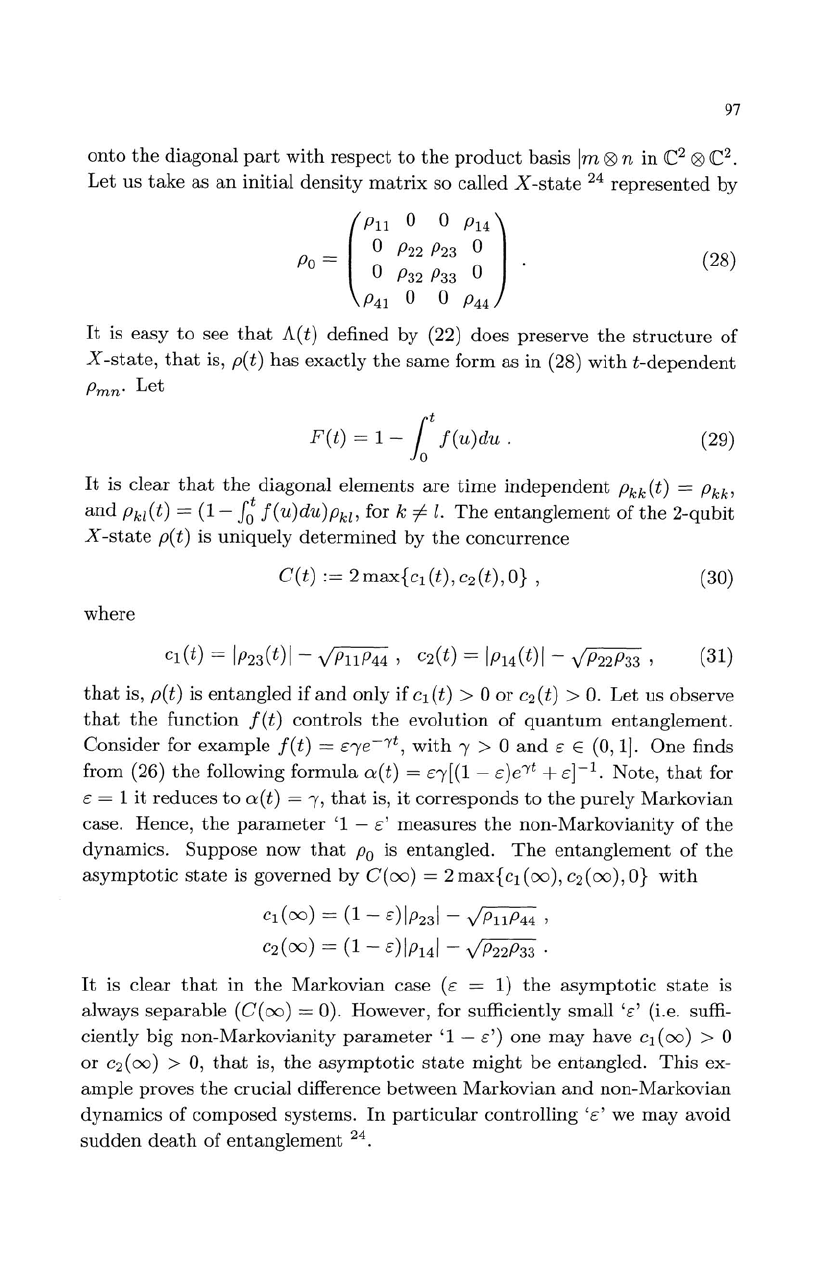
97

onto

the

diagonal

part

with

respect

to

the

product

basis

1m

(9

n

in

((:2

(9

((:2.

Let

us

take

as

an

initial density

matrix

so called X

-state

24

represented by

Po

=

(P~l

P~2 P~3

P~4)

.

o

P32 P33

0

P41

0 0

P44

(28)

It

is easy

to

see

that

A(t) defined by (22) does preserve

the

structure

of

X-state,

that

is, p(t)

has

exactly

the

same

form as

in

(28)

with

t-dependent

P

mn

. Let

F(t) = 1

-lot

f(u)du

.

(29)

It

is clear

that

the

t

diagonal elements

are

time

independent

Pkk(t) =

Pk~'

and

Pkl(t) =

(1-

fa

f(u)du)Pkl' for k

=1-1.

The

entanglement

of

the

2-qublt

X-state

p(t) is uniquely

determined

by

the

concurrence

C (t) : = 2

max

{

Cl

(t),

C2

(t),

O}

,

(30)

where

Cl(t)

=

Ip23(t)l-

VPllP44 ,

C2(t)

=

IP14(t)l-

VP22P33

,

(31)

that

is,

p(

t) is

entangled

if

and

only if

Cl

(t) > 0

or

C2

(t) >

O.

Let us observe

that

the

function

f(t)

controls

the

evolution

of

quantum

entanglement.

Consider for example

f(t)

=

qe-,t,

with

'Y

> 0

and

E E (0,1]. One finds

from (26)

the

following formula a(t) =

q[(l

-

E)e

lt

+

E]-l.

Note,

that

for

E = 1

it

reduces

to

a(t) =

'Y,

that

is,

it

corresponds

to

the

purely

Markovian

case. Hence,

the parameter

'1 -

E'

measures

the

non-Markovianity

of

the

dynamics. Suppose now

that

Po

is entangled.

The

entanglement

of

the

asymptotic

state

is governed by C(oo) =

2max{cdoo),

C2(00),

O}

with

Cl(oo) =

(1-

E)lp231- VPllP44 ,

C2(00)

=

(1

- E)lp141-

VP22P33

.

It

is clear

that

in

the

Markovian case

(E

=

1)

the

asymptotic

state

is

always separable

(C(oo) = 0). However, for sufficiently small

'E'

(i.e. suffi-

ciently big non-Markovianity

parameter

'1 -

E')

one

may

have

Cl

(00) > 0

or

C2(00)

>

0,

that

is,

the

asymptotic

state

might

be

entangled.

This

ex-

ample proves

the

crucial difference between Markovian

and

non-Markovian

dynamics

of

composed systems.

In

particular

controlling

'E'

we

may

avoid

sudden

death

of

entanglement

24.

98

4.

Conclusions

In

conclusion,

any

non-Markovian

quantum

evolution

may

be

described

ei-

ther

by

the

non-local

equation

(9)

or

by

a

time-local

equation

(12).

Local

approach

is

more

simple

and

well

suited

for

practical

purposes.

The

price

we

pay

for

the

local

approach

is

that

in

general

the

corresponding

gener-

ator

may

be

highly

singular

(for

example

£(t)

=

tan

t £3)

and

it

keeps a

memory

about

the

starting

point

'to'.

Our

examples

show

the

power

of

this

approach

-

one

is

able

to

provide

(possibly

singular)

local

generator

but

the

construction

of

the

corresponding

memory

kernel K(t) is

not

feasible.

We

stress

that

the

problem

of

necessary

and

sufficient

condition

for

the

local

generator

which

do

guarantee

that

the

corresponding

dynamical

map

gives rise

to

the

legitimate

quantum

evolution

is

still

open

and

it

deserves

further

studies.

Acknowledgments

This

work

was

partially

supported

by

the

Polish

Ministry

of

Science

and

Higher

Education

Grant

No

3004/B/H03/2007/33.

References

1.

H.-P.

Breuer

and

F.

Petruccione,

The Theory

of

Open Quantum

Systems

(Oxford Univ. Press, Oxford, 2007).

2.

M.

A. Nielsen

and

I.

L.

Chuang,

Quantum

Computation and Quantum In-

formation

(Cambridge

Univ. Press,

Cambridge,

2000).

3. J. Wilkie,

Phys.

Rev. E

62,

8808 (2000); J. Wilkie

and

Yin

Mei Wong, J.

Phys.

A:

Math.

Theor.

42,

015006 (2009).

4.

A. A.

Budini,

Phys.

Rev. A

69,

042107 (2004); ibid.

74,

053815 (2006).

5. H.-P.

Breuer,

Phys.

Rev. A

69

022115 (2004); ibid.

70,012106

(2004).

6.

S.

Daffer

et

al.

Phys.

Rev. A

70,

010304 (2004).

7.

A.

Shabani

and

D.A. Lidar,

Phys.

Rev. A

71,

020101(R) (2005).

8.

S.

Maniscalco,

Phys.

Rev. A

72,

024103 (2005).

9.

S.

Maniscalco

and

F.

Petruccione,

Phys.

Rev. A

73,

012111 (2006).

10.

J.

Piilo, K.

Harkonen,

S.

Maniscalco, K.-A. Suominen,

Phys.

Rev.

Lett.

100,

180402 (2008);

Phys.

Rev. A

79,

062112 (2009).

11. E. Andersson, J. D. Cresser

and

M. J.

W.

Hall, J. Mod.

Opt.

54,

1695 (2007).

12. A. Kossakowski

and

R. Rebolledo,

Open

Syst. Inf. Dyn.

14,

265 (2007); ibid.

15,

135 (2008).

13. A. Kossakowski

and

R. Rebolledo,

Open

Syst. Inf. Dyn.

16,

259 (2009).

14. H.-P.

Breuer

and

B. Vacchini,

Phys.

Rev.

Lett.

101

(2008) 140402; Phys.

Rev. E

79,

041147 (2009).

15. M.

Moodley

and

F.

Petruccione,

Phys.

Rev. A

79,

042103 (2009).

16. B. Vacchini

and

H.-P.

Breuer,

Phys.

Rev. A

81,

042103 (2010).

99

17. D. Chruscinski, A. Kossakowski,

and

S.

Pascazio,

Phys.

Rev. A

81,

032101

(2010)

18. D. Chruscinski

and

A. Kossakowski,

Phys.

Rev.

Lett

.

104,070406

(2010).

19. G.

Lindblad,

Comm.

Math.

Phys.

48,

119 (1976).

20. V. Gorini, A. Kossakowski,

and

E.C.G

.

Sudarshan,

J.

Math.

Phys.

17

, 821

(1976).

21. R. Alicki

and

K.

Lendi,

Quantum

Dynamical Semigroups and Applications

(Springer, Berlin, 1987).

22.

S.

Nakajima,

Prog.

Theor.

Phys.

20

, 948 (1958);

R.

Zwanzig, J.

Ch

ern. Phys.

33,

1338 (1960).

23. H.-P. Breuer, B.

Kappler

and

F.

Petruccione,

Phys.

Rev. A

59,1633

(1999).

24.

T.

Yu

and

J.

H.

Eb

erly,

Opt.

Comm

.

264

, 393 (2006); Q. Inf.

Comp

. 7, 459

(2007);

Phys

. Rev. Le

tt.

97

, 140403 (2006); ibid.

93

, 140404 (2004).

Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics

Подождите немного. Документ загружается.