Werner Leonhard Control of Electrical Drives
Подождите немного. Документ загружается.

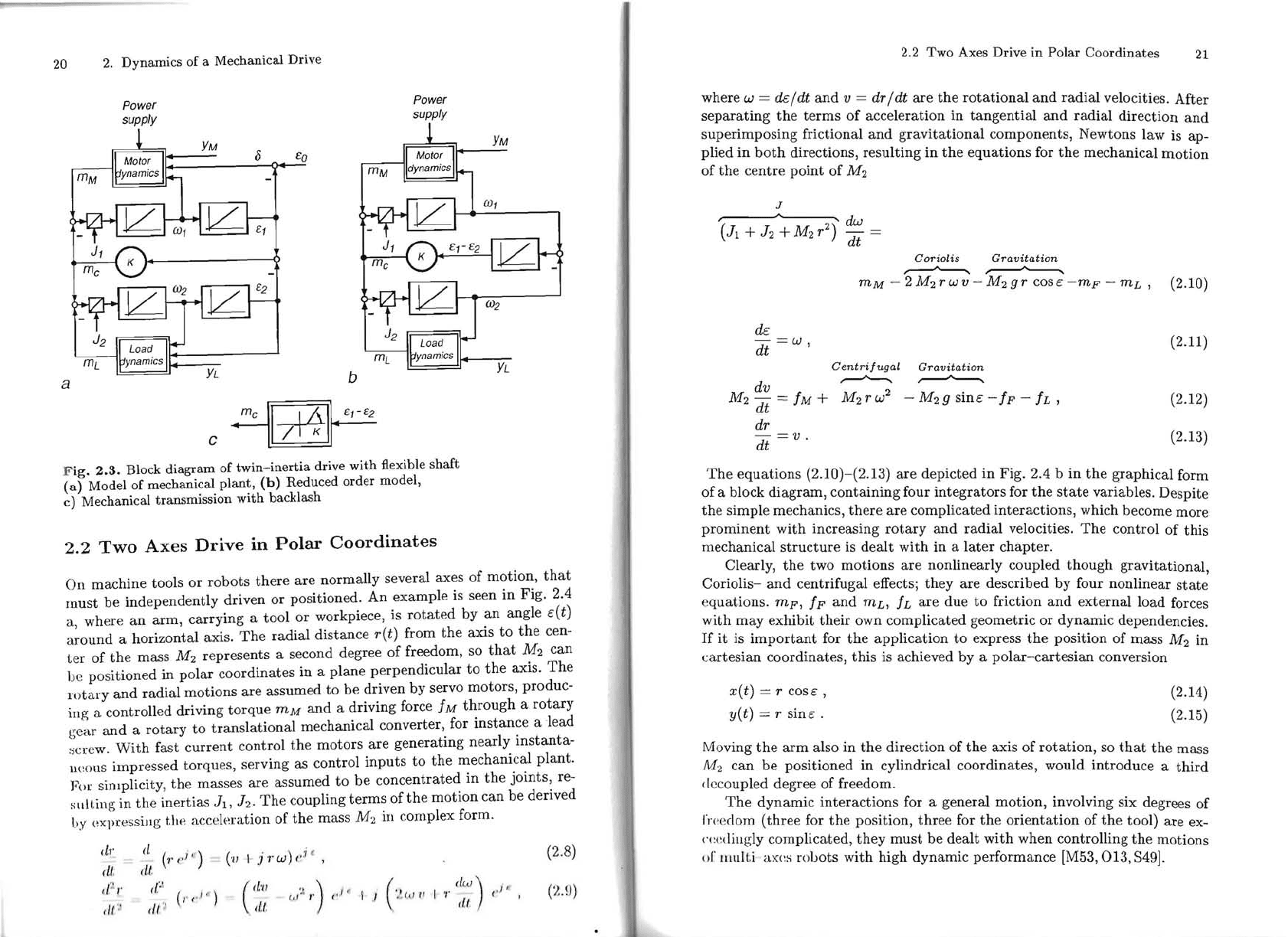
20
21
2.
Dynamics
of a Mechanical Drive
Power
Power
supply
supp/y
fO
b
a
c~JP
F
ig.
2.3.
Block
diagram
of
twin-inertia
drive
with
flexible
shaft
(a)
Model of mechanical
plant,
(b)
Reduced
order
model,
c)
Mechanical
transmission
with
backlash
2.2
Two
Axes
Drive
in
Polar
Coordinates
On
machine tools or robots
there
are
normally several axes of motion,
that
rnust be independently driven or positioned. An example is seen in Fig. 2.4
a, where
an
arm, carrying a tool or workpiece, is
rotated
by
an
angle e:(t)
around
a horizontal axis.
The
radial distance
r(t)
from
the
axis
to
the
cen-
ter
of
the
mass M
2
represents a second degree
of
freedom, so
that
M2 can
bc positioned in po
lar
coordinates in a plane perpendicular
to
the
axis.
The
rotary
and
radial motions
are
assumed
to
be
driven by servo motors, produc-
ing a controlled driving
torque
mM
and
a driving force
1M
through
a
rotary
gear
and
a
rotary
to
translational
mechanical converter, for instance a
lead
screw.
With
fast
current
control
the
motors are generating nearly
instanta-
\ICOIlS
impressed torques, serving as control
inputs
to
the
mechanical
plant
o
For simplicity,
the
masses are assumed
to
be
concentrated in
th.e
joints, re-
sltlt.ing in
the
inertias
h,
J
2
.
The
coupling
terms
of
the
motion can be derived
by
(~xpressillg
t,he
acc
e
h~ration
of
the
mass M 2 in complex
formo
dr
!l . )
(2.8)
(1'
( .
.1
'
('II
I
:i
r
w)
(
.3
~
,
dI
(li
,,
~
\
,(
J.
)'
(/L'II
I_,
.}
.
~
;I
,.
)
t'
I
(,
.
.\1 "
(
,
'~~
(LI
'
fi
I
'I'
Il
lU
)
('
j '
' I
(/
..
!J
)
. (
1<
i' )
dI
,
11
',
\
,II
~
,
d'
2.2
Two
Axes Drive
in
Polar
Coordinates
where w =
dE;
/ dt
and
v = dr/
dt
are
the
rotational
and
radial
veloci ties. After
separating
the
terms
of
acceleration in
tangential
and
radial direction
and
superimposing frictional
and
gravitational
components, Newtons law is ap-
plied in
both
directions, resulting in
the
equations for
the
mechanical
motion
of
the
centre
point of M
2
J
r,,-_~A
,
dw
(ll
+ h
+M2r2)
dt
=
Coriolis
Gravitation
,...-"'-...
,.-"'-----....
mM
-
2M
2
rwv-M
2
gr
cose:-mF
-mL,
(2
.10)
de:
dt
= w , (2.11)
C e
ntrifugai
Gravitation
dv
M
2
dt
= 1M +
,-"--..
M
2
r w
2
,...-"'-...
-
M
2
g sine: -
lF
-
h,
(2.12)
dr
dt
= v .
(2.13)
The
equations (2.10)-(2.13) are depicted in Fig. 2.4 b in
the
graphical form
of a block diagram, containing four integrators for
the
state
variables. Despite
the
simple mechanics,
there
are
complicated interactions, which become more
prominent with increasing
rotary
and
radial velocities.
The
control of this
mechanical
structure
is dealt
with
in a
later
chapter.
Clearly,
the
two motions
are
nonlinearly coupled
though
gravitational,
Coriolis-
and
centrifugaI effects; they are described by four nonlinear
state
equations.
mF,
lF
and
mL,
h are due
to
friction
and
external load forces
with
may
exhibit
their
own complicated geometric or dynamic dependencies,
If
it is
important
for
the
application
to
express
the
position of mass M
2
in
cartesian coordinates, this is achieved by a
polar-cartesian
conversion
x (t) = r cos
e:
,
(2.14)
y(t) = r sine: .
(2
.15)
Moving
the
arm
also in
the
direction of
the
axis
of
rotation,
so
that
the
mass
M
2
can be positioned in cylindrical coordinates, would introduce a
third
dccoupled degree of freedom.
The
dynamic
interactions for a general motion, involving six degrees of
r)'(
~e
dom
(three for
the
position,
three
for
the
orientation
of
the
tool) are ex-
('
cedilJgly complicated,
they
must
be dealt
with
when controlling
the
motions
01'
Jllult.i
a
x
(
~
s
ro
uots
with high dynamic performance [M53,
013,
849].

23
'22
2.
Dynamics
of a
Mechanical
Drive
mLosd
Friction
M
V
J
,
+J2+
M
2
r2
rw
2
grcos
E
gsinE
I
y/,
x
fLoad
b
Fig.
2.4.
Two
axes drive
in
polar
coordinates
(a)
Mechanical
plant
(b)
Block
diagram
2.3
Steady
State
Characteristics
of
Different
Types
of
Motors
and
Loads
Consider first
the
steady
state
condition, when
the
torque
and
speed of a
single axis
lumped
inertia
drive are
constant
and
the
angle changes linearly
with
timej this condition
is
reached when
mM
-
mL
=
O.
With
some motors,
such as single phase induction motors, or loads, for example piston compres-
sors or punches,
the
torque
is a periodic function of
the
angle of rotationj
in this case,
the
steady
state
condition
is
reached, when
the
mean
values of
both
torques are equal,
mM
-
mL
=
O.
The
speed
then
contains periodic
oscillations, which
must
be kept within limits by a sufficiently large inertia.
The
stcady
state
characteristics of a
motor
or
1030<1
are often functions
given
in
graphical [o
fi
II
, <:onnN:ting main
varíilhl(~K,
sllch as speed
and
torque;
lh('
provisíou
i:i
1.11;t\.
:lllxili<tJ"y
01'
COlltrol
íllPIlII
.H,
ror
('X;UllPlc
Sllpply voltage,
li(.ld
(·II!"l"('IIt.,
rll
'
illJ
~
f
lllJ
~ It',
hrw;h
pm;ll.ioll
01" r,·(·" ru
\.<',
;I],(~
lIlailll
,
;ú,\(~d
COIl-
Ht.iI.lIl..
I"
1'11\ '
).
,
1,
/1
,1
,
11,1""
1.,Ypi(,iI.I
ri
II
ll'
Jl
l'\.l'
I'l
ll
I.
Ic-
II
"I'
(,1(,(
'
1.1'1('
JlJoI,ol"X
11.1'('
:;
\iOWII.
'1'1". "
11
,
\1
",111
,,,,,,,11
11"
(h
ll
' I
,(
·
t."r1
r
ll.
iI'
li
l (
IId
,y vtdl" 1,"' ('t
lll::L
;
UIl
:;p(,,'cI, ::
11\('('
1.111'
Friction
r
a
2.3
Steady
State
Characteristics
of
Motors
and
Loads
variable is
the
load angle
0,
i.
e,
the
displacement of
the
shaft from
its
no-
load
angular
position.
When
the
maximum
torque is exceeded,
the
motor
falls
out
of step; asynchronous
operation
of
larger motors
is
not allowed for
extended periods of
time
because of
the
high currents
and
pulsating
torque,
The
electrical
transients
usually cannot be neglected
with
synchronous
motor
drives.
The
rigid speed of synchronous motors when supplied by a
constant
fre-
quency source makes
them
suitable for only a
few
applications, for exarnple
large slow-speed drives for reciprocating compressors or synchronous gener-
ators
operating
as motors in
pumped
storage hydro power stationsj
at
the
low end of
the
power scale are electromechanical clocks,
The
situation
is dif-
ferent, when
the
synchronous
motor
is
fed from a variable frequency inverter
because
then
the
speed of
the
drive can be varied freely (Chap. 14).
With
the
progress of power electronics, these drives are now more widely used.
mM
Fig.
2.5.
Steady
state
torque-speed
characteristics
of
(li) electrical
and
(b)
mechanical
drives
The
"asynchronous" or "shunt-typ" characteristic in Fig, 2.5 a
is
slightly
clrooping; often
there
is also a pronounced
maximum
torque.
The
lower por-
Lioll
of
the
curve
is
forbidden in steady
state
in view of
the
high losses.
With
I.hree- phase asynchronous
motors
the
rotor angle has no effect on
the
torque
i
Il
steady
state.
Motors
with
"series-type" characteristic show considerably larger speed
drop
under
loadj with DC or AC
commutator
motors,
this
is
achieved by
;1.
suitable connection of
the
field winding.
The
main
area
of
applications
at
larger
ratings
are
traction
drives because
the
curved characteristic resembling
;1
.
hyperbola
facilitates load sharing on multi pie drives
and
permits
nearly
collst.ant power
operation
over a wide speed range
without
gear changej this
is par\.icularly
suited
to
a Diesel-electric
or
turbo-
electric drive, where
the
1',,11
!)()Wl'r
o[
lhe
thermal
engine
must
be used.
For
('olllparisoll,
SOIlJC
t:ypi
cal charactcrist.ics
of
a Lurbine
anel
a Diesel
('I\/';ill('
ai
. ('ClIlHl.n,lll.
flld
illjc('.t.ioJl
pc
r H
t.I'III('
lU'"
i'
iC'('1\
iII l"il':.
:~.S
h.
- 2008 — 2025 «СтудМед»
