Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.


Appendix
G
The
Gelfand-Levitan-Marchenko
Equation
Let
us
consider the stationary Schrodinger equation (with
fi
=
1,
m
=
1/2),
on
the real line
R,
d2
-9
dx2
+
(k2
-
U(z))(P
=
0,
(G.1)
where the potential
U(x)
is
assumed to be a
fast-decreasing
function at
foo:
lim
U(z)
=
0.
X+fCQ
If
4(z,
Ic)
is
a
solution of Eq.
(G.l)
with the following asymptotic behavior:
4(z,
k)
Go0
exp[ikz]
(G.2)
then, by parity,
4(z,
-k)
is
a
solution of Eq.
(G.l)
whose asymptotic behavior
is given by
$(z,
-k)
,,”,
exp[-ikz]
.
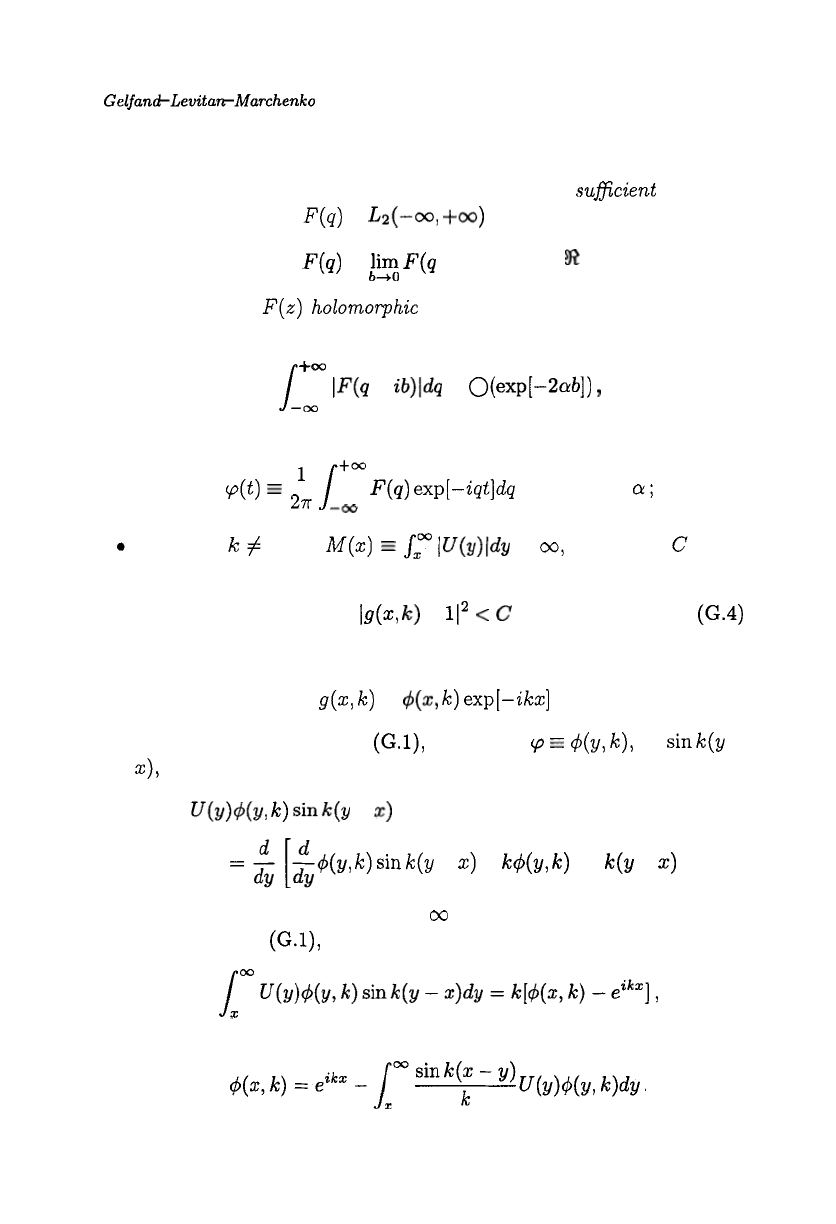
Moreover, it is not difficult to provel4?l6 that the solution
+(z,k)
can be
expressed in the following form:
To
this end let
us
observe that
414
The
Gelfan+LevitarrMarchenko
Equation
415
0
the following theorem holds:
Theorem
45
(Titchmarch)
A
necessary and suficient condition
for a real function
F(q)
E
Lz(-03,
+CO)
be the real limit
F(q)
=
6i
F(q
+
ib)
,
vq
E
9
of a function
F(t)
holomorphic
in
the upper complex plane
(b
>
0)
and satisfying the condition
+a
IF(q
+
ib)ldq
=
O(exp[-2abl)
9
L
2T
Jtm
-a
is
that
p(t)
-
F(q)
exp[-iqtldq
=
0,
Vt
<
a;
0
for
b
>
0,
k
#
0
and
M(x)
1,"
/U(y)(dy
<
03,
a
constant
C
exists
such that
ldx,
k)
-
112
<
c
,
(G.4)
where
g(x,
k)
=
r#(x,
k)
exp[-ikx] .
Indeed, by multiplying
Eq.
(G.l),
written for
p
3
#(y,
k),
by sink(y
-
x),
we obtain
U(Y)+(Y,
k)
sink-(y
-
k)
sink(y
-
x)
-
k+(y,
k)
cos
k(y
-
x)
,
1
which, integrated between
x
and
00
with the boundary condition ex
-
pressed by
Eq.
(G.l),
gives
or

416
The
Gelfan&~evata~~archenko Equation
The above equation, expressed
in
terms
of
g(z, k)
=
#(x, k) exp[--ikx],
reads
A
solution
of
the above equation
is
expressed by the expansion
dz,
k)
=
gn@, k)
1
nEN
with
-
U(Y)g"(Y, k)dy
,
go(%,
k)
=
1,
SnCl(X7
k)
=
f,
2ik
whose
uniform
convergence can
be
easily checked.
Indeed,
since
y
3
x
and
k
=
q
+
ib
with
b
>
0,
we
have
m
exp'-2ik'z
-
so
that, with ~(x}
=
f,"
~U~Y)~~Y,
Moreover,
so
that
from
the
induction
hypothesis
we have
Thus,

The
Ceuand-Levitan-Marhenko Equation
417
It follows that, for
b
>
0,
the function
h(z,
k)
=
#(s,
k)
-
expfikz]
=
exp[ikz][g(s,
k)
-
11
,
k
=
q
+
ib
)
is
square integrable with respect
p
E
8,
since
Ih(z,
k)l
=
I
eXP[ik4fs(z*
k)
-
111
=
exp
[--b~]
19
(z,
k)
-
11
,
so
that
lh(z,
k)I2
=
exp[-2bz]Ig(e,
k)
-
112
<
~exp[-2bz]
o(
o(exp[-2bz]).
Therefore,
+m
I&,
W124
=
O(eXP[-2W)
9
L
2n
s'"
--M
and we can apply Titchmarch' theorem, to write
A(z,y)
-
h(z,
k)
exp[-iqyjdq
=
0,
Vy
<
z
,
whose inversion, for y
>
z,
gives
h(z,
k)
=
I+"
A(z,
y)
exp[iky]dy
,
y
>
z
.
F'rom
the expression
of
h(z,
k)
and the above equation, we have Eq.
(G.3),
Thus, it has been shown that the solution
+(z,
k)
=
exp[ikz]
+
A@,
y) exp[iky]dy
(G.5)
I"
is analytic in the complex open upper plane defined by
Qmk
>
0.
Moreover,
every solution of
(G.l)
can be expressed
as
a linear combination of
#(z,
k)
and
#(z,
-k),
the last being linearly independent.
Therefore, two solutions
$(z,
k)
and
$(s,
-k)
of
(G.l),
having the following
asymptotic behavior:
N
+(t,
k)
I-+--oo
exPI-ik.zl
Y
-k)
a+--M
exp[W
,
N

418
The
Gelfanct-LevitawMarchenko
Equation
The coefficients
a(k),
P(k),
d(k)
and
p(k)
can be easily expressed in terms of
the functions
$(x,
k)
and
$(x,
k).
Indeed, by using
Eq.
(G.6),
the Wronskian
W
of
4(x7
k)
and
$(x,
k),
d d
W[4(x7
k),
$(x,
k)l
=
+(XI
k)-&(X’k)
-
$(x,
k)Z4(Zj
k)
>
is related to the Wronskian
W
of
4(x,
k)
and
+(x,
-k)
by
W[4(.,
k),
$(x,
k>l
=
a(~)W[4(x,
k),
4(x,
411
*
Since the Wronskian of two solutions of the Schrodinger equation does not
depend on
x,
we have
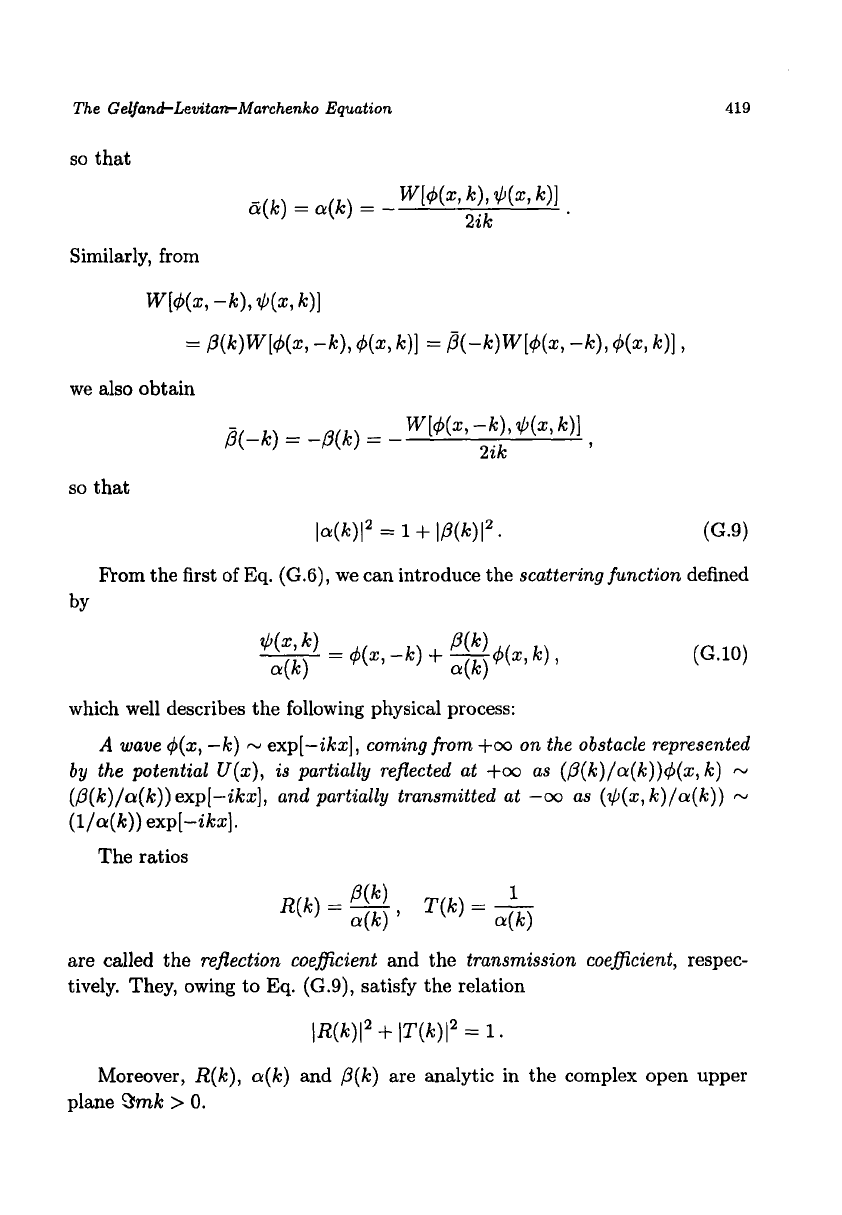
The
Gelfan&Levitan-Marchenko
Equation
419
so
that
Wr6(z,
k),
442,
k>l
b(k)
=
a(k)
=
-
2ik
Similarly, from
we also obtain
so
that
14V12
=
1
+
IP(W
*
(G.9)
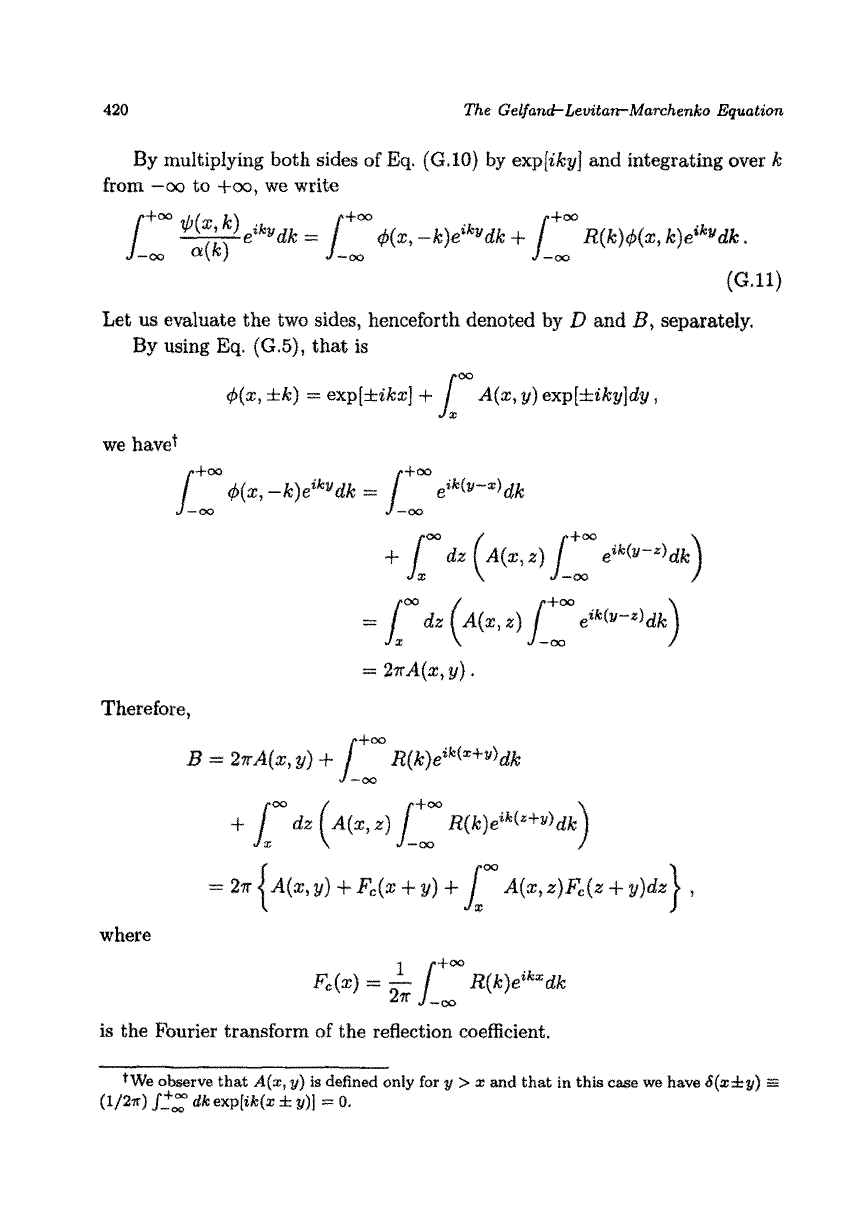
F’rom
the first
of
Eq.
(G.6),
we can introduce the
scattering function
defined
by
(G.lO)
which well describes the following physical process:
A
wave
+(z,
-k)
N
exp[-ikz],
coming from
+oo
on the obstacle represented
by
the potential
U(z),
is partially reflected at
+oo
as (P(k)/a(k))$(z,k)
N
(P(k)/a(k))
exp[-ikz],
and partially transmitted at
--oo
as
($(q
k)/a(k))
-
(l/a(k))
exp[-ikz].
The ratios
are called the
reflection coeficient
and
the
transmission coeficient,
respec-
tively. They, owing to
Eq.
(G.9),
satisfy the relation
IR(k)I2
+
IT(k)I2
=
1
Moreover,
R(k),
a(k)
and
P(k)
are analytic in the complex open upper
plane
Smk
>
0.
420
The
Gelfan&Levitan-Marchenko
Equation
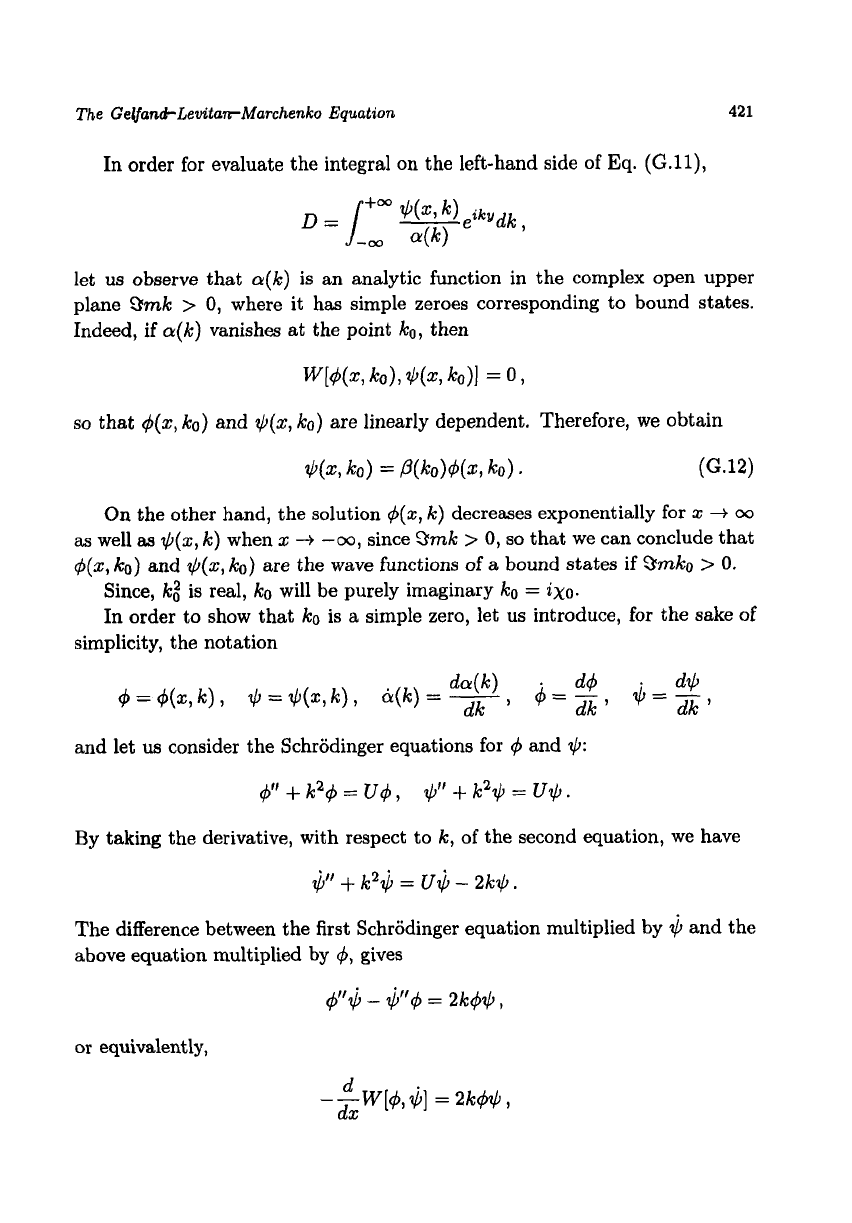
By
muItip~ying
both
sides
of
Eq.
(G.10)
by
exp[iky]
and integrating over
k
from
-m
to
$00,
we
write
(G.11)
Therefore,
where
Fc(x)
=
2;;
1
L,
+O0
~~k)e~~x~k
is
the Fourier transform of the refiection coefficient.
+We
observe
that
A(x,
g)
is
defined
only
for
y
>
x
and
that
in
this
case
we
have
6(zfgf
z
fi/zz)
J_*,-
ctkexp[iB(z
rt
y)j
=
0.
The
Gelfond-Levitan-Marchenko
Equation
421
In order for evaluate the integral on the left-hand side of Eq.
(G.ll),
let
us
observe that
a(k)
is an analytic function in the complex open upper
plane
Smk
>
0,
where it has simple zeroes corresponding to bound states.
Indeed,
if
a(k)
vanishes at the point
ko,
then
W[4(.,
ko), $(x,
w1
=
0
1
so
that
+(x, ko)
and
$(z,
Ico)
are linearly dependent. Therefore, we obtain
$(x, ko)
=
P(ko)d(z, ko)
'
(G.12)
On
the other hand, the solution
4(z,
k)
decreases exponentially
for
z
00
as
well
w
$(z,
k)
when
2
-+
-00,
since
Smk
>
0,
so
that we can conclude that
4(x,
Icg)
and
$(z,
ko)
are the wave functions of
a
bound states
if
Smko
>
0.
Since,
k:
is real,
ko
will be purely imaginary
ko
=
ixo.
In order to show that
ko
is
a simple zero, let us introduce, for the sake
of
simplicity, the notation
and let
us
consider the Schrodinger equations for
4
and
$:
4"
+
k2$
=
U4,
$'I
+
k2$
=
U$
.
By taking the derivative, with respect to
k,
of
the second equation, we have
8"
+
k2d
=
Ud
-
2k$.
The difference between the first Schrodinger equation multiplied by
11
and the
above equation multiplied by
4,
gives
-
=
2k4$,
or equivalently,

The
Gelfan&LevituPrMarchenlco
Equation
422
so
that
where
1
is an arbitrary parameter.
With the same procedure we also have
(G.13)
(G.14)
On
the other hand, by using
Eq,
(G.81,
we
can write
((2.15)
d
-(2ika)
=
2Za(k)
+
Zik&{k)
=
-W[$,
@]
-
W[#,
41.
dk
Let
us
now observe that
0
the above equation does not depend
on
x;
e
for
k
=
ko,
both
4s
and
$
vanish;
0
both
W[$,$]
and
W[#,q]
vanish
at
z
=
il
-+
fm.
Therefore, in such limits, by adding
Eqs.
(G.13)
and
(G.14),
Eq,
(G.15)
gives
+a
+m
#(x,
kaFa)$(s, ko)ds
=
2koP(ko)
f
4s2(s,
k.o)dg
#
0,
L
-m
flZikoCy(k0)
=
2k.O
(G.16)
where
Eq.
(G.12)
has been used.
The above equation shows that
ka
is a simple zero
of
a(k).
Let
us
continue the calculation of the integral
D,
by applying the
~sz~~es
~e~~o~
according to which
7t
for
every domain
d’D
completely belonging
to
the
field
of
the analyticity
of
the
function
#
and
containing
it
finite number
of
singular isolated points
Zk.
For
our
purpose,
let
us
choose the half-circle, with an infinite radius, con-
tained in the upper plane,
csink
>
0.
For an infinite radius
of
the ha~f-circle, the factors
of
the type exp~~k.~~~~)~,
with
y
>
z,
give rise to
a
vanishing contribution from the integral
along
the
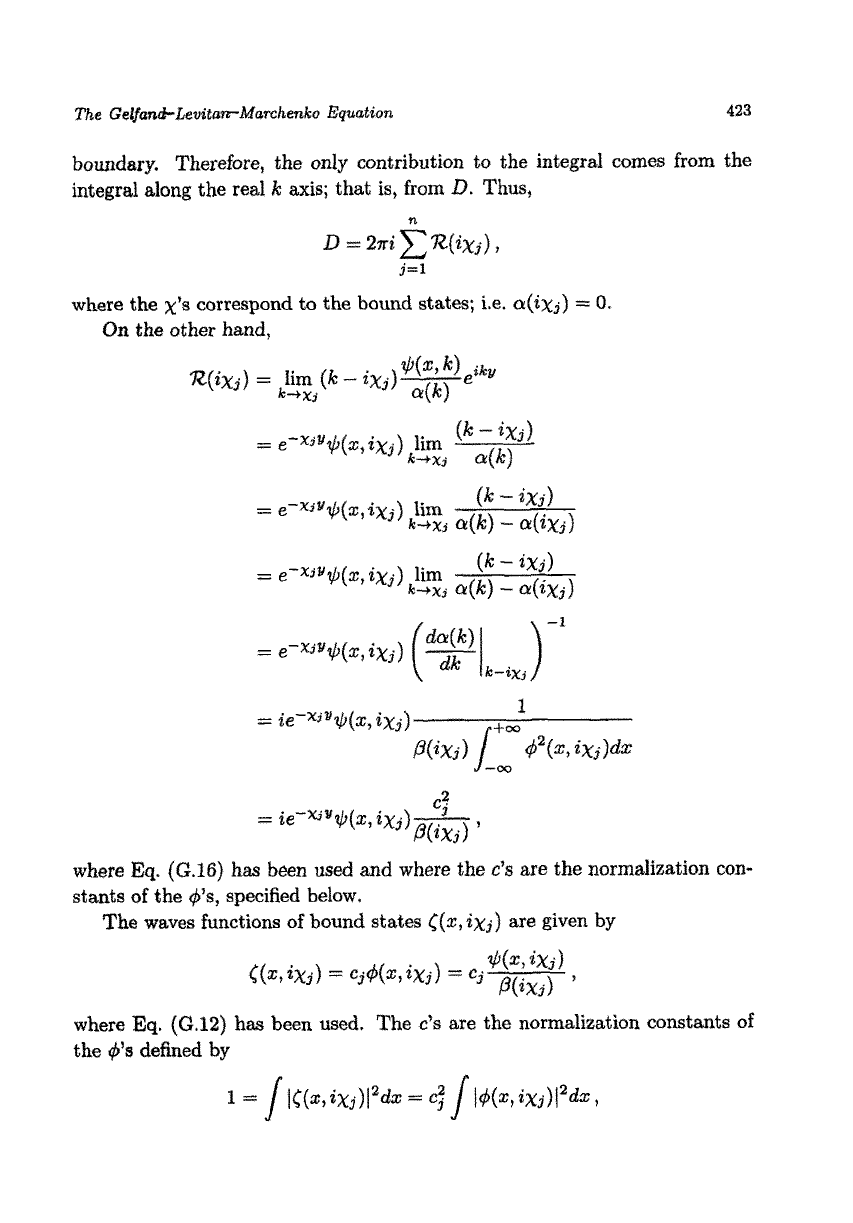
bo~d~ry, Therefore3 the
only
con~r~b~~~on
to
the integral comes
from
the
integral dong the real
k
axis;
that
is,
from
D.
Thus,
n
j=l
where
the
x’s
correspond
to
the bound states;
i.e.
a(ixj)
=
0.
On
the other hand,
where
Eq.
(G.16)
has
been used
and
where
the
c’s
are the normalization
con-
stants
of
the
#s,
spec~fi~d below,
The waves functions
of
bound states
((z,
ixj)
are given by
where
Eq.
(6.12)
has been used. The c’s are the normalization constants of
the
4’s
defined by
