Taylor M.E. Partial Differential Equations III: Nonlinear Equations
Подождите немного. Документ загружается.


68 13. Function Space and Operator Theory for Nonlinear Analysis
Most of the applications of the material of this section made in the following
chapters of this book will involve symbol smoothing, (10.60)–(10.61), with ı<1.
However, we will establish some basic results on operator calculus for symbols of
the form (10.64).
We will analyze products a.x; D/b.x; D/ D p.x; D/ when we are given
a.x; / 2 S
1;1
.R
n
/ and b.x;/ 2 BS
m
1;1
.R
n
/. We are particularly interested in
estimating the remainder r
.x; /,arisingin
(10.65) a.x; D/b.x; D/ D p
.x; D/ C r
.x; D/;
where
(10.66) p
.x; / D
X
j˛j
i
j˛j
˛Š
@
˛
a.x; / @
˛
x
b.x; /:
Proposition 10.8 below is a variant of results of [Bon]and[Mey], established
in [AT].
To begin the analysis, we have the formula
(10.67) r
.x; / D
1
.2/
n
Z
h
a.x; C/
X
j˛j
˛
˛Š
@
˛
a.x; /
i
e
ix
O
b.; / d:
Write
(10.68) r
.x; / D
X
j 0
r
j
.x; /;
with
r
j
.x; / D
Z
b
A
j
.x;;/
b
B
j
.x;;/d
D
Z
A
j
.x;;y/B
j
.x; ; y/ dy;(10.69)
where the terms in these integrands are defined as follows. Pick #>1,andtakea
Littlewood–Paley partition of unity f'
2
j
W j 0g, such that '
0
./ is supported in
jj1, while for j 1; '
j
./ is supported in #
j 1
jj#
j C1
.Thenweset
(10.70)
b
A
j
.x;;/ D
1
.2/
n
h
a.x; C /
X
j˛j
˛
˛Š
@
˛
a.x; /
i
'
j
./;
b
B
j
.x;;/ D
O
b.; /'
j
./e
ix
:
Note that
(10.71) B
j
.x;;y/ D '
j
.D
y
/b.x C y; /:

10. Paradifferential operators 69
Thus
(10.72) kB
j
.x; ; /k
L
1
C#
rj
kb.;/k
C
r
:
Also,
(10.73) supp
O
b.; / fjj <jjg H) B
j
.x;;y/ D 0; for #
j 1
jj:
We next estimate the L
1
-norm of A
j
.x; ; /. Now, by a standard proof of
Sobolev’s imbedding theorem, given K>n=2,wehave
(10.74) kA
j
.x; ; /k
L
1
C k
j
b
A
j
.x; ; /k
H
K
;
where
j
f./ D f.#
j
/,so
j
b
A
j
is supported in jj#. Let us use the
integral formula for the remainder term in the power-series expansion to write
(10.75)
b
A
j
.x;;#
j
/ D
'
j
.#
j
/
.2/
n
X
j˛jDC1
C1
˛Š
Z
1
0
.1 s/
C1
@
˛
a.x; C s#
j
/ ds
#
j j˛j
˛
:
Since jj# on the support of
j
b
A
j
,ifalso#
j 1
<jj,thenj#
j
j <
#
2
jj.Now,given 2 .0; 1/, choose #>1such that #
3
<1. This implies
hih C s#
j
i,foralls 2 Œ0; 1. We deduce that the hypothesis
(10.76) j@
˛
a.x; /jC
˛
hi
2
j˛j
; for j˛j C 1;
implies
(10.77) kA
j
.x; ; /k
L
1
C
#
j.C1/
hi
2
1
; for #
j 1
<jj:
Now, when (10.72)and(10.77) hold, we have
(10.78) jr
j
.x; /jC
#
j.C1r/
hi
2
1
kb.;/k
C
r
;
and if (10.73) also applies, we have
(10.79) jr
.x; /jC
hi
2
r
kb.;/k
C
r
if C 1>r;
since
X
#
j 1
<jj
#
j.C1r/
C jj
C1r
in such a case.
70 13. Function Space and Operator Theory for Nonlinear Analysis
To estimate derivatives of r
.x; /, we can write
D
ˇ
x
D
r
j
.x; / D
(10.80)
X
ˇ
1
Cˇ
2
Dˇ
X
1
C
2
D
ˇ
ˇ
1
!
1
!
Z
D
ˇ
1
x
D
1
A
j
.x;;y/ D
ˇ
2
x
D
2
B
j
.x; ; y/ dy:
Now D
ˇ
1
x
D
1
A
j
.x;;y/ is produced just like A
j
.x;;y/, with the symbol
a.x; / replaced by D
ˇ
1
x
D
1
a.x; /,andD
ˇ
2
x
D
2
B
j
.x; ; y/ is produced
just like B
j
.x; ; y/, with b.x; / replaced by D
ˇ
2
x
D
2
b.x; /. Thus, if we
strengthen the hypothesis (10.76)to
(10.81) j@
ˇ
x
@
˛
a.x; /jC
˛ˇ
hi
2
j˛jCjˇj
; for j˛j C 1;
we have
(10.82) kD
ˇ
1
x
D
1
A
j
.x; ; /k
L
1
C
#
j.C1/
hi
2
j
1
jCjˇ
1
j1
;
for #
j 1
<jj. Furthermore, extending (10.72), we have
(10.83) kD
ˇ
2
x
D
2
B
j
.x; ; /k
L
1
C#
.jˇ
2
jr/j
kD
2
b.;/k
C
r
:
Now
(10.84)
X
#
j 1
<jj
#
j.C1Cjˇ
2
jr/
C jj
C1Cjˇ
2
jr
if C 1>r, so as long as (10.73) applies, (10.82)and(10.83) yield
(10.85) jD
ˇ
x
D
r
.x; /jC
X
1
C
2
D
hi
2
Cjˇjj
1
jr
kD
2
b.;/k
C
r
if C 1>r. These estimates lead to the following result:
Proposition 10.8. Assume
(10.86) a.x; / 2 S
1;1
;b.x;/2 BS
m
1;1
:
Then
(10.87) a.x; D/b.x; D/ D p.x; D/ 2 OP S
Cm
1;1
:
Assume furthermore that
(10.88) j@
ˇ
x
@
˛
a.x; /jC
˛ˇ
hi
2
j˛jCjˇj
; for j˛j C 1;

10. Paradifferential operators 71
with
2
, and that
(10.89) kD
˛
b.;/k
C
r
C
˛
hi
m
2
j˛j
:
Then, if C 1>r, we have (10.65)–(10.66), with
(10.90) r
.x; D/ 2 OP S
2
Cm
2
r
1;1
:
The following is a commonly encountered special case of Proposition 10.8.
Corollary 10.9. In Proposition 10.8, replace the hypothesis (10.89)by
(10.91) D
ˇ
x
b.x; / 2 S
m
2
1;1
; for jˇjDK;
where K 2f1; 2; 3; : : : g is given. Then we have (10.65)–(10.66), with
(10.92) r
.x; / 2 OP S
2
Cm
2
K
1;1
if K:
Proof. The hypothesis (10.91) implies (10.89), with r D K.
We can also deduce from Proposition 10.8 that a.x; D/b.x; D/ has a complete
asymptotic expansion if b.x;/ is a symbol of type .1; ı/ with ı<1.
Corollary 10.10. If 0 ı<1and
(10.93) a.x; / 2 S
1;1
;b.x;/2 S
m
1;ı
;
then a.x; D/b.x; D/ 2 OP S
Cm
1;1
, and we have (10.65)–(10.66), with
(10.94) r
.x; D/ 2 OP S
Cm.1ı/
1;1
:
Proof. Altering b.x; / by an element of S
1
1;0
, one can arrange that the condition
(10.73) on supp
O
b.; / hold. Then, apply Corollary 10.9, with m
2
D m CKı,so
m
2
K D m K.1 ı/,andtakeK D .
Note that, under the hypotheses of Corollary 10.10,
(10.95)
X
j˛jD
1
˛Š
@
˛
a.x; / @
˛
x
b.x; / 2 S
Cm.1ı/
1;1
;
so we actually have
(10.96) r
1
.x; D/ 2 OP S
Cm.1ı/
1;1
:
The family [
m
OP BS
m
1;1
does not form an algebra, but the following result is
a useful substitute:

72 13. Function Space and Operator Theory for Nonlinear Analysis
Proposition 10.11. If p
j
.x; / 2 B
j
S
m
j
1;1
and D
1
C
2
C
1
2
<1,then
p
1
.x; /p
2
.x; / 2 B
S
m
1
Cm
2
1;1
;
p
1
.x; D/p
2
.x; D/ 2 OP B
S
m
1
Cm
2
1;1
:
(10.97)
Proof. The result for the symbol product is obvious; in fact, one can replace
by
1
C
2
.AsforA.x; D/ D p
1
.x; D/p
2
.x; D/, we already have from
Proposition 10.8 that A.x; / 2 S
m
1
Cm
2
1;1
; we merely need to check the support of
b
A.; /. We can do this using the formula
(10.98)
b
A.; / D
Z
Op
1
. ; C / Op
2
.; / d :
Note that given .; /, if there exists 2 R
n
such that Op
1
. ; C / ¤ 0 and
Op
2
.; / ¤ 0,then
j j
1
j C j; jj
2
jj;
so
jj
1
j C jCjj
1
jjC
1
jjC
2
jj.
1
C
2
C
1
2
/jj:
This completes the proof.
Exercises
1. Prove the commutator property:
(10.99) ŒOPS
;OPA
r
0
S
m
1;ı
OPS
mCr
1;ı
;0 r<1;0 ı<1:
2. Prove that, for 0 ı<1,
(10.100) P 2 OP A
r
0
S
m
1;ı
H) P
2 OP A
r
0
S
m
1;ı
:
(Hint:UseP.x;D/
D P
.x; D/, with P
.x; /
P
D
˛
x
D
˛
p.x;/. Show that
p.x; / 2 A
r
0
S
m
1;ı
H) D
˛
x
D
˛
p.x; / 2 A
r
0
S
m.1ı/j˛j
1;ı
:/
3. Show that
(10.101)
X
j˛jm
a
˛
.x; D
m1
u/D
˛
u D M.uIx;D/u C R;
where R 2 C
1
and, for 0<r<1,
(10.102) u 2 C
m1Cr
H) M.uIx;/ 2 A
r
0
S
m
1;1
C S
mr
1;1
:
Exercises 73
Deduce that you can write
(10.103) M.uIx; / D M
#
.x; / C M
b
.x; /;
with
(10.104) M
#
.x; / 2 A
r
0
S
m
1;ı
;M
b
.x; / 2 S
mrı
1;1
:
Note that the hypothesis on u is weaker than in Proposition 10.7.
4. The estimate (10.9) follows from the formula
D
˛
g.h/ D
X
˛
1
CC˛
D˛
C.˛
1
;:::;˛
/h
.˛
1
/
h
.˛
/
g
./
.h/;
which is a consequence of the chain rule. Show that the following Moser-type estimate
holds:
(10.105) kD
`
g.h/k
L
1
C
X
1`
kg
0
k
C
1
khk
1
L
1
kD
`
hk
L
1
:
5. The paraproduct of J.-M. Bony [Bon] is defined by applying symbol smoothing to the
multiplication operator, M
f
u D f u.Onetakes
(10.106) T
f
u D
X
k
‰
k5
.D/f
k
.D/u;
where, as in (10.62), ‰
`
./ D
P
j `
j
./. Show that, with T
f
D F.x;D/,
(10.107) f 2 L
1
.R
n
/ H) F.x;/ 2 S
0
1;1
.R
n
/:
Show that, for any r 2 R,
(10.108)
f 2 C
r
.R
n
/ H) jD
ˇ
x
D
˛
F.x;/jC
˛ˇ
kf k
C
r
hi
rj˛jCjˇ j
; for j˛j1:
6. Using Propositions 10.8–10.11, show that if p.x;/ 2 B
1=2
S
m
1;1
,then
(10.109) f 2 C
0
H) ŒT
f
;p.x;D/2 OP BS
m
1;1
:
Applications of this are given in [AT].
7. Show that p.x; / 2 BS
m
1;1
implies p.x; D/
2 OP S
m
1;1
, and, if is sufficiently small,
(10.110) p.x; / 2 B
S
m
1;1
H) p.x; D/
2 OP BS
m
1;1
:
8. Investigate properties of operators with symbols in
(10.111) B
r
S
m
1;1
D BS
m
1;1
\ A
r
0
S
m
1;1
:
74 13. Function Space and Operator Theory for Nonlinear Analysis
11. Young measures and fuzzy functions
Limits in the weak
topology of sequences f
j
2 L
p
./ are often not well be-
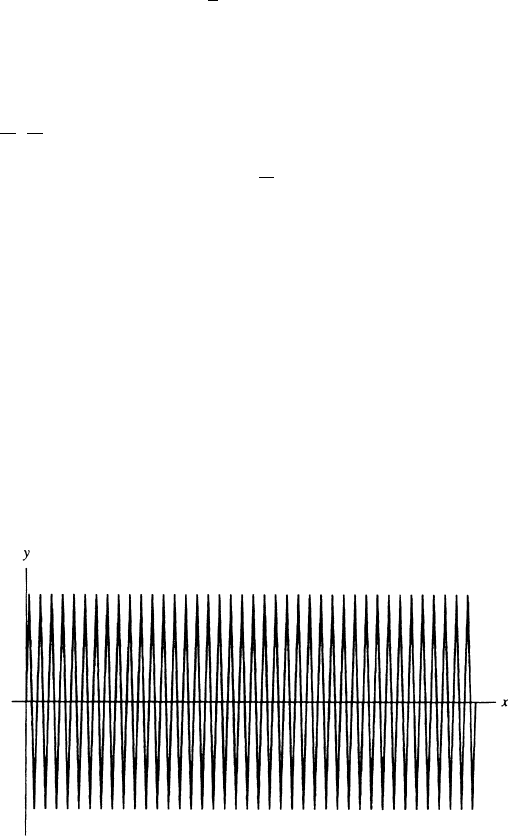
haved under the pointwise application of nonlinear functions. For example,
(11.1) sin nx ! 0 weak
in L
1
Œ0;
;
while
(11.2) sin
2
nx !
1
2
weak
in L
1
Œ0;
(see Fig. 11.1). A fuzzy function is endowed with an extra piece of structure,
allowing for convergence under nonlinear mappings.
Assume is an open set in R
n
.Given1 p 1, we define an element of
Y
p
./ to be a pair .f; /,wheref 2 L
p
./ and is a positive Borel measure
on
R (R D Œ1; 1), having the properties
(11.3) y 2 L
p
R;d.x;y/
;
(so, in particular, f˙1ghas measure zero),
(11.4) .E R/ D L
n
.E/;
for Borel sets E ,whereL
n
is Lebesgue measure on ,and
(11.5)
“
ER
yd.x;y/D
Z
E
f.x/ dx;
for each Borel set E . We can equivalently state (11.4)and(11.5)as
(11.6)
“
'.x/ d.x; y/ D
Z
'.x/ dx
FIGURE 11.1 Approaching a Fuzzy Limit

11. Young measures and fuzzy functions 75
and
(11.7)
“
'.x/y d.x; y/ D
Z
'.x/f.x/ dx;
for ' 2 C
0
./, that is, for continuous and compactly supported '.
Note that (11.5) implies
(11.8)
Z
E
jf.x/j dx
“
ER
jyj d.x; y/;
since we can write E D E
1
[ E
2
with f 0 on E
1
and f<0on E
2
.Ifwe
partition E into tiny sets, on each of which f is nearly constant, we obtain
(11.9)
Z
E
jf.x/j
p
dx
“
ER
jyj
p
d.x; y/:
We say that .f; / is a fuzzy function, and is a Young measure, representing f .
A special case of such is
f
,definedby
(11.10)
“
.x; y/ d
f
.x; y/ D
Z
x; f.x/
dx;
for 2 C
0
. R/.Wesay.f;
f
/ is sharply defined.
Fuzzy functions arise as limits of sharply defined functions in the following
sense. Suppose f
j
2 L
p
./; 1 < p 1,and.f; / 2 Y
p
./.Wesay
(11.11) f
j
! .f; / in Y
p
./;
provided
(11.12) f
j
! f weak
in L
p
./
and
(11.13)
f
j
! weak
in M. R/;
and furthermore,
(11.14) kyk
L
p
.R;d
f
j
/
C<1:
Actually, (11.12) is a consequence of (11.13)and(11.14), thanks to (11.9).
76 13. Function Space and Operator Theory for Nonlinear Analysis
To take an example, if D .0; / and f
n
.x/ D sin nx,asin(11.1), it is easily
seen that
(11.15) f
n
! .0;
0
/ in Y
1
./;
where
(11.16) d
0
.x; y/ D
Œ1;1
.y/
2dxdy
p
1 y
2
:
Also,
(11.17) f
2
n
!
1
2
;
1
in Y
1
./;
where
(11.18) d
1
.x; y/ D
Œ0;1
.y/
2dxdy
p
y.y 1/
:
The following result illustrates the use of Y
p
./ in controlling the behavior of
nonlinear maps. We make rather restrictive hypotheses for this first result, to keep
the argument short and reveal its basic simplicity.
Proposition 11.1. Let ˆ W R ! R be continuous. If f
j
! .f; / in Y
1
./,
then
(11.19) ˆ.f
j
/ ! g weak
in L
1
./;
where g 2 L
1
./ is specified by
(11.20)
Z
g.x/'.x/ dx D
“
ˆ.y/'.x/ d.x; y/; ' 2 C
0
./:
Proof. We need to check the behavior of
R
ˆ.f
j
/' dx.Sinceˆ.f
j
/ is bounded
in L
1
./, it suffices to take ' in C
0
./, which is dense in L
1
./.LetI be a
compact interval in .1; 1/, containing the range of each function ˆ.f
j
/.Now,
for any ' 2 C
0
./,
Z
ˆ.f
j
/' dx D
“
I
'.x/ˆ.y/ d
f
j
.x; y/
!
“
I
'.x/ˆ.y/ d.x; y/;
(11.21)
since
f
j
! weak
in M. I/. This proves the proposition.

11. Young measures and fuzzy functions 77
Under the hypotheses of Proposition 11.1, we see that, more precisely than
(11.19),
(11.22) ˆ.f
j
/ ! .g; / in Y
1
./;
where g isgivenby(11.20)and is specified by
(11.23)
“
.x; y/ d.x; y/ D
“
x; ˆ.y/
d.x; y/; 2 C
0
. R/:
Thus is the natural image of under the map
e
ˆ.x; y/ D
x; ˆ.y/
of I !
R. One often writes D
e
ˆ
. The extra information carried by (11.22)is
that
ˆ.f
j
/
! ; weak
in M. R/, which follows from
“
.x; y/ d
ˆ.f
j
/
.x; y/ D
“
x; ˆ.y/
d
f
j
.x; y/
!
“
x; ˆ.y/
d.x; y/:(11.24)
We can extend Proposition 11.1 and its refinement (11.22)to
(11.25) f
j
! .f; / in Y
p
./ H) ˆ.f
j
/ ! .g; / in Y
q
./;
with 1<p;q<1,whereg and are given by the same formulas as above,
provided that ˆ W R ! R is continuous and satisfies
(11.26) jˆ.y/jC jyj
p=q
:
We need this only for large jyj if has finite measure.
This result suggests defining the action of ˆ on a fuzzy function .f; / by
(11.27) ˆ.f; / D .g; /;
where g and are given by the formulas (11.20)and(11.23). Thus (11.22) can be
restated as
(11.28) f
j
! .f; / in Y
1
./ H) ˆ.f
j
/ ! ˆ.f; / in Y
1
./:
It is now natural to extend the notion of convergence f
j
! .f; / in Y
p
./ to
.f
j
;
j
/ ! .f; / in Y
p
./, provided all these objects belong to Y
p
./ and we
have, parallel to (11.12)–(11.14),
f
j
! f weak
in L
p
./;(11.29)
j
! weak
in M. R/;(11.30)
