Tabak J. Mathematics and the Laws of Nature: Developing the Language of Science

Подождите немного. Документ загружается.

140 MATHEMATICS AND THE LAWS OF NATURE

Kelvin’s version worth considering is that he began from a dif-

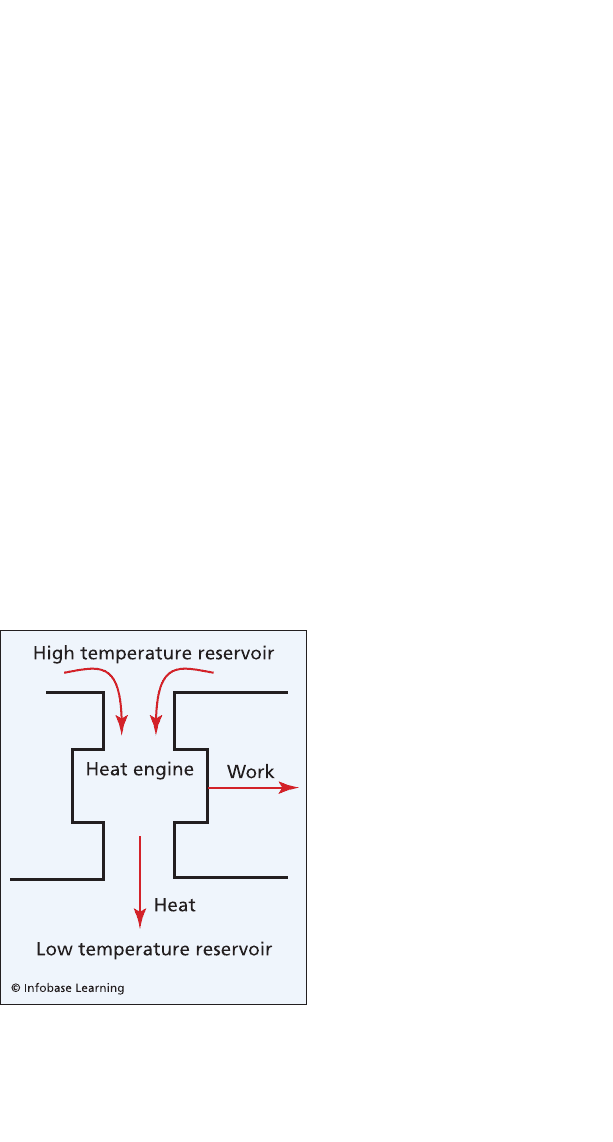
ferent point of view. In his article “Account of Carnot’s Theory

of the Motive Power of Heat,” Kelvin imagined two bodies, a

warm one and a cool one, and a heat engine placed between the

two bodies. The engine can harness the flow of heat to do work.

Alternatively he supposed that the two bodies are simply placed in

contact with each other with no engine between them. Heat then

flows directly from the warm body to the cool one. In the absence

of phase changes the warm body cools and the temperature of the

cool body increases. But what, Kelvin asked, happens to the work

that could have been produced by the heat engine?

Kelvin’s answer is that the work is lost. It cannot be recovered.

When heat dissipates—when it flows between two thermal reser-

voirs and evens out their temperatures—the work that could have

been produced by those two reservoirs is lost forever. What Kelvin

recognized is that heat dissipation is inevitable. It often occurs

slowly, but it goes on continuously. In his book Carnot emphasized

the motive power of heat. Heat drives all the processes on which life

depends, but heat flows only when there are temperature differenc-

es. These temperature differ-

ences are slowly, continually,

and inexorably disappearing.

As the temperature differences

disappear, so does the motive

power of heat. Everything that

depends on the transmission of

heat begins to slow and even-

tually everything stops. This

occurs not because energy dis-

appears; energy cannot disap-

pear. It is conserved. Instead

energy becomes unavailable to

do work. This peculiar state

of affairs, in which all ther-

mal processes cease, is heat

death. It is the final state of the

universe. The subject of heat

death has inspired many inter-

esting philosophical papers.

When the engine is not present, the

work that might have been accom-

plished by the heat that flowed from

the higher- to the lower-temperature

reservoir is irrevocably lost.

Mathematics and the Laws of Thermodynamics 141

entropy

Clausius’s first paper on thermodynamics was just the beginning. He

was interested in formulating a mathematical statement of the second

law, and to this end he defined the concept of entropy. Entropy is a

mathematical function with a physical interpretation. It conveys infor-

mation about the amount of energy that can be transferred between

thermodynamic systems in the form of work. It is through the concept of

entropy that Clausius was able to express his ideas about thermodynam-

ics in mathematical language.

For a particular system the value of the entropy function can range

from 0 to a maximal value. The smaller the value of the entropy, the more

of the system’s energy that can be converted to work. A large value for

the entropy means that very little of the system’s energy can be trans-

formed into work. The larger the value of the entropy function, the more

evenly dispersed is the energy of the system.

What is often of interest to scientists, however, is not so much the entro-

py of the system as the change of entropy. Imagine a system consisting

of a heat engine operating between a high-temperature thermal reservoir

and a low-temperature thermal reservoir. Practically speaking, no system

can be completely isolated from its surroundings, so we can expect some

heat to flow between our system and the environment. Suppose that we

compute the change of entropy of the environment caused by the opera-

tion of the engine. We can, of course, also compute the change of entropy

that occurred in our not-quite-isolated system. Finally, we add the two

entropy changes to get the change in the total entropy. When we do this

we get a very famous inequality, which we can write like this:

(Change in total entropy) = (change in entropy of environment)

+ (change in system entropy) ≥ 0

This inequality actually applies to all processes. In other words no matter

what we do, no matter what processes we consider, the total entropy

never decreases. Because entropy is a measure of the energy that can

be converted into work—the greater the entropy, the less energy we can

convert to work—this statement is a mathematical version of Kelvin’s

ruminations about lost work. In most natural processes the change in the

total entropy is positive. As a consequence the longer the universe “runs

on,” the less energy is available to do work. Clausius also formulated his

version of the second law so that it does not depend on the concept of a

refrigerator. Here is a commonly quoted version of his more philosophical

statement of the second law:

The entropy of the universe tends toward its maximal value.

142 MATHEMATICS AND THE LAWS OF NATURE

Kelvin’s ruminations on the loss of the availability of energy are

reflected in what is usually called Kelvin’s version of the second

law:

It is impossible to construct a cyclic machine whose only effect

is to perform work using heat extracted from a single reservoir

that is the same temperature throughout.

Like Clausius’s version of the second law, Kelvin’s version is a

negative statement. It, too, states a basic law of nature in terms of

the impossibility of constructing a certain type of machine. Kelvin

used a heat engine in his formulation; Clausius used a refrigerator.

Notice that if Kelvin’s formulation of the second law were false,

then we could attach a cyclic heat engine to a single thermal res-

ervoir—the surface of the Earth, for example—and run it as long

as we wanted. The engine would have no other effect than that

of cooling Earth’s surface as it converted heat into work. Given

the amount of thermal energy in the Earth, this would be, for

all practical purposes, a perpetual motion machine. This kind of

machine has never been constructed. The failure to build this type

of device is one of the principal arguments supporting the truth of

the second law of thermodynamics.

Energy is one of the most important concepts in science, and

its importance has only increased in the years since Clausius pub-

lished his discoveries. It is now a fundamental concept in under-

standing the inner workings of galaxies and the inner workings of

atoms. It is as important in the philosophy of science as it is in the

design of refrigerators. It is, perhaps, the only physical principle

to find such wide applicability. The discoveries of Clausius and

Kelvin are among the most important in the history of science.

Models and Reality

The classical conservation laws, the laws of conservation of

momentum, mass, and energy, constitute the axioms for a deduc-

tive model of nature that remains one of the most used and use-

ful of all such models. Since the late 19th century, many other

Mathematics and the Laws of Thermodynamics 143

phenomena have been modeled in conceptually similar ways.

Electromagnetic phenomena, nuclear phenomena, and quantum

mechanical phenomena all demanded their own laws of nature,

and these proved to be very distinct from those governing classical

mechanics and thermodynamics. In each case, the laws formed a

basis for a new science, enabling engineers and scientists to cre-

ate new knowledge by reasoning deductively—their conclusions

always subject to additional experimental verification. No single

volume is sufficient to describe all of these deductive systems, but

the classical laws remain an excellent representative of all such

laws of nature. The preceding chapters reveal several important

characteristics that all laws of nature share.

First, every law of nature must be generalizable, which is anoth-

er way of saying that it must apply in circumstances other than the

ones in which it was first observed to apply. The more generaliz-

able the law, the more useful it is. Under certain circumstances,

for example, Leonardo’s conservation of volume law can be used

to investigate the flow of fluids such as water or air, but because

the conservation of mass law applies to a broader array of situa-

tions—in particular, it can be used anywhere that the conservation

of volume law is used—it is the better choice.

Second, laws of nature should be “fundamental” in the same

way that mathematical axioms are fundamental. Laws of nature

are not logical consequences of other phenomena. They are the

starting point from which reasoning begins. Within classical phys-

ics, for example, one cannot explain the assertion that energy is

conserved. Instead, the assertion that energy is conserved is used

to explain other phenomena. Similar statements are true for the

laws of conservation of mass and momentum. As has been pointed

out several times, experiments exist that demonstrate that mass,

for example, is not always conserved but within the class of phenom-

ena considered in classical physics, mass is a conserved quantity, and

the assumption that it is not conserved in “classical processes” will

yield results that are at variance with experiment.

Third, as mentioned at the beginning of this book, laws of nature

must be consistent in the sense that one cannot prove something

both true and false as a consequence of a set of natural laws.

144 MATHEMATICS AND THE LAWS OF NATURE

Finally, laws of nature must be as complete as possible in

the sense that those phenomena that are of importance to the

researcher must be deducible from the natural laws at hand.

The search for completeness explains why Clausius continued

his research after formulating the first law of thermodynamics:

Although all real processes conform to the first law, so do many

impossible processes. He concluded that there must be additional

restrictions—restrictions that he had not yet identified—and his

solution to this difficulty was the second law of thermodynamics.

In summary, engineers and scientists are model builders. They

construct deductive models of nature, and they use these models

to discover new knowledge. But these are “only” models. It would

be naïve to mistake the model for the phenomenon it claims to

describe. As more is learned about any particular model and the

phenomena it purports to describe, shortcomings in the model are

discovered, and new ideas are required.

When expressed in words, the classical conservation laws are

very broad statements, but their real utility is that they can also be

expressed mathematically, usually as differential equations. Their

mathematical expression depends on the application, and one law

may find many different mathematical expressions. The peculiar

mathematical form of each law of nature depends on the physi-

cal characteristics of the system that is being modeled. The dif-

ferential equations that describe the flow of water along a stream

bed, for example, are quite different from those that describe the

flow of air past the propeller of an airplane, and both those sets of

equations are very different from those that describe the flow of

blood through capillaries. Differences arise because each fluid—

and in engineering terminology anything that flows is classified

as a fluid—has its own density, its own viscosity (or stickiness),

its own degree of compressibility, as well as other less obvious

but still important properties, and each of these properties must

be incorporated into the mathematical equations that express the

relevant conservation laws. Only by incorporating fluid-specific

terms into the equations can researchers represent how a particu-

lar fluid responds to changing forces and temperatures. Similar

statements can be made about solids. The conservation laws are

Mathematics and the Laws of Thermodynamics 145

broadly applicable; their mathematical formulations are specific to

each situation.

Researchers are further constrained by the need for simplicity.

Although the differential equations must be expressive enough to

capture the relevant properties of the phenomena of interest, they

must also be simple enough to be solvable. Resolving the conflict-

ing demands of accuracy and simplicity is a delicate balancing act

that depends on the skill and goals of the researcher.

Now suppose that a researcher has chosen the relevant con-

servation laws, and suppose that the researcher has also decided

upon the best way to express these laws mathematically. Even at

this point, there is not sufficient information to begin solving the

resulting system of equations, which is the mechanism by which

new knowledge is deduced. The problem is that most systems of

equations have multiple solutions. To see why, imagine the flow

of water past the hull of a moving ship. Any solution to a system

of equations describing this flow will provide information about

the velocity of the water as it moves past the hull and the pressure

of the water against the hull. But there are many such solutions.

Isolating the relevant solutions from the irrelevant ones requires

additional information. The additional information is expressed

as initial conditions. The initial conditions might, for example,

include information about the velocity of the water far enough

upstream of the ship that it has not yet been disturbed by the pres-

ence of the ship. Once the initial conditions have been unambigu-

ously specified the researcher is ready to solve the equations and

discover new knowledge.

But specifying the initial conditions in a way that yields useful

information can be difficult. Often the initial conditions cannot

be known with as much precision as one would like. Consider the

plight of the automotive engineer who is attempting to model the

flow of air along the body of an automobile in order to reduce

wind resistance and so reduce fuel consumption. The engineer

wants a practical solution but does not know the speed at which

the car will normally be driven. These things matter. A design that

works well under one set of conditions may not work as well under

another set of conditions. The engineer can specify a certain set

146 MATHEMATICS AND THE LAWS OF NATURE

of initial conditions and then solve the conservation equations to

obtain the unique solution that satisfies these initial conditions, but

this approach cannot be entirely satisfactory because no single set

of initial conditions will accurately describe the day-to-day opera-

tion of the car. The engineer can, of course, solve the conservation

equations for many different initial conditions. Usually, each such

solution will be different from all previous solutions because previ-

ous solutions were the result of different initial conditions. In each

case, the design that minimizes fuel consumption may be differ-

ent. Deciding upon a final design requires engineering judgment

because no unique way exists for producing an optimal design in

the face of these sometimes conflicting requirements.

Natural laws, their mathematical expression, the choice of initial

conditions, the search for solutions, and their experimental verifi-

cation . . . This is a complex methodology. But it has also proved

to be a highly productive one. It is the method by which scientists

and engineers pursue their work, and it is a methodology upon

which all of us depend because it is the means by which progress

is made.

147

new insights into

conservation laws

Ancient science was, for the most part, applied geometry. Questions

that were essentially geometric—what, for example, is the cir-

cumference of Earth?—were studied intensively, but questions

that were not geometric were often ignored. Beginning in the

Renaissance, researchers began constructing the methodology that

was described in chapters 5 to 7, a system of inquiry that depended

on experiment, conservation laws, and mathematics, especially dif-

ferential equations. It is not that geometry was abandoned. It still

had a role to play, but geometric questions were no longer at the

heart of what it meant to “do” science. The study of conservation

laws eventually led back to geometry when it was shown that geo-

metric symmetries and conservation laws are, in a sense, two sides

of the same coin. The person responsible for this insight was the

German mathematician Emmy Noether (1882–1935).

Emmy Noether

Emmy Noether grew up in Erlangen, Germany, home to the

University of Erlangen, an institution that boasted a number of

distinguished mathematicians. Her father was a capable math-

ematician and was himself a member of the mathematics faculty

at Erlangen. Emmy, however, did not immediately gravitate to

mathematics. She showed facility with languages, and her origi-

nal plan was to teach foreign languages in secondary schools.

She even received her certification in English and French, but

she never taught languages. Instead she turned her attention to

148 MATHEMATICS AND THE LAWS OF NATURE

mathematics. First, she stud-

ied mathematics at Erlangen.

She also studied at Göttingen

University and eventually

earned a Ph.D. in mathemat-

ics at Erlangen.

Pursuing an advanced edu-

cation in the mathematical

sciences was a difficult career

path for a woman in Germany

at the time. Women were,

with permission of the instruc-

tor, allowed to take individual

courses. As a general rule,

however, women were barred

from completing the exami-

nations necessary to become

a full member of the faculty

of a university. At Erlangen

Noether sometimes taught a

class for her father, but she did so without pay. Her mathematical

talents, however, were eventually recognized by two of the most

distinguished of all mathematicians of that era, David Hilbert

and Felix Klein, both of whom were then at Göttingen. Noether

moved to Göttingen 1915.

Initially Noether taught courses under Hilbert’s name. Though

both Klein and Hilbert advocated that the university offer her a

position on the faculty, their request was denied. Other faculty

members objected to the hiring of women. It took time to over-

come some of the discrimination that she faced, and during this

time she taught classes without pay. As word of her discoveries

spread, however, mathematicians from outside Göttingen began

to show up in her classes. In 1919 she was offered a position on

the faculty.

Noether was Jewish, and in 1933, when the Nazis gained power

in Germany, she, like other Jewish faculty members, lost her job.

By this time, however, her contributions to mathematics had made

Emmy Noether, founder of the modern

theory of conservation laws

(Canaday

Library, Bryn Mawr College)

New Insights into Conservation Laws 149

her known to mathematicians throughout the world. Within a

few months of her dismissal she left Germany for the United

States. The remainder of her working life was spent at Bryn Mawr

College, Bryn Mawr, Pennsylvania, and the Princeton Institute

for Advanced Study, Princeton, New Jersey. Noether died within

a few years of moving to the United States of complications that

followed surgery.

Noether’s main interest was algebra. Abstract algebra is the

study of mathematical structure, and she had a particularly

insightful approach to the subject. She is often described as one of

the most creative algebraicists of the 20th century. By contrast her

work in the study of conservation laws was just a brief interlude.

She might not have become interested in the topic at all had not

her chief sponsor at Göttingen, David Hilbert, asked her for help.

During Noether’s stay at Göttingen several of the most promi-

nent mathematicians at the university—and at the time Göttingen

was home to some of the best mathematicians in the world—were

hard at work studying the mathematical basis of Albert Einstein’s

newly published general theory of relativity. Ideas were changing

quickly, and the mathematicians at Göttingen were in the thick

of it. David Hilbert, for example, had published equations similar

to those of Einstein within a few months of the date of Einstein’s

own papers.

Mathematically the problem with the equations that describe

relativity theory is that they are very complex. Initially the goal

was simply to try to understand what the equations imply about

the physical structure of the universe. There were questions, for

example, about the relationships between Einstein’s theory and

the theories of classical physics. In particular a number of math-

ematicians had questions about the role of conservation of energy

in Einstein’s model. Hilbert asked Noether for her help on this

issue, and Noether, though her interests were in pure mathemat-

ics and not physics, agreed to examine the issue. She quickly

identified a profound connection between conservation laws and

geometry. Her ideas on this matter have had a permanent influ-

ence on mathematicians’ and physicists’ understanding of what a

conservation law is.