Skiadas C.H., Dimotikalis I. (editors) Chaotic Systems: Theory and Applications
Подождите немного. Документ загружается.


318
V. V.
Sokolov
Independently
of
the
symmetry
class considered,
this
contribution
perfectly
compensates
the
second
term
in
the
r.h.s.
of
the
Eq
. (7).
The
resulting
me
an
cross
section
is identical
to
that
of
the
Efetov's
imaginary-potential
model
[6].
On
the
contrar
y, in
the
limit
of
weak
absorbtion
'"
«
(~~;::J2
the
result
which r
ea
ds as
(10)
strongly
depends
on
presence
or
abs
ence
of
the
time-r
ev
ersal
symmetry.
In
the
first case
the
well
known
asymptotic
expansion
[2]
of
the
two-point
cor-
relation
function
yields in
the
conside
red
case
of
perfect coupling
to
the
con-
tinuum
[11]
W(GOE)(r) =
5(r
-
rw)
-
~5'(r
-
rw)
+
~
5//(r
-
rw)
+
...
(11)
tH
2tH
whereas
in
the
second
ca
se
W(GUE)(r) =
5(r
-
rw)
+
...
(12)
where
contributions
of
the
omitted
terms
in (8)
are
O(1
/(M-
7
/
2
))
.
Within
this
accuracy
we
obtain
for
the
transport
functions (2):
C(GOE)
(E)
=
M!%[2
[
(1-~)
-
~
(1-
~~)J,
C(GUE)(E)
=
M!%I2
(1
_
~).
The
difference
i1C(E)
=
cC
GU
E)
(E)
_ C
(GOE)
(E)
= JvhM
2
(1
_
~!
"'''is)
(13)
M2
8
4M
is referred
to
as
the
weak localiz
ation
whereas
suppression
of
this
term
im-
plies
dephasing.
We conclude, therefore,
that
the
decay of
quasi-particles
in
the
e
nvironm
e
nt
not
only
induc
es
reduction
of
th
e
total
outgoing
flow
but
accounts
also for
the
dephasing
effect.
The
regime
of
weak a
bsorption
considered a
bov
e is
restricted
to
the
range
o <
'"
~
(r~~;::)2
which is very
narrow
if one
out
of
the
two
widths
r
s
,
rw
appreciably
exceeds
another.
Indeed
, if for
example
F.,
»
rw
and
th
ere
fore
'"
«
4rw
/
rs
« 1
the
probability
to
penetrate
into
the
environment
prevails
and
the
particle
can
finally
escap
e from
the
cavity
through
an
open
channel
only
if
the
decay
width
of
quasi-particle
in
th
e e
nvironment
is
small
enough,
"ie
« 4"is/M .
Otherwise
absorption
dominates.
On
the
contrary,
if
rw
»
rs
and
therefore",
«
4rs/
rw
= 4"is/ M
the
penetration
probability
is
small
and
particles
mostly
leave
the
cavity
before
penetrating.
In
the
both
cases
the
parameter
~
« k
and
the
influence
of
the
environment
in
Eq.
(13)

Electron Quantum Transport through a Mesoscopic Device 319
is negligible.
The
discussed
range
becomes
maximal,
0 <
""
:s
1,
when
rs
:::::;
rw·
In reality,
the
spreading
width
rs
which describes relatively weak
influence
of
the
environment
is
expected
to
be
smaller
than
the
characteristic
escape
width
rw
if
the
number
of
open
channels
M »
1.
But
the
adduced
arguments
show
that
the
role
of
the
considered
mechanism
of
suppression
of
the
weak localization increases in
the
case
of
small
number
of
open
channels,
which is
the
most
interesting
one
from
the
practical
point
of
view.
It
should
be
stressed
that,
rigorously,
the
asymptotic
expansions
(11, 12) for
the
weight
functions
w(r)
are
not
justified
[11]
in
the
case of few
number
of channels.
However,
at
least
qualitatively,
our
arguments
remain
valid.
References
1.A.G.
Huibers,
S.R.
Patel,
C.M. Marcus,
P.W.
Brouwer, S.L Durui:iz,
and
J.S.
Harris
(Jr.).
Phys. Rev. Lett.,
81
1917 (1998).
2.J.J.M.
Verbaarschot, H.A. Weidenmiiller,
and
M.R.
Zirnbauer,
Phys.
Rep.
129
367 (1985).
3.C.W.J.
Beenakker, Rev. Mod. Phys.
69
731
(1997).
4.Y. Alhassid, Rev. Mod.
Phys.
72895
(2000).
5.M. Biittiker,
Phys.
Rev. B
33
3020 (1986).
6.K.B. Efetov, Phys. Rev.
Lett.
742299
(1995).
7.E.
McCann
and
LC. Lerner,
J.
Phys.
Condo
Matter
8 6719 (1996).
8.P.W.
Brouwer
and
C.W.J.
Beenakker,
Phys.
Rev. B
55
4695 (1997).
9.V.V.
Sokolov
and
V.G. Zelevinsky, Nuc!.
Phys.
A
504
562 (1989).
1O.Valentin V.
Sokolov
and
Vladimir
Zelevinsky,
Phys.
Rev. C
56
311 (1997).
11.Valentin V. Sokolov,
AlP
Conf.
Proc.
995
85 (2008).

320
Composing Chaotic Music from a Varying Second
Order Recurrence Equation
Anastasios
D.
Sotiropoulos and Vaggelis
D.
Sotiropoulos
School
of
Music, Composition-Theory Division
University
of
Illinois at Urbana-Champaign, Urbana
Illinois 61801,
USA
(e-mail:
sotirop
1 @illinois.edu, asotiro2@illinois.edu)
Abstract:
Mu
sic
is
composed algorithmically from a varying second order recurrence
equation which defines sound frequency,
x,
at each step n knowing sound frequency and
amplitude,
y,
of
the previous step,
n-l,
as:
Xn
= C -
aX
n
_l
2
+
bYn
-l where the amplitude is
given as
Yn-l=
f (x
n
_],
X
n
-2)
with f
an
arbitrary function, and
a,
b, and c are also arbitrary
constants to be chosen by the composer. This compositional model includes as special
cases well-known recurrence equations as the logistic map, the
Henon map, and the
delayed Julia set. Another feature
of
our model is that the sound amplitude does not
necessarily have the same dependence on previous frequencies
in
each step. In fact,
by
the use
of
discrete form boxcar functions it can change either in consecutive steps or after
a finite number
of
steps. Compositional patterns are also incorporated by use
of
specific
equations for the change
of
frequency. The effect
of
initial frequencies chosen is
examined as well. Numerical examples are presented to show the chaotic behavior
of
our
model. A final score is presented
in
musical notation.
1.
Introduction
Music composition is a set
of
notes each
of
which
is
described by its
loudness (amplitude) and frequency. Any mathematical formula that
relates the amplitude and frequency
of
a note to the amplitude and
frequency
of
the previous note(s) and applied repeatedly constitutes an
algorithm and results in a complete music composition. When the
mathematical formula that relates the new sound to the previous sound
is non-linear, the resulting composition can exhibit the phenomena
of
bifurcation and chaos.
Previous works in the literature have dealt with musical
algorithms.
In
the 50's, Iannis Xenakis was the pioneer in introducing
mathematics and patterns in music composition which is described in
his book, Xenakis [1].
Other recent books such as the ones by Taube
[2]
and Karlheinz
[3]
exemplify the wide use
of
algorithmic music
composition
of
which mathematical based models include, among
many others, the magic squares by Browning
[4]
and manifolds by
Tipei [5]. The aesthetics
of
algorithmic compositions was addressed by
Garnett [6].

Composing Chaotic Music from Varying Second Order Recurrence Equation
321
The phenomena
of
bifurcation and chaos in music
composition have been examined in the literature mostly for well
known recurrence equations as the logistic map or the
Henon map. A
comprehensive description
of
the use
of
the logistic
equ~tion
in
composing music is posted in the world wide web by Elaine Walker
[7].
In
a very recent paper
of
ours [8], another recurrence relation that
leads to chaos was proposed based on a frequency amplitude triangle,
where the frequency
of
a note is given
as
the square root
of
the side
of
a
triangle whose other two sides are given respectively by the frequency
and sound
of
the previous note.
In
the present paper, we propose yet a
new compositional model based on a varying second order recurrence
relation that leads to chaos.
2. The model
The compositional model we propose is based on a varying second
order recurrence equation which defines sound frequency, x, at each
step n knowing sound frequency and amplitude,
y,
of
the previous step,
n-l,as:
where f is an arbitrary function and
a,
b, and c are also arbitrary
constants
to
be chosen by the composer. We note that the second order
effect comes from the dependence
of
the sound amplitude on the
previous sound frequency. For given
a,
band
c, the variations in the
equation come from choosing, in general, different functions f at
different steps. The composer needs to also choose the initial two
frequencies.
It
is convenient from the computational point
of
view
to
use small numbers for x that are used in the mathematical literature for
recurrence equations and do not correspond to actual sound
frequencies. The mathematical frequencies
Xn
are then converted to
standard MIDI (Musical Instrument Digital Interface) note numbers
ranging from
0 to 127 by using the following equation
midikey
= floor (127(2+x)/4 +0.5)
(2)
where midikey is the MIDI note number and floor(z) is the largest
integer not greater than
z.
We note that equation (3) is valid for x
between -2 to +2.
If
the largest value
of
x that comes out
of
the
recurrence equation
(1) is greater than 2 then, in equation (3), x is
adjusted accordingly
to
yield note numbers between 0 and 127. The
322 A.
D.
Sotiropoulos
and
V.
D.
Sotiropoulos
actual frequency, f,
of
notes in an A440 equal temperament is given in
terms
of
MIDI note numbers
by
the fonnuia
f I (440 Hz) =
imidikey-69)!l2
(3)
3. Analysis,
numerical
results,
and
discussion
The
reCUlTence
equation proposed given by equation
0),
includes as
special cases well known classical recurrence relations that lead to
chaos.
If
c=l
and
Yo-I=
XII-I.
then equation
(l)
reduces to the well known
Henon equation.
If
a =
0,
b
=1
and
Yn-[=
XII},
then equation
(1)
becomes the delayed Julia set, whose chaotic features have been
discussed in
a recent
book
by Skiadas and Skiadas [9].
We
will now consider in detail a special case
of
the recurrence
equation
(1), defined by
Xn+l = I Xn-I I A -
l'
(4)
where A and r are arbitrary constants.
We
note that when A =2, equation
(4) results in a delayed real domain Julia set which for 1'=2, is well
known that
it yields chaos. The choice
of
the initial
two
frequencies has
a pronounced effect
on
the composition.
If
X2=Xj,
then the frequencies
of
the composition repeat once.
If
X2:f:Xj, then there is no frequency
repetition.
When
X2 = I
XI
I"
-
f,
the resulting frequencies repeat once
as welL
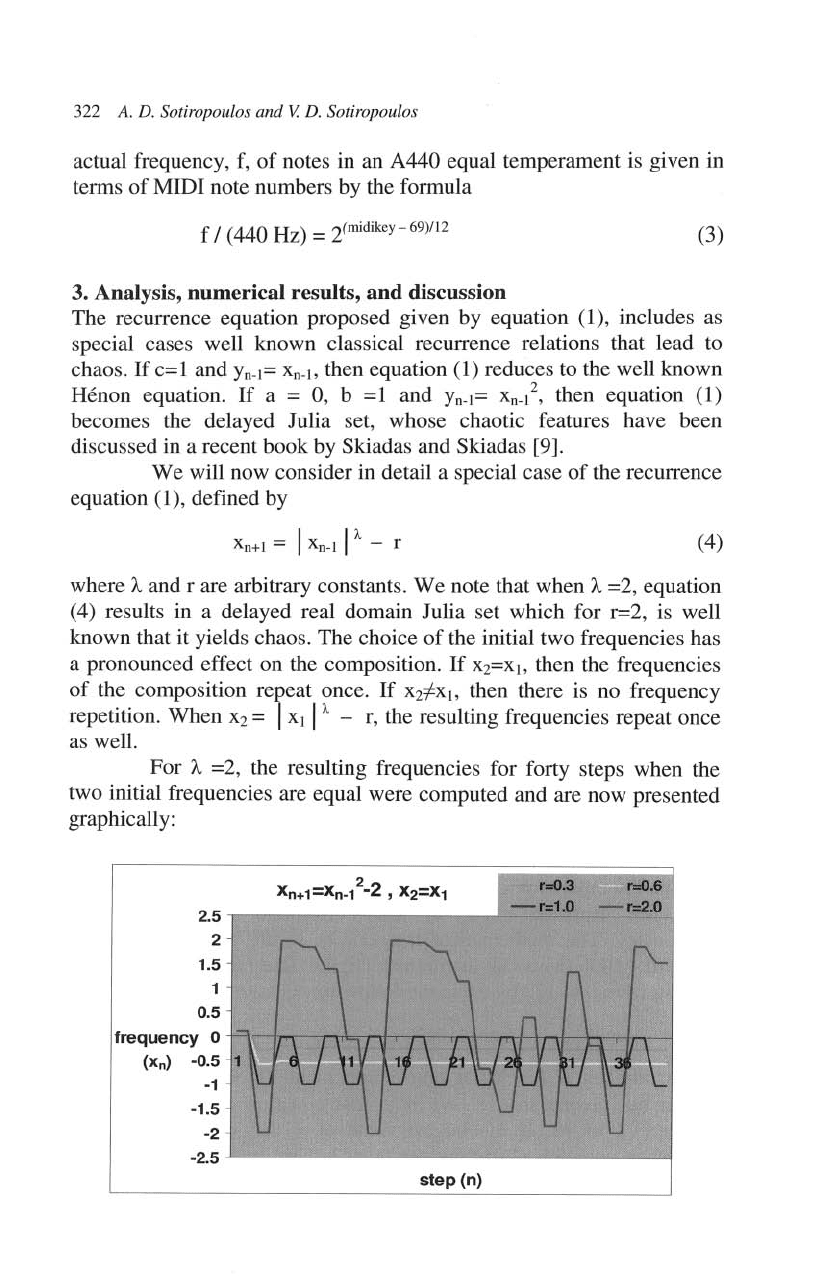
For
A =2, the resulting frequencies for fOlty steps when the
two initial frequencies are equal were computed and are now presented
graphically:
2.5
2
1.5
1
0.5
frequency 0
(xn) -0.5
-1
-1.5
-2
-2.5
Composing Chaotic Musicfrom Valying Second Order Recurrence Equation 323
The frequency repetition is apparent. When
1'=1
a period doubling
is
observed. When r=2, as expected, there
is
chaos.
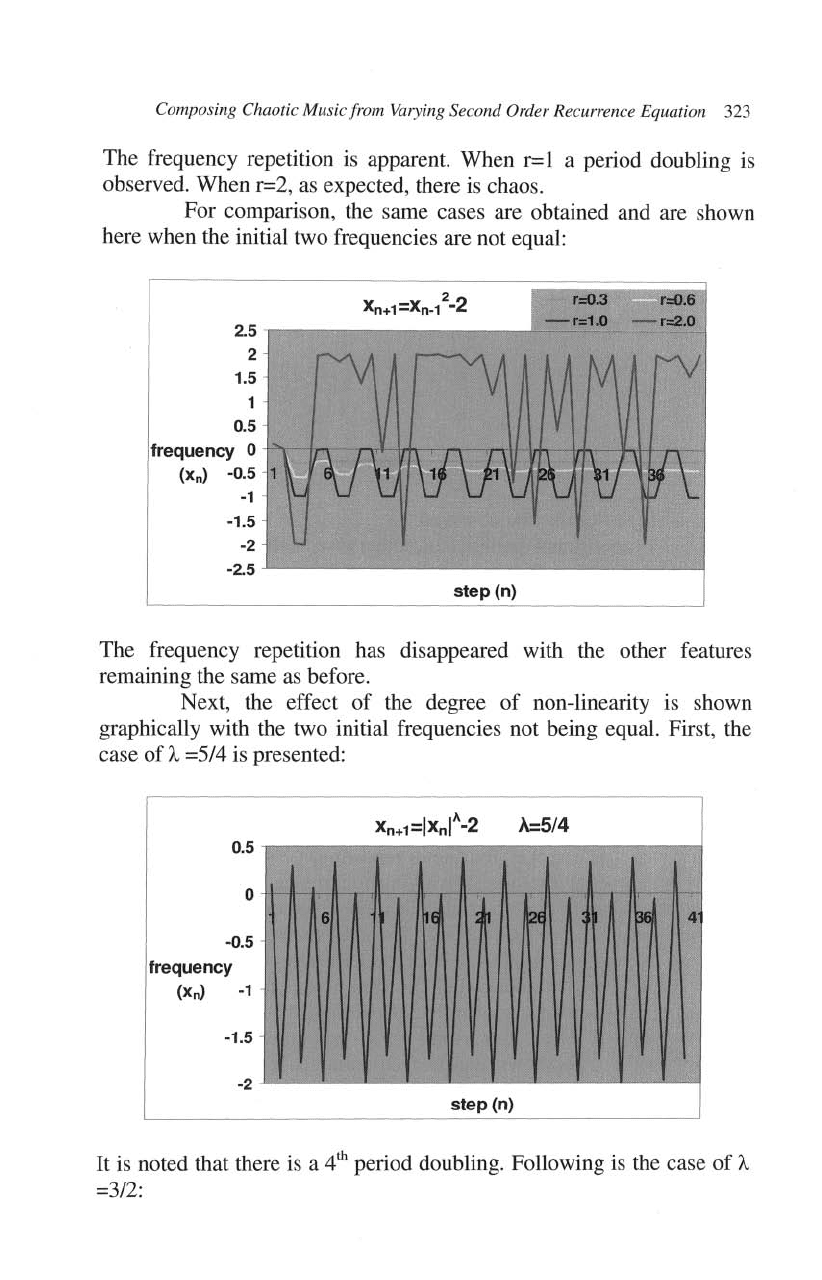
For comparison, the same cases are obtained and are shown
here when the initial two frequencies are not equal:
2.5
2
1.5
1
0.5
!frequency 0
(x
n
)
-0.5
-1
-1.5
-2
-2.5
Thc frequency repetition has disappearcd with the other features
remaining the same
as
before.
Next, the effect
of
the degree
of
non-linernity is shown
graphically with the two initial frequencies not being equal. First, the
case
of
Ie
=5/4 is presented:
0.5
o
-0.5
ifrequency
{Xn}
-1
-1.5
-2
step
(n)
It is noted that there is a
4th
period doubling. Following is the case
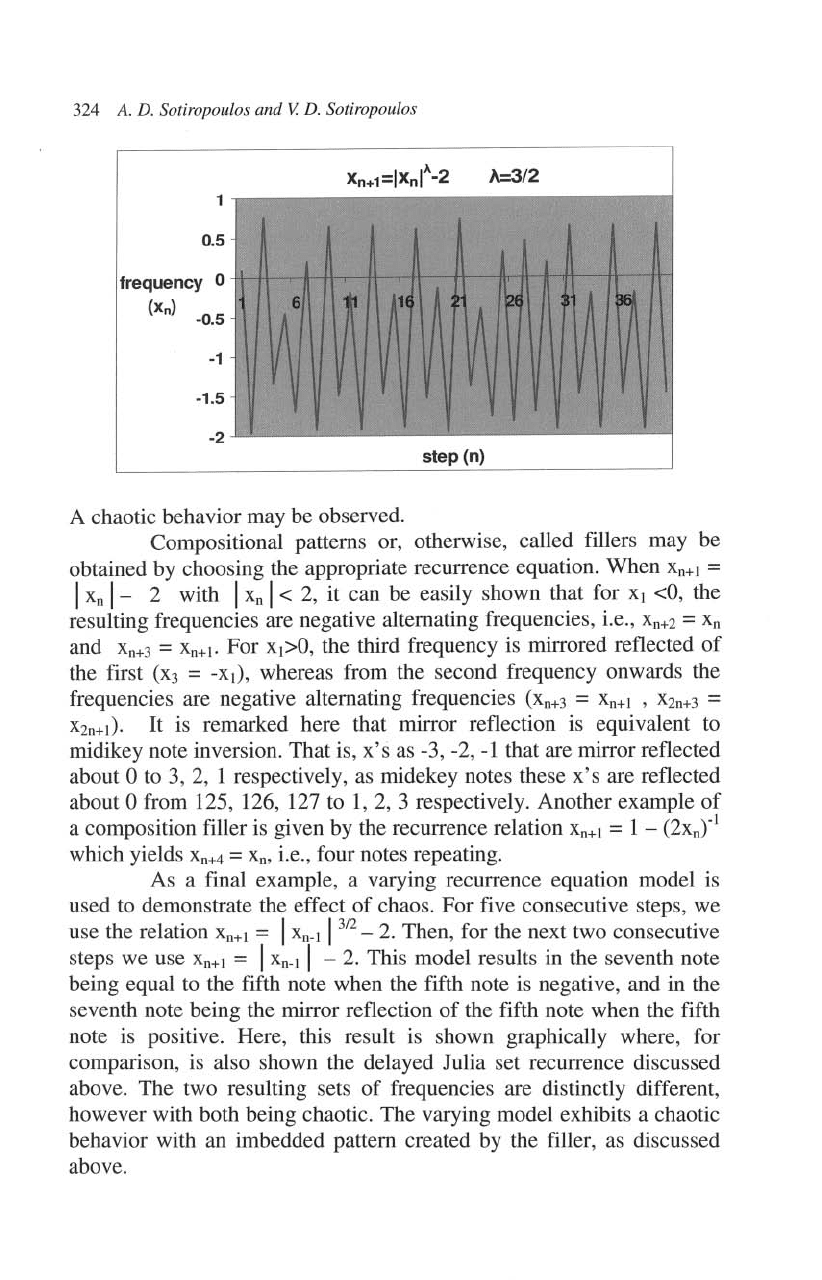
of
Ie
=3/2:
324 A.
D.
Sotimpoulos
and
ED.
Sotiropoulos
1
0.5
0
(:xn)
-0.5
·1
-1.5
-2
A chaotic behavior may be observed.
Compositional pattems or, otherwise, called fillers may be
obtained by choosing the
appropliate recurrence equation.
When
Xn+l
==
I
Xu
1-
2 with I
Xn
I < 2, it can be easily shown that for
Xl
<0, the
resulting frequencies are negative altemating frequencies, i.e
..
Xn+2
==
Xl!
and Xn+3
==
Xn+l.
For
Xj>O, the third frequency is mirrored reflected
of
the first (
X3
=
-xd,
whereas from the second frequency onwards the
frequencies are negative alternating frequencies (Xn+3
==
Xn+l , X2n+3
==
X2n+l)'
It
is remarked here that mirror rel1ection is equivalent to
midikey note inversion.
That
is,
x'
s as -3, -2,
-1
that are mirror reflected
about
0 to 3,
2,
1 respectively, as midekey notes these
x's
are reflected
about
0 from 125, 126, 127
to
1,
2,3
respectivel
y.
Another example
of
a composition fiIler is given by the recurrence relation Xn+l
==
1 - (2xnr
1
which yields Xn+4
==
Xn. i.e., four notes repeating.
As a final example, a varying
reCUlTence
equation model is
used to demonstrate the effect
of
chaos.
For
five consecutive steps,
we
use the relation Xn+!
;;:;;
I X
n
-l 1
312
- 2. Then, for the next two consecutive
steps we
use
)(n+1
;;:;;
I Xn-l I -2. This model results
in
the seventh note
being equal to
the fifth note when the fifth note is nega
ti
ve, and in the
seventh note being the mirror ret1ection
of
the fifth note when the fifth
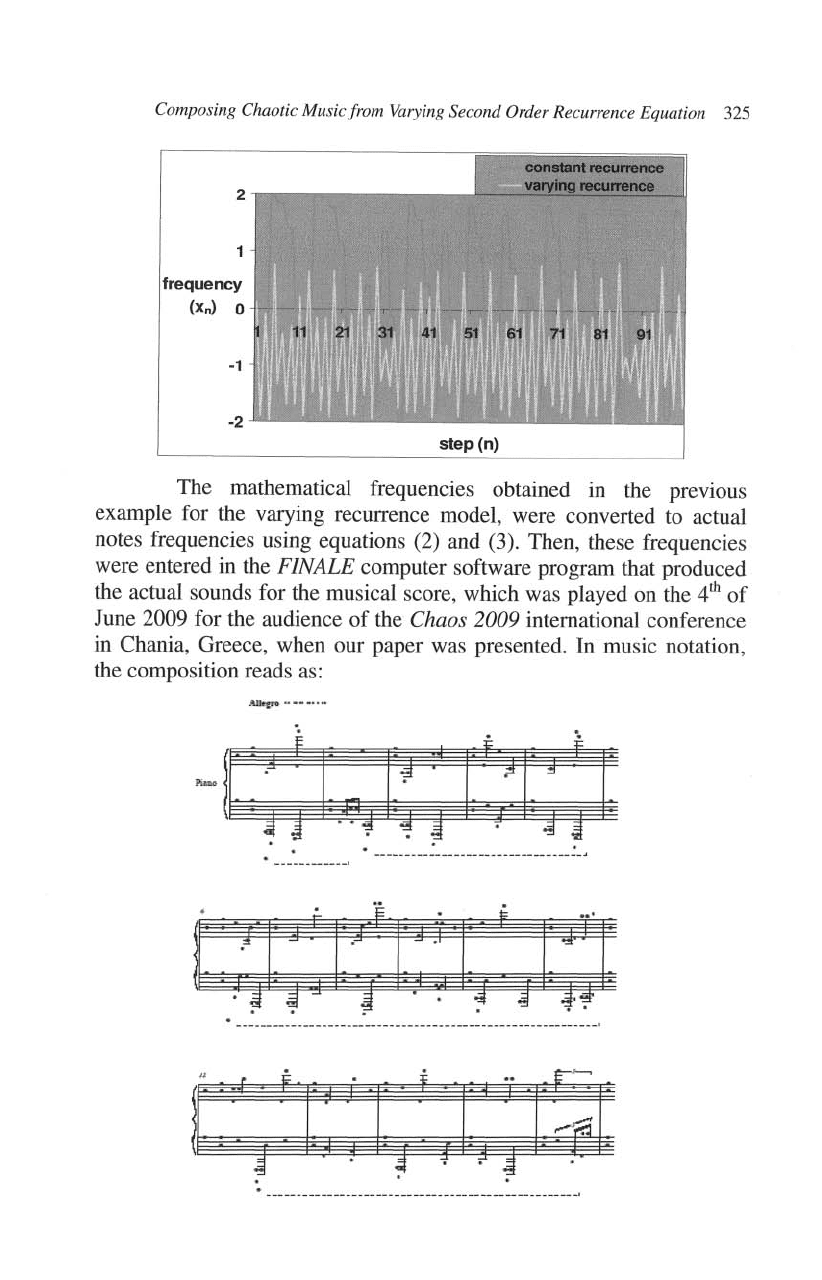
note is positive. Here, this result is shown graphically where, for
comparison, is also shown the delayed Julia set recurrence discussed
above.
The
two resulting sets
of
frequencies are distinctly different,
however with both being chaotic. The varying model exhibits a chaotic
behavior with an imbedded pattern created
by
the filler, as discussed
above.
Composing Chaotic Music/rom Varying Second Order Recurrence Equation
325
frequency
(x
n) 0
-1
-2
The mathematical frequencies obtained
in
the previous
example for the varying recurrence model, were converted
to
actual
notes frequencies using equations (2) and (3). Then, these frequencies
were entered in the
FINALE computer software program that produced
the actual sounds for the musical score, which was played on the
4th
of
June 2009 for the audience
of
the Chaos 2009 international conference
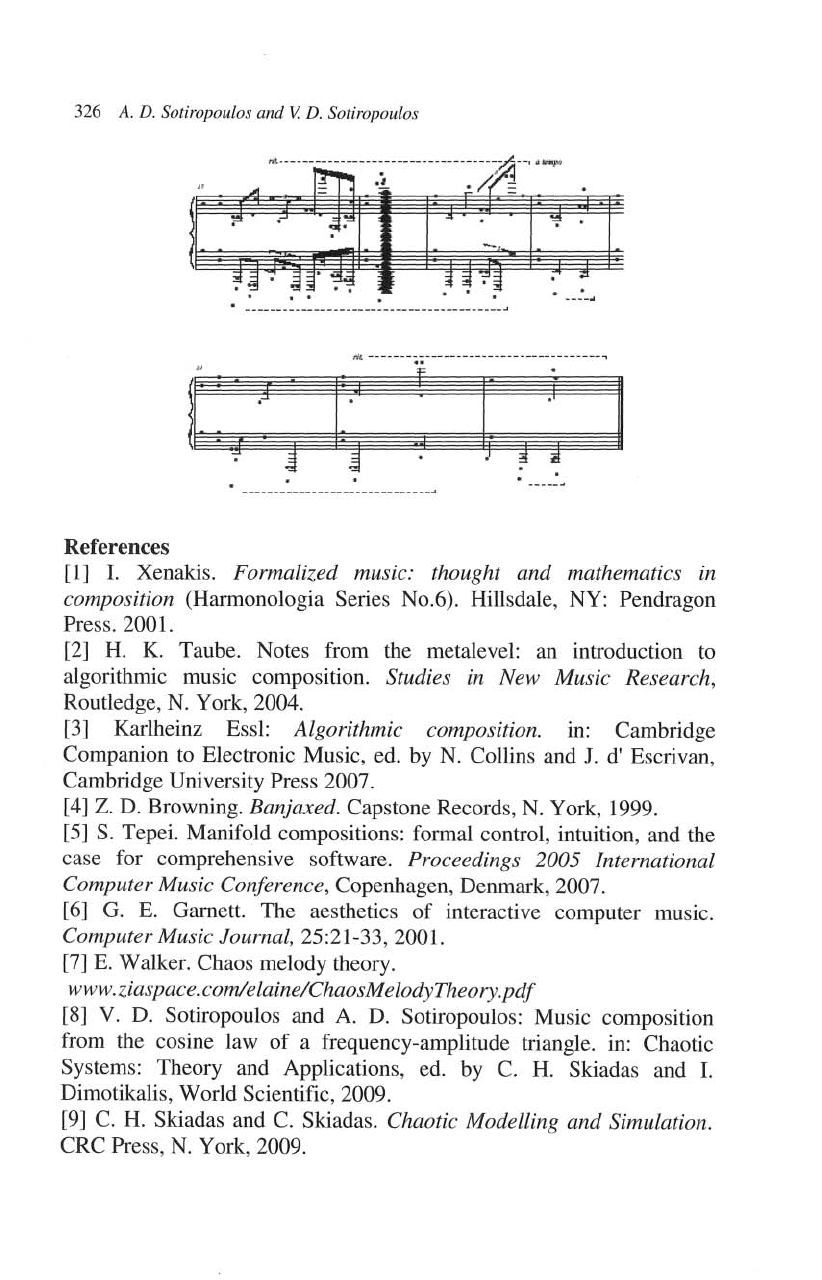
in Chania, Greece, when our paper was presented. In music notation,
the composition reads
as:
AJJouo
••
--
_
••••
-
r;
; r 8 I
::
: I::
~
I
~;
: I:
..
4 @
. .
. .
--
--
---
--
--
-- - - - - -----
--
--
--
--
--
-_
..
.
J.
. .
..
. .
~
326
A.
D.
Sotiropoulos and
V.
D.
Sotiropoulos
m __________
------------------------~~-
-,
4:ii¥!J'"
.!.. •
/';
-
.
"
1-,.
____
~
. ---------
--
------
-----
--
--
----
----
----
--~
...
rit:
-----
--~;
---
---
-----
~
---
-
------
------,
References
[1]
1.
Xenakis. Formalized music: thought
and
mathematics in
composition
(Harmonologia Series No.6). Hillsdale, NY: Pendragon
Press. 2001.
f2]
H.
K Taube. Notes from the metalevel: an introduction
to
algorithmic music composition. Studies in New Music Research,
Routledge,
N.
York, 2004.
[3] Karlheinz Essl: Algorithmic composlllon. in: Cambridge
Companion to Electronic Music, cd. by
N.
Collins and J. d' Escrivan,
Cambridge University
Press 2007.
[4] Z. D. Browning.
B01~jaxed.
Capstone Records, N. York, 1999.
[5)
S.
Tepei. Manifold compositions: formal control, intuition, and the
case for comprehensive software. Proceedings
2005
International
Computer Music
Cmfference, Copenhagen, Denmark, 2007.
[6]
G.
E.
Garnett. The aesthetics
of
interactive computer music.
Computer Music Journal, 25:21-33,
200l.
[7]
E.
Walker. Chaos melody theory.
www.ziaspace.com/eiaine!ChaosMeiodyTheory.pdj
[8] V. D. Sotiropoulos and A. D. Sotiropoulos: Music composltlon
from the cosine law
of
a frequency-amplitude triangle. in: Chaotic
Systems: Theory and Applications, ed. by C. H. Skiadas and
1.
Dimotikalis, World Scientific, 2009.
191
c.
H. Skiadas and C. Skiadas. Chaotic Modelling
and
Simulation.
CRC Press,
N.
York, 2009.

Music Composition from the Cosine Law
of
a
Frequency-Amplitude Triangle
Vaggelis D. Sotiropoulos and Anastasios D. Sotiropoulos
School
of
Music, Composition-Theory Division
University
of
Illinois at Urbana-Champaign, Urbana, Illinois 61801, USA
(e-mail:
sotiropl@illinois.edu,
asotiro2@illinois.edu)
327
Abstract:
We
propose a frequency-amplitude triangle whose cosine law
is
used
as
a recurrence
equation to algorithmically compose music. The triangle is defined by two sides and the angle
between them; one of the sides is the sound frequency,
x,
at a step, the other side is the sound
amplitude,
y,
at the same step, while the angle between them is a free parameter
to
be chosen by the
composer. The resulting third side of the triangle is defined
as
the square root
of
the sound
frequency,
x,
of
the next step. The sound amplitude,
y,
at each step depends,
in
general, on the
amplitude and frequency of the same or previous step(s)
in
any way the composer chooses. To test
our proposition for bifurcation and chaos against known solutions,
we
consider in detail the case
where the amplitude depends only on the frequency of the same or some previous step
as
Yn
= b(x" . .,)' , with b being a free parameter, m either zero or a positive integer, and
'A
a real number
greater than or equal
to
zero.
If
'A
is taken
as
zero, the resulting equation after a change
of
variables
becomes the one parameter logistic recurrence equation. From the known range
of
the parameter for
bifurcation and chaos,
we
obtain the range of values for sound amplitude and angle 8 for periodic
and chaotic music. For specific values, the frequencies corresponding
to
musical notes are obtained
from the proposed triangular recurrence equation and musical chaotic scores are composed and
presented.
Other
'A's
are also considered and their effect on the periodicity
of
the frequencies is
examined.
1.
Introduction
In the present paper
we
are concerned with algorithmic music composition. Each
note in a music composition is described by its loudness (amplitude) and
frequency. Any mathematical formula that relates the amplitude and frequency
of
a note to the amplitude and frequency
of
the previous note(s) and applied
repeatedly constitutes an algorithm and results in a complete music composition.
When the mathematical formula that relates the new sound
to
the previous sound
is non-linear, the resulting composition can exhibit the phenomena
of
bifurcation and chaos.
The principles
of
algorithmic composition have been used since
medieval times. An example
of
a musical algorithm
is
the round,
of
which the
earliest traced is Sumer Is Icumen In (1260). In the round, singers are instructed
to sing the same melody line, but starting at set delays with respect to each
other. In the 20
th
century with the appearance
of
computers, algorithmic music
composition blossomed. In the
50's, Iannis Xenakis was the pioneer in
introducing mathematics and patterns in music composition which is described
in his book, Xenakis [1]. Other recent books such as the ones by Taube
[2]
and
Karlheinz
[3]
exemplify the wide use
of
algorithmic music composition
of
which mathematical based models include, among many others, the magic
squares by Browning
[4]
and manifolds by Tipei [5]. Moreover, the aesthetics
of
algorithmic compositions was addressed by Garnett [6].
