Poto?nik P. (Ed.) Natural Gas
Подождите немного. Документ загружается.

Steady State Compressible Fluid Flow in Porous Media 481
p
p y
a
2
2 2
1
(36)
Where
( )
3
a
x72.0
2
x48.1x96.4
6
2
2
p
3
a
x36.0
2
a
x5.0
a
x1
a
p
aa
a
y
aa
)(
+++
+++=
+
( )
2
a
x72.0
a
x96.196.4
6
a
p
u
++
)L
2
S
p2
(AA
a
p
aa +=
R
2
T
2
z
2
2
psinM2
2
S
,
M
5
p
gd
2
RW
2
T
2
z
p
f621139.1
2p
AA
=
=
R
av
T
a
av
z
LsinM2
a
x
,
M
5
p
gd
2
RW
av
T
av
z
p
f621139.1
a
p
u
=
=
Where:
p
1
= Pressure at inlet end of porous medium p
2
= Pressure at exit end of porous medium
f
p
= Friction factor of porous medium.
θ = Angle of inclination of porous
medium with horizontal in degrees.
z
2
= Gas deviation factor at exit end of
porous medium.
T
2
= Temperature at exit end of porous
medium
T
1
= Temperature at inlet end of porous
medium
z
a v
= Average gas deviation factor
evaluated with T
a v
and p
a v
T
a v
= Arithmetic average temperature of
the porous medium given by
0.5(T
1
+ T
2
) and
p
a v
a
=
a
p
aa
2
2
p +
In equation (36), the component k
4
in the Runge - Kutta algorithm was given some
weighting to compensate for the variation of temperature (T) and gas deviation factor (z)
between the mid section and the inlet end of the porous medium. In isothermal flow where
there is little variation of the gas deviation factor between the mid section and the inlet end
of the porous medium, the coefficients of x
a
change slightly, then,
( )
)
2
a
x5.0
a
x25(
6
2
p
u
)
3
a
x5.0
2
a
x2
a
x5(
6
2
2
p
3
a
x25.0
2
a
x5.0
a
x1
a
p
aa
a
y
+++
+++
+++=
Application of the Runge-Kutta algorithm to equation (34) produces.
p p y
b
2 2
1 2
(37)
(
)
3
b
x36.0
2
b
x5.0
b
x1
b
p
aa
b
y +++=
( )
2
b
x72.0
2
b
x48.1
b
x96.4
6
2
2
p
+++
( )
2
b
x72.0
b
x96.196.4
6
2
p
u
+++
Natural Gas482
Where aa
p
b
=
L )
2
S
/
2p
AA( +
kM
2
p
d
RW
2
T
2
zc546479.2
kM
2
2
d
RW
2
T
2
zc8
kM
p
A
RW
2
T
2
zc2
/
2p
AA
′
=
′
=
′
=
Mk
2
p
d
RW
av
T
b
av
zc2.546479
Mk
P
A
RW
av
T
b
av
zc2
b
p
u ,
R
2
T
2
z
2
2
psinM2
2
S
′
=
′
==
R
av
T
b
av
z
LsinM2
b
x
=
Where
=
b
av
z Average gas deviations factors evaluated with T
a v
and p
a v
b
T
a v
= Arithmetic average Temperature of the porous medium = 0.5(T
1
+T
2
),
aa5.0 p
b
p
2
2
b
va
p
All other variables remain as defined in equation (36). In isothermal flow where there is not
much variation in the gas deviation factor (z) between the mid section and inlet and of the
porous medium there is no need to make compensation in the k
4
parameter in the Runge
Kuta algorithm, then equation (37) becomes:
p p
y
bT
2 2
1 2
(38)
Where:
++++= )
3
b
x5.0
2
b
x25.0
2
b
x5.0
b
x1(
b
p
aa
bT
y
p
x x x
b b b
2 3
2
5 2 0.5
6
b
u
p
x x
b b
2
5 2 0.5
6
Equation (36) can be arranged as:
PU] )
3
c
x36.0
2
c
x5.0
c
x1(
2
T
2
z[
a
p
BB
p
f
2
W ++++
c c c
c c c
2
p
2 2
1
p
- x x x -
p
1 2
6
- S x x x
2
2 2
4.96 1.48 0.72
2 3
1 0.5 0.36
(39)
Where
(
)
2
x72.0
2
x48.1x96.4Tz PU
ccc
v
aav
++=
,
R
2
T
2
z
2
2
psinM2
2
S,
M
5
p
dg6
RL621139.1
a
p
BB
==
M L
x
c
c
z T R
av av
2 sin
c
av
z
Average gas deviations factors
evaluated with T
av
and p
av
c
and
2
2
2
p
2
1
p
p
c
av
+
=
All other variables remain as defined in previous equations.
In isothermal flow where there is no significant change in the gas deviation factor (z),
equation (39) becomes:
x x x
c c c
a
W f BB z T
p
P
x x
c c
2 3
(1 0.5 0.25
2
2 2
2
(5 2 0.5 )
)(
)
Steady State Compressible Fluid Flow in Porous Media 483
Where aa
p
b
=
L )
2
S
/
2p
AA( +
kM
2
p
d
RW
2
T
2
zc546479.2
kM
2
2
d
RW
2
T
2
zc8
kM
p
A
RW
2
T
2
zc2
/
2p
AA
′
=
′
=
′
=
Mk
2
p
d
RW
av
T
b
av
zc2.546479
Mk
P
A
RW
av
T
b
av
zc2
b
p
u ,
R
2
T
2
z
2
2
psinM2
2
S
′
=
′
==
R
av
T
b
av
z
LsinM2
b
x
=
Where
=
b
av
z Average gas deviations factors evaluated with T
a v
and p
a v
b
T
a v
= Arithmetic average Temperature of the porous medium = 0.5(T
1
+T
2
),
aa5.0 p
b
p
2
2
b
va
p
All other variables remain as defined in equation (36). In isothermal flow where there is not
much variation in the gas deviation factor (z) between the mid section and inlet and of the
porous medium there is no need to make compensation in the k
4
parameter in the Runge
Kuta algorithm, then equation (37) becomes:
p p
y
bT
2 2
1 2
(38)
Where:
++++= )
3
b
x5.0
2
b
x25.0
2
b
x5.0
b
x1(
b
p
aa
bT
y
p
x x x
b b b
2 3
2
5 2 0.5
6
b
u
p
x x
b b
2
5 2 0.5
6
Equation (36) can be arranged as:
PU] )
3
c
x36.0
2
c
x5.0
c
x1(
2
T
2
z[
a
p
BB
p
f
2
W ++++
c c c
c c c
2
p
2 2
1
p
- x x x -
p
1 2
6
- S x x x
2
2 2
4.96 1.48 0.72
2 3
1 0.5 0.36
(39)
Where
(
)
2
x72.0
2
x48.1x96.4Tz PU
ccc
v
aav
++=
,
R
2
T
2
z
2
2
psinM2
2
S,
M
5
p
dg6
RL621139.1
a
p
BB
==
M L
x
c
c
z T R
av av
2 sin
c
av
z
Average gas deviations factors
evaluated with T
av
and p
av
c
and
2
2
2
p
2
1
p
p
c
av
+
=
All other variables remain as defined in previous equations.
In isothermal flow where there is no significant change in the gas deviation factor (z),
equation (39) becomes:
x x x
c c c
a
W f BB z T
p
P
x x
c c
2 3
(1 0.5 0.25
2
2 2
2
(5 2 0.5 )
)(
)

Natural Gas484
- -
-
p
p x x x p
c c c
S L
x x x
c c c
2
2 2 3 2
1
5 2 0.5
1 2
6
2 3
2
1 0.5 0.25
6
(40)
When the porous medium is horizontal, S
2
= 0 and x
c
= 0 then from equation (40),
-p p
f
p
a
W BB z T z T
p
av av
2 2
1 2
2
4.96
2 2
(41)
In an isothermal flow where there is no variation in z,
p
f
=
2
T
2
z
a
p
BB
2
W6
2
2
p-
2
1
p
(42)
Example 4
The following data came from the book of (Giles et al., 2009) called “theory and problem of
fluid mechanics and hydraulics”
W = 0.75 1b/sec of air, R = 1544, L = 1800ft, d = 4inch = 0.333333ft,
g = 32.2ft/sec
2
, z
2
= z
av
a
= 1 (air is fluid), T
2
= R
0
550 F
0
90
av
T ==
(Isothermal flow), p
1
= 49.5psia = 7128psf, P
2
= 45.73 psia = 6585.12 psf.
Pipe is horizontal.
(a)
Calculate friction factor of the pipe (f)
(b)
If the pipe were to be filled with a homogenous porous material having a porosity
of 20% what would be the friction factor (fp )?
Solution
(a) Let BB
a
the equivalent BB
P
a
by use of a pipe then.
RL
a
BB
gd M
`1.621139
5
6
1195610.824
97.28
5
333333.02.326
18001544621139.1
=
×××
××
=
0.20463
550 11195610.824
2
0.75 6
2
6585.12-
2
7128
2
T
2
z
a
BB
2
W6
2
2
p-
2
1
p
f
=
××××
==
The calculated f agrees with f = 0.0205 obtained by Giles et al., who used another equation.
0.149071ft 0.2ft 333333.0
p
d )b( =×=
62.10934995
97.28
5
1490751.02.326
18001544621139.1
M
5
p
gd6
RL621139.1`
a
p
BB
=
×××
××
=
=
2
T
2
z
a
p
BB
2
W6
2
2
p-
2
1
p
p
f =
4-E 3.667626
550 1 210934995.6
2
0.75 6
2
6585.12-
2
7128
=
××××
=
The equation for pressure transverse in a porous medium by use of Darcian lost head
(equation (37) can be arranged as:
(
)
( )
+++
+++
]
[
2
c
x72.0
c
1.96x 96.4
av
T
b
av
z
c
3
x36.0
2
c
x5.0
c
x 1
2
T
2
z
k
b
p
BB
2
W
- -
-
p
p
x x x p
c c
b
S L
x x x
c c c
2
2 2 3 2
1
4.96 1.48 0.36
1 2
6
2 2
2
1 0.5 0.3
6
(43)
Where
,
M
2
p
d6
RLc576479.2
M
p
A6
RLc2
b
p
BB
′
=
′
=
Steady State Compressible Fluid Flow in Porous Media 485
- -
-
p
p x x x p
c c c
S L
x x x
c c c
2
2 2 3 2
1
5 2 0.5
1 2
6
2 3
2
1 0.5 0.25
6
(40)
When the porous medium is horizontal, S
2
= 0 and x
c
= 0 then from equation (40),
-p p
f
p
a
W BB z T z T
p
av av
2 2
1 2
2
4.96
2 2
(41)
In an isothermal flow where there is no variation in z,
p
f
=
2
T
2
z
a
p
BB
2
W6
2
2
p-
2
1
p
(42)
Example 4
The following data came from the book of (Giles et al., 2009) called “theory and problem of
fluid mechanics and hydraulics”
W = 0.75 1b/sec of air, R = 1544, L = 1800ft, d = 4inch = 0.333333ft,
g = 32.2ft/sec
2
, z
2
= z
av
a
= 1 (air is fluid), T
2
= R
0
550 F
0
90
av
T ==
(Isothermal flow), p
1
= 49.5psia = 7128psf, P
2
= 45.73 psia = 6585.12 psf.
Pipe is horizontal.
(a)
Calculate friction factor of the pipe (f)
(b)
If the pipe were to be filled with a homogenous porous material having a porosity
of 20% what would be the friction factor (fp )?
Solution
(a) Let BB
a
the equivalent BB
P
a
by use of a pipe then.
RL
a
BB
gd M
`1.621139
5
6
1195610.824
97.28
5
333333.02.326
18001544621139.1
=
×××
××
=
0.20463
550 11195610.824
2
0.75 6
2
6585.12-
2
7128
2
T
2
z
a
BB
2
W6
2
2
p-
2
1
p
f
=
××××
==
The calculated f agrees with f = 0.0205 obtained by Giles et al., who used another equation.
0.149071ft 0.2ft 333333.0
p
d )b( =×=
62.10934995
97.28
5
1490751.02.326
18001544621139.1
M
5
p
gd6
RL621139.1`
a
p
BB
=
×××
××
=
=
2
T
2
z
a
p
BB
2
W6
2
2
p-
2
1
p
p
f =
4-E 3.667626
550 1 210934995.6
2
0.75 6
2
6585.12-
2
7128
=
××××
=
The equation for pressure transverse in a porous medium by use of Darcian lost head
(equation (37) can be arranged as:
(
)
( )
+++
+++
]
[
2
c
x72.0
c
1.96x 96.4
av
T
b
av
z
c
3
x36.0
2
c
x5.0
c
x 1
2
T
2
z
k
b
p
BB
2
W
- -
-
p
p
x x x p
c c
b
S L
x x x
c c c
2
2 2 3 2
1
4.96 1.48 0.36
1 2
6
2 2
2
1 0.5 0.3
6
(43)
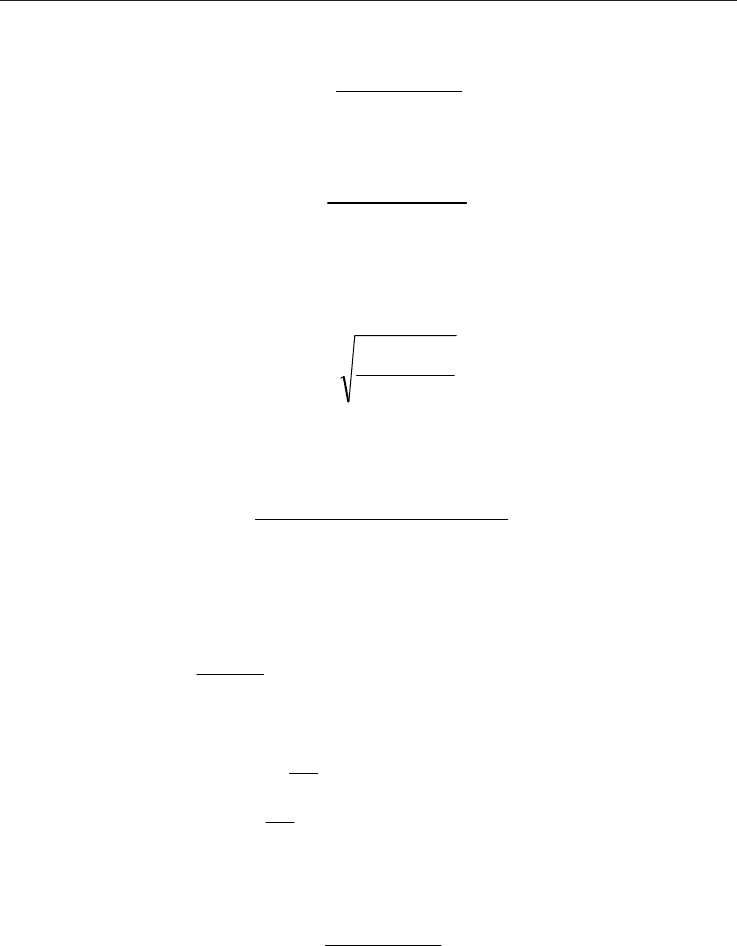
Where
,
M
2
p
d6
RLc576479.2
M
p
A6
RLc2
b
p
BB
′
=
′
=
Natural Gas486
R
2
T
2
z
2
2
P sinM2
2
S
=
R
av
T
c
av
z
L sinM2
c
x
=
z
a v
c
=
Average gas deviation factor calculated with p
a v
c
and T
a v
p
a v
c
=
2
p p
2
2
2
1
When the porous medium is horizontal, S
2
= 0, , and x
c
= 0, then ,
-p p
c
b
W BB z T 4.96 z T
p
av av
k
2 2
1 2
2
2 2
(44)
When the flow is isothermal and there is no significant variation in the gas deviation factor
(z) equation (44) becomes.
+++
+++
]
[
)
2
c
x5.0
c
2x 5(
2
T
2
z
)
c
3
x25.0
2
c
x5.0
c
x 1(
2
T
2
z
k
b
p
BB
2
W
- -
-
p
p x x x
c c c
S L
x x x
c c c
p
2
2 2 3 2
1
5 2 0.5
1 2
6
2 2
2
1 0.5 0.25
6
[
]
(45)
When the porous medium is horizontal, equation (45) becomes
-
b
WBB z T
p
k
p p
6
2 2
2 2
1 2
(46)
Example 5
The following problem came from the book of (Amyx et al., 1960). During a routine
permeability test, the following data were obtained.
Flow rate (Q) = 1,000cc
of air in 500sec.
Pressure down stream of core (p
2
) = 1 atm. absolute
Flowing temperature (T) = 70
0
F
Viscosity or air at test temperature (μ) = 0.02c
p
Cross-sectional area of core (A
p
) = 2cm
2
Pressure upstream of core (p
1
) = 1.45 atm absolute
Length of core (L
p
) = 2cm
Compute the permeability of the core in millidarcy
Solution
In oil field units in which pressure is in atmospheres and temperature is expressed in degree
Kelvin, R = 82.1
Here, T = 70
0
F = (70 + 460)
0
R =
8.1
R
0
530
K
0
4.294=
Q =
cc cc1000 / 500 sec 2 / sec
c
z z z 1 (air is fluid )
av1 2
The volumetric flow rate can be converted to weight flow rate by:
W=
Q where
=
p
M
zTR
Substituting given values
W
gm
1 28.97 2
1 82.1 294.4
0.002397163 / sec
Taking the core to be horizontal
where
pp
2
T
2
z
b
P
wBB6
k
2
2
2
1
) units ofset consistent ain 1c( ,
MA6
RL2
BB
p
p
b
2
_
E889311.1
97.2826
21.8202.02
=
××
×××
=

Steady State Compressible Fluid Flow in Porous Media 487
R
2
T
2
z
2
2
P sinM2
2
S
=
R
av
T
c
av
z
L sinM2
c
x
=
z
a v
c
=
Average gas deviation factor calculated with p
a v
c
and T
a v
p
a v
c
=
2
p p
2
2
2
1
When the porous medium is horizontal, S
2
= 0, , and x
c
= 0, then ,
-p p
c
b
W BB z T 4.96 z T
p
av av
k
2 2
1 2
2
2 2
(44)
When the flow is isothermal and there is no significant variation in the gas deviation factor
(z) equation (44) becomes.
+++
+++
]
[
)
2
c
x5.0
c
2x 5(
2
T
2
z
)
c
3
x25.0
2
c
x5.0
c
x 1(
2
T
2
z
k
b
p
BB
2
W
- -
-
p
p x x x
c c c
S L
x x x
c c c
p
2
2 2 3 2
1
5 2 0.5
1 2
6
2 2
2
1 0.5 0.25
6
[
]
(45)
When the porous medium is horizontal, equation (45) becomes
-
b
WBB z T
p
k
p p
6
2 2
2 2
1 2
(46)
Example 5
The following problem came from the book of (Amyx et al., 1960). During a routine
permeability test, the following data were obtained.
Flow rate (Q) = 1,000cc
of air in 500sec.
Pressure down stream of core (p
2
) = 1 atm. absolute
Flowing temperature (T) = 70
0
F
Viscosity or air at test temperature (μ) = 0.02c
p
Cross-sectional area of core (A
p
) = 2cm
2
Pressure upstream of core (p
1
) = 1.45 atm absolute
Length of core (L
p
) = 2cm
Compute the permeability of the core in millidarcy
Solution
In oil field units in which pressure is in atmospheres and temperature is expressed in degree
Kelvin, R = 82.1
Here, T = 70
0
F = (70 + 460)
0
R =
8.1
R
0
530
K
0
4.294=
Q =
cc cc1000 / 500 sec 2 / sec
c
z z z 1 (air is fluid )
av1 2
The volumetric flow rate can be converted to weight flow rate by:
W=
Q where
=
p
M
zTR
Substituting given values
W
gm
1 28.97 2
1 82.1 294.4
0.002397163 / sec
Taking the core to be horizontal
where
pp
2
T
2
z
b
P
wBB6
k
2
2
2
1
) units ofset consistent ain 1c( ,
MA6
RL2
BB
p
p
b
2
_
E889311.1
97.2826
21.8202.02
=
××
×××
=
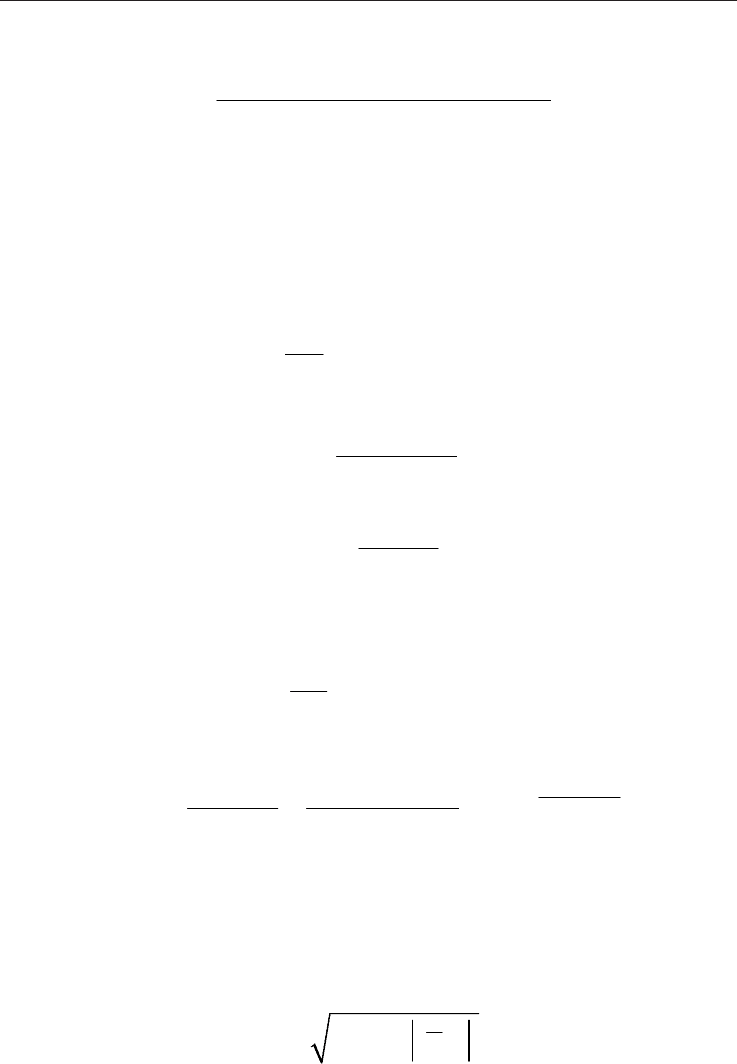
Natural Gas488
Then
millidarcy 72.56 darcy07256.0
4.29412E889311.13E397163.26
k
22
1-45.1
==
××××
=
Amyx , et al obtained the permeability of this core as 72.5md with a less rigorous equation.
Horizontal and Downhill Gas Flow in Porous Media
In downhill flow, the negative (-) sign in the numerator of equation (23) in used. Neglecting
the kinetic effect, equation (23) becomes:
-
dp
AA B P
P P
d
p
2
2
(47)
Where
,
M
5
p
gd
zTR
p
f621139.1
p
AA
=
zTR
sinM2
p
B
=
By use of the Darcian lost head, the differential equation for downhill gas flow in porous
media becomes.
_
dp
AA B
p
P P
d
p
2
2
(48)
Where
,
Mk
2
p
d
zTRWc546479.2
Mk
p
A
zTRWc2
P
AA
′
=
′
=
′
zTR
sinM2
p
B
=
Solution to the differential equation for horizontal and downhill flow
The Runge-Kutta numerical algorithm that was used to provide a solution to the differential
equation for horizontal and uphill flow can also be used to solve the differential equation for
horizontal and downhill flow. Application of the Runge - Kutta algorithm to equation (47)
produces.
_
p
p y
c
2
2 1
(49)
Where
- -
- -
c
y a a x x x
c p
d d d
p
x x x
d d d
2 3
(1 0.5 0.3 )
2
1
2 3
5.2 2.2 0.6
6
-
c
u
p
x x
d d
3
5.2 2.2 0.6
6
-
1 1
c
p
aa (AA
p
S )L
f z T RW
p
AA
p
gd M
p
M p
S
z T R
2
1.621139
1 1
,
1
5
2
2 sin
1
1
1 1
,
M
5
p
gd
2
RW
av
T
d
av
z
p
1.621139f
c
p
u
=
R
av
T
d
av
z
2MsinθL
d
x
=
d
av
z
= Gas deviation factor (z) calculated
with
T 0.5(T T )
av 1 2
and
d c
p p aa
av p
2
1
Other variables remain as defined in previous equations.
In equation (49), the parameter k
4
in the Runge-Kutta algorithm is given some weighting to
compensate for the variation of the temperature (T) and the gas deviation factor between the
mid section and the exit end of the porous medium. In isothermal flow in which there is no
significant variation of the gas deviation factor (z) between the midsection and the exit end
of the porous medium, equation (49) becomes.
-p p y
cT
2
2 1
(50)
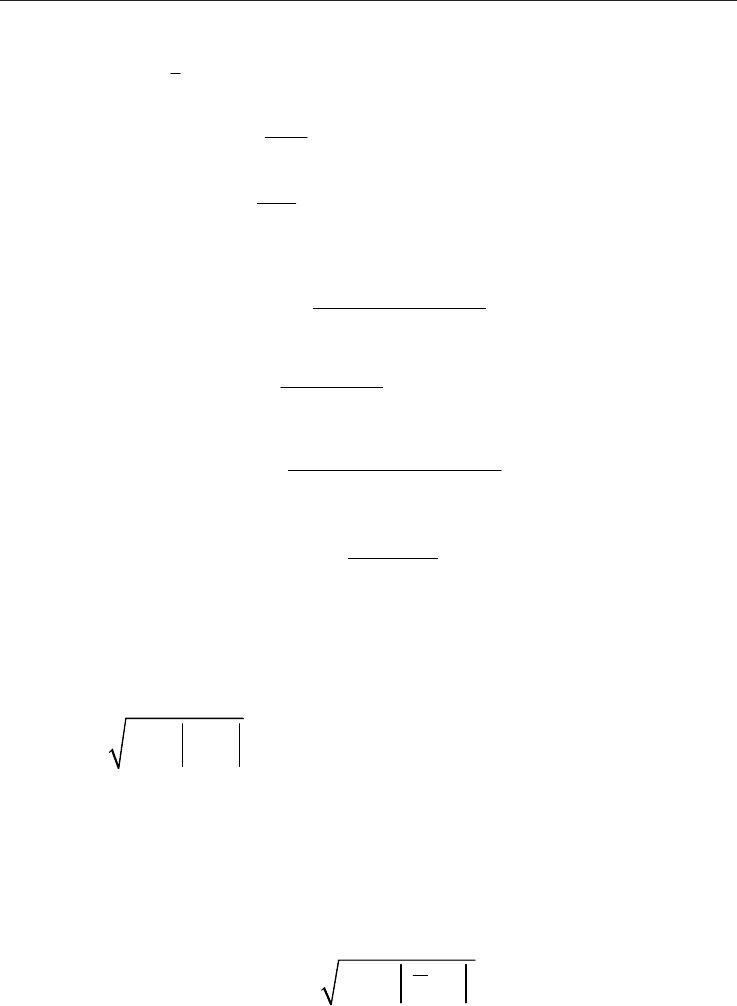
Steady State Compressible Fluid Flow in Porous Media 489
Then
millidarcy 72.56 darcy07256.0
4.29412E889311.13E397163.26
k
22
1-45.1
==
××××
=
Amyx , et al obtained the permeability of this core as 72.5md with a less rigorous equation.
Horizontal and Downhill Gas Flow in Porous Media
In downhill flow, the negative (-) sign in the numerator of equation (23) in used. Neglecting
the kinetic effect, equation (23) becomes:
-
dp
AA B P
P P
d
p
2
2
(47)
Where
,
M
5
p
gd
zTR
p
f621139.1
p
AA
=
zTR
sinM2
p
B
=
By use of the Darcian lost head, the differential equation for downhill gas flow in porous
media becomes.
_
dp
AA B
p
P P
d
p
2
2
(48)
Where
,
Mk
2
p
d
zTRWc546479.2
Mk
p
A
zTRWc2
P
AA
′
=
′
=
′
zTR
sinM2
p
B
=
Solution to the differential equation for horizontal and downhill flow
The Runge-Kutta numerical algorithm that was used to provide a solution to the differential
equation for horizontal and uphill flow can also be used to solve the differential equation for
horizontal and downhill flow. Application of the Runge - Kutta algorithm to equation (47)
produces.
_
p
p y
c
2
2 1
(49)
Where
- -
- -
c
y a a x x x
c p
d d d
p
x x x
d d d
2 3
(1 0.5 0.3 )
2
1
2 3
5.2 2.2 0.6
6
-
c
u
p
x x
d d
3
5.2 2.2 0.6
6
-
1 1
c
p
aa (AA
p
S )L
f z T RW
p
AA
p
gd M
p
M p
S
z T R
2
1.621139
1 1
,
1
5
2
2 sin
1
1
1 1
,
M
5
p
gd
2
RW
av
T
d
av
z
p
1.621139f
c
p
u
=
R
av
T
d
av
z
2MsinθL
d
x
=
d
av
z
= Gas deviation factor (z) calculated
with
T 0.5(T T )
av 1 2
and
d c
p p aa
av p
2
1
Other variables remain as defined in previous equations.
In equation (49), the parameter k
4
in the Runge-Kutta algorithm is given some weighting to
compensate for the variation of the temperature (T) and the gas deviation factor between the
mid section and the exit end of the porous medium. In isothermal flow in which there is no
significant variation of the gas deviation factor (z) between the midsection and the exit end
of the porous medium, equation (49) becomes.
-p p y
cT
2
2 1
(50)
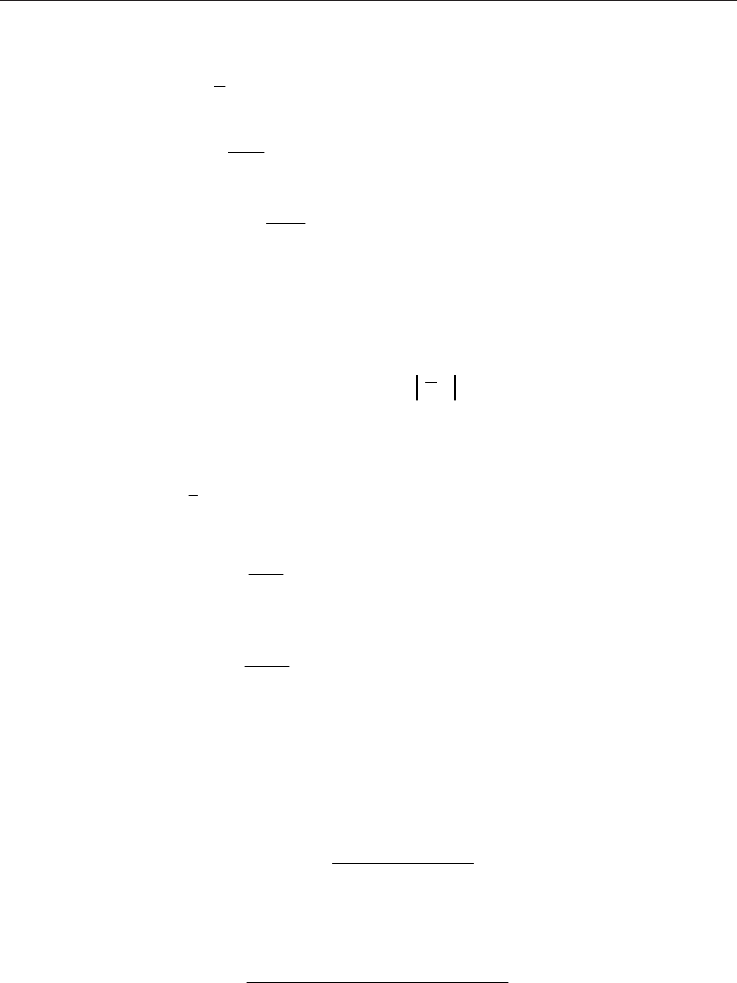
Natural Gas490
Where
- -
- -
c
y aa x x x
p
cT
d d d
p
x x x
d d d
2 3
(1 0.5 0.35 )
2
2 3
1
5.0 2.0 0.7
6
c
u
p
- x - x
d d
2
5.0 2.0 0.7
6
Other variables in equation (50) remain as defined in equation (49).
Application of the Runge -Kutta algorithm to the down hill differential equation by use of
Darcian lost head (equation (48)) gives
-
p
p y
d
2 2
2 1
(51)
Where
( )
3
d
x6.0-
2
e
x2.2
e
x2.5-
6
2
1
p
)
3
e
x3.0-
2
e
x5.0
e
x-1(
d
p
aa
d
y
++
+=
( )
2
e
x6.0-
e
x2.2-2.5
6
d
p
u
+
)L
1
S-
1
/
(AAp
d
p
aa =
,
Mk
2
p
d
RW
av
T
e
av
zc54679.2
Mk
p
A
RW
1
T
1
zc2
1
/
AAp
′
=
′
=
2
2Msinθ P
1
S
2
z T R
1 1
,
RTz
L 2Msinθ
x
av
av
e
e
Mk
2
p
d
RW
av
T
e
av
zμc2.546479
Mk
P
A
RW
av
T
av
μzc2
d
p
u
′
=
′
=
e
z
av
= Gas deviation factor (z) calculated
with T
a v
and
e
av
p
T T T
av
0.5( )
1 2
e d
p p aa
av p
2_
1
Equation (49) can be written as:
p p f f f
a
p f f f
S L
f W J x x x
BB Z T x x x XX
2 2 3
1
2 3
1 1
(1 0.5 0.3 )
6
(1 0.5 0.3 )
(53)
Where
f
av av f f
XX z T x x
2
(5.2 2.2 0.6 )
( )
1
S≥
a
p
BB if
2
2
p-
3
f
x6.0-
2
f
x2.2
f
x2.5-
6
2
1
p
-
2
i
P
p
J +=
( )
i
S
a
p
BB if
2
2
p-
f
3
x6.0-
f
2
x2 .. 2
f
x2 . 5
6
2
1
p
-
2
1
p-
2
2
P
p
J <+=
RL RL
a
BB
p
gd M gd M
p p
1.621139 0.270110
5 5
6
R
av
T
f
av
z
LsinM2
f
x,
M
5
p
gd6
2
1
psinM2
1
S
==
