Poto?nik P. (Ed.) Natural Gas

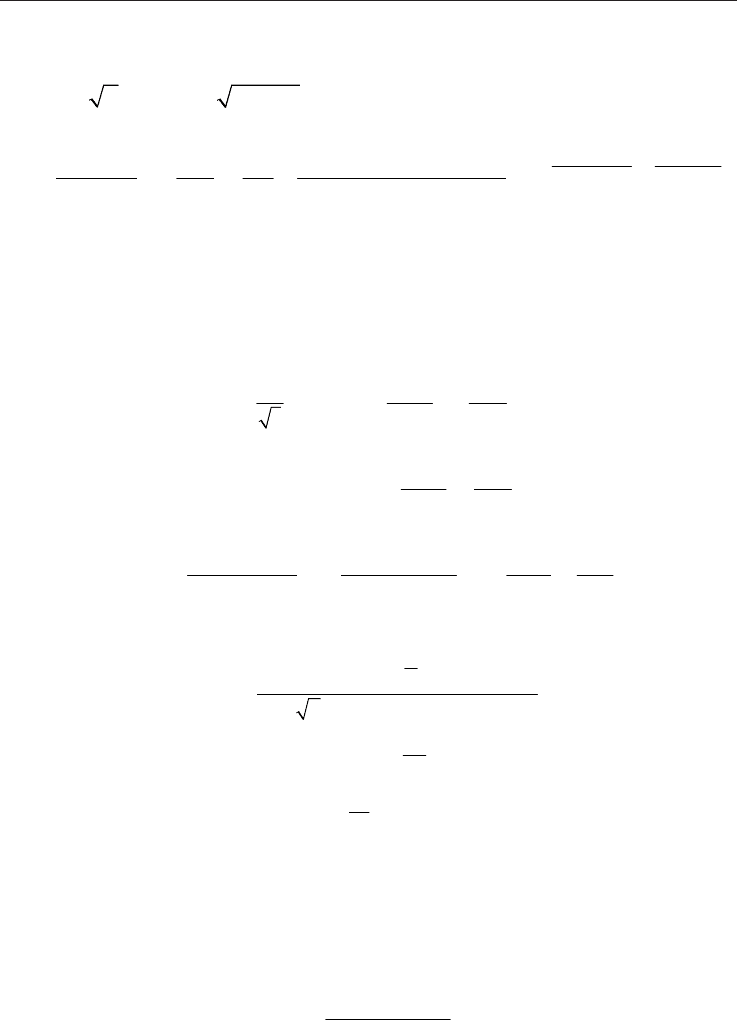
Static behaviour of natural gas and its ow in pipes 461

2 3

x 1 x 0.5x 0.36x

a

   

2 3

x 4.96x 1.48x 0.72x

b

  

2

x 4.96 1.96x 0.72x

c

  

The above equations are in oil field units in which d is expressed in inches, L in feet,

in centipoises, T in degrees rankine and pressure in pounds per square inches. In this

system of units, p

b

= 14.7 psia, T

b

= 520

R, z

b

= 1.0. The subscript 2 refers to

surface condition in the gas well.

Example 7

A gas well has the following data:

L = 5700ft, G

g

= 0.6,

90 ,



  z

2

= 0.78, z

av

= 0.821, f = 0.0176, T

2

= 543

R ,

T

av

= 581.5

R , d = 1.9956 in, absolute roughness of pipe= 0.0006 in.,p

1

= 2545 psia, p

2

=

2122 psia,

2

, viscosity of gas at p and T

2

0.0133 cp



. Calculate the flow rate of

the well ( Q

b

) in MM SCF / Day,

Solution

Substituting given values,

0.6 1 5700

x 0.0375016 0.268250

0.822 581.5

 

  



5

0.6 5700

B 4.191201 452.6012689

1.9956



  

2

0.6 1 2122

S 0.0375016 238.680281

0.78 543

 

  



2 3

x 1 x 0.5x 0.36x

a

   

= 1+0.268250 + 0.5x0.268250

2

+0.36x0.268250

3

= 1.311178

2 3

x 4.96x 1.48x 0.72x

b

  

= 4.96x0.268250+1.48x0.268250

2

+0.72x0.268250

3

= 1.45096

2

x 4.96 1.96x 0.72x

c

  

= 4.96+ 1.96x0.268250+0.72x0.268250

2

=5.53758

 

 

20071 0.6

0.5

0.0133 1.9956 452.6012689 1.45096 0.78 543 0.822 581.5 5.53758

288996.2

0.5

1.45096 1

2 2

2545 - 2122 1 - 238.680281 5700 1.311178

6 6

 

 

 



 

 

 

 

 

     



  

From example 3, actual Reynolds number is 2.34E06 and f = 0.01527. Then,

R f 234000 0.01527

N

    = 289158.1

b

Q

0.0006 2.51

- 2 d

2 0.0133 1.9956 288996.2

2.51

2

log log

3.7 1.9956 288996.2

3.7 d

20071 G 20071 0.6

g

 

 

 

 

 

 

 

 



   



   







= 5.154 MMSCF / Day

Direct calculation of the gas volumetric rate in downhill flow

Combination of equation (35) in oil field units, with the Reynolds number, equation (16 )

and equation (17 ) which is the Colebrook friction factor equation, leads to:

1

1 2.51

- 2 log

3.7 d

f

 



 

 



 

… …. (39)

N 1

1

R

2.51

-2 log

3.7 d



 



 

 



 

… .… (40)

1

g

b

Q

- d R

- 2 d

2.51

av

av N

log

20071 G

3.7 d

20071 G



 

 



  

 



 

(40)

 

1

1 1 1

g

d

d av av f

20071 G

0.5

d B z T x z T x

0.5

1

J ( S Lx )

6

 

 

 



 

 

41)

e

x

2 2

J p - p 1 , if B S

2

1

6

 

  

 

 

(42a)





e

x

2 2

J p 1 - - p , if B S

1 2

6

 

(42b)

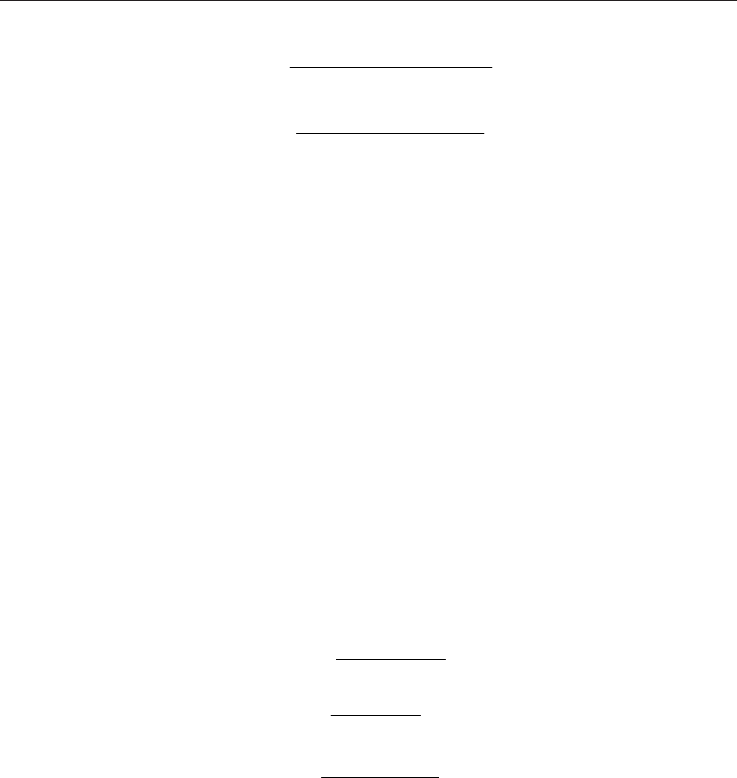
d

2 3

x 1 x 0.5x 0.3x   

2 3

x - 5.2x 2.2x - 0.6x

e

 

f

2

x 5.2 - 2.2x 0.6x  

4.191201 G L

g

B

5

d



Natural Gas462

1

2

10.03075016 G g sin p

S

z T

1





0.0375016 G

g

x

z

av

sin L

T av





z

av

and

av



are evaluated with T

av

= 0.5(T

1

+ T

2

) and p

av

= (2p

1

p

2

) / (p

1

+ p

2

)

The subscript 1 refers to surface condition and 2 to exit condition in the gas injection well.

The above equations are in oil field units in which d is expressed in inches, L in feet,

in centipoises, T in degrees rankine and pressure in pounds per square inches. In this

system of units, p

b

= 14.7 psia, T

b

= 520

R, z

b

= 1.0. The subscript 2 refers to surface

condition in the gas well.

Example 8

A gas injection well has the following data:

L = 5700ft, G

g

= 0.6, θ = 90

o

, sin90

o

= 1, z

1

= 0.78059, z

av

= 0.821, T

1

= 543

o

R,

T

av

= 543

o

R, d = 1.9956 in, p

1=

2545 psia, p

2=

2327.92 psia, absolute roughness of tubing() =

0.0006 in. Calculate the gas injection rate in MMSCF / Day. Take the viscosity of the at

surface condition(

1



) as 0.015045 cp and the average viscosity of the gas(

av



) as

0.0142 cp.

Solution

0.6 1 5700

x 0.0375016 0.26865

0.821 581.5

 

  



5

0.6 5700

B 4.191201 452.89458

1.9956



  

2

0.6 1 2545

S 0.0375016 343.83795

0.78059 543

 

  



d

2 3

x 1 x 0.5x 0.3x   

= 1 - 0.26865 + 0.5



0.26865

2

+0.36



0.26865

3

= 0.76162

e

2 3

x - 5.2x 2.2x - 0.6x 

2 3

- 5.2 0.26865 2.2 0.26865 - 0.6 0.26865    

= - 1.24982

f

2

x 5.2 - 2.2x 0.6x  

=

2

5.2 - 2.2 0.26865 0.60. 26865 4.65228

  

Since B > S,

e

x

2 2

J p - p 1 is used

2

1

6

 

 

 

 

1.24982

2 2

J 2327.92 - 2545 1 - 291372.42

6

 

 

 

 

d

1

( S Lx )

6

=

1

( 343.83795 5700 0.76162) 248780.17

6

  

0.5

1 1

av av

d f

z T x z T x

 





 

 

0.5

0.78 543 0.76162 0.821 581.5 4.65228    

= 50.43440

 

1 1 1

g

d

d av av f

20071 G

0.5

d B z T x z T x

0.5

1

J ( S Lx )

6

 

 

 



 

 

 

0.5

20071 0.6 291372.42 248780.17

0.015045 1.9956 452.89458 50.43440

 



  

274641.7

From example 5, actual Reynolds number is 2066877 and f = 0.01765. Then,

N

R f 2066877 0.01765    = 274591.4

g

b

Q

0.0006 2.51

- 2 d

2 0.0142 1.9956 274641.7

2.51

av

log log

3.7 1.9956 274641.7

3.7 d

20071 G 20071 0.6

 

 

 

 

 

 

 

 



   



   







= 5.227 MMSCF / Day

By use of an average viscosity of 0.0140 cp, the calculated Q

b

= 5.153 MMSCF / Day.

Taking 0.0140 cp as the accurate value of the average gas viscosity, the absolute error in

estimated average viscosity is (0.0002 / 0.014 = 1.43 %) . The equation for the gas volumetric

rate is very sensitive to values of the average gas viscosity. Accurate values of the average

gas viscosity should be used in the direct calculation of the gas volumetric rate.

Conclusions

1. A general differential equation that governs static behavior of any fluid and

its flow in horizontal, uphill and downhill pipes has been developed.

2.

classical fourth order Runge-Kutta numerical method is programmed in

Fortran 77, to test the equation and results are accurate. The program shows

that a length ncrement as large as 10,000 ft can be used in the Runge-Kutta

method of solution to differential equation during uphill gas flow and up to

5700ft for downhill gas flow

3.

The Runge-Kutta method was used to generate a formulas suitable to the

direct calculation of pressure transverse in static gas pipes and pipes that

transport gas uphill or downhill. The formulas yield very close results to other

tedius methods available in the literature.

Static behaviour of natural gas and its ow in pipes 463

1

2

10.03075016 G g sin p

S

z T

1





0.0375016 G

g

x

z

av

sin L

T av





z

av

and

av



are evaluated with T

av

= 0.5(T

1

+ T

2

) and p

av

= (2p

1

p

2

) / (p

1

+ p

2

)

The subscript 1 refers to surface condition and 2 to exit condition in the gas injection well.

The above equations are in oil field units in which d is expressed in inches, L in feet,

in centipoises, T in degrees rankine and pressure in pounds per square inches. In this

system of units, p

b

= 14.7 psia, T

b

= 520

R, z

b

= 1.0. The subscript 2 refers to surface

condition in the gas well.

Example 8

A gas injection well has the following data:

L = 5700ft, G

g

= 0.6, θ = 90

o

, sin90

o

= 1, z

1

= 0.78059, z

av

= 0.821, T

1

= 543

o

R,

T

av

= 543

o

R, d = 1.9956 in, p

1=

2545 psia, p

2=

2327.92 psia, absolute roughness of tubing() =

0.0006 in. Calculate the gas injection rate in MMSCF / Day. Take the viscosity of the at

surface condition(

1



) as 0.015045 cp and the average viscosity of the gas(

av



) as

0.0142 cp.

Solution

0.6 1 5700

x 0.0375016 0.26865

0.821 581.5

 

  



5

0.6 5700

B 4.191201 452.89458

1.9956



  

2

0.6 1 2545

S 0.0375016 343.83795

0.78059 543

 

  



d

2 3

x 1 x 0.5x 0.3x   

= 1 - 0.26865 + 0.5



0.26865

2

+0.36



0.26865

3

= 0.76162

e

2 3

x - 5.2x 2.2x - 0.6x 

2 3

- 5.2 0.26865 2.2 0.26865 - 0.6 0.26865    

= - 1.24982

f

2

x 5.2 - 2.2x 0.6x  

=

2

5.2 - 2.2 0.26865 0.60. 26865 4.65228

  

Since B > S,

e

x

2 2

J p - p 1 is used

2

1

6

 

 

 

 

1.24982

2 2

J 2327.92 - 2545 1 - 291372.42

6

 

 

 

 

d

1

( S Lx )

6

=

1

( 343.83795 5700 0.76162) 248780.17

6

  

0.5

1 1

av av

d f

z T x z T x

 

 

 

 

0.5

0.78 543 0.76162 0.821 581.5 4.65228    

= 50.43440

 

1 1 1

g

d

d av av f

20071 G

0.5

d B z T x z T x

0.5

1

J ( S Lx )

6

 

 

 



 

 

 

0.5

20071 0.6 291372.42 248780.17

0.015045 1.9956 452.89458 50.43440

 

 

  

274641.7

From example 5, actual Reynolds number is 2066877 and f = 0.01765. Then,

N

R f 2066877 0.01765    = 274591.4

g

b

Q

0.0006 2.51

- 2 d

2 0.0142 1.9956 274641.7

2.51

av

log log

3.7 1.9956 274641.7

3.7 d

20071 G 20071 0.6

 

 

 

 

 

 

 

 



   



   







= 5.227 MMSCF / Day

By use of an average viscosity of 0.0140 cp, the calculated Q

b

= 5.153 MMSCF / Day.

Taking 0.0140 cp as the accurate value of the average gas viscosity, the absolute error in

estimated average viscosity is (0.0002 / 0.014 = 1.43 %) . The equation for the gas volumetric

rate is very sensitive to values of the average gas viscosity. Accurate values of the average

gas viscosity should be used in the direct calculation of the gas volumetric rate.

Conclusions

1. A general differential equation that governs static behavior of any fluid and

its flow in horizontal, uphill and downhill pipes has been developed.

2.

classical fourth order Runge-Kutta numerical method is programmed in

Fortran 77, to test the equation and results are accurate. The program shows

that a length ncrement as large as 10,000 ft can be used in the Runge-Kutta

method of solution to differential equation during uphill gas flow and up to

5700ft for downhill gas flow

3.

The Runge-Kutta method was used to generate a formulas suitable to the

direct calculation of pressure transverse in static gas pipes and pipes that

transport gas uphill or downhill. The formulas yield very close results to other

tedius methods available in the literature.

Natural Gas464

4. The direct pressure transverse formulas developed are suitable for wells and

pipelines with large temperature gradients.

5.

Contribution of kinetic effect to pressure transverse in pipes that transport gas

is small and ca n be neglected..

6.

The pressure transverse formulas developed in this work are combined with

the Reynolds number and Colebrook friction factor equation to provide

accurate formulas for the direct calculation of the gas volumetric rate. The

direct calculating formulas are applicable to gas flow in uphill and downhill

pipes.

Nomenclature

p = Pressure



= Specific weight of flowing fluid

v = Average fluid velocity

g = Acceleration due to gravity in a consistent set of umits.

d



= Change in length of pipe

 = Angel of pipe inclination with the horizontal, degrees

dh

l

= Incremental pressure head loss

f = Dimensionless friction factor

L = Length of pipe

d = Internal diameter of pipe

W = Weight flow rate of fluid

C

f

= Compressibility of a fluid

C

g

= Compressibility of a gas

K = Constant for expressing the compressibility of a gas

M = Molecular weight of gas

T= Temperature

R

N

= Reynolds number



= Mass density of a fluid



=Absolute viscosity of a fluid

z = Gas deviation factor

R = Universal gas constant in a consistent set of umits.

Q

b

= Gas volumetric flow rate referred to P

b

and T

b

,



b

= specific weight of the gas at p

b

and T

b

p

b

= Base pressure, absolute unit

T

b

= Base temperature, absolute unit

z

b

= Gas deviation at p

b

and T

b

usually taken as 1

G

g

= Specific gravity of gas (air = 1) at standard condition

g



= Absolute viscosity of a gas

 = Absolute roughness of tubing

GTG = Geothermal gradient

f

2

= Moody friction factor evaluated at outlet end of pipe.

2

z = Gas deviation factor calculated with exit pressure and temperature of gas

1

z = Gas deviation factor calculated with exit pressure and temperature of gas

2

p = Pressure at exit end of pipe.

p

1

= Pressure at inlet end of pipe p

1

> p

2

T

2

= Temperature at exit end of pipe

T

1

= Temperature at inlet end of pipe

T

a v =

0.5( T

1

+ T

2

)

z

av

 Gas deviation factor evaluated with T

a v

and average pressure (p

a v

) given

by

2

a v

2

p 0.5aap  in uphill flow, and

2

1

p 0.5 aap

av

 

in downhill flow

SI Metric Conversion Factors

 

F - 32 / 18

C

ft 3.048000 *E-01 m

m 2.540 * E 00 cm

lbf 4.448222 E 00 N

lbm 4.535924 E - 01 k

g

ps

  

 

  

 

i 6.894757 E 03 Pa  

2

lb sec / ft 4.788026 E 01 Pa.s   

cp 1.0 E - 3

Pas





foot

3

/ second (ft

3

/ sec) x 2.831685 E – 02 = metre

3

/ sec ( m

3

/ s )

foot

3

/ second x 8.64 *E - 02 = MMSCF / Day

MMSCF / Day x 1.157407 E + 01 = foot

3

/ second (ft

3

/ sec)

MMSCF / Day x 3.2774132E + 01 = metre

3

/ sec ( m

3

/ s )

MMSCF / Day = 4.166667E + 04 ft

3

/hr

ft

3

/hr = 7.865792E – 06m

3

/s

* Conversion factor is exact.

References

1. Colebrook, C.F.J. (1938), Inst. Civil Engineers, 11, p 133

2. Cullender, M.H. and R.V. Smith (1956). “Practical solution of gas flow equations for wells

and pipelines with large temperature gradients”. Transactions, AIME 207, pp 281-89.

3. Giles, R.V., Cheng, L. and Evert, J (2009).. Schaum’s Outline Series of Fluid Mechanics

and Hydraulics, McGraw Hill Book Company, New York.

4. Gopal, V.N. (1977), “Gas Z-factor Equations Developed for Computer”, Oil and Gas

Journal, pp 58 – 60.

5. Ikoku, C.U. (1984), Natural Gas Production Engineering, John Wiley & Sons, New York,

pp. 317 - 346

Static behaviour of natural gas and its ow in pipes 465

4. The direct pressure transverse formulas developed are suitable for wells and

pipelines with large temperature gradients.

5.

Contribution of kinetic effect to pressure transverse in pipes that transport gas

is small and ca n be neglected..

6.

The pressure transverse formulas developed in this work are combined with

the Reynolds number and Colebrook friction factor equation to provide

accurate formulas for the direct calculation of the gas volumetric rate. The

direct calculating formulas are applicable to gas flow in uphill and downhill

pipes.

Nomenclature

p = Pressure



= Specific weight of flowing fluid

v = Average fluid velocity

g = Acceleration due to gravity in a consistent set of umits.

d



= Change in length of pipe

 = Angel of pipe inclination with the horizontal, degrees

dh

l

= Incremental pressure head loss

f = Dimensionless friction factor

L = Length of pipe

d = Internal diameter of pipe

W = Weight flow rate of fluid

C

f

= Compressibility of a fluid

C

g

= Compressibility of a gas

K = Constant for expressing the compressibility of a gas

M = Molecular weight of gas

T= Temperature

R

N

= Reynolds number



= Mass density of a fluid



=Absolute viscosity of a fluid

z = Gas deviation factor

R = Universal gas constant in a consistent set of umits.

Q

b

= Gas volumetric flow rate referred to P

b

and T

b

,



b

= specific weight of the gas at p

b

and T

b

p

b

= Base pressure, absolute unit

T

b

= Base temperature, absolute unit

z

b

= Gas deviation at p

b

and T

b

usually taken as 1

G

g

= Specific gravity of gas (air = 1) at standard condition

g



= Absolute viscosity of a gas

 = Absolute roughness of tubing

GTG = Geothermal gradient

f

2

= Moody friction factor evaluated at outlet end of pipe.

2

z = Gas deviation factor calculated with exit pressure and temperature of gas

1

z = Gas deviation factor calculated with exit pressure and temperature of gas

2

p = Pressure at exit end of pipe.

p

1

= Pressure at inlet end of pipe p

1

> p

2

T

2

= Temperature at exit end of pipe

T

1

= Temperature at inlet end of pipe

T

a v =

0.5( T

1

+ T

2

)

z

av

 Gas deviation factor evaluated with T

a v

and average pressure (p

a v

) given

by

2

a v

2

p 0.5aap  in uphill flow, and

2

1

p 0.5 aap

av

 

in downhill flow

SI Metric Conversion Factors

 

F - 32 / 18

C

ft 3.048000 *E-01 m

m 2.540 * E 00 cm

lbf 4.448222 E 00 N

lbm 4.535924 E - 01 k

g

ps

  

 

  

 

i 6.894757 E 03 Pa  

2

lb sec / ft 4.788026 E 01 Pa.s   

cp 1.0 E - 3

Pas





foot

3

/ second (ft

3

/ sec) x 2.831685 E – 02 = metre

3

/ sec ( m

3

/ s )

foot

3

/ second x 8.64 *E - 02 = MMSCF / Day

MMSCF / Day x 1.157407 E + 01 = foot

3

/ second (ft

3

/ sec)

MMSCF / Day x 3.2774132E + 01 = metre

3

/ sec ( m

3

/ s )

MMSCF / Day = 4.166667E + 04 ft

3

/hr

ft

3

/hr = 7.865792E – 06m

3

/s

* Conversion factor is exact.

References

1. Colebrook, C.F.J. (1938), Inst. Civil Engineers, 11, p 133

2. Cullender, M.H. and R.V. Smith (1956). “Practical solution of gas flow equations for wells

and pipelines with large temperature gradients”. Transactions, AIME 207, pp 281-89.

3. Giles, R.V., Cheng, L. and Evert, J (2009).. Schaum’s Outline Series of Fluid Mechanics

and Hydraulics, McGraw Hill Book Company, New York.

4. Gopal, V.N. (1977), “Gas Z-factor Equations Developed for Computer”, Oil and Gas

Journal, pp 58 – 60.

5. Ikoku, C.U. (1984), Natural Gas Production Engineering, John Wiley & Sons, New York,

pp. 317 - 346

Natural Gas466

6. Matter, L.G.S. Brar, and K. Aziz (1975), Compressibility of Natural Gases”, Journal of

Canadian Petroleum Technology”, pp. 77-80.

7. Ohirhian, P.U.(1993), “A set of Equations for Calculating the Gas Compressibility

Factor” Paper SPE 27411, Richardson, Texas, U.S.A.

8.

Ohirhian, P.U.: ”Direct Calculation of the Gas Volumeric Rates”, PetEng. Calculators,

Chemical Engineering Dept. Stanford University, California, U.S.A, 2002

9. Ohirhian, P.U. and I.N. Abu (2008), “A new Correlation for the Viscosity of Natural Gas”

Paper SPE 106391 USMS, Richardson, Texas, U.S.A.

10. Ohirhian, P.U. (2005) “Explicit Presentation of Colebrook’s friction factor equation”.

Journal of the Nigerian Association of Mathematical physics, Vol. 9, pp 325 – 330.

11. Ohirhian, P.U. (2008). “Equations for the z-factor and compressibility of Nigerian

Natural gas’’, Advances in Materials and Systems Technologies, Trans Tech

Publications Ltd, Laubisrtistr. 24, Stafa – Zurich, Switzerland..

12. Ouyang, I and K. Aziz (1996) “Steady state Gas flow in Pipes”, Journal of Petroleum

Science and Engineering, No. 14, pp. 137 – 158.

13. Standing, M.B., and D.L. Katz (1942) “Density of Natural Gases”, Trans AIME 146, pp. 140–9.

14. Standing, M.B. (1970), Volumetric and Phase Behavior of Oil Field Hydrocarbon

Systems, La Habra, California.

Steady State Compressible Fluid Flow in Porous Media 467

Steady State Compressible Fluid Flow in Porous Media

Peter Ohirhian

X

Steady State Compressible Fluid

Flow in Porous Media

Peter Ohirhian

University of Benin, Petroleum Engineering Department

Benin City, Nigeria

Introduction

Darcy showed by experimentation in 1856 that the volumetric flow rate through a porous

sand pack was proportional to the flow rate through the pack. That is:

v

/

K Q

/

K

p

d

pd

==



( i )

(Nutting, 1930) suggested that the proportionality constant in the Darcy law (K

/

) should be

replaced by another constant that depended only on the fluid property. That constant he

called permeability. Thus Darcy law became:

k v

p

d

pd



=



(ii)

Later researches, for example (Vibert, 1939) and (LeRosen, 1942) observed that the Darcy

law was restricted to laminar (viscous) flow.

(Muskat, 1949) among other later researchers suggested that the pressure in the Darcy law

should be replaced with a potential (



). The potential suggested by Muskat is:

gzp









Then Darcy law became:

g

k v

p

d

pd

- 



±=



(iii)

(Forchheimer, 1901) tried to extend the Darcy law to non laminar flow by introducing a

second term. His equation is:

20

Natural Gas468

2

v - g

k v

p

d

pd

- 



±=



( iv )

(Brinkman, 1947) tried to extend the Darcy equation to non viscous flow by adding a term

borrowed from the Navier Stokes equation. Brinkman equation takes the form:

2

p

d

v

2

d

/

g

k v

p

d

pd

-









+±=

(v)

In 2003, Belhaj et al. re- examined the equations for non viscous flow in porous media. The

authors observed that; neither the Forchheimer equation nor the Brinkman equation used

alone can accurately predict the pressure gradients encountered in non viscous flow,

through porous media. According to the authors, relying on the Brinkman equation alone

can lead to underestimation of pressure gradients, whereas using Forchheimer equation can

lead to overestimation of pressure gradients. Belhaj et al combined all the terms in the Darcy

, Forchheimer and Brinkman equations together with a new term they borrowed from the

Navier Stokes equation to form a new model. Their equation can be written as:

p

d

vdv

- g

2

v

k

v

-

2

p

d

v

2

d

p

d

pd













+=

( vi )

In this work, a cylindrical homogeneous porous medium is considered similar to a pipe. The

effective cross sectional area of the porous medium is taken as the cross sectional area of a

pipe multiplied by the porosity of the medium. With this approach the laws of fluid

mechanics can easily be applied to a porous medium. Two differential equations for gas

flow in porous media were developed. The first equation was developed by combining

Euler equation for the steady flow of any fluid with the Darcy equation; shown by

(Ohirhian, 2008) to be an incomplete expression for the lost head during laminar (viscous)

flow in porous media and the equation of continuity for a real gas. The Darcy law as

presented in the API code 27 was shown to be a special case of this differential equation. The

second equation was derived by combining the Euler equation with the a modification of

the Darcy-Weisbach equation that is known to be valid for the lost head during laminar and

non laminar flow in pipes and the equation of continuity for a real gas.

Solutions were provided to the differential equations of this work by the Runge- Kutta

algorithm. The accuracy of the first differential equation (derived by the combination of the

Darcy law, the equation of continuity for a real gas and the Euler equation) was tested by

data from the book of (Amyx et al., 1960). The book computed the permeability of a certain

porous core as 72.5 millidracy while the solution to the first equation computed it as 72.56

millidarcy. The only modification made to the Darcy- Weisbach formula (for the lost head in

a pipe) so that it could be applied to a porous medium was the replacement of the diameter

of the pipe with the product of the pipe diameter and the porosity of the medium. Thus the

solution to the second differential equation could be used for both pipe and porous

medium. The solution to the second differential equation was tested by using it to calculate

the dimensionless friction factor for a pipe (f) with data taken from the book of (Giles et al.,

2009). The book had f = 0.0205, while the solution to the second differential equation

obtained it as 0.02046. Further, the dimensionless friction factor for a certain core (f

p

)

calculated by the solution to the second differential equation plotted very well in a graph of

f

p

versus the Reynolds number for porous media that was previously generated by

(Ohirhian, 2008) through experimentation.

Development of Equations

The steps used in the development of the general differential equation for the steady flow of

gas pipes can be used to develop a general differential equation for the flow of gas in porous

media. The only difference between the cylindrical homogenous porous medium lies in the

lost head term.

The equations to be combined are;

(a) Euler equation for the steady flow of any fluid.

(b) The equation for lost head

(c) Equation of continuity for a gas.

The Euler equation is:

dp

vdv

d dh

p

l

g

sin 0







  



(1)

In equation (1), the positive sign (+) before



sind

p



corresponds to the upward direction

of the positive z coordinate and the negative sign (-) to the downward direction of the

positive z coordinate. In other words, the plus sign before



sind

p



is used for uphill flow

and the negative sign is used for downhill flow.

The Darcy-Weisbach equation as modified by (Ohirhian, 2008) (that is applicable to laminar

and non laminar flow) for the lost head in isotropic porous medium is:

c v d

p

dh

L

k

/









(2)

The (Ohirhian, 2008) equation (that is limited to laminar flow) for the lost head in an

isotropic porous medium is;

c v d

p

dh

L

d

p

32

2









(3)

Steady State Compressible Fluid Flow in Porous Media 469