Pecharsky V.K., Zavalij P.Y. Fundamentals of Powder Diffraction and Structural Characterization of Materials
Подождите немного. Документ загружается.

Fundamentals of diffraction 24
1
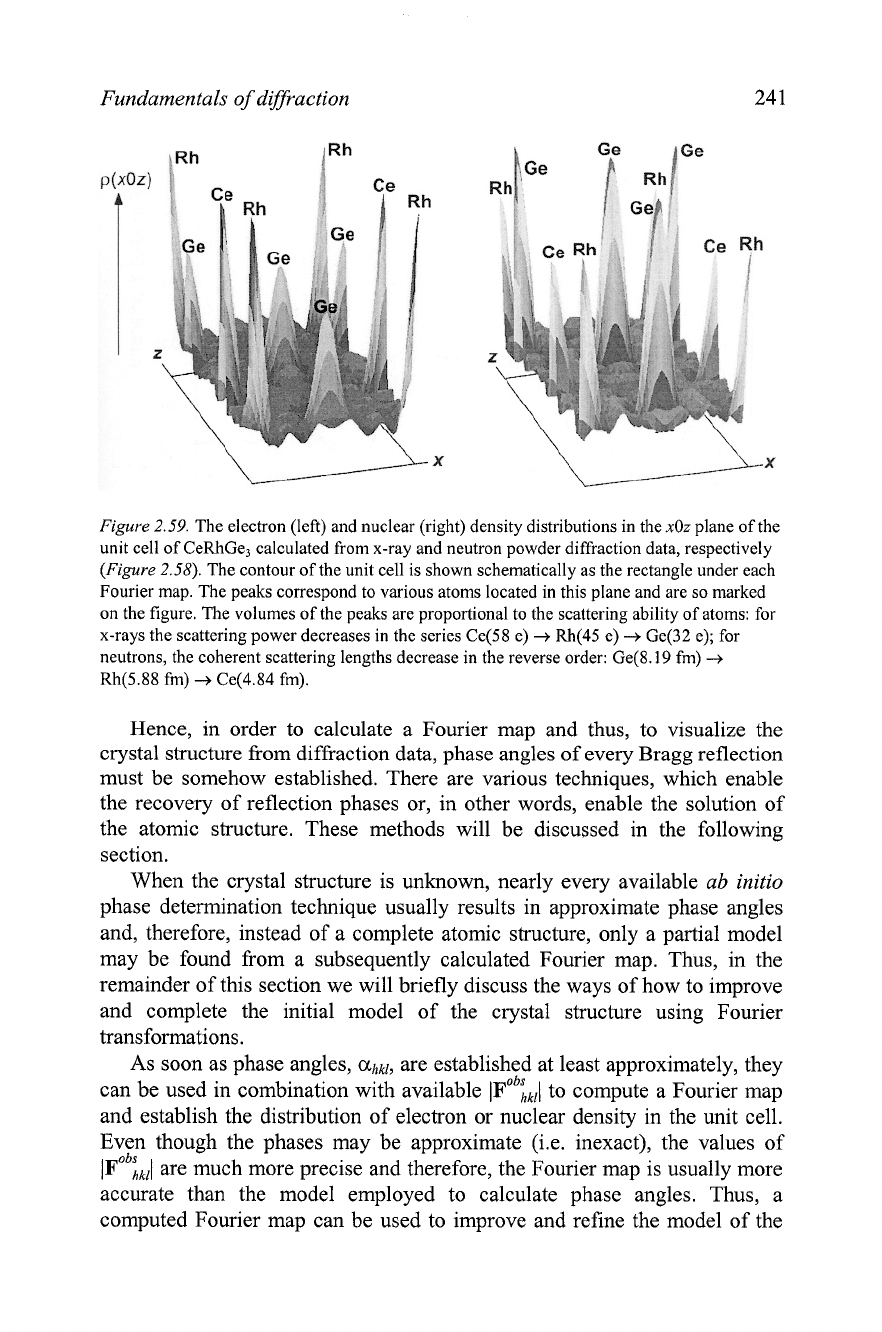
Figure 2.59. The electron (left) and nuclear (right) density distributions in the
xOz
plane of the
unit cell of CeRhGe3 calculated from x-ray and neutron powder diffraction data, respectively
(Figure 2.58). The contour of the unit cell is shown schematically as the rectangle under each
Fourier map. The peaks correspond to various atoms located in this plane and are so marked
on the figure. The volumes of the peaks are proportional to the scattering ability of atoms: for
x-rays the scattering power decreases in the series Ce(58 e)
+
Rh(45 e)
+
Ge(32 e); for
neutrons, the coherent scattering lengths decrease in the reverse order: Ge(8.19 fm)
+
Rh(5.88 fm)
+
Ce(4.84 fm).
Hence, in order to calculate a Fourier map and thus, to visualize the
crystal structure from diffraction data, phase angles of every Bragg reflection
must be somehow established. There are various techniques, which enable
the recovery of reflection phases or, in other words, enable the solution of
the atomic structure. These methods will be discussed in the following
section.
When the crystal structure is unknown, nearly every available
ab initio
phase determination technique usually results in approximate phase angles
and, therefore, instead of a complete atomic structure, only a partial model
may be found from a subsequently calculated Fourier map. Thus, in the
remainder of this section we will briefly discuss the ways of how to improve
and complete the initial model of the crystal structure using Fourier
transformations.
As soon as phase angles, ahkl, are established at least approximately, they
can be used in combination with available
IF"^",,^
to compute a Fourier map
and establish the distribution of electron or nuclear density in the unit cell.
Even though the phases may be approximate (i.e. inexact), the values of
IF"^:,^
are much more precise and therefore, the Fourier map is usually more
accurate than the model employed to calculate phase angles. Thus, a
computed Fourier map can be used to improve and refine the model of the

242
Chapter
2
crystal structure by finding coordinates of atoms with a higher precision
(when compared to the initial model) and by locating missing atoms.
If a crude initial model was used to determine approximate phases, some
atoms might not appear on the map, and these must be deleted because they
were not confirmed as a result of Fourier transformation. The new or
improved model is then used as a next level approximation to calculate the
new set of phase angles and a subsequent Fourier map must be calculated
using the new set of phases combined with experimental
/F"D",~~.
This process may be repeated as many times as needed until all atoms in
the unit cell are located and the following Fourier map(s) do not improve the
model. Equations 2.132 to 2.134 may be combined with a least squares
refinement using the observed data, which results in a more accurate model
of the crystal structure, including positional and displacement parameters of
the individual atoms already included in the model. The success in the
solution of the crystal structure is critically dependent on both the accuracy
of the initial model (initial set of phase angles) and the accuracy of the
experimental structure amplitudes. Needless to say, when the precision of
the latter is low, then the initial model should be more detailed and precise.
The effectiveness of Fourier transformation in locating missing atoms
may be improved by using the so-called difference Fourier map, which is
defined as
which is equivalent to Eq. 2.132, except that the observed complex structure
amplitudes are reduced by the complex structure amplitudes calculated from
the existing model. The resulting Fourier map, therefore, will not contain
"known" atoms and only the missing ones should become "visible". This
approach works especially well when locating weakly scattering atoms in the
presence of strong scatterers (e.g. when locating hydrogen atoms in organic
compounds) because the difference Fourier map reveals fine details, which
may be otherwise hidden in the background of the normal (Eq. 2.132) map.
The difference electron density distribution also finds an interesting
application when the fully refined model of the crystal structure is used to
compute a Fourier transformation. Although it may seem that such a Fourier
map should result in zero electron density throughout the unit cell (since the
differences in Eq. 2.135 are expected to approach zero), this is true only if
electron shells of atoms in the crystal structure were not deformed. In reality,
atoms do interact and form chemical bonds with their neighbors. This causes
a redistribution of the electron density when compared to isolated atoms, for
which atomic scattering functions are known.

Fundamentals of diffraction
243
Thus, the difference Fourier map calculated using Eq. 2.135 for a
complete and accurate model using highly precise x-ray diffraction data
reveals the so-called deformation electron density distribution. The latter is
essentially a difference between the electron density in a real crystal,
composed from chemically bound atoms, and individual isolated atoms or
ions, which sometimes enables the visualization of excess electron density
due to the formation of chemical bonds.' This technique has many
restrictions and requirements, the major of which are: extremely high
accuracy of the experimental diffraction data (including the accuracy of the
observed structure amplitudes) and availability of data at high
sinelh (often
low temperatures are required to achieve this). Unfortunately, powder
diffraction fails in the first requirement, except very simple structures, due to
the intrinsic and often unavoidable overlapping of Bragg reflections.
Other types of Fourier transformations may also be calculated when the
coefficients in Eq. 2.132 are modified or substituted. For example, when
obs
2
squared observed structure amplitudes IF
,,I
(in this case phase angles are
not required!) or normalized structure amplitudes E"~;, are used instead of
Fob",,, the resultant maps usually provide means to solve the crystal structure
(see next section). Different modifications of F"~;, may reveal the
distribution of the electrostatic potential and other properties of crystals.
2.14
Phase
problem
Despite the apparent simplicity with which a crystal structure can be
restored by applying Fourier transformation to diffraction data (Eqs. 2.132 to
2.135), the fact that the structure amplitude is a complex quantity creates the
so-called phase problem. In the simplest case (Eq. 2.133), both the absolute
values of the structure amplitudes and their phases (Eq. 2.105) are needed to
locate atoms in the unit cell. The former are relatively easily determined
from powder (Eq. 2.65) or single crystal diffraction data but the latter are
lost during the experiment.
Determination of the crystal structure of an unknown material is
generally far from a straightforward procedure, especially when only powder
diffraction data are available. It is truly a problem solving process and not a
simple refinement, which often may be fully automated. The latter is a
technique, which improves structural parameters of the approximately or
partially known model, usually by using a least squares minimization against
available diffraction data. It is worth noting that the least squares method is
'
See
V.G.
Tsirelson and
R.P.
Ozerov, Electron density and bonding in crystals: principles,
theory and x-ray diffraction experiments in solid state physics and chemistry, Institute of
Physics, Bristol,
UK
(1996); P. Coppens, X-ray charge densities and chemical bonding.
IUCr Texts on Crystallography
4,
Oxford University Press, Oxford, New York (1997)

Chapter
2
inapplicable to the
ab
initio
structure solution because the structure
amplitude is a non-linear function of multiple individual atomic parameters
(Eq.
2.107).
Thus, during the crystal structure solving process phase angles,
which have been lost, must be recovered using suitable numerical technique.
A
large variety of methods, developed with a specific goal to solve the
crystal structure from diffraction data, can be divided into two major groups.
The first group entails techniques that are applicable in direct space by
constructing a model of the crystal structure from considerations other than
the available array of structure amplitudes. These include:
-
Purely geometrical modeling in the case of simple inorganic structures.
-
Examining various ways of packing and differences in conformations of
molecules with known geometry when dealing with molecular structures.
-
Finding analogies with closely related compounds, such as isostructural
series of intermetallics and partially isostructural host frameworks in
various intercalates.
-
Using a range of minimization methods, including quantum-chemical,
energy, entropy and geometry optimizations, and other recently
developed advanced techniques.
When one or more models are constructed, they are tested against the
experimental diffraction data. Often some of these approaches are combined
together but they always stem from the requirement that the generated model
must make physical, chemical and crystallographic sense. Thus, their
successful utilization requires a certain level of experience and knowledge of
how different classes of crystals are built, e.g. what to expect in terms of
coordination and bond lengths for a particular material based solely on its
chemical composition. Direct space modeling approaches will be discussed,
to some extent, in Chapter
6.
The second group of methods uses an experimental array of diffraction
data, i.e. the absolute values of structure amplitudes, to provide initial clues
about the crystal structure of a material. Hence, they are applicable in the
reciprocal space. The first of the two reciprocal space methods, reviewed in
this section, is the Patterson technique, which is best known for its
applications in the so-called heavy atom method. Furthermore, as we will see
below, even though the phase angles of Bragg reflections are not directly
observed or measured, they are usually in certain relationships with one
another and with the absolute values of structure amplitudes. This property
supports a second reciprocal space approach, the so-called direct phase
determination techniques. The latter are always referred in a plural form
because they are based on several basic principles and usually contain
several different algorithms combined together. Needless to say, the crystal
structure determined using any of the reciprocal space methods should also
be reasonable from physical, chemical and crystallographic points of view.

Fundamentals of diffraction
245
2.14.1
Patterson technique
As suggested by Patterson' in 1934, the complex coefficients in the
forward Fourier transformation (Eqs. 2.129 and 2.132) may be substituted by
the squares of structure amplitudes, which are real, and therefore, no
information about phase angles is required to calculate the distribution of the
following density function in the unit cell:
Here, the multiplier 2 appears because only one-half of a reciprocal space is
used in the summation, thus the validity of Friedel's law is implicitly
assumed.
The resultant function, unfortunately, does not reveal the distribution of
atoms in the unit cell directly but it represents the distribution of interatomic
vectors, all of which begin in a common point
-
the origin of the unit cell.
Thus,
P,,,
is often called the function of interatomic vectors and it is also
known as the Patterson function of the ~~-~ourier series. The corresponding
vector density distribution in the unit cell is known as the Patterson map.
The interpretation of the Patterson function is based on a specific
property of Fourier transformation (denoted as
3[
...I)
when it is applied to
convolutions
(8)
of functions:
As follows from Eq. 2.137, the multiplication of functions in the
reciprocal space (e.g. structure amplitudes) results in a convolution of
functions (e.g. electron or nuclear density) in the direct space, and
vice
versa.
Since Eq. 2.136 contains the structure amplitude multiplied by itself,
the resultant Patterson function, P,,, represents a self-convolution of the
electron (nuclear) density. Hence, it may be described as follows:
'
A.L.
Patterson,
A
Fourier series representation of the average distribution of the scattering
power in crystals, Phys. Rev. 45,763
(1934),
A.L.
Patterson,
A
Fourier series method for
the determination of the components of the interatomic distances in crystals, Phys. Rev.
46,372 (1934).
246 Chapter 2
where
P,,,
in every point (u, v, w) inside the unit cell is calculated as the
sum (integral) of products of the electron (nuclear) density at two points
separated by a vector (u, v, w).
For simplicity, assume that the distribution of electron or nuclear density
in the unit cell is discrete rather than continuous and is zero everywhere
except for the locations of atoms, viewed as dimensionless points (see
Figure 2.59, which illustrates that both electron and nuclear density
decreases rapidly away from the centers of atoms). Then, the result of
Eq.
2.138 is a set of peaks originating in (0, 0,
0)
and ending at (u, v, w) with
heights (more precisely with peak volumes since atoms are not
dimensionless points),
Hy,
given as
where
Zi
and
Zj
are the number of electrons (or scattering lengths) of the ith
and
jth
atoms that are connected
by
a vector (u, v, w) defined as
u
=
+[xi
-
xj].
According to this interpretation of
Eq.
2.138, the value of the Patterson
function is zero at any other point in the unit cell.
An
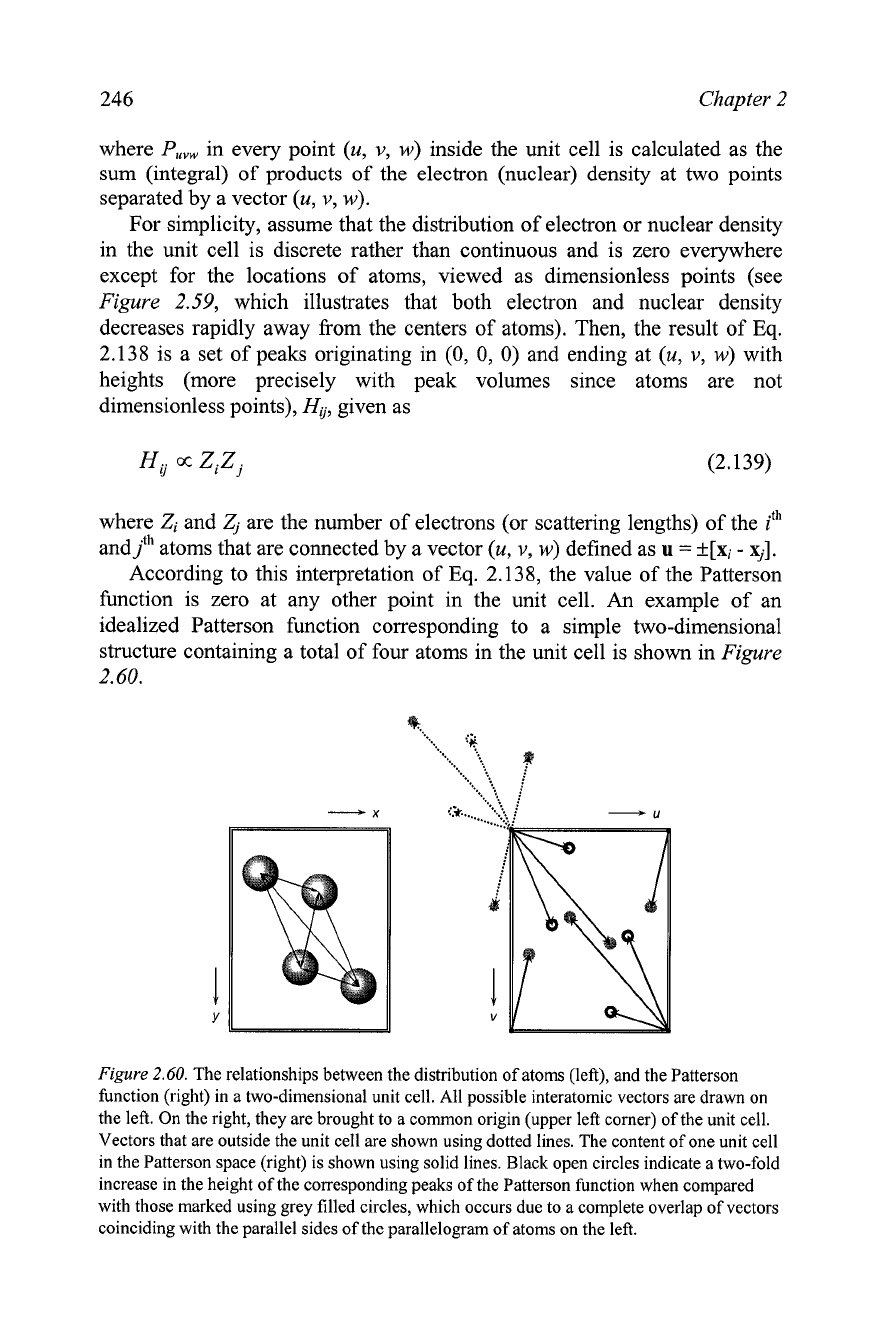
example of an
idealized Patterson function corresponding to a simple two-dimensional
structure containing a total of four atoms in the unit cell is shown in Figure
Figure
2.60.
The relationships between the distribution of atoms (left), and the Patterson
function (right) in a two-dimensional unit cell. All possible interatomic vectors are drawn on
the left. On the right, they are brought to a common origin (upper left corner) of the unit cell.
Vectors that are outside the unit cell are shown using dotted lines. The content of one unit cell
in the Patterson space (right) is shown using solid lines. Black open circles indicate a two-fold
increase in the height of the corresponding peaks of the Patterson function when compared
with those marked using grey filled circles, which occurs due to a complete overlap of vectors
coinciding with the parallel sides of the parallelogram of atoms on the left.
Fundamentals
of
diffraction
247
Thus, since a Patterson map contains peaks, which are related to the real
distribution of atoms in the unit cell, it is possible to establish both the
coordinates of atoms and their scattering power by analyzing coordinates
and heights of Patterson peaks. Unfortunately, the analysis of the distribution
of interatomic vector density function is sometimes easier said than done due
to the presence of several complicating factors.
The first difficulty is that Patterson peaks are usually broader than
electron (nuclear) density peaks, which is the result of convolution (Eq.
2.138). The second complication is that the total number of Patterson peaks
in the unit cell equals to n(n-1), where n is the total number of atoms in the
unit cell (see Figure 2.60 where four atoms shown on the left produce
12
Patterson peaks shown on the right, four pairs of which are completely
overlapped). The third difficulty is derivative of the first two and it arises
from overlapping (often quite substantial) of different interatomic vectors.
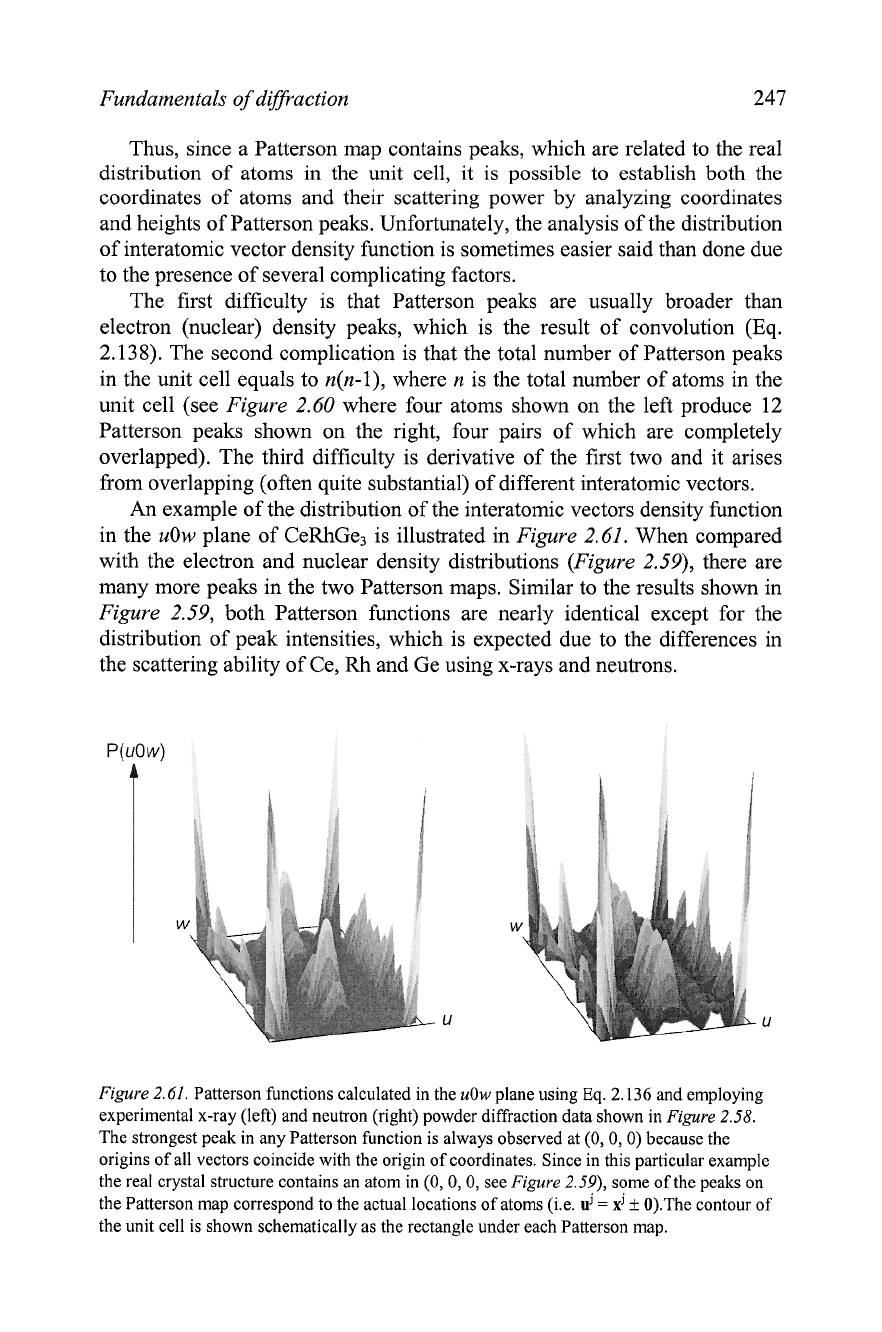
An
example of the distribution of the interatomic vectors density function
in the uOw plane of CeRhGe3 is illustrated in Figure
2.61.
When compared
with the electron and nuclear density distributions (Figure 2.59), there are
many more peaks in the two Patterson maps. Similar to the results shown in
Figure 2.59, both Patterson functions are nearly identical except for the
distribution of peak intensities, which is expected due to the differences in
the scattering ability of Ce,
Rh
and Ge using x-rays and neutrons.
Figure
2.61. Patterson functions calculated in the uOw plane using
Eq. 2.136
and employing
experimental x-ray (left) and neutron (right) powder diffraction data shown in
Figure
2.58.
The strongest peak in any Patterson function is always observed at (0, 0, 0) because the
origins of all vectors coincide with the origin of coordinates. Since in this particular example
the real crystal structure contains an atom in (O,0,0, see
Figure
2.59), some of the peaks on
. .
the Patterson map correspond to the actual locations of atoms (i.e.
uJ
=
xJ
rt
O).The contour of
the unit cell is shown schematically as the rectangle under each Patterson map.
248
Chapter
2
The complicating factors mentioned above, reduce the resolution of the
Patterson map, which may make it extremely difficult or impossible to
recover the atomic structure, especially in cases of complex crystal structures
containing many atoms with nearly equal atomic numbers, e.g. organic
compounds. On the other hand, when only a few atoms in the unit cell have
much stronger scattering ability than the rest, the identification of Patterson
peaks corresponding to these strong scatterers is relatively easy: according to
Eq. 2.139, these peaks will be stronger than all others, except for the peak at
the origin.
The application of the Patterson technique to locate strongly scattering
atoms is often called the heavy atom method (which comes from the fact that
heavy atoms scatter x-rays better and the Patterson technique is most often
applied to analyze x-ray diffraction data). This allows constructing of a
partial structure model ("heavy7' atoms only), which for the most part define
phase angles of all reflections (see Eq. 2.107). The "heavy atoms-only"
model can be relatively easily completed using sequential Fourier syntheses
(either or both standard, Eq. 2.133, and difference, Eq. 2.135), sometimes
enhanced by a least squares refinement of all found atoms.
The analysis of the Patterson hnction requires extensive use of
symmetry. Consider all possible interatomic vectors (calculated as uii
=
f
[xi
-
xj]) originating from an-atom in the general site position of the space
P2Jm, which are listed in
Table
2.18.
Only three of the vectors (shaded in
the first row of the table) are unique, and the relationships between them are
established by the combination of symmetry elements in the unit cell.
Table
2.18.
Interatomic vectors (shown in bold) produced by an atom located in the general
site position in the monoclinic crystal system, space group P2,Im.
Symmetry Symmetry
element
I
ooeration
I
XYJ
Thus, a vector 2x, 1/2,2z is the result of a 21 screw axis parallel to the
b-
axis, and when the former is correctly identified on the Patterson map, the
two coordinates,
x
and z, of the corresponding atom are found.
A
second
vector
-
0, -112-2y, 0
-
is due to a mirror plane and it yields the missing
coordinate
y,
while a vector 2x, 2y, 22 is due to a center of inversion, which
in this case could be used to confirm all three coordinates.
When a structure contains only a single independent heavy atom, the
solution is nearly always trivial. It may be possible to solve
a
structure with
two to four independent heavy atoms manually, even though the task

Fundamentals
of
diffraction 249
becomes much more challenging. Solving a structure from a Patterson map
in the case of more complex crystal structures is usually performed using
computer programs.
A
more detailed analysis of Table
2.18
indicates that symmetry of the
Patterson function is different from that of the original crystal structure. A
comprehensive examination of Patterson symmetry exceeds the scope of this
book. It can be shown, however, that symmetry elements with a translational
component, present in the space of the crystal structure, are transformed into
conforming finite symmetry elements in the Patterson function space except
for the lattice translations, which are preserved. Thus, screw axes become
rotation axes and glide planes are transformed into mirror planes. Moreover,
a center of inversion is always added to the symmetry of Patterson function.
For example, space group
P2,Im discussed above results in Patterson
symmetry corresponding to space group P2lm; 142m is transformed into
I4/mmm, Fdd2 turns into Fmmm, and so on. A complete list of Patterson
function symmetry for all space groups can be found in the International
Tables for Crystallography, vol.
A.
2.14.2
Direct methods
In
this approach, the phase angles of reflections are derived directly from
the observed structure amplitudes through mathematical relationships
between intensities and indices of the reflections. The relationships are based
on the following postulations:
1.
The electron density is non-negative anywhere in the unit cell, i.e.
p,,,
2
0
for all
x,
y,
z.
2. The atomic structure is composed from nearly spherical atoms spread
nearly evenly throughout the unit cell volume.
These two general properties of the electron density result in special
relationships between phase angles of triplets of reflections, which have
arithmetically (but not symmetrically) related indices. The triplets of related
reflections are defined as follows
First reflection,
h:
h, k, I
Second reflection,
h':
h', k', 1'
Third reflection,
h-h':
h-h',
k-k', 2-1'
The phase relationships within a triplet are not strict and their probability
depends on the magnitude of the associated structure amplitudes. The latter
are scaled and normalized in order to reduce their dependence on the atomic
scattering factor and vibrational motion since both reduce the structure
amplitude exponentially at high
sinelh, see
Eq.
2.91, Figure
2.53
and Figure

250 Chapter
2
2.55.
The normalized structure factor is commonly denoted as Ehkl and it is
calculated from the conventional structure factor as follows:
where the expected
estimated as:
(2.141)
average value of the structure factor,
<F',,>, is
and A(s) is the atomic scattering factor of the
jth
atom;
s
is sinelh of the
reflection (hkl).
In
the centrosymmetric structures, the relationships between the signs of
the reflections forming
a
triplet
(Eq.
2.140) are described by the Sayre
equation:'
where s
=
+1
when
ahk,
=
0
(positive Fhkl),
s
=
-1 when
ahkl=
.n
(negative
Fhkl), and the symbol
=
has a meaning of "probably equal", for example,
s123
=
s102s021.
The probability of this sign relationship in the triplet is defined as:
where
P+
is the probability of the positive sign for a reflection
h,
and:
N-"'
=
a,
lo:"
and
o,
=
z,!"
Here
Z
is the atomic number and
n
is the number of atoms in the unit cell.
In
the non-centrosymmetric structures, reflection phases in the triplet are
in the following relationship, also given by sayre:'
D.
Sayre, The squaring method: a new method for phase determination, Acta Cryst.
5,
60
(1952).
