Myint Tyn U., Debnath L. Linear Partial Differential Equations for Scientists and Engineers

Подождите немного. Документ загружается.

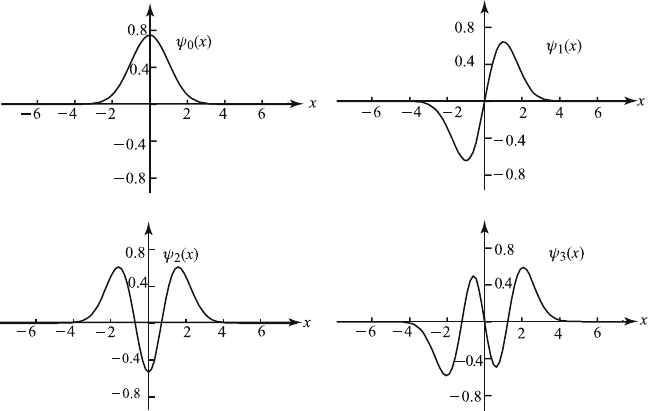
8.14 Exercises 321

Figure 8.13.1 Eigenfunctions ψ

for n =0, 1, 2, 3.

8.14 Exercises

1. Determine the eigenvalues and eigenfunctions of the following regular

Sturm–Liouville systems:

(a) y

′′

+ λy =0,

y (0) = 0,y(π)=0.

(b) y

′′

+ λy =0,

y (0) = 0,y

′

(1) = 0.

′′

+ λy =0,

′

(0) = 0,y

′

(π)=0.

(d) y

′′

+ λy =0,

y (1) = 0,y(0) + y

′

(0) = 0.

322 8 Eigenvalue Problems and Special Functions

2. Find the eigenvalues and eigenfunctions of the following periodic Sturm–

Liouville systems:

(a) y

′′

+ λy =0,

y (−1) = y (−1) ,y

′

(−1) = y

′

(1).

(b) y

′′

+ λy =0,

y (0) = y (2π) ,y

′

(0) = y

′

(2π).

′′

+ λy =0,

y (0) = y (π) ,y

′

(0) = y

′

(π).

3. Obtain the eigenvalues and eigenfunctions of the following Sturm–

Liouville systems:

(a) y

′′

+ y

′

+(1+λ) y =0,

y (0) = 0,y(1) = 0.

(b) y

′′

+2y

′

+(1− λ) y =0,

y (0) = 0,y

′

(1) = 0.

′′

− 3y

′

+ 3 (1 + λ) y =0,

′

(0) = 0,y

′

(π)=0.

4. Find the eigenvalues and eigenfunctions of the following regular Sturm–

Liouville systems:

(a) x

′′

+3xy

′

+ λy =0, 1 ≤ x ≤ e,

y (1) = 0,y(e)=0.

(b)

(2 + x)

′

+ λy =0, −1 ≤ x ≤ 1,

y (−1) = 0,y(1) = 0.

′′

+ 2 (1 + x) y

′

+3λy =0, 0 ≤ x ≤ 1,

y (0) = 0,y(1) = 0.

8.14 Exercises 323

5. Determine all eigenvalues and eigenfunctions of the Sturm–Liouville

systems:

(a) x

′′

+ xy

′

+ λy =0,

y (1) = 0,y,y

′

are bounded at x =0.

(b) y

′′

+ λy =0,

y (0) = 0,y,y

′

are bounded at inﬁnity.

6. Expand the function

f (x)=sinx, 0 ≤ x ≤ π

in terms of the eigenfunctions of the Sturm–Liou ville problem

′′

+ λy =0,

y (0) = 0,y(π)+y

′

(π)=0.

7. Find the expansion of

f (x)=x, 0 ≤ x ≤ π

in a series of eigenfunctions of the Sturm–Liouvil le system

′′

+ λy =0,

′

(0) = 0,y

′

(π)=0.

8. Transform each of the following equations into the equivalent self-

adjoint form:

(a) The Laguerre equation

′′

+(1− x) y

′

+ ny =0,n=0, 1, 2,....

(b) The Hermite equation

′′

− 2xy

′

+2ny =0,n=0, 1, 2,....



1 − x



′′

− xy

′

+ n

y =0,n=0, 1, 2,....

9. If q (x)ands (x) are continuous and p (x) is twice continuously diﬀer-

entiable in [a, b], show that the solutions of the fourth-order Sturm–

Liouville system

324 8 Eigenvalue Problems and Special Functions

[p (x) y

′′

]

′′

+[q (x)+λs (x)] y =0,

y + a

(py

′′

)

′

x=a

=0,

y + b

(py

′′

)

′

x=b

=0,

′

+ c

(py

′′

)]

x=a

=0, [d

′

+ d

(py

′′

)]

x=b

=0,

where a

+ a

=0,b

+ b

=0,c

+ c

=0,d

+ d

=0,

are orthogonal with respect to s (x)in[a, b].

10. If the eigenfunctions of th e problem

(ry

′

)+λy =0, 0 <r<a,

y (a)+c

′

(a)=0,

lim

r→0+

y (r ) < ∞,

satisfy

lim

r→0+

′

(r)=0,

show that all the eigenvalues are real for real c

and c

11. Find the Green’s function for each of the following problems:

(a) L [y]=y

′′

=0,

y (0) = 0,y

′

(1) = 0.

(b) L [y]=



1 − x



′′

− 2xy

′

=0,

y (0) = 0,y

′

(1) = 0.

′′

+ a

y =0,a= constant,

y (0) = 0,y(1) = 0.

12. Determine the solution of each of the following b ou nd ary-value prob-

lems:

(a) y

′′

+ y =1,

y (0) = 0,y(1) = 0.

8.14 Exercises 325

(b) y

′′

+4y = e

y (0) = 0,y

′

(1) = 0.

′′

=sinx,

y (0) = 0,y(1) + 2y

′

(1) = 0.

(d) y

′′

+4y = −2,

y (0) = 0,y





=0.

(e) y

′′

= −x,

y (0) = 2,y(1) + y

′

(1) = 4.

(f) y

′′

= −x

y (0) + y

′

(0) = 4,y

′

(1) = 2.

(g) y

′′

= −x,

y (0) = 1,y

′

(1) = 2.

13. Determine the solution of th e following boundary-value problems:

(a) y

′′

= −f (x) ,y(0) = 0,y

′

(1) = 0.

(b) y

′′

= −f (x) ,y(−1) = 0,y(1) = 0.

14. Find the solution of the following boundary-value problems:

(a) y

′′

− y = −f (x) ,y(0) = y (1) = 0.

(b) y

′′

− y = −f (x) ,y

′

(0) = y

′

(1) = 0.

15. Show that the Green’s function G (t, ξ) for the forced harmonic oscilla-

tor described by initial-value problem

¨x + ω

x =





sin Ωt,

x (0) = a, ˙x (0) = 0,

326 8 Eigenvalue Problems and Special Functions

G (t, ξ)=

sin ω (t − ξ) .

Hence, the particular solution is

(t)=

mω



sin ω (t − ξ)sin(Ωξ) dξ.

16. Determine the Green’s function for the boundary-value problem

′′

+ y

′

= −f (x) ,

y (1) = 0, lim

x→0

|y (x)| < ∞.

17. Determine the Green’s function for the boundary-value problem

′′

+ y

′

−

y = −f (x) ,

y (1) = 0, lim

x→0

|y (x)| < ∞.

18. Determine the Green’s function for the boundary-value problem



1 − x



′

−

(1 − x

)

y = −f (x) ,h=1, 2, 3,...,

lim

r→+

|y (x)| < ∞.

19. Prove the uniqueness of the Green’s function for the boundary-value

problem

L [y]=−f (x) ,

y (a)+a

′

(a)=0,

y (b)+b

′

(b)=0.

20. Find the Green’s function for the boundary-value problem

L [y]=y

(iv)

= −f (x) ,

y (0) = y (1) = y

′

(0) = y

′

(1) = 0.

Prove that the homogeneous problem has a trivial solution only, and

prove that the nonhomogeneous problem has a unique solution.

8.14 Exercises 327

21. Determine the Green’s function for the boundary-value problem

′′

= −f (x) ,y(−1) = y (1) ,y

′

(−1) = y

′

(1) .

22. Consider the nonself-adjoint boundary-value problem

L [y]=y

′′

+3y

′

+2y = −f (x) ,

2 y (0) − y (1) = 0,y

′

(1) = 2.

By direct int egration of GL [y] from 0 to 1, show that

y (x)=−2 G (1,x) −



G (x, ξ) f (ξ) dξ

is the solution of the boundary-value problem, if G satisﬁes the system

ξξ

− 3G

+2G =0,ξ= x,

G (0,x)=0,

6 G (1,x) − 2 G

(1,x)+G

(0,x)=0.

Find the Green’s function G (x , ξ).

23. Show that

dG (x, ξ)



ξ=x+

ξ=x−

p (x)

is equivalent to

dG (x, ξ)



x=ξ+

x=ξ−

= −

p (ξ)

24. (a) Apply the Pr¨ufer transformation

= y

+ p

′

)

,θ= tan

−1



′



to transform the Sturm–Liouville equation (8.1.3) into the ﬁrst order

nonlinear equation in the form



− q − λr



sin 2θ,

dθ

=(q + λr)sin

θ +

cos

θ,

where a<x<b.

(b) Draw the direction ﬁeld



dθ



in the (θ, x)planewithp = x, q = −

and r = x. Hence draw the solution curves of the θ-equation for diﬀerent

λ with data θ (a)=α and θ (b) is arbitrary.

Boundary-Value Problems and Applicat io ns

“The enormous usefulness of mathematics in the natural sciences is some-

thing bordering on the mysterious and there is no rational explanation for

it. It is not at all natural that “laws of nature” exist, much less that man is

able to discover them. The miracle of the appropriateness of the language

of mathematics for the formulation of the laws of physics is a wonderful gift

which we neither understand nor deserve.”

Eugene Wigner

9.1 Boundary-Value Problems

In the preceding chapters, we have treated the initial-value and initial

boundary-value problems. In this chapter, we shall be concerned with

boundary-value problems. Mathematically, a boundary-value problem is

ﬁnding a function which satisﬁes a given partial diﬀerential equation and

particular boundary conditions. Physically speaking, the problem is inde-

pendent of time, involving only space co or din ates. Just as initial-value prob-

lems are associated with hyperbolic partial d i ﬀerential equations, boundary-

value problems are associated with partial diﬀerential equations of elliptic

type. In marked contrast to initial-value problems, boundary-value prob-

lems are considerably more diﬃcult to solve. This is due to the physical

requirement that solutions must hold in the large unlike the case of initial-

value problems, where solutions in the small, say over a short interval of

time, may still be of physical interest.

The second-order partial diﬀerential equation of the elliptic type in n

independent variables x

, x

, ..., x

is of the form

▽

u = F (x

...,x

,...,u

) , (9.1.1)

where

330 9 Boundary-Value Problems and Applications

▽

u =



i=1

Some well-known elliptic equations include

A. Laplace equation

▽

u =0. (9.1.2)

B. Poisson equation

▽

u = g (x) , (9.1.3)

where

g (x)=g (x

,...,x

) .

C. Helmholtz equation

▽

u + λu =0, (9.1.4)

where λ is a positive constant

D. Schr¨odinger equation (time independent)

▽

u +[λ − q (x)] u =0. (9.1.5)

We shall not attempt to treat general elliptic partial diﬀerential equa-

tions. Instead, we shall begin by presenting the simplest boundary-value

problems for the Laplace equation in two dimensions.

We ﬁrst deﬁne a harmonic function. A fu nction is said to be harmonic

in a domain D if it satisﬁes the Laplace equation and if it and its ﬁrst two

derivatives are continuous in D.

We may note here that, since the Laplace equation is linear and homo-

geneous, a linear combination of harmonic functions is harmonic.

1. The First Boundary-Value Problem

The Dirichlet Problem: Find a function u(x, y), harmonic in D, which sat-

isﬁes

u = f (s)onB, (9.1.6)

where f (s) is a prescribed continuous function on the boundary B of the

domain D. D is the interior of a simple closed piecewise smooth curve B.

We may physically interpret the solution u of the Dirichlet problem as

the steady-state temperature distribution in a body containing no sources or

sinks of heat, with the temperature prescribed at all points on the boundary.