Moran M.J., Shapiro H.N. Fundamentals of Engineering Thermodynamics

Подождите немного. Документ загружается.

7.5 Exergy Rate Balance for Control Volumes at Steady State 383

Analysis:

(a)

The temperature T

of the exiting combustion gases can be found by reducing the mass and energy rate bal-

ances for the control volume at steady state to obtain

0 5 Q

2 W

1 m

2 h

2 V

1 g1z

2 z

1 m

2 h

2 V

1 g1z

2 z

where m

is the common mass flow rate of the two streams. The underlined terms drop out by listed assumptions,

leaving

0 5 m

2 h

1 m

2 h

Dividing by m

and solving for h

5 h

1 h

2 h

From Table A-22, h

5 617.53 kJ/kg, h

5 888.27 kJ/kg, h

5 1068.89 kJ/kg. Inserting values

5 1068.89 1 617.53 2 888.27 5 798.15 kJ

Interpolating in Table A-22 gives T

5 778 K (5058C).

(b) The net change in the flow exergy rate from inlet to exit for the air stream flowing from 1 to 2 can

be evaluated using Eq. 7.18, neglecting the effects of motion and gravity. With Eq. 6.20a and data from

Table A-22

2 e

5 m

2 h

2 T

2 s

5 m

2 h

22 T

82s

82R ln

5 90

c1888.27 2 617

.532

2 300 K a2.79783 2 2.42644 2

8.314

28.97

9.7

? K

5 14,103

1 MW

5 14.1 MW

Engineering Model:

The control volume shown in the accompanying

figure is at steady state.

2. For the control volume, Q

5 0, W

5 0, and the

effects of motion and gravity are negligible.

3. Each stream has the properties of air modeled as an

ideal gas.

4. T

5 300 K, p

5 1 bar.

= 860 K

= 9.7 bar

= 610 K

= 10 bar

= 1020 K

= 1.1 bar

ΔT

ave

= 1 bar

Compressor

Air

Turbine

Fuel

Combustion

gases

Combustor

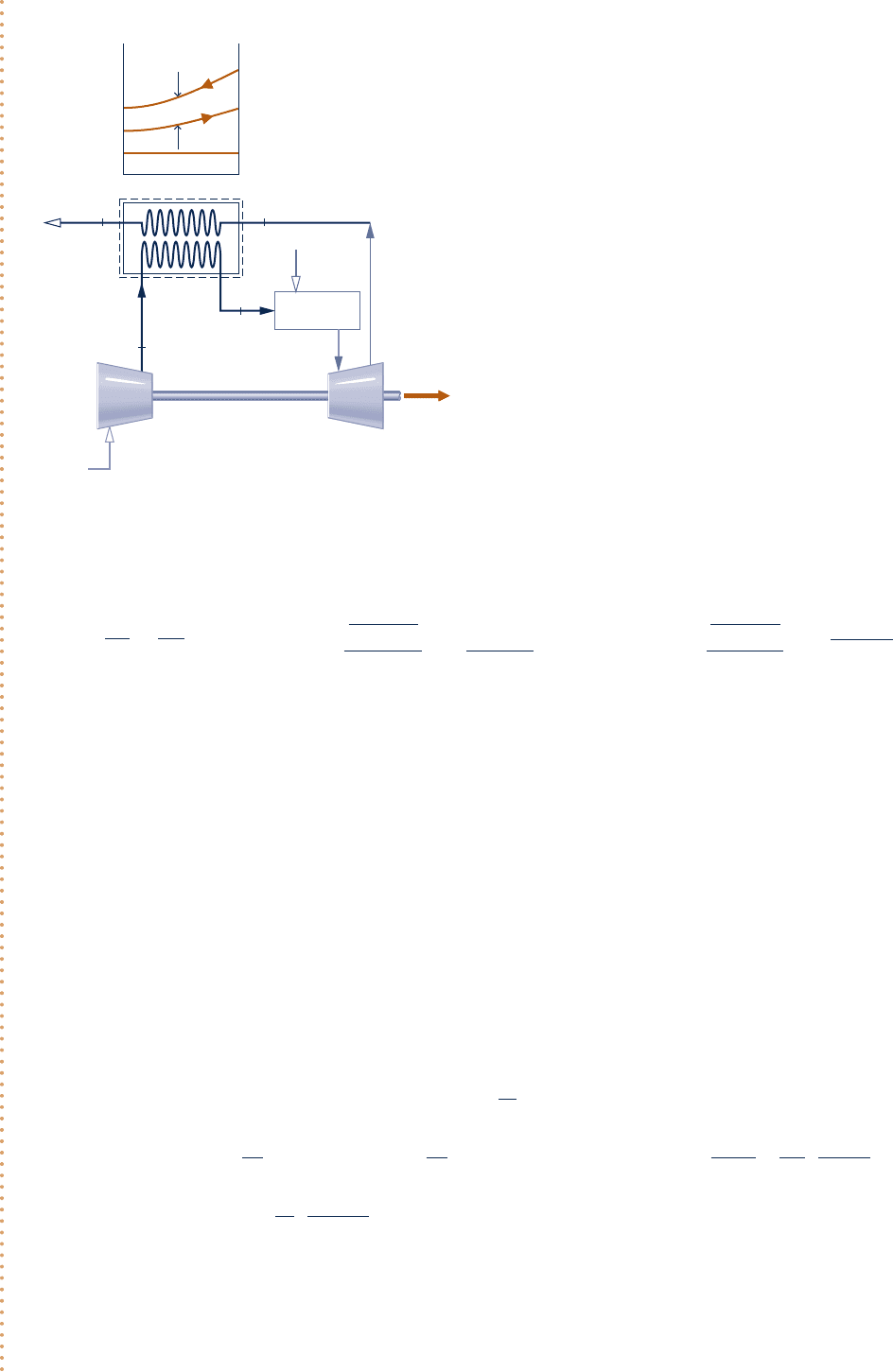
Fig. E7.6

Schematic and Given Data:

c07ExergyAnalysis.indd Page 383 7/12/10 6:52:47 AM user-s146c07ExergyAnalysis.indd Page 383 7/12/10 6:52:47 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

384 Chapter 7 Exergy Analysis

➋ As the air flows from 1 to 2, its temperature increases relative to T

and the flow exergy increases.

Similarly, the change in the flow exergy rate from inlet to exit for the combustion gas is

2 e

25 m

2 h

22 T

82s

82R ln

5 90

1798.15 2 1068.8922 300

2.68769 2 2.99034 2

8.314

28.97

1.1

5216,934

1 MW

5216.93 MW

As the combustion gas flows from 3 to 4, its temperature decreases relative to T

and the flow exergy

decreases.

➌ (c) The rate of exergy destruction within the control volume can be determined from an exergy rate balance,

Eq. 7.13a,

0 5

a1 2

2 W

1 m

2 e

21 m

2 e

22 E

Solving for E

and inserting known values

5 m

2 e

1 m

2 e

➍ 5

214.1 MW

16.93 MW

5 2.83 MW

Comparing results, the exergy increase of the compressed air: 14.1 MW is less than the magnitude of the exergy

decrease of the combustion gas: 16.93 MW, even though the energy changes of the two streams are equal in magnitude.

The difference in these exergy values is the exergy destroyed: 2.83 MW. Thus, energy is conserved but exergy is not.

➊ Heat exchangers of this type are known as regenerators (see Sec. 9.7).

➋ The variation in temperature of each stream passing through the heat exchanger is sketched in the schematic.

The dead state temperature T

also is shown on the schematic for reference.

➌ Alternatively, the rate of exergy destruction can be determined using

5 T

, where s

is the rate of entropy production evaluated from an

entropy rate balance. This is left as an exercise.

➍ Exergy is destroyed by irreversibilities associated with fluid friction and

stream-to-stream heat transfer. The pressure drops for the streams are indi-

cators of frictional irreversibility. The average temperature difference between

the streams, ¢T

ave

, is an indicator of heat transfer irreversibility.

If the mass flow rate of each stream were 105 kg/s, what would

be the rate of exergy destruction, in MW? Ans. 3.3 MW.

Ability to…

❑

apply the energy and exergy

rate balances.

❑

evaluate exergy destruction.

✓

Skills Developed

Determining Cost of Exergy Destruction

c c c c EXAMPLE 7.7 c

For the heat pump of Examples 6.8 and 6.14, determine the exergy destruction rates, each in kW, for the com-

pressor, condenser, and throttling valve. If exergy is valued at $0.08 per kW

h, determine the daily cost of

electricity to operate the compressor and the daily cost of exergy destruction in each component. Let T

5 273 K

(08C), which corresponds to the temperature of the outside air.

In previous discussions we have noted the effect of irreversibilities on thermody-

namic performance. Some economic consequences of irreversibilities are considered

in the next example.

c07ExergyAnalysis.indd Page 384 7/12/10 6:52:49 AM user-s146c07ExergyAnalysis.indd Page 384 7/12/10 6:52:49 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

SOLUTION

Known:

Refrigerant 22 is compressed adiabatically, condensed by heat transfer to air passing through a heat

exchanger, and then expanded through a throttling valve. Data for the refrigerant and air are known.

Find: Determine the daily cost to operate the compressor. Also determine the exergy destruction rates and

associated daily costs for the compressor, condenser, and throttling valve.

Schematic and Given Data:

See Examples 6.8 and 6.14.

Engineering Model:

See Examples 6.8 and 6.14.

2. T

5 273 K (08C).

Analysis: The rates of exergy destruction can be calculated using

5 T

together with data for the entropy production rates from Example 6.8. That is,

comp

5 1273 K2117.5 3 10

5 0.478 kW

valve

273

9.94 3 10

5 0.271 kW

cond

273

7.95 3 10

5 0.217 kW

The costs of exergy destruction are, respectively

daily cost of exergy destruction due

to compressor irreversibilities

5 10.478 kW2 a

$0.08

kW ? h

24 h

5 $0.92

daily cost of exergy destruction due to

irreversibilities in the throttlin

value

5 10.271210.082

5 $0.52

daily cost of exergy destruction due to

irreversibilities in the condenser

5 10.217210.082

5 $0.42

From the solution to Example 6.14, the magnitude of the compressor power is 3.11 kW. Thus, the daily cost is

daily cost of electricity

to operate compressor

5 13.11 kW2

$0.08

kW ? h

24 h

5 $5.97

➊ Associating exergy destruction with operating costs provides a rational basis

for seeking cost-effective design improvements. Although it may be possible

to select components that would destroy less exergy, the trade-off between

any resulting reduction in operating cost and the potential increase in equip-

ment cost must be carefully considered.

Expressed as a percent, how much of the cost of electricity to

operate the compressor is attributable to exergy destruction in the three

components? Ans. 31%.

Ability to…

❑

evaluate exergy destruction.

❑

conduct an elementary eco-

nomic evaluation using exergy.

✓

Skills Developed

7.5.3

Exergy Accounting in Control Volumes at Steady State

For a control volume, the location, types, and true magnitudes of inefficiency and loss

can be pinpointed by systematically evaluating and comparing the various terms of

7.5 Exergy Rate Balance for Control Volumes at Steady State 385

➊

c07ExergyAnalysis.indd Page 385 7/12/10 6:52:51 AM user-s146c07ExergyAnalysis.indd Page 385 7/12/10 6:52:51 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

386 Chapter 7 Exergy Analysis

the exergy balance for the control volume. This is an extension of exergy accounting,

introduced in Sec. 7.4.4.

The next two examples provide illustrations of exergy accounting in control vol-

umes. The first involves the steam turbine with stray heat transfer considered previ-

ously in Example 6.6, which should be quickly reviewed before studying the current

example.

Exergy Accounting of a Steam Turbine

c c c c EXAMPLE 7.8 c

Steam enters a turbine with a pressure of 30 bar, a temperature of 4008C, and a velocity of 160 m/s. Steam exits

as saturated vapor at 1008C with a velocity of 100 m/s. At steady state, the turbine develops work at a rate of

540 kJ per kg of steam flowing through the turbine. Heat transfer between the turbine and its surroundings

occurs at an average outer surface temperature of 350 K. Develop a full accounting of the net exergy carried in

by the steam, in kJ per unit mass of steam flowing. Let T

5 258C, p

5 1 atm.

SOLUTION

Known:

Steam expands through a turbine for which steady-state data are provided.

Find: Develop a full exergy accounting of the net exergy carried in by the steam, in kJ per unit mass of steam

flowing.

Schematic and Given Data: See Fig. E6.6. From Example 6.6, W

5 540 kJ

, Q

5222.6 kJ

Engineering Model:

See the solution to Example 6.6.

2. T

5 258C, p

5 1 atm.

Analysis: The net exergy carried in per unit mass of steam flowing is obtained using Eq. 7.18

2 e

5 1h

2 h

22 T

2 s

2 V

1 g1z

From Table A-4, h

5 3230.9 kJ/kg, s

5 6.9212 kJ/kg

K. From Table A-2, h

5 2676.1 kJ/kg, s

5 7.3549 kJ/kg

Hence, the net rate exergy is carried in is

2 e

5 c13230.9 2 2676.12

2 29816.9212 2 7.35492

1 c

11602

2 11002

1 N

1 k

? m

1 kJ

N ? m

5 691.84 kJ

The net exergy carried in can be accounted for in terms of the exergy transfers accompanying work and heat

transfer and the exergy destruction within the control volume. At steady state, the exergy transfer accompanying

work is simply the work, or W

5 540 kJ

. The quantity Q

is evaluated in the solution to Example 6.6

using the steady-state forms of the mass and energy rate balances: Q

5222.6 kJ

. The accompanying exergy

transfer is

5 a1 2

b a

1 2

298

350

222.6

523.36

where T

denotes the temperature on the boundary where heat transfer occurs.

c07ExergyAnalysis.indd Page 386 7/12/10 6:52:54 AM user-s146c07ExergyAnalysis.indd Page 386 7/12/10 6:52:54 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

The next example illustrates the use of exergy accounting to identify opportunities

for improving thermodynamic performance of the waste heat recovery system con-

sidered in Example 4.10, which should be quickly reviewed before studying the cur-

rent example.

The exergy destruction can be determined by rearranging the steady-state form of the exergy rate balance,

Eq. 7.17, to give

➊

5 a1 2

1 1e

2 e

Substituting values

523.36 2 540 1 691.84 5 148.48 kJ

The analysis is summarized by the following exergy balance sheet in terms of exergy magnitudes on a rate basis:

Net rate of exergy in: 691.84 kJ/kg (100%)

Disposition of the exergy:

• Rate of exergy out

work 540.00 kJ/kg (78.05%)

heat transfer 3.36 kJ/kg (0.49%)

• Rate of exergy destruction 148.48 kJ/kg (21.46%)

691.84 kJ/kg (100%)

Note that the exergy transfer accompanying heat transfer is small relative to

the other terms.

➊ The exergy destruction can be determined alternatively using E

5 T

where s

is the rate of entropy production from an entropy balance. The

solution to Example 6.6 provides s

5 0.4983 kJ

kg ? K.

By inspection of the exergy balance sheet, specify an exergy-

based efficiency for the turbine. Ans. 78.05%.

Ability to…

❑

evaluate exergy quantities

for an exergy accounting.

❑

develop an exergy accounting.

✓

Skills Developed

Exergy Accounting of a Waste Heat Recovery System

c c c c EXAMPLE 7.9 c

Suppose the system of Example 4.10 is one option under consideration for utilizing the combustion products

discharged from an industrial process.

(a) Develop a full accounting of the net exergy carried in by the combustion products.

(b) Use the results of (a) to identify opportunities for improving thermodynamic performance.

SOLUTION

Known:

Steady-state operating data are provided for a heat-recovery steam generator and a turbine.

Find: Develop a full accounting of the net rate exergy is carried in by the combustion products and use the results

to identify opportunities for improving thermodynamic performance.

7.5 Exergy Rate Balance for Control Volumes at Steady State 387

c07ExergyAnalysis.indd Page 387 7/12/10 6:52:56 AM user-s146c07ExergyAnalysis.indd Page 387 7/12/10 6:52:56 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

388 Chapter 7

Exergy Analysis

Engineering Model:

See solution to Example 4.10.

2. T

5 5378R.

Combustion

products out

= 260°F

= 1atm

= 354°F

= 40 lbf/in.

Turbine

Steam

generator

Water in

= 40 lbf/in.

= 102°F

= 275 lb/min

= 1 lbf/in.

= 93%

Combustion products in

= 9230.6 lb/min

= 1 atm

= 400°F

Water out

= 49,610 Btu/min

Fig. E7.9

Analysis:

(a)

We begin by determining the net rate exergy is carried into the control volume. Modeling the combustion

products as an ideal gas, the net rate is determined using Eq. 7.18 together with Eq. 6.20a as

2 e

45 m

2 h

2 T

2 s

5 m

2 h

2 T

82s

82R ln

With data from Table A-22E, h

5 206.46 Btu/lb, h

5 172.39 Btu/lb, s8

5 0.71323 Btu/lb ? 8R, s8

5 0.67002

Btu/lb ? 8R, and p

5 p

, we have

2 e

45 9230.6

min

c1206.46 2 172.392

Btu

2 5378R10.71323 2 0.670022

Btu

lb ? 8R

5 100,300 Btu

min

Next, we determine the rate exergy is carried out of the control volume. Exergy is carried out of the control

volume by work at a rate of 49,610 Btu/min, as shown on the schematic. Additionally, the net rate exergy is car-

ried out by the water stream is

2 e

45 m

2 h

2 T

2 s

From Table A-2E, h

< h

1028F

5 70 Btu

lb, s

< s

1028F

5 0.1331 Btu

lb ? 8R. Using saturation data at 1 lbf/in.

from Table A-3E with x

5 0.93 gives h

5 1033.2 Btu/lb and s

5 1.8488 Btu/lb ? 8R. Substituting values

2 e

45 275

min

c11033.2 2 702

Btu

2 5378R11.8488 2 0.13312

Btu

lb ? 8R

5 11,510 Btu

min

Next, the rate exergy is destroyed in the heat-recovery steam generator can be obtained from an exergy rate

balance applied to a control volume enclosing the steam generator. That is, Eq. 7.13a takes the form

0 5

a1 2

2 W

1 m

2 e

21 m

2 e

22 E

Evaluating (e

2 e

) with Eq. 7.18 and solving for E

5 m

2 e

21 m

2 h

2 T

2 s

The first term on the right is evaluated above. Then, with h

5 1213.8 Btu/lb, s

5 1.7336 Btu/lb ? 8R at 3548F,

40 lbf/in.

from Table A-4E, and previously determined values for h

and s

5 100,300

Btu

min

1 275

min

c170 2 1213.82

Btu

2 5378R10.1331 2 1.73362

Btu

lb ? 8R

5 22,110 Btu

min

Schematic and Given Data:

c07ExergyAnalysis.indd Page 388 7/12/10 6:55:03 AM user-s146 c07ExergyAnalysis.indd Page 388 7/12/10 6:55:03 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

7.6 Exergetic (Second Law) Efficiency

The objective of this section is to show the use of the exergy concept in assessing the

effectiveness of energy resource utilization. As part of the presentation, the exergetic

efficiency

concept is introduced and illustrated. Such efficiencies are also known as

second law efficiencies.

Finally, the rate exergy is destroyed in the turbine can be obtained from an exergy rate balance applied to a

control volume enclosing the turbine. That is, Eq. 7.17 takes the form

0 5

a1 2

2 W

1 m

2 e

22 E

Solving for E

, evaluating (e

2 e

) with Eq. 7.18, and using previously determined values

52W

1 m

2 h

2 T

2 s

5249,610

Btu

min

1 275

min

c11213.8 2 1033.22

Btu

2 5378R11.7336 2 1.84882

Btu

lb ? 8R

5 17,070 Btu

min

The analysis is summarized by the following exergy balance sheet in terms of exergy magnitudes on a rate basis:

Net rate of exergy in: 100,300 Btu/min (100%)

Disposition of the exergy:

• Rate of exergy out

power developed 49,610 Btu/min (49.46%)

water stream 11,510 Btu/min (11.48%)

• Rate of exergy destruction

heat-recovery steam generator 22,110 Btu/min (22.04%)

turbine 17,070 Btu/min (17.02%)

100,300 Btu/min (100%)

(b) The exergy balance sheet suggests an opportunity for improved thermodynamic performance because only

about 50% of the net exergy carried in is obtained as power developed. The remaining nearly 50% of the net

exergy carried in is either destroyed by irreversibilities or carried out by the water stream. Better thermodynamic

performance might be achieved by modifying the design. For example, we might reduce the heat transfer irre-

versibility by specifying a heat-recovery steam generator with a smaller stream-to-stream temperature difference,

and/or reduce the effects of friction by specifying a turbine with a higher isentropic efficiency. Thermodynamic

performance alone would not determine the preferred system embodiment, however, for other factors such as

cost must be considered, and can be overriding. Further discussion of the use

of exergy analysis in design is provided in Sec. 7.7.2.

➊ Alternatively, the rates of exergy destruction in control volumes enclosing the

heat-recovery steam generator and turbine can be determined using E

5 T

where s

is the rate of entropy production for the respective control volume

evaluated from an entropy rate balance. This is left as an exercise.

exergetic efficiency

For the turbine of the waste heat recovery system, evaluate the

isentropic turbine efficiency and comment. Ans. 74%. This isentropic tur-

bine efficiency value is at the low end of the range for steam turbines today,

indicating scope for improved performance of the heat recovery system.

Ability to…

❑

evaluate exergy quantities

for an exergy accounting.

❑

develop an exergy accounting.

✓

Skills Developed

7.6 Exergetic (Second Law) Efficiency 389

➊

c07ExergyAnalysis.indd Page 389 7/12/10 6:55:12 AM user-s146 c07ExergyAnalysis.indd Page 389 7/12/10 6:55:12 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

390 Chapter 7

Exergy Analysis

7.6.1

Matching End Use to Source

Tasks such as space heating, heating in industrial furnaces, and process

steam generation commonly involve the combustion of coal, oil, or natural

gas. When the products of combustion are at a temperature significantly

greater than required by a given task, the end use is not well matched to

the source and the result is inefficient use of the fuel burned. To illustrate

this simply, refer to Fig. 7.7, which shows a closed system receiving a heat

transfer at the rate Q

at a source temperature T

and delivering Q

at a

use temperature T

. Energy is lost to the surroundings by heat transfer at

a rate Q

across a portion of the surface at T

. All energy transfers shown

on the figure are in the directions indicated by the arrows.

Assuming that the system of Fig. 7.7 operates at steady state and there is no work,

the closed system energy and exergy rate balances Eqs. 2.37 and 7.10 reduce, respec-

tively, to

5 1Q

2 Q

22 W

5 ca1 2

2 a1 2

d2 cW

2 p

d2 E

These equations can be rewritten as follows

5 Q

1 Q

(7.19a)

a1 2

5 a1 2

1 a1 2

1 E

(7.19b)

Equation 7.19a indicates that the energy carried in by heat transfer, Q

, is either

used, Q

, or lost to the surroundings, Q

. This can be described by an efficiency in

terms of energy rates in the form product/input as

h 5

(7.20)

In principle, the value of h can be increased by applying insulation to reduce the loss.

The limiting value, when Q

5 0, is h 5 1 (100%).

Equation 7.19b shows that the exergy carried into the system accompanying the

heat transfer Q

is either transferred from the system accompanying the heat transfers

and Q

or destroyed by irreversibilities within the system. This can be described

by an efficiency in terms of exergy rates in the form product/input as

e 5

11 2 T

(7.21a)

Introducing Eq. 7.20 into Eq. 7.21a results in

e 5 h a

1 2

1 2 T

(7.21b)

The parameter e, defined with reference to the exergy concept, may be called an

exergetic efficiency. Note that h and e each gauge how effectively the input is con-

verted to the product. The parameter h does this on an energy basis, whereas e does

it on an exergy basis. As discussed next, the value of e is generally less than unity

even when h 5 1.

Equation 7.21b indicates that a value for h

as close to unity as practical is important

for proper utilization of the exergy transferred from the hot combustion gas to the

system. However, this alone would not ensure effective utilization. The temperatures

System boundary

Air

Fuel

Fig. 7.7 Schematic used to discuss the

efficient use of fuel.

c07ExergyAnalysis.indd Page 390 7/12/10 6:55:14 AM user-s146 c07ExergyAnalysis.indd Page 390 7/12/10 6:55:14 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

and T

are also important, with exergy utilization improving as the use temperature

approaches the source temperature T

. For proper utilization of exergy, therefore,

it is desirable to have a value for h as close to unity as practical and also a good

match between the source and use temperatures.

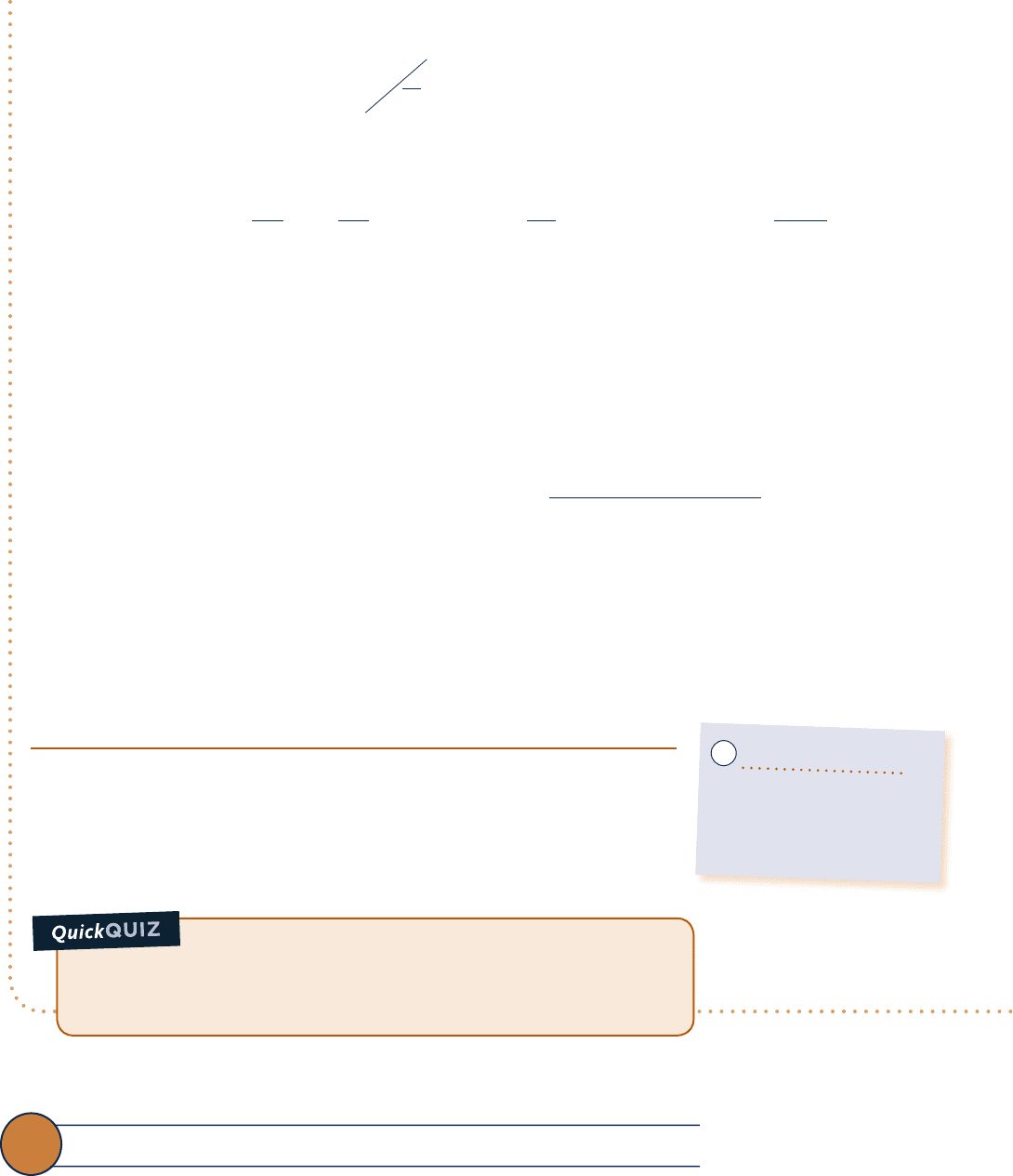
To emphasize further the central role of the use temperature, a graph of Eq. 7.21b

is provided in Fig. 7.8. The figure gives the exergetic efficiency e versus the use tem-

perature T

for an assumed source temperature T

5 2200 K (39608R). Figure 7.8

shows that e tends to unity (100%) as the use temperature approaches T

. In most

cases, however, the use temperature is substantially below T

. Indicated on the graph are

efficiencies for three applications: space heating at T

5 320 K (5768R), process steam

generation at T

5 480 K (8648R), and heating in industrial furnaces at T

5 700 K

(12608R). These efficiency values suggest that fuel is used far more effectively in

higher-temperature industrial applications than in lower-temperature space heating.

The especially low exergetic efficiency for space heating reflects the fact that fuel is

consumed to produce only slightly warm air, which from an exergy perspective has

little utility. The efficiencies given on Fig. 7.8 are actually on the high side, for in

constructing the figure we have assumed h to be unity (100%). Moreover, as addi-

tional destruction and loss of exergy is associated with combustion, the overall effi-

ciency from fuel input to end use would be much less than indicated by the values

shown on the figure.

Costing Heat Loss

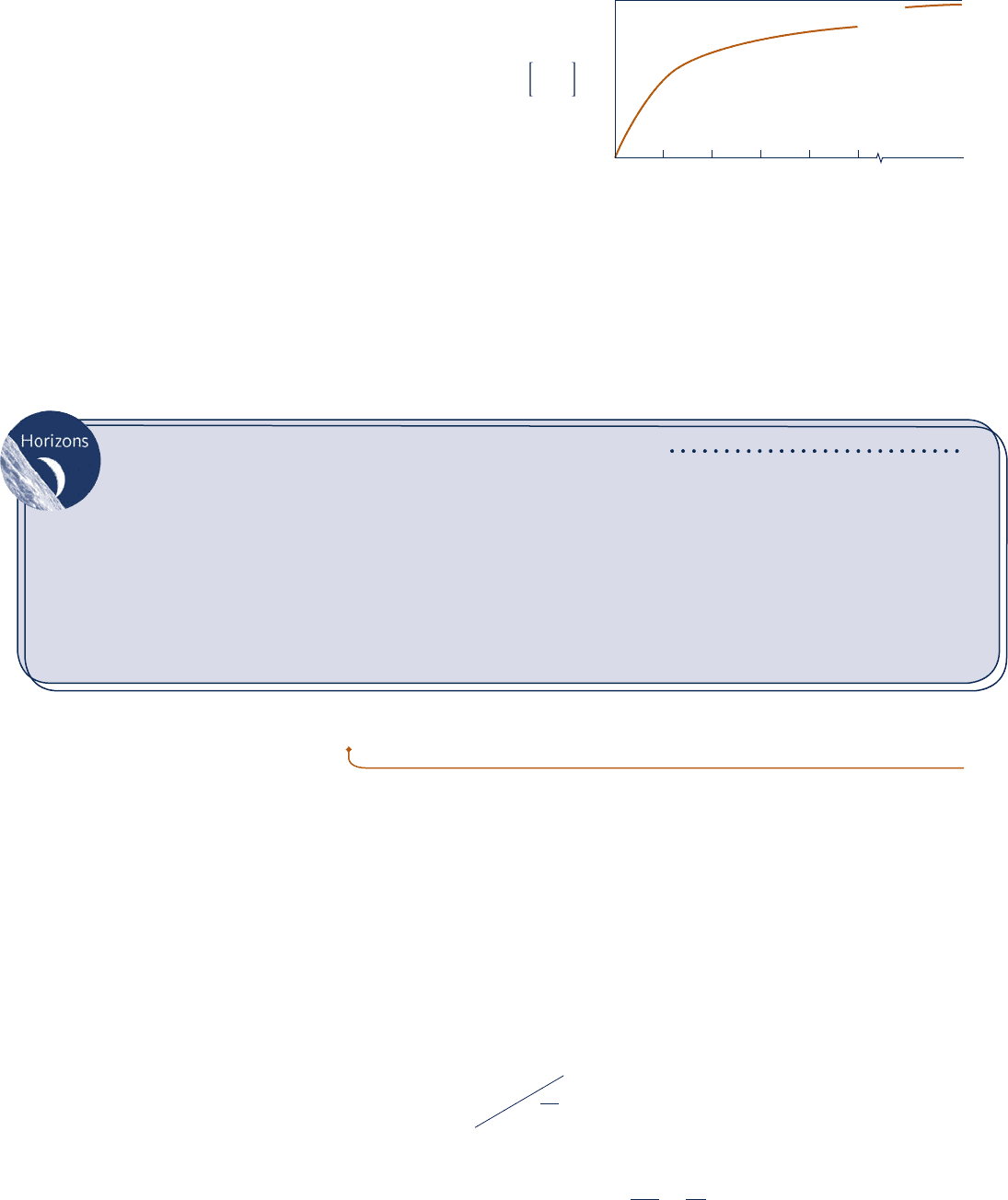
For the system in Fig. 7.7, it is instructive to consider further the rate of exergy loss

accompanying the heat loss Q

, that is 11 2 T

. This expression measures the

true thermodynamic value of the heat loss and is graphed in Fig. 7.9. The figure shows

that the value of the heat loss in terms of exergy depends significantly on the tem-

perature at which the heat loss occurs. We might expect that the economic value of

such a loss varies similarly with temperature, and this is the case.

since the source of the exergy loss by heat transfer is the fuel

input (see Fig. 7.7), the economic value of the loss can be accounted for in terms of

the unit cost of fuel based on exergy, c

(in $/kW

h, for example), as follows

cost rate of heat loss

at temperature T

d5 c

11 2 T

(7.22)

Equation 7.22 shows that the cost of such a loss is less at lower temperatures than at

higher temperatures. b b b b b

Fig. 7.8 Effect of use temperature T

on the exergetic efficiency e (T

5 2200 K, H 5 100%).

Heating in industrial furnaces

Process steam generation

Space heating

0.5

300

540

500 K

900°R

1000 K

1800°R

1500 K

2700°R

1.0

e → 1 (100%)

as T

→ T

7.6 Exergetic (Second Law) Efficiency 391

c07ExergyAnalysis.indd Page 391 8/2/10 10:32:02 AM users-133 c07ExergyAnalysis.indd Page 391 8/2/10 10:32:02 AM users-133 /Users/users-133/Desktop/Ramakant_04.05.09/WB00113_R1:JWCL170/New/Users/users-133/Desktop/Ramakant_04.05.09/WB00113_R1:JWCL170/New

392 Chapter 7

Exergy Analysis

The previous example illustrates what we would expect of a rational costing

method. It would not be rational to assign the same economic value for a heat trans-

fer occurring near ambient temperature, where the thermodynamic value is negligible,

as for an equal heat transfer occurring at a higher temperature, where the thermo-

dynamic value is significant. Indeed, it would be incorrect to assign the same cost to

heat loss independent of the temperature at which the loss is occurring. For further

discussion of exergy costing, see Sec. 7.7.3.

/ T

123456

1 –

0__

Fig. 7.9 Effect of the tem-

perature ratio T

on the

exergy loss associated with

heat transfer.

Traditional oil reserves are now widely anticipated

to decline in years ahead. But the impact could be

lessened if cost-effective and environmentally-benign

technologies can be developed to recover oil-like substances

from abundant oil shale and oil sand deposits in the United

States and Canada.

Production means available today are both costly and inefficient

in terms of exergy demands for the blasting, digging, transporting,

crushing, and heating of the materials rendered into oil. Current

production means not only use natural gas and large amounts of

water, but also cause wide-scale environmental devastation, includ-

ing air and water pollution and huge amounts of toxic waste.

Although significant rewards await developers of improved

technologies, the challenges also are significant. Some say

efforts are better directed to using traditional oil reserves more

efficiently and to developing alternatives to oil-based fuels such

as cellulosic ethanol produced with relatively low-cost biomass

from urban, agricultural, and forestry sources.

Oil from Shale and Sand Deposits—The Jury Is Still Out

7.6.2

Exergetic Efficiencies of Common Components

Exergetic efficiency expressions can take many different forms. Several examples are

given in the current section for thermal system components of practical interest. In

every instance, the efficiency is derived by the use of the exergy rate balance. The

approach used here serves as a model for the development of exergetic efficiency

expressions for other components. Each of the cases considered involves a control

volume at steady state, and we assume no heat transfer between the control volume

and its surroundings. The current presentation is not exhaustive. Many other exergetic

efficiency expressions can be written.

Turbines

For a turbine operating at steady state with no heat transfer with its surroundings,

the steady-state form of the exergy rate balance, Eq. 7.17, reduces as follows:

0 5

a1 2

2 W

1 m

2 e

22 E

This equation can be rearranged to read

2 e

(7.23)

c07ExergyAnalysis.indd Page 392 7/12/10 6:55:17 AM user-s146 c07ExergyAnalysis.indd Page 392 7/12/10 6:55:17 AM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New