Menke W. Geophysical Data Analysis: Discrete Inverse Theory
Подождите немного. Документ загружается.


268
14
Applications
of
Inverse Theory to Geophysics
sedimentation rate is itself a function of time. Measurements of iso-
topic ratio
r(z)
as a function of depth
z
cannot be converted to the
variation of
r(t)
without knowledge of the sedimentation function
z(t)
[or equivalently
t(z)].
Under certain circumstances, the function
r(t)
is known
apriori
(for
instance, oxygen isotopic ratio correlates with temperature, which can
be estimated independently). In these instances it is possible to use the
observed
rob”(z)
and the predicted
rpm(t)
to invert for the function
t(z).
This is essentially a problem in signal correlation: distinctive features
that can
be
correlated between
rob”(z)
and
r”(t)
establish the function
t(z).
The inverse problem is
rOb”(z)
=
rpm[t(z)] (14.8)
and is therefore a problem in nonlinear continuous inverse theory. The
unknown function
t(z)
-
often called the mapping function
-
must
increase monotonically with
z.
The solution of this problem is dis-
cussed by Martinson
et
al.
[Ref.
441
and Shure and Chave [Ref.
591.
14.5
Tectonic Plate Motions
The motion of the earth’s rigid tectonic plates can be described by an
Euler vector
o,
whose orientation gives the pole of the rotation and
whose magnitude gives its rate. Euler vector can be used to represent
relative rotations, that is, the rotation of one plate relative to another,
or absolute motion, that is, motion relative to the earth’s mantle. If we
denote the relative rotation of plate
A
with respect to plate
B
as
o,,
then the Euler vectors of three plates
A,
B,
and C satisfy the relationship
o,
+
ow
+
wcA
=
0.
Once the Euler vectors for plate motion are
known, the relative velocity between two plates at any point on their
boundary can easily be calculated from trigonometric formulas.
Several geologic features provide information on the relative rota-
tion between plates, including the faulting directions of earthquakes at
plate boundaries and the orientation of transform faults, which con-
strain the direction of the relative velocity vectors, and spreading rate
estimates based on magnetic lineations at ridges, which constrain the
magnitude of the relative velocity vectors.
These data can be used in an inverse problem to determine the Euler
vectors [Refs.
28,3
1,48,
and
491.
The main difference between various

14.6
Gravity and Geomagnetism
269
authors’ approaches is in the manner in which the Euler vectors are
parameterized: some authors use their Cartesian components; others
use their magnitude, azimuth, and inclination. Since the relationship
between the magnitude and direction of the relative velocity vectors
(the data) and either choice of these model parameters is nonlinear, the
two inversions can behave somewhat differently in the presence of
noise. Parameterizations in terms of the Cartesian components of the
Euler vectors seem to produce somewhat more stable inversions.
14.6
Gravity and Geomagnetism
Inverse theory plays an important role in creating representations of
the earth’s gravity and magnetic fields. Field measurements made at
many points about the earth need to be combined into a smooth
representation
of
the field, a problem which is mainly one of interpola-
tion in the presence
of
noise and incomplete data. Both spherical
harmonic expansions and harmonic spline functions [Refs.
58,60,
and
6
I]
have been used in the representations. In either case, the trade-off
of resolution and variance is very important. Studies of the earth’s core
and geodynamo require that measurements of the magnetic field at the
earth’s surface be extrapolated to the core
-
mantle boundary. This
is
an inverse problem
of
considerable complexity, since the short-wave-
length components of the field that are most important at the core-
mantle boundary are very poorly measured at the earth’s surface. Fur-
thermore, the rate of change of the magnetic field with time (called
“secular variation”) is
of
critical interest. This quantity must
be
deter-
mined from fragmentary historical measurements, including measure-
ments
of
compass deviation recorded in old ship logs. Consequently,
these inversions introduce substantial
a
priori
constraints on the be-
havior of the field near the core, including the assumption that the
root-mean-square time rate of change
of
the field is minimized and the
total energy dissipation in the core is minimized [Ref.
271.
Once the
magnetic field and its time derivative at the core-mantle boundary
have been determined, they can be used in inversions for the fluid
velocity near the surface
of
the outer core.
Fundamentally, the earth’s gravity field
g(x)
is determined by its
density structure
p(x).
In parts
of
the earth where electric currents and
magnetic induction are unimportant, the magnetic field
H(x)
is caused
by the magnetization
M(x)
of the rocks. These quantities are related
by
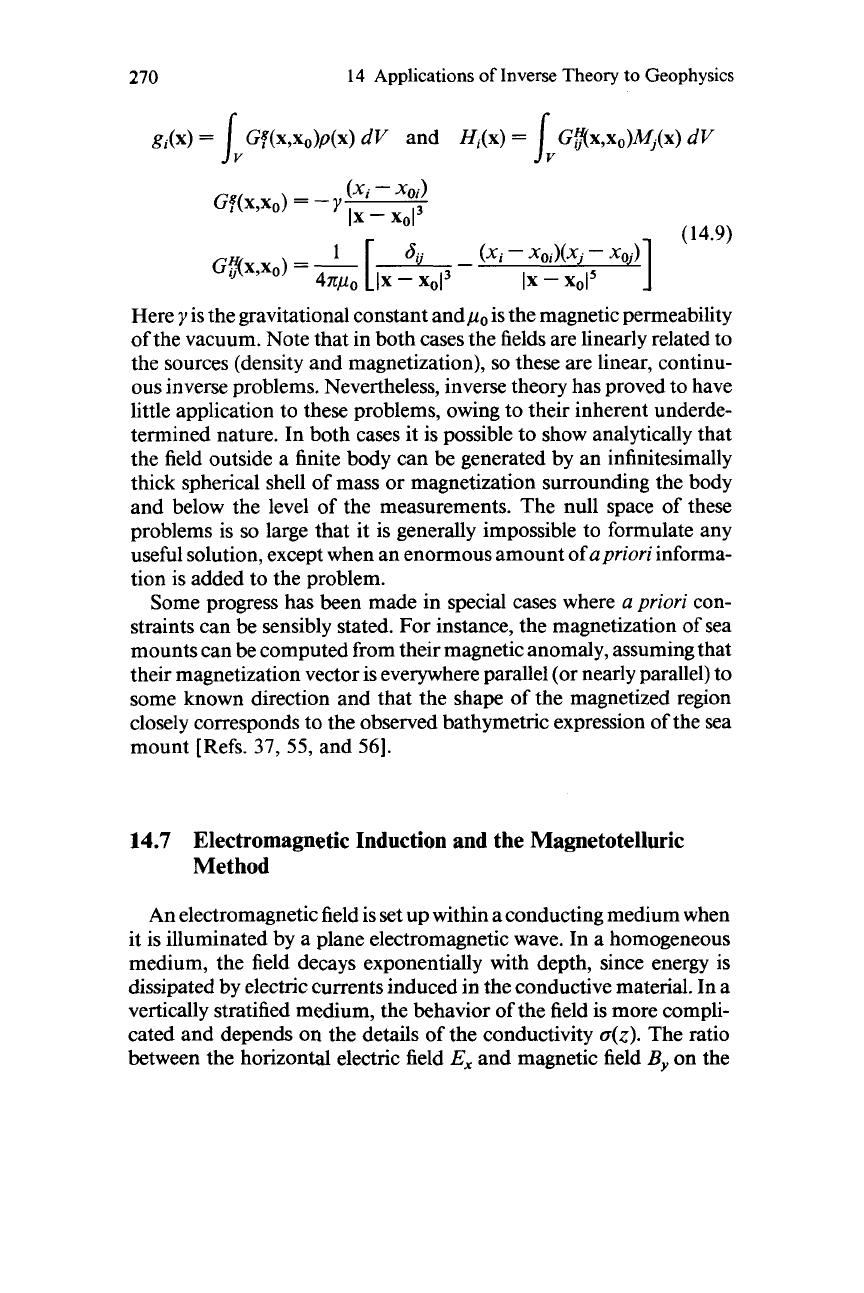
270
14
Applications
of
Inverse Theory
to
Geophysics
gi(x)
=
IvGf(x,x0)p(x)
dV
and
Hi(x)
=
GZx,x,)M,(x)
dV
b
Here
y
is the gravitational constant andPo is the magnetic permeability
of the vacuum. Note that in both cases the fields are linearly related to
the sources (density and magnetization),
so
these are linear, continu-
ous
inverse problems. Nevertheless, inverse theory has proved to have
little application to these problems, owing to their inherent underde-
termined nature. In both cases it is possible to show analytically that
the field outside a finite body can be generated by an infinitesimally
thick spherical shell of mass or magnetization surrounding the body
and below the level
of
the measurements. The null space of these
problems is
so
large that it is generally impossible to formulate any
useful solution, except when an enormous amount
of
apriori
informa-
tion is added to the problem.
Some progress has been made in special cases where
a
priori
con-
straints can be sensibly stated. For instance, the magnetization of sea
mounts can be computed from their magnetic anomaly, assuming that
their magnetization vector is everywhere parallel (or nearly parallel) to
some known direction and that the shape
of
the magnetized region
closely corresponds to the observed bathymetric expression of the sea
mount [Refs.
37,
55,
and
561.
14.7
Electromagnetic Induction and the Magnetotelluric
Method
An electromagnetic field is set up within a conducting medium when
it is illuminated by a plane electromagnetic wave. In a homogeneous
medium, the field decays exponentially with depth, since energy is
dissipated by electric currents induced in the conductive material. In a
vertically stratified medium, the behavior of the field is more compli-
cated and depends
on
the details of the conductivity
a(z).
The ratio
between the horizontal electric field
Ex
and magnetic field
By
on the

14.8
Ocean Circulation
27
1
surface of the medium (at
z
=
0)
is called the admittance and is defined
by
(14.10)
where
w
is frequency. The inverse problem is to determine the conduc-
tivity
a(z)
from measurements of the admittance
Z(w)
at a suite of
frequencies
w.
Parker [Ref. 541 shows that this problem can
be
cast
into a standard linear continuous inverse theory problem by using the
calculus of variations (see Section
12.10)
to calculate a linearized data
kernel. This method has been used to determine the conductivity ofthe
crust and mantle on a variety of scales [e.g., Refs. 52 and 531. Olden-
burg
[
Ref.
5
13
discusses the discretization of the problem and the
application of extremal inversion methods (see Chapter
6).
14.8
Ocean Circulation
Ocean circulation models, that is, models of the velocity field of the
water and the flux of heat and salt (and other chemical components),
are generally based on relatively sparse data, much of it concentrated in
the upper part of the water column. Inverse theory provides a means of
incorporating
a
priori
information, such
as
the dynamical constraints
provided by the Navier- Stokes equations of fluid flow and conserva-
tion laws for mass and energy, into the models and thus improving
them. One common approach is “box models”-models that are very
coarsely parametrized into regions of constant velocity and flux. Box
models are discussed in some detail by Wunsch and Minster [Ref.
671.
This page intentionally left blank

APPENDIX
A
IMPLEMENTING
CONSTRAINTS
WITH
LAGRANGE MULTIPLIERS
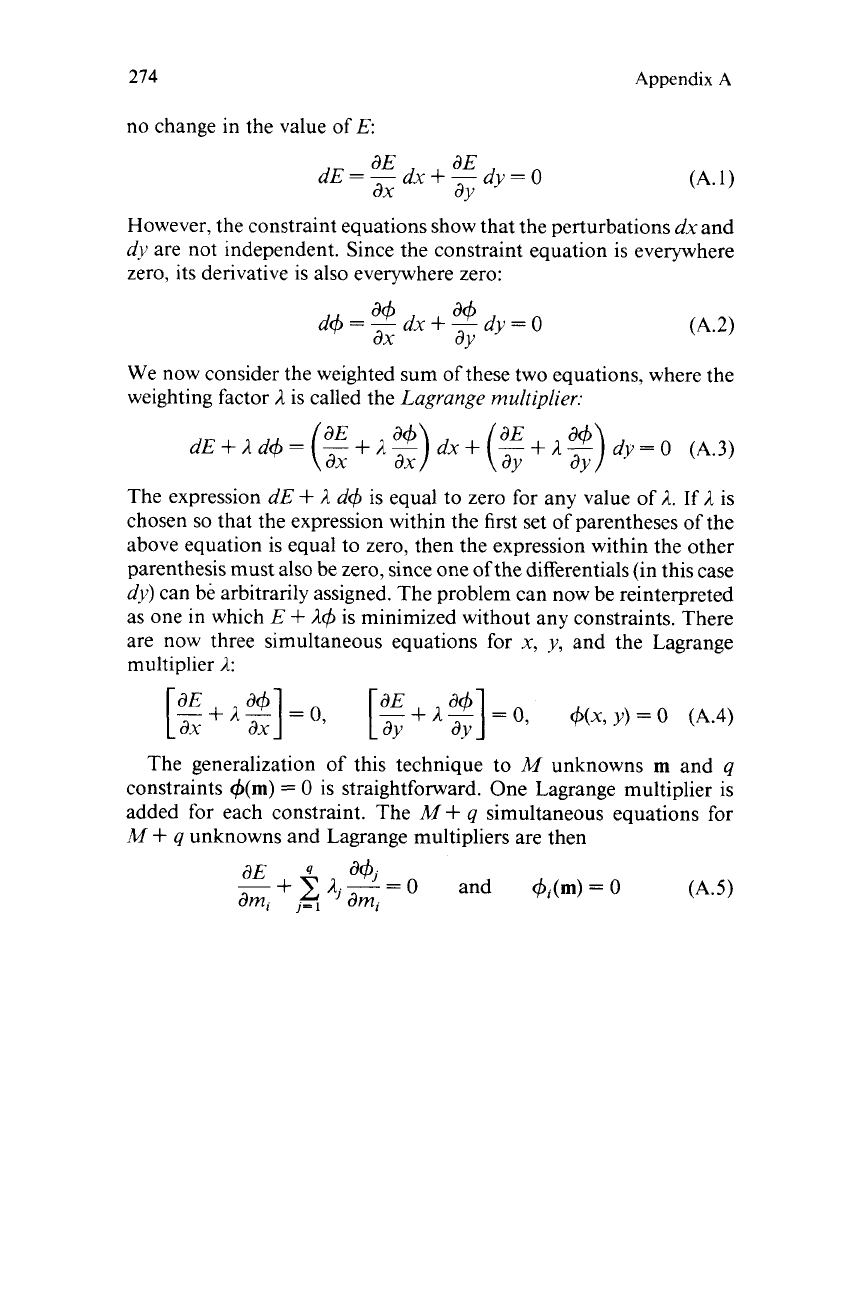
Consider the problem of minimizing a function of two variables,
say,
E(x,
y),
with respect to
x
and
y,
subject to the constraint that
&x,
y)
=
0.
One way to solve this problem
is
to first use
$(x,
y)
=
0
to
write
y
as a function of
x
and then substitute this function into
E(x,
y).
The resulting function
of
a single variable
E(x,
y(x))
can now be
minimized by setting
dE/dx
=
0.
The constraint equation is used to
explicitly reduce the number of independent variables.
One problem with this method is that it
is
rarely possible to solve
&x,
y)
explicitly for either
y(x)
or
x(y).
The method
of
Lagrange
multipliers provides a method
of
dealing with the constraints in their
implicit form.
When the function
E
is minimized, small changes in
x
and
y
lead to
273
274
Appendix
A
no change in the value of
E:
However, the constraint equations show that the perturbations
dx
and
dy
are not independent. Since the constraint equation is everywhere
zero, its derivative is also everywhere zero:
We now consider the weighted
sum
of these two equations, where the
weighting factor
A
is called the
Lagrange multiplier:
The expression
dE
+
A
d4
is equal to zero for any value of
A.
If
A
is
chosen
so
that the expression within the first set of parentheses of the
above equation is equal to zero, then the expression within the other
parenthesis must also be zero, since one of the differentials (in this case
dy)
can be arbitrarily assigned. The problem can now be reinterpreted
as one in which
E
+
A4
is minimized without any constraints. There
are now three simultaneous equations for
x,
y,
and the Lagrange
multiplier
A:
[g+Lg]=O,
[$+Ag]=O,
$(x,y)=O
(A.4)
The generalization of this technique to M unknowns
m
and
q
constraints
&m)
=
0
is straightforward. One Lagrange multiplier is
added for each constraint. The
M+
q
simultaneous equations for
M
+
q
unknowns and Lagrange multipliers are then
d~
a4j
~
+
1.-
=
0
and
4i(m)
=
0
(‘4.5)
dmi
j=,
’
dmi

APPENDIX
B
L,
INVERSE
THEORY
WITH
COMPLEX
QUANTITIES
Some inverse problems (especially those that deal with data that has
been operated on by Fourier or other transforms) involve complex
quantities. Many
of
these problems can readily be solved with a simple
modification of the theory (the exception is the part involving inequal-
ity constraints, which we shall not discuss). The definition of the
L,
norm must be changed to accommodate the complex nature of the
quantities. The appropriate change is to define the squared length
of
a
vector
v
to be
Ilull:
=
vHv,
where
vH
is the
Hermetian transpose,
the
transpose of the complex conjugate
of
the vector
v.
This choice ensures
that the norm is a nonnegative real number. When the results of
L,
inverse theory are rederived for complex quantities, the results are very
similar to those derived previously; the only difference is that all the
ordinary transposes are replaced by Hermetian transposes. For in-
stance, the least squares solution is
[GHG]-*GHd
(B.1)
mest
=
275
276
Appendix
B
Note that all the square symmetric matrices of real inverse theory now
become square Hermetian matrices:
[cov
xIij
=
J
[xi
-
(xi>lH[xi
-
(xi)]P(x)
hr,
[GHG], [GGH],
etc.
These matrices have real eigenvalues,
so
no special problems arise
when deriving eigenvalues or singular-value decompositions.
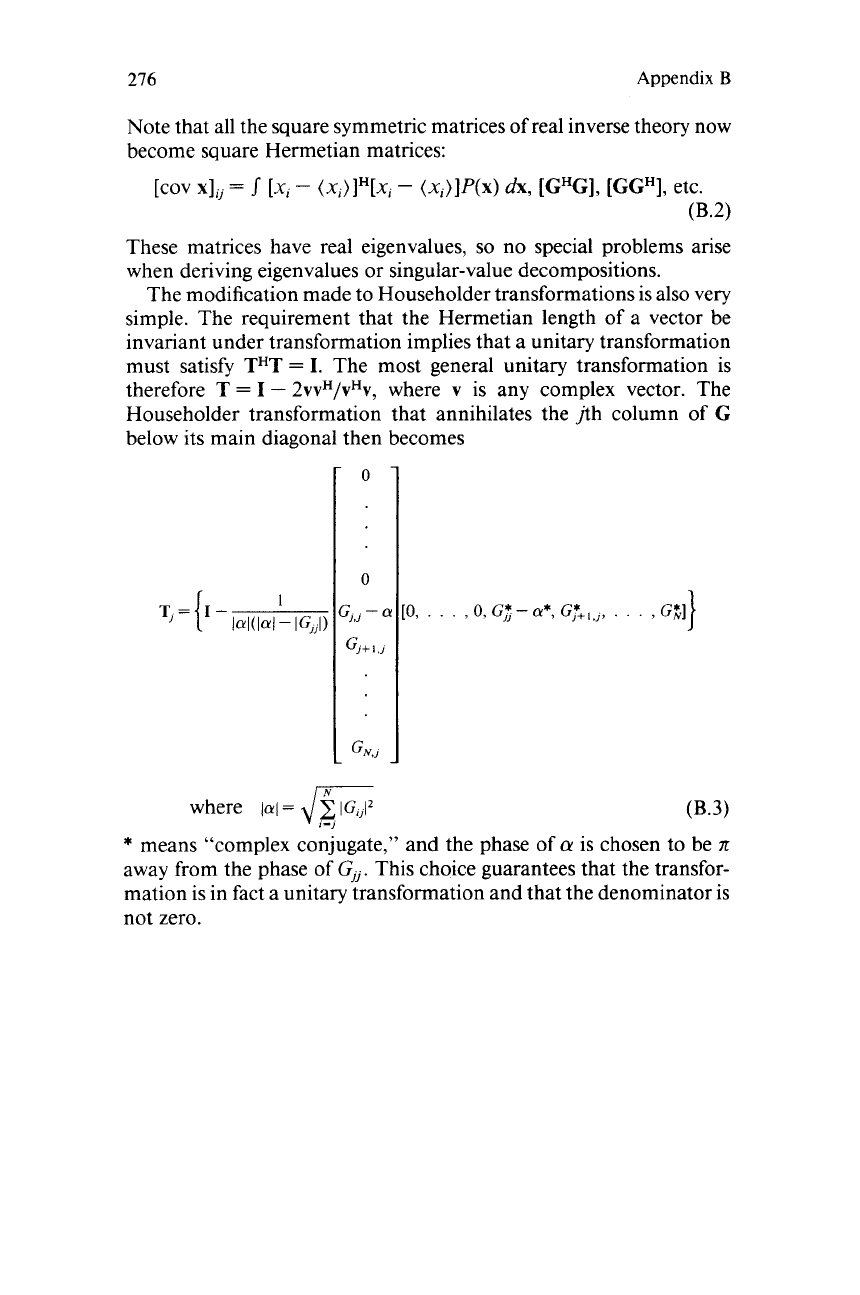
The modification made to Householder transformations is also very
simple. The requirement that the Hermetian length of a vector be
invariant under transformation implies that a unitary transformation
must satisfy
THT
=
I.
The most general unitary transformation is
therefore
T
=
I
-
2vvH/vHv,
where
v
is any complex vector. The
Householder transformation that annihilates the
Jth
column of
G
below its main diagonal then becomes
(B.2)
*
means “complex conjugate,” and the phase of
(Y
is chosen to be
n
away from the phase of
GJi.
This choice guarantees that the transfor-
mation is in fact a unitary transformation and that the denominator is
not zero.

REFERENCES
1.
Backus,
G.
E.,
and Gilbert, J. F. (1967). “Numerical application of a formalism for
geophysical inverse problems.”
Geophys.
J.
Roy. Astron. SOC.
13,
247-276.
2.
Backus, G.
E.,
and Gilbert,
J.
F.
(1968). “The resolving power
of
gross earth data.”
Geophys.
J.
Roy. Astron. SOC.
16,
169-205.
3. Backus,
G.
E.,
and Gilbert, J.
F.
(I
970). “Uniqueness in the inversion ofgross Earth
data.”
Phil.
Trans. Roy. SOC. London, Ser.
A
266,
123- 192.
4. Claerbout,
J.
F.,
and Muir,
F.
(1973). “Robust modelling with erratic data.”
Geophysics
38,
826
-
844.
5. Crosson, R.
S.
(1
976). “Crustal structure modeling
of
earthquake data:
1.
Simulta-
neous least squares estimation of hypocenter and velocity parmeters.”
J.
Geophys.
Res.
81,
3036-3046.
6. Cuer,
M.,
and Bayer, R. (1980). ‘‘FORTRAN routines
for
linear inverse prob-
lems.’”
Geophysics
45,
1706
-
17 19.
7. Fisher, R.
A.
(1953). “Dispersion
on
a
sphere.”
Phil. Trans. Roy. SOC. London, Ser.
A
217,
295-305.
8. Franklin,
J.
N. (1970). “Well-posed stochastic extensions
of
ill-posed linear prob-
lems.’’
J.
Math. Anal. and Appl.
31,
682-716.
9. Jackson, D.
D.
(1972). “Interpretation
of
inaccurate, insufficient and inconsistent
data.”
Geophys.
J.
Roy. Astron. SOC.
28,
97-
110.
10.
Jackson,
D.
D. (1979). ”The use ofa priori data
to
resolve non-uniqueness in linear
inversion.”
Geophys.
J.
Roy. Astron.
SOC.
57,
137- 157.
277
