Menke W. Geophysical Data Analysis: Discrete Inverse Theory

Подождите немного. Документ загружается.

208

Sample Inverse Problems

sphere

Fig.

12.20.

Coordinate system used in Fisher distribution.

This distribution is peaked near the mean direction

0),

and its

width depends

the value of the

precision parameter

(Fig.

12.2 1).

The reciprocal of this parameter serves a role similar to the variance in

the Gaussian distribution. When

the distribution is completely

white

random on the sphere, but when

it becomes very

peaked near the mean direction.

the data are specified by NCartesian unit vectors

(x,

y,,

z,)

and the

mean by its unit vector

(ml

m2,

m3),

then the cosine of the inclination

for any data is just the dot product cos(8,)

[xlml

y,m2

z~z~I.

The joint distribution for the data

P(0,

is then the product of

distributions

the form

P(8,,

4,)

sin(@,). The sin(8,) term must be

included since we shall be maximizing the joint probability with

respect

and not with respect to solid angle. Thejoint distribution

is then

P(0)

[~/4n

sinh(ic)IN exp

where cos(Oi)

xim,

-k

ylm,

zim3

and the likelihood function is

log(P)

log(K)

log(&)

log[sinh(~)]

(12.24)

12.7

Finding

the

Mean

Set

Unit

Vectors

209

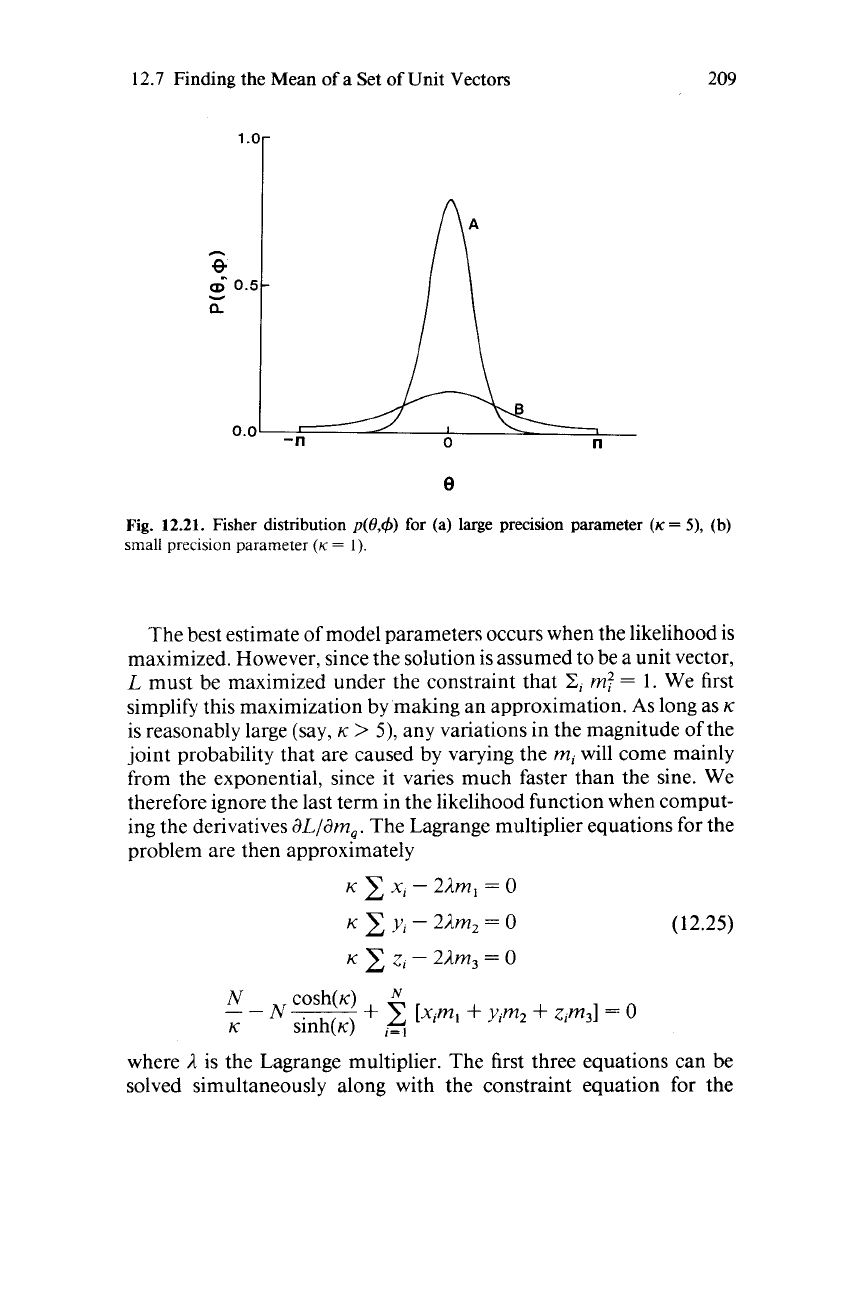
Fig.

12.21. Fisher

distribution

p(@#~)

for (a) large precision parameter

5),

(b)

small

precision

parameter

I).

The best estimate of model parameters occurs when the likelihood is

maximized. However, since the solution is assumed to be a unit vector,

must be maximized under the constraint that

rnf

We first

simplify this maximization by making an approximation.

long as

is reasonably large (say,

5),

any variations in the magnitude of the

joint probability that are caused by varying the

rn,

will come mainly

from the exponential, since it varies much faster than the sine. We

therefore ignore the last term in the likelihood function when comput-

ing the derivatives

dL/drn,.

The Lagrange multiplier equations for the

problem are then approximately

2h,

Urn,

2h3

2.25)

cosh(ic)

sinh(ic)

[xirn,

yirn,

zirn3]

where

is the Lagrange multiplier. The first three equations can be

solved simultaneously along with the constraint equation for the

Sample Inverse Problems

Fig.

12.22.

Maximum likelihood estimate

the Fisher distribution’s central vector

(bold)

parallel

the vector

sum

the data.

model parameters as

(12.26)

and the fourth equation is then an implicit transcendental equation for

Since we have assumed that

we can use the approximation

cosh(lc)/sinh(lc)

and the fourth equation yields

N/[N

cos(8,)].

The mean vector is the normalized vector sum of the individual

observed unit vectors (Fig.

12.22).

12.8

Gaussian Curve Fitting

Many types

spectral data consist of several overlapping peaks,

each

which has a Gaussian shape (Fig.

12.23).

The problem is to

determine the location, area, and width of each peak through least

squares curve-fitting.

Suppose that the data consist of

(z,

pairs, where the auxiliary

variable

represents spectral frequency. Each

of,

say,

peaks is

12.8

Gaussian

Curve

Fitting

frequency

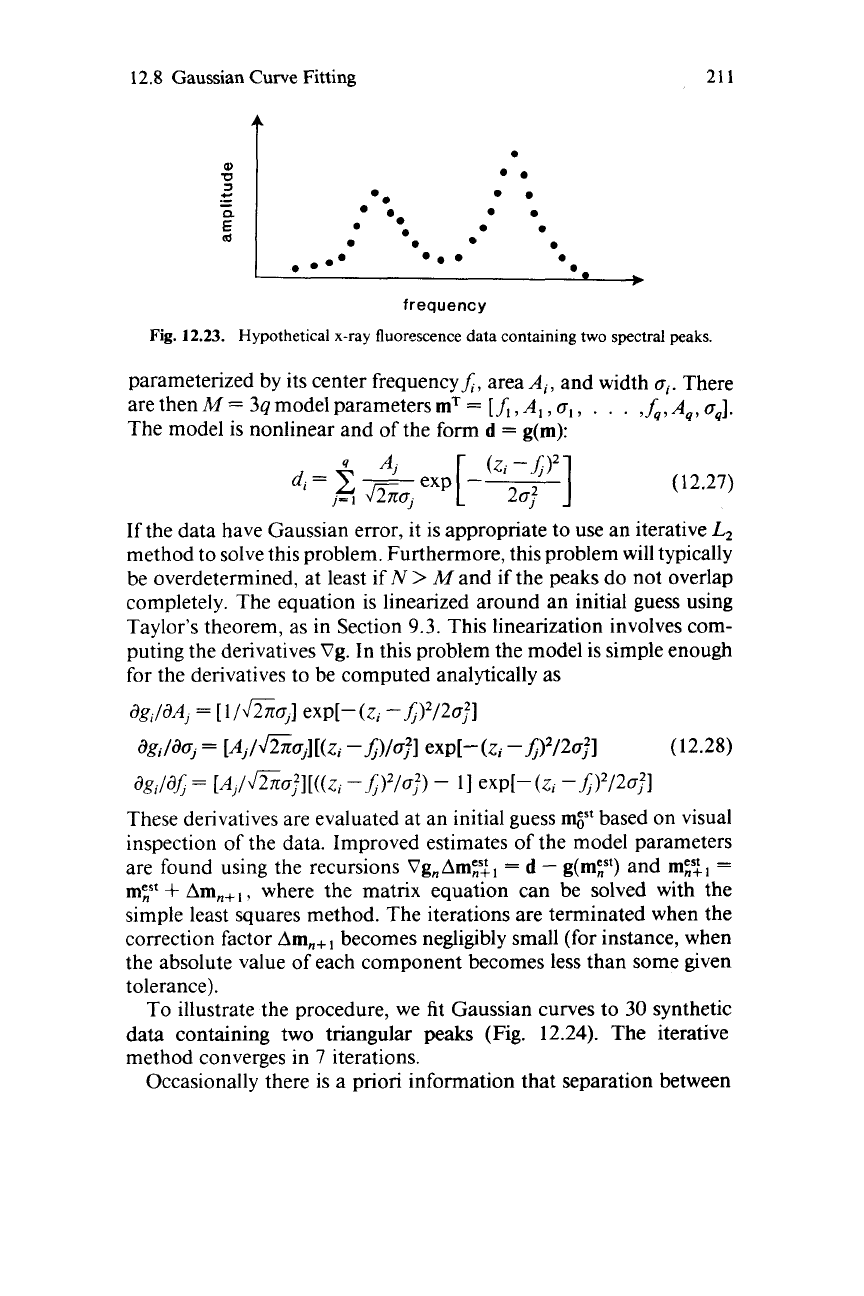
Fig.

12.23.

Hypothetical x-ray fluorescence data containing two spectral peaks.

parameterized by its center frequencyf;, area

Ai,

and width

ai.

There

are then

model parameters

[

. .

,f,,

A,,

aq].

The model is nonlinear and of the form

g(m):

2.27)

the data have Gaussian error, it is appropriate to use an iterative

method to solve this problem. Furthermore, this problem will typically

be overdetermined, at least if

and if the peaks do not overlap

completely. The equation is linearized around an initial guess using

Taylor's theorem, as in Section

9.3.

This linearization involves com-

puting the derivatives

Vg.

In this problem the model is simple enough

for the derivatives to be computed analytically as

ag,/aA,

[

~/Go,I

exp[-(z,

-J)~/~o,ZI

%/aJ

[A,/GO,21[((Z1

-J)2/Of)

exP[-(Z,

-J)*/241

dg,

/do,

[A,

/&a,]

KZ,

J)/~,zI

exp[--

(z,

J)~/~o,zI

(12.28)

These derivatives are evaluated at an initial guess

based on visual

inspection of the data. Improved estimates of the model parameters

are found using the recursions

Vg,AmFi,

g(myt)

and

m",":,

myf

Am,,,

where the matrix equation can be solved with the

simple least squares method. The iterations are terminated when the

correction factor

Am,,

becomes negligibly small (for instance, when

the absolute value of each component becomes less than some given

tolerance).

illustrate the procedure, we fit Gaussian curves to

synthetic

data

containing two triangular peaks (Fig.

12.24).

The iterative

method converges in

iterations.

Occasionally there is a priori information that separation between

212

Sample

Inverse

Problems

frequency

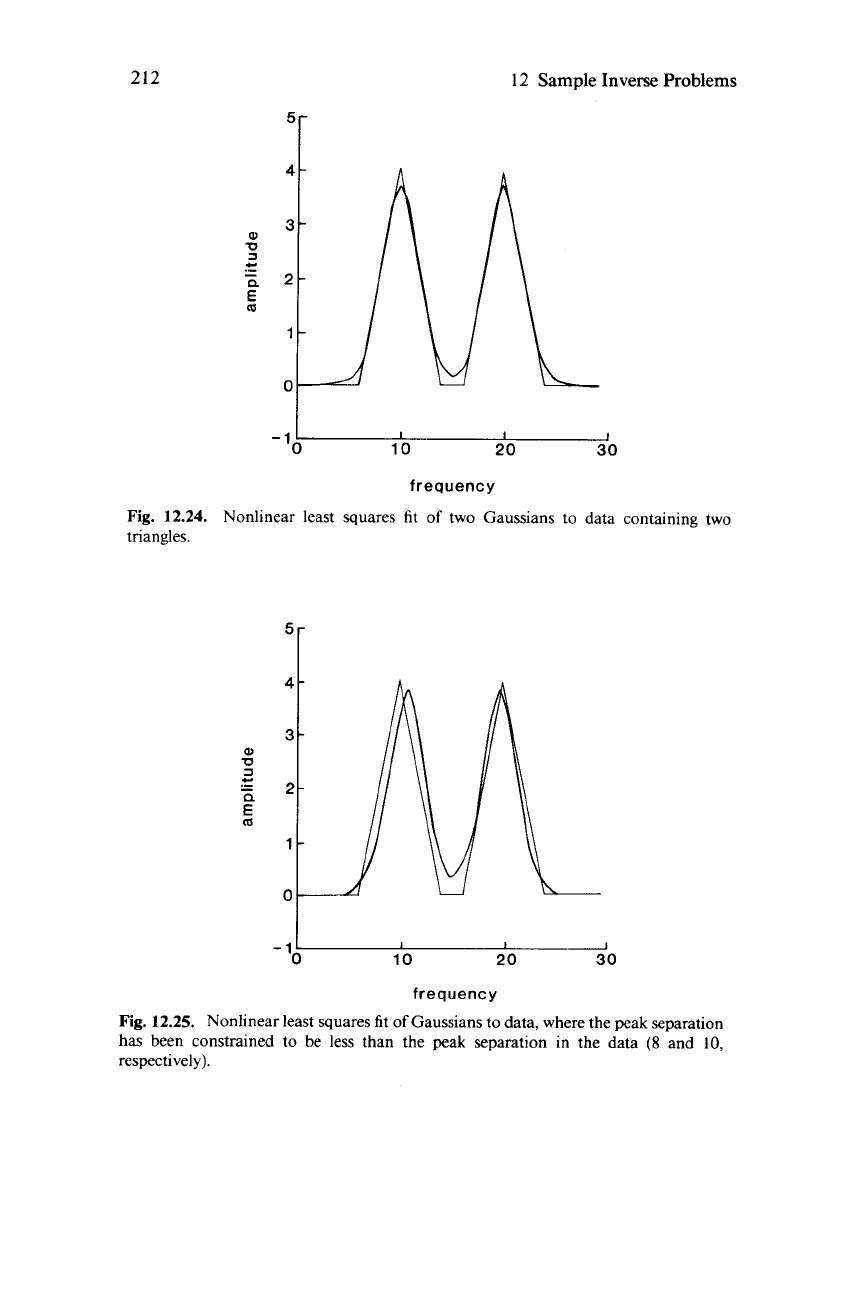
Fig.

12.24.

Nonlinear least squares fit

two Gaussians to data containing two

triangles.

frequency

Fig.

12.25.

Nonlinear least squares fit

Gaussians to data, where the peak separation

has been constrained to

less than the peak separation in the data

and

10,

respectively).

12.9

Earthquake

Location

213

two peaks must equal a gven constantf;

-4

(sjj).

This constraint

can be implemented by imposing it at each iteration;

Amy:,”:,

where

(12.29)

These constraints can be implemented either by Lagrange multipliers

or by Householder transformations. An example computed by the

latter method

shown in Fig. 12.25. The peak separation is con-

strained to be somewhat larger than the peak separation in the data,

the fit is not particularly good. This calculation also converges in seven

iterations.

One of the drawbacks to these iterative methods is that if the initial

guess is too far

off,

the solution may oscillate wildly or diverge from

one iteration to the next. There are several possible remedies for this

difficulty. One is to force the perturbation

Amn+

to be less than a given

length. This result can be achieved by examining the perturbation on

each iteration and, if it is longer than some empirically derived limit,

decreasing its length (but not changng its direction). This procedure

will prevent the method from wildly “overshooting” the true mini-

mum but will also slow the convergence. Another possibility is to

constrain some of the model parameters to equal a priori values for the

first few iterations, thus allowing only some of the model parameters to

vary. The constraints are relaxed after convergence, and the iteration is

continued until the unconstrained solution converges.

12.9

Earthquake

Location

When a fault ruptures within the earth, seismic compressional Pand

shear

waves are emitted. These waves propagate through the earth

and can be recorded by instruments on the earth’s surface. The

earthquake location problem is to determine the location

(xo,

yo,

zo)

and time

of the rupture on the basis

measurements of the time of

amval of the waves at the instruments.

The forward problem- that of predicting the travel time of seismic

waves given a particular source (fault), receiver geometry, and seismic

velocity structure in the earth-is quite formidable. We shall not

discuss it here, but note that it usually requires finding the path, or

ray,

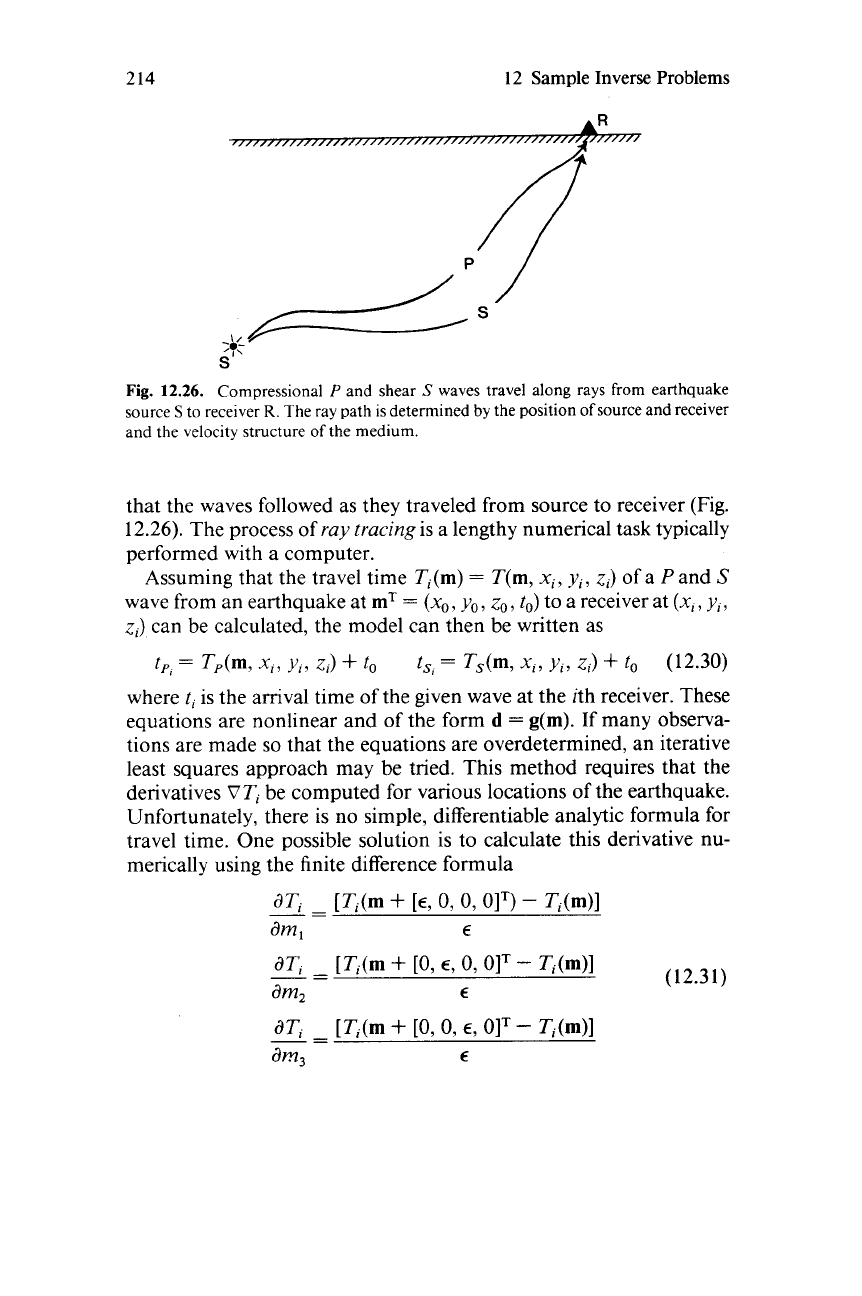
214

Sample Inverse Problems

Fig. 12.26.

Compressional

and shear

waves travel along rays

from

earthquake

source

to receiver

The ray path is determined by the position

source and receiver

and the velocity structure

the medium.

that the waves followed as they traveled from source to receiver (Fig.

12.26). The process of

ray

tracing

is a lengthy numerical task typically

performed with a computer.

Assuming that the travel time

T,(m)

T(m,

x,,

y,,

z,)

of a

and

wave from an earthquake at

(xo,

yo,

zo,

to)

to a receiver at

(x,

y,,

z,)

can be calculated, the model can then be written as

tP,

TP(m,

Y,,

z,)

ts,

Ts(m,

x,,

Y,,

z,)

(12.30)

where

is the amval time of the given wave at the ith receiver. These

equations are nonlinear and of the form

g(m).

many observa-

tions are made

that the equations are overdetermined, an iterative

least squares approach may be tried. This method requires that the

derivatives

OT,

be computed for various locations of the earthquake.

Unfortunately, there is no simple, differentiable analytic formula for

travel time. One possible solution is to calculate this derivative nu-

merically using the finite difference formula

aT,

[T,(m

[E,

0, 0,

0lT)

T,(m)l

dT,

[T,(m

[O,

E,O,

OIT

T,(m)l

dT,

[T,(m

[O,

OIT

T,Wl

dm,

dm2

dm,

(12.31)

12.9

Earthquake

Location

215

Note that

aTJam,

since the travel time depends only on the

location of the earthquake and not on its time of occurence. These

equations represent moving the location of the earthquake a small

distance

along the directions of the coordinate axes and then com-

puting the change in travel time. This approach has two disadvantages.

First, if

is made very small

that the finite difference approximates a

derivative very closely, the terms in the numerator become nearly

equal and computer round-off error can become very significant.

Second, this method requires that the travel time be computed for

three additional earthquake locations and, therefore, is four times as

expensive as computing travel time alone.

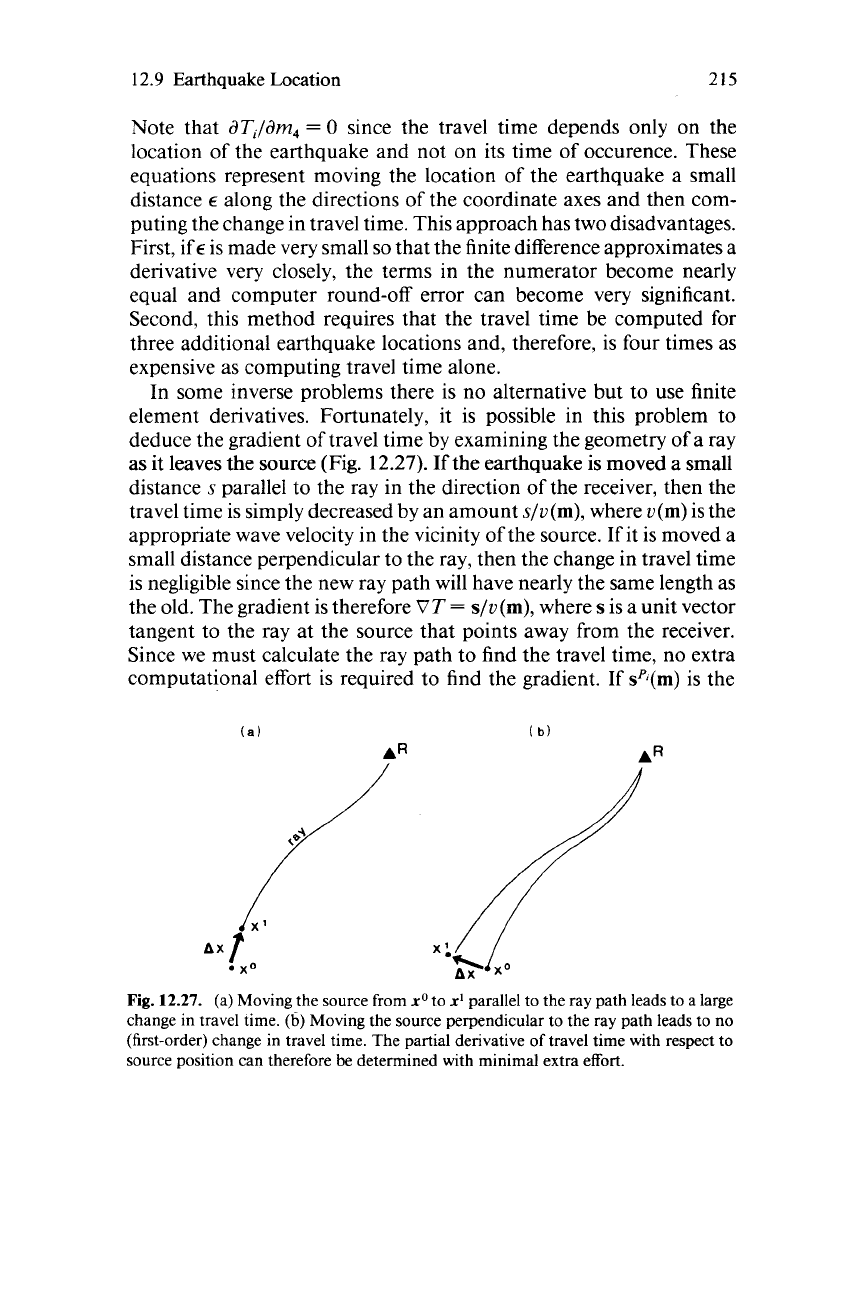
In some inverse problems there is no alternative but to use finite

element derivatives. Fortunately, it is possible in this problem to

deduce the gradient of travel time by examining the geometry of a ray

it leaves the source (Fig.

12.27).

the earthquake

moved a small

distance

parallel to the ray in the direction of the receiver, then the

travel time is simply decreased by an amount

s/v(m),

where

u(m)

is the

appropriate wave velocity in the vicinity of the source.

it is moved a

small distance perpendicular to the ray, then the change in travel time

is negligible since the new ray path will have nearly the same length as

the old. The gradient is therefore

s/u(m),

where

is a unit vector

tangent to the ray at the source that points away from the receiver.

Since we must calculate the ray path to find the travel time, no extra

computational effort is required to find the gradient.

sPl(rn)

is the

Fig.

12.27.

(a) Moving the source from

parallel to the ray path leads to a large

change in travel time.

(6)

Moving the source perpendicular to the ray path leads to

(first-order) change in travel time. The partial derivative

travel time with respect to

source position can therefore

determined with minimal extra effort.

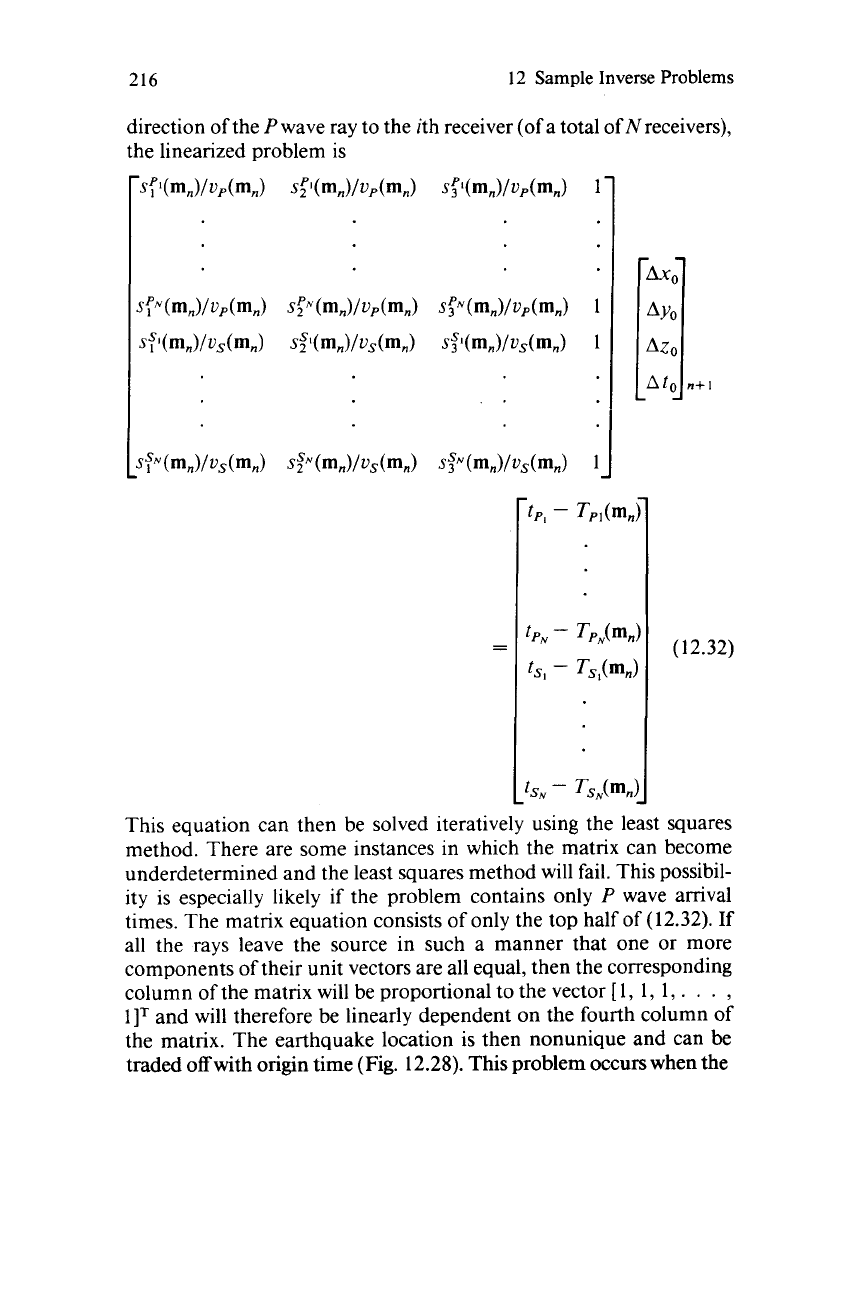
216

Sample Inverse Problems

direction of the

wave ray to the ith receiver (of a total of Nreceivers),

the linearized problem is

This equation can then be solved itera

Ts,<m,>

ively using the least squa

method. There are some instances in which the matrix can become

underdetermined and the least squares method will fail. This possibil-

ity

especially likely

the problem contains only

wave amval

times. The matrix equation consists of only the top half of

(12.32).

all the rays leave the source in such a manner that one or more

components of their unit vectors are all equal, then the corresponding

column of the matrix will be proportional to the vector

[

. .

1IT

and will therefore be linearly dependent on the fourth column of

the matrix. The earthquake location is then nonunique and can

traded

off

with

origin time (Fig.

12.28).

This problem

occurs

when the

12.10

Vibrational

Problems

217

(b)

Fig.

12.28.

(a) In layered media, rays follow “refracted” paths, such that rays to all

receivers (triangles) leave the source (star) at the same angle. The position and travel

time of the source on the dashed line therefore trade

off.

(b)

This phenomenon also

occurs in nonlayered media if the earthquake is far from the receivers.

earthquake is far from all of the stations. The addition ofS wave arrival

times resolves the underdeterminacy (the columns are then propor-

tional to

[I,

1, 1,

. . .

vs/vp,

. .

and are not linearly

dependent on the fourth column). It is therefore wise to use singular-

value decomposition and the natural inverse to solve earthquake

location problems, permitting easy identification of underdetermined

cases.

The earthquake location problem can also be extended to locate the

earthquake and determine the velocity structure of the medium simul-

taneously. It then becomes similar to the tomography problem (Sec-

tion

12.4)

except that the orientations of the ray paths are unknown.

To ensure that the medium is crossed by a sufficient number

rays to

resolve the velocity structure, one must locate simultaneously a large

set of earthquakes-on the order of

100

[Ref.

51.

12.10

Vibrational

Problems

There are many inverse problems that involve determining the

structure of an object from measurements of its characteristic fre-

quencies of vibration (eigenfrequencies). For instance, the solar and

terrestrial vibrational frequencies can be inverted for the density and

elastic structure of those two bodies. These inverse problems are

typically nonlinear. Special effort must therefore be made to find

simple means of calculating the partial derivatives

eigenfrequency

with respect to the structure. We shall describe a general procedure for

calculating these derivatives that is based on perturbation theory and

that is applicable whenever the vibrations satisfy a linear, second-order