Mavko G., Mukerji T., Dvorkin J. The Rock Physics Handbook
Подождите немного. Документ загружается.

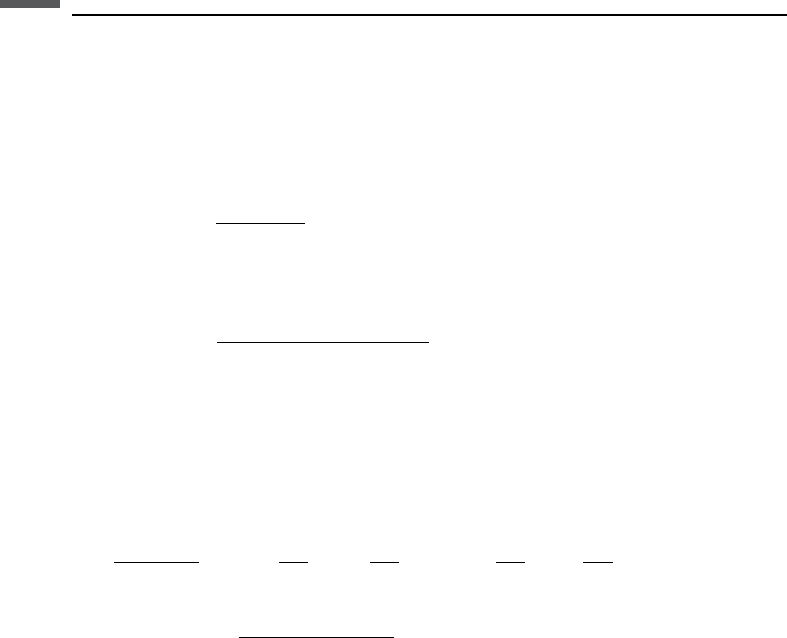
Shapiro and Zien (1993) generalized the O’Doherty–Anstey formula for non-
normal incidence. The derivation is based on a small perturbation analysis and
requires the fluctuations of material parameters to be small (<30%). The generalized
formula for plane pressure (scalar) waves in an acoustic medium incident at an
angle y with respect to the layer normal is
jAðoÞj exp
^
Rðo cos Þ
cos
4
T
rt
whereas
jAðoÞj exp
ð2 cos
2
1Þ
2
^
Rðo cos Þ
cos
4
T
rt
"#
for SH-waves in an elastic medium (Shapiro et al., 1994).
For a perfectly periodic stratified medium made up of two constituents with phase
velocities V
1
, V
2
;densitiesr
1
, r
2
; and thicknesses l
1
, l
2
, the velocity dispersion relation
may be obtained from the Floquet solution (Christensen, 1991) for periodic media:
cos
oðl
1
þ l
2
Þ
V
¼cos
ol
1
V
1
cos
ol
2
V
2
sin
ol
1
V
1
sin
ol
2
V
2
¼
ð
1
V
1
Þ
2
þð
2
V
2
Þ
2
2
1
2
V
1
V
2
The Floquet solution is valid for arbitrary contrasts in the layer properties. If the
spatial period (l
1
þ l
2
) is an integer multiple of one-half wavelength, multiple reflec-
tions are in phase and add constructively, resulting in a large total accumulated
reflection. The frequency at which this Bragg scattering condition is satisfied is called
the Bragg frequency. Waves cannot propagate within a stop-band around the Bragg
frequency.
Uses
The results described in this section can be used to estimate velocity dispersion and
attenuation caused by scattering for normal-incidence wave propagation in layered
media.
Assumptions and limitations
The methods described in this section apply under the following conditions:
layers are isotropic, linear elastic with no lateral variation;
propagation is normal to the layers except for the generalized O’Doherty–Anstey
formula; and
plane-wave, time-harmonic propagation is assumed.
137 3.11 Stratigraphic filtering and velocity dispersion

3.12 Waves in layered media: frequency-dependent anisotropy,
dispersion, and attenuation
Synopsis
Waves in layered media undergo attenuation and velocity dispersion caused by
multiple scattering at the layer interfaces. Thinly layered media also give rise to
velocity anisotropy. At low frequencies this phenomenon is usually described by the
Backus average. Velocity anisotropy and dispersion in a multilayered medium are
two aspects of the same phenomenon and are related to the frequency- and angle-
dependent transmissivity resulting from multiple scattering in the medium. Shapiro
et al. (1994) and Shapiro and Hubral (1995, 1996, 1999) have presented a whole-
frequency-range statistical theory for the angle-dependent transmissivity of layered
media for scalar waves (pressure waves in fluids) and elastic waves. The theory
encompasses the Backus average in the low-frequency limit and ray theory in the
high-frequency limit. The formulation avoids the problem of ensemble averaging
versus measurements for a single realization by working with parameters that are
averaged by the wave-propagation process itself for sufficiently long propagation
paths. The results are obtained in the limit when the path length tends to infinity.
Practically, this means the results are applicable when path lengths are very much
longer than the characteristic correlation lengths of the medium.
The slowness (s) and density (r) distributions of the stack of layers (or a continu-
ous inhomogeneous one-dimensional medium) are assumed to be realizations of
random stationary processes. The fluctuations of the physical parameters are small
(<30%) compared with their constant mean values (denoted by subscripts 0):
s
2
ðzÞ¼
1
c
2
0
½1 þ "
s
ðzÞ
ðzÞ¼
0
½1 þ "
ðzÞ
where the fluctuating parts e
s
(z) (the squared slowness fluctuation) and e
r
(z) (the
density fluctuation) have zero means by definition. The depth coordinate is denoted
by z, and the x- and y-axes lie in the plane of the layers. The velocity
c
0
¼ s
2
1=2
corresponds to the average squared slowness of the medium. Instead of the squared
slowness fluctuations, the random medium may also be characterized by the P- and
S-velocity fluctuations, a and b, respectively, as follows:
ðzÞ¼
hi½
1 þ "
ðzÞ ¼
0
½1 þ "
ðzÞ
ðzÞ¼ hi½1 þ "
ðzÞ ¼
0
½1 þ "
ðzÞ
In the case of small fluctuations "
"
s
=2 and
hi
c
0
ð1 þ 3
2
=2Þ, where
2
is the normalized variance (the variance divided by the square of the mean)
138 Seismic wave propagation

of the velocity fluctuations. The horizontal wavenumber k
x
is related to the incidence
angle y by
k
x
¼ k
0
sin ¼ op
where k
0
¼ o/c
0
, p ¼ sin y/c
0
is the horizontal component of the slowness (also called
the ray parameter), and o is the angular frequency. For elastic media, depending on the
type of the incident wave, p ¼sin y/a
0
or p ¼ sin y/b
0
. The various autocorrelation and
cross-correlation functions of the density and velocity fluctuations are denoted by
B
ðÞ¼ "
ðzÞ"
ðz þ Þ
B
ðÞ¼ "
ðzÞ"
ðz þ Þ
hi
B
ðÞ¼ "
ðzÞ"
ðz þ Þ
B
ðÞ¼ "
ðzÞ"
ðz þ Þ
B
ðÞ¼ "
ðzÞ"
ðz þ Þ
B
ðÞ¼ "
ðzÞ"
ðz þ Þ
These correlation functions can often be obtained from sonic and density logs. The
corresponding normalized variances and cross-variances are given by
2
¼ B
ð0Þ;
2
¼ B
ð0Þ;
2
¼ B
ð0Þ;
2
¼ B
ð0Þ;
2
¼ B
ð0Þ;
2
¼ B
ð0Þ
The real part of the effective vertical wavenumber for pressure waves in acoustic
media is (neglecting higher than second-order powers of the fluctuations)
k
z
¼ k
stat
z
k
2
0
cos
2
Z
1
0
d BðÞ sinð2k
0
cos Þ
k
stat
z
¼ k
0
cos ð1 þ
2
=2 þ
2
= cos
2
Þ
BðÞ¼ B
ðÞþ2B
ðÞ= cos
2
þ B
ðÞ= cos
4
For waves in an elastic layered medium, the real part of the vertical wavenumber
for P-, SV-, and SH-waves is given by
k
P
z
¼ l
a
þ o
^
A
P
o
2
Z
1
0
d ½B
P
ðÞsinð2 l
a
ÞþB
BB
ðÞsinðl
ÞþB
DD
ðÞsinðl
þ
Þ
k
SV
z
¼ l
b
þ o
^
A
SV
o
2
Z
1
0
d ½B
SV
ðÞsinð2l
b
ÞB
BB
ðÞsinðl
ÞþB
DD
ðÞsinðl
þ
Þ
k
SH
z
¼ l
b
þ o
^
A
SH
o
2
Z
1
0
d ½B
SH
ðÞsinð2l
b
Þ
and the imaginary part of the vertical wavenumber (which is related to the attenuation
coefficient due to scattering) is
139 3.12 Anisotropy, dispersion, and attenuation

P
¼ o
2
Z
1
0
d ½B
P
ðÞcosð2l
a
ÞþB
BB
ðÞcosðl
ÞþB
DD
ðÞcosðl
þ
Þ
SV
¼ o
2
Z
1
0
d ½B
SV
ðÞcosð2l
b
ÞþB
BB
ðÞcosðl
ÞþB
DD
ðÞcosðl
þ
Þ
SH
¼ o
2
Z
1
0
d ½B
SH
ðÞcosð2l
b
Þ
where l
a
¼ o
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1=
2
0
p
2
p
; l
b
¼ o
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1=
2
0
p
2
p
; l
þ
¼ l
a
þ l
b
,andl
¼ l
b
l
a
(only real-valued l
a,b
are considered). The other quantities in the preceding expressions are
B
P
ðÞ¼ ðX
2
2
0
Þ
1
½B
ðÞC
2
1
þ 2B
ðÞC
1
þ 2B
ðÞC
1
C
2
þ 2B
ðÞC
2
þ B
ðÞþB
ðÞC
2
2
B
SV
ðÞ¼ ðY
2
2
0
Þ
1
½B
ðÞC
2
7
þ 2B
ðÞC
7
C
8
þ B
ðÞC
2
8
B
SH
ðÞ¼ ðY
2
2
0
Þ
1
½B
ðÞY
4
þ 2B
ðÞC
10
Y
2
þ 2B
ðÞC
2
10
B
BB
ðÞ¼ p
2
0
ð4XY
0
Þ
1
½B
ðÞC
2
3
þ 2B
ðÞC
3
C
4
þ B
ðÞC
2
4
B
DD
ðÞ¼ p
2
0
ð4XY
0
Þ
1
½B
ðÞC
2
5
þ 2B
ðÞC
5
C
6
þ B
ðÞC
2
6
^
A
P
¼ð2X
0
Þ
1
A
P
;
^
A
SV
¼ð2Y
0
Þ
1
A
SV
;
^
A
SH
¼ð2Y
0
Þ
1
A
SH
A
P
¼
2
ð1 4p
2
2
0
ZÞþ2
2
ð1 4p
2
2
0
Þþ8
2
p
2
2
0
ð1 2ZÞ
þ 3
2
16p
2
2
0
2
þ 16p
2
2
0
2
ð1 ZÞ
A
SV
¼
2
ð1 C
9
Þþ
2
ð2 4C
9
Þþ
2
ð3 4C
9
Þ
A
SH
¼
2
Y
2
þ 2
2
ð2Y
2
1Þþ
2
ð4Y
2
1Þ
where
X ¼
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
ð1 p
2
2
0
q
Þ; Y ¼
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
ð1 p
2
2
0
Þ
q
; Z ¼ p
2
ð
2
0
2
0
Þ
C
1
¼ X
2
ð1 4p
2
2
0
Þ; C
2
¼8p
2
2
0
X
2
C
3
¼ Xð3 4
2
0
p
2
ÞY½ð
0
=
0
Þþð2
0
=
0
Þ4
0
0
p
2
C
4
¼ 4Xð1 2
2
0
p
2
Þ4Y½ð
0
=
0
Þ2
0
0
p
2
C
5
¼ Xð4
2
0
p
2
3ÞY½ð
0
=
0
Þþð2
0
=
0
Þ4
0
0
p
2
C
6
¼ 4Xð2
2
0
p
2
1Þ4Y½ð
0
=
0
Þ2
0
0
p
2
; C
7
¼ Y
2
ð1 4p
2
2
0
Þ
C
8
¼ 1 8p
2
2
0
Y
2
; C
9
¼ 4Y
2
Z
2
0
=
2
0
; C
10
¼ 1 2p
2
2
0
140 Seismic wave propagation

For multimode propagation (P–SV), neglecting higher-order terms restricts the range
of applicable pathlengths L . This range is approximately given as
maxfl; ag < L < Y
2
maxfl; ag=
2
where l is the wavelength, a is the correlation length of the medium, and s
2
is the
variance of the fluctuations. The equations are valid for the whole frequency range,
and there is no restriction on the wavelength to correlation length ratio.
The angle- and frequency-dependent phase and group velocities are given by
c
phase
¼
o
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
k
2
x
þ k
2
z
p
¼
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
p
2
þ k
2
z
=o
2
p
c
group
¼
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
@o
@k
x
2
þ
@o
@k
z
2
s
In the low- and high-frequency limits the phase and group velocities are the same.
The low-frequency limit for pressure waves in acoustic media is
c
low freq
fluid
c
0
1
2
=2
cos
2
2
hi
and for elastic waves
c
low freq
P
¼
0
ð1 A
P
=2Þ
c
low freq
SV
¼
0
ð1 A
SV
=2Þ
c
low freq
SH
¼
0
ð1 A
SH
=2Þ
These limits are the same as the result obtained from Backus averaging with
higher-order terms in the medium fluctuations neglected. The high-frequency limit
of phase and group velocities is
c
high freq
fluid
¼ c
0
1 þ
2
=2 cos
2
for fluids, and
c
high freq
P
¼
0
1
2
1 3p
2
2
0
=2
= 1 p
2
2
0
c
high freq
SV;SH
¼
0
1
2
1 3p
2
2
0
=2
= 1 p
2
2
0
for elastic media, which are in agreement with ray theory predictions, again neglect-
ing higher-order terms.
141 3.12 Anisotropy, dispersion, and attenuation

Shear-wave splitting or birefringence and its frequency dependence can be char-
acterized by
Sðo; pÞ¼
c
SV
ðo; pÞc
SH
ðo; pÞ
c
SV
ðo; pÞ
c
SV
ðo; pÞc
SH
ðo; pÞ
0
In the low-frequency limit S
low freq
(o, p) (A
SH
– A
SV
)/2, which is the shear-wave
splitting in the transversely isotropic medium obtained from a Backus average of the
elastic moduli. In the high-frequency limit S
high freq
(o, p) ¼ 0.
For a medium with exponential correlation functions s
2
exp (–x/a) (where a is the
correlation length) for the velocity and density fluctuations with different variances
s
2
but the same correlation length a, the complete frequency dependence of the phase
and group velocities is expressed as
c
phase
fluid
¼ c
0
1 þ
ð2k
2
0
a
2
2
2
1
2
cos
2
2
1 þ 4k
2
0
a
2
cos
2
!
c
group
fluid
¼ c
0
1 þ
N
ð1 þ 4k
0
2
a
2
cos
2
Þ
2
"#
N ¼ 2k
0
2
a
2
2
ð3 þ 4k
2
0
a
2
cos
2
Þþ
2
cos
2
2
þ
2
!
ð4k
2
0
a
2
cos
2
1Þ
for pressure waves in acoustic media. For elastic P-, SV-, and SH-waves in a
randomly layered medium with exponential spatial autocorrelation with correlation
length a, the real part of the vertical wavenumber (obtained by Fourier sine trans-
forms) is given as (Shapiro and Hubral, 1995, 1996, 1999)
k
P
z
¼ l
a
þ oA
P
o
2
a
2
B
P
ð0Þ
2l
a
1 þ 4a
2
l
2
a
þ B
BB
ð0Þ
l
1 þ a
2
l
2
þ B
DD
ð0Þ
l
þ
1 þ a
2
l
2
þ
"#
k
SV
z
¼ l
b
þ oA
SV
o
2
a
2
B
SV
ð0Þ
2l
b
1 þ 4a
2
l
2
b
B
BB
ð0Þ
l
1 þ a
2
l
2
þ B
DD
ð0Þ
l
þ
1 þ a
2
l
2
þ
"#
k
SH
z
¼ l
b
þ oA
SH
o
2
a
2
B
SH
ð0Þ
2l
b
1 þ 4a
2
l
2
b
and the imaginary part of the vertical wavenumber (which is related to the attenuation
coefficient due to scattering) is
P
¼ o
2
aB
P
ð0Þ
1
1 þ 4a
2
l
2
a
þ B
BB
ð0Þ
1
1 þ a
2
l
2
þ B
DD
ð0Þ
1
1 þ a
2
l
2
þ
"#
142 Seismic wave propagation

SV
¼ o
2
aB
SV
ð0Þ
1
1 þ 4a
2
l
2
b
þ B
BB
ð0Þ
1
1 þ a
2
l
2
þ B
DD
ð0Þ
1
1 þ a
2
l
2
þ
"#
SH
¼ o
2
aB
SH
ð0Þ
1
1 þ 4a
2
l
2
b
The shear-wave splitting for exponentially correlated randomly layered media is
Sðo; pÞS
low freq
þ oa
2
0
Y
2l
b
1 þ 4a
2
l
2
b
½B
SV
ð0ÞB
SH
ð0Þ
(
l
þ
1 þ a
2
l
2
þ
B
DD
ð0Þþ
l
1 þ a
2
l
2
B
BB
ð0Þ
)
These equations reveal the general feature that the anisotropy (change in velocity
with angle) depends on the frequency, and the dispersion (change in velocity with
frequency) depends on the angle. Stratigraphic filtering causes the transmitted ampli-
tudes to decay as expðLÞ, where L is the path length and g is the attenuation
coefficient for the different wave modes as described in the equations above.
One-dimensional layered poroelastic medium
The small-perturbation statistical theory has been extended to one-dimensional
layered poroelastic media (Gurevich and Lopatnikov, 1995; Gelinsky and Shapiro,
1997b; Gelinsky et al., 1998). In addition to the attenuation due to multiple scattering
in random elastic media, waves in a random porous saturated media cause inter-layer
flow of pore fluids, leading to additional attenuation and velocity dispersion. The
constituent poroelastic layers are governed by the Biot equations (see Section 6.1),
and can support two P-waves, the fast and the slow P-wave. The poroelastic param-
eters of the random one-dimensional medium consist of a homogeneous background
(denoted by subscript 0) upon which is superposed a zero-mean fluctuation. The
fluctuations are characterized by their variance and a normalized spatial correlation
function B(r/a), such that B(0) ¼ 1, where a is the correlation length. All parameters
of the medium are assumed to have the same normalized correlation function and the
same correlation length, but can have different variances. The poroelastic material
parameters include:
f, porosity; r, saturated bulk rock density; , permeability;
f
; fluid density; ,
fluid viscosity, and K
f
, fluid bulk modulus. P
d
¼ K
d
þ
4
3
d
is the dry (drained) P-
wave modulus, with K
d
and m
d
being the dry bulk and shear moduli, respectively.
¼ 1 K
d
=K
0
is the Biot coefficient (note that in this section, a denotes the
Biot coefficient, not the P-wave velocity). K
0
is the mineral bulk modulus;
M ¼ =K
f
þð Þ=K
0
½
1
; H ¼ P
d
þ
2
M is the saturated P-wave modulus
143 3.12 Anisotropy, dispersion, and attenuation

(equivalent to Gassmann’s equation). N ¼ MP
d
=H; o
0
¼ N=a
2
is the characteris-
tic frequency separating inter-layer-flow and no-flow regimes. o
c
¼ =
f
is the
Biot critical frequency.
Plane P-waves are assumed to be propagating vertically (along the z-direction)
normal to the stack of horizontal layers. The fast P-wavenumber and attenuation
coefficient g are given by (Gelinsky et al., 1998):
¼ k
R
1
þ A
Z
1
0
Bðz=aÞ
ffiffiffi
2
p
D expðzk
I
Þcosðzk
R
=4Þ
h
þ
ffiffiffi
2
p
D expðzk
I
þ
Þcosðzk
R
þ
=4ÞþC expð2zk
I
1
Þsinð2zk
R
1
Þ
i
dz
¼ k
I
1
þ
Z
1
0
Bðz=aÞ
ffiffiffi
2
p
D expðzk
I
Þcosðzk
R
þ =4Þ
h
ffiffiffi
2
p
D expðzk
I
þ
Þcosðzk
R
þ
þ =4ÞþC expð2zk
I
1
Þcosð2zk
R
1
Þ
i
dz
where superscripts R and I denote real and imaginary parts;
~
k
1
¼ k
R
1
þ ik
I
1
and
~
k
2
¼ k
R
2
þ ik
I
2
are the complex wavenumbers for the Biot fast and slow P-
waves in the homogeneous background medium;
~
k
þ
¼ k
R
þ
þ ik
I
þ
¼
~
k
2
þ
~
k
1
;
~
k
¼ k
R
þ ik
I
¼
~
k
2
~
k
1
. The quantities A, C, and D involve complicated functions
of frequency and linear combinations of the variances and covariances of the medium
fluctuations. Approximations for these quantities are discussed below. The above
expressions assume small fluctuations in the poroelastic parameters but are not limited
by any restriction on the relation between wavelength and the correlation length of the
medium fluctuations. The phase velocity for the fast P-wave is given as V
P
¼ o = .
For a medium with an exponential correlation function with correlation length a, the
expressions after carrying out the integrations are (Gelinsky et al., 1998):
o
V
P
¼ k
R
1
þ A
Da 1 þ aðk
R
þ k
I
Þ
1 þ 2ak
I
þ a
2
½ðk
R
Þ
2
þðk
I
Þ
2
Da 1 þ aðk
R
þ
þ k
I
þ
Þ
1 þ 2ak
I
þ
þ a
2
ðk
R
2
þ
þ k
I
2
þ
Þ
2Ca
2
k
R
1
1 þ 4ak
I
1
þ 4a
2
ðk
R
2
1
þ k
I
2
1
Þ
¼ k
I
1
þ
Da 1 aðk
R
k
I
Þ
1 þ 2ak
I
þ a
2
ðk
R
2
þ k
I
2
Þ
þ
Da 1 aðk
R
þ
k
I
þ
Þ
1 þ 2ak
I
þ
þ a
2
ðk
R
2
þ
þ k
I
2
þ
Þ
þ
Cað1 þ 2ak
I
1
Þ
1 þ 4ak
I
1
þ 4a
2
ðk
R
2
1
þ k
I
2
1
Þ
144 Seismic wave propagation
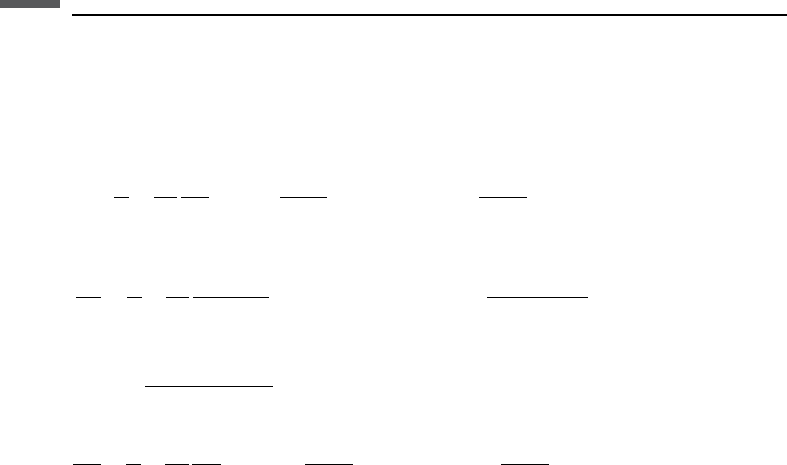
Gelinsky et al. (1998) introduce approximate expressions for A, C, and D, valid
in the frequency range below Biot’s critical frequency. Other approximations
used are:
~
k
þ
~
k
~
k
2
;
~
k
2
ð1 þ iÞk
2
;
~
k
1
k
R
1
¼ k
1
; k
1
¼ o
ffiffiffiffiffiffiffiffiffi
=H
p
; and k
2
¼
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
oo
c
f
=ð2NÞ
p
:
A ¼
o
2
ffiffiffiffiffiffi
H
0
r
P
d0
H
0
2
PP
þ
2
0
M
0
P
d0
2
MM
þ 2
2
P
þ
4
0
M
2
0
P
2
d0
2
2D
k
2
¼
o
2
ffiffiffiffiffiffi
H
0
r
P
d0
2
0
M
0
H
2
0
"
2
PP
2
2
PM
þ
2
MM
2
P
d0
2
0
M
0
P
d0
2
P
2
M
þ
P
d0
2
0
M
0
2
P
2
d0
2
#
C
2k
1
¼
o
8
ffiffiffiffiffiffi
H
0
r
P
2
d0
H
2
0
2
PP
þ 2
2
0
M
0
P
d0
2
PM
þ 2
2
P
þ
4
0
M
2
0
P
2
d0
2
MM
þ 4
2
þ 4
2
M
The phase velocity has three limiting values: a quasi-static value for o o
0
and
o ! 0, V
qs
¼ o k
1
þ A½
1
; an intermediate no-flow velocity for o o
0
,V
nf
¼
o k
1
þ A 2D=k
2
½
1
, and a ray-theoretical limit V
ray
¼ o k
1
þ A 2D=k
2
C=½
ð2k
1
Þ
1
. The quasi-static limit is equivalent to the poroelastic Backus average (see
Section 4.15). The inter-layer flow effect contributes significantly to the total attenu-
ation in the seismic frequency range for highly permeable thin layers with correlation
lengths of a few centimeters.
Uses
The equations described in this section can be used to estimate velocity dispersion
and frequency-dependent anisotropy for plane-wave propagation at any angle in
randomly layered, one-dimensional media. They can also be used to apply angle-
dependent amplitude corrections to correct for the effect of stratigraphic filtering in
amplitude-versus-offset (AVO) modeling of a target horizon below a thinly layered
overburden. The corrected amplitudes are obtained by multiplying the transmissivity
by expðLÞ for the down-going and up-going ray paths (Widmaier et al., 1996). The
equations for poroelastic media can be used to compute velocity dispersion and
attenuation in heterogeneous, fluid-saturated one-dimensional porous media.
Assumptions and limitations
The results described in this section are based on the following assumptions:
layers are isotropic, linear elastic or poroelastic with no lateral variation;
the layered medium is statistically stationary with small fluctuations (<30%) in the
material properties;
145 3.12 Anisotropy, dispersion, and attenuation
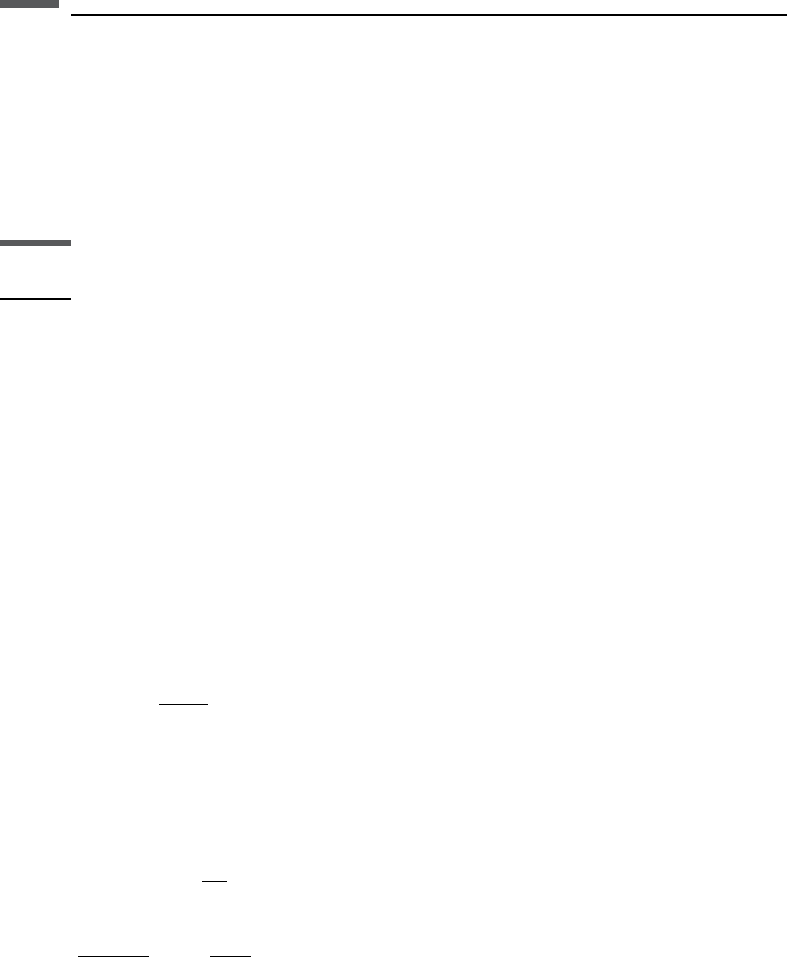
the propagation path is very much longer than any characteristic correlation length
of the medium; and
incident plane-wave propagation is assumed. The expressions for poroelastic media
assume plane waves along the normal to the layers (0
angle of incidence).
Numerical calculations (Gelinsky et al., 1998) indicate that the expressions give
reasonable results for angles of incidence up to 20
.
3.13 Scale-dependent seismic velocities in heterogeneous media
Synopsis
Measurable travel times of seismic events propagating in heterogeneous media
depend on the scale of the seismic wavelength relative to the scale of the geological
heterogeneities. In general, the velocity inferred from arrival times is slower when the
wavelength, l, is longer than the scale of the heterogeneity, a, and faster when the
wavelength is shorter (Mukerji et al., 1995b).
Layered (one-dimensional) media
For normal-incidence propagation in stratified media, in the long-wavelength limit
ðl=a 1Þ, where a is the scale of the layering, the stratified medium behaves as a
homogeneous effective medium with a velocity given by effective medium theory as
V
EMT
¼
M
EMT
av
1=2
The effective modulus M
EMT
is obtained from the Backus average. For normal-
incidence plane-wave propagation, the effective modulus is given by the harmonic
average
M
EMT
¼
X
k
f
k
M
k
!
1
1
av
V
2
EMT
¼
X
k
f
k
k
V
2
k
av
¼
X
k
f
k
k
where f
k
, r
k
, M
k
, and V
k
are the volume fractions, densities, moduli, and velocities of
each constituent layer, respectively. The modulus M can be interpreted as C
3333
or
K þ 4m/3 for P-waves and as C
2323
or m for S-waves (where K and m are the bulk and
shear moduli, respectively).
146 Seismic wave propagation
