Marathe K. Topics in Physical Mathematics

Подождите немного. Документ загружается.

178 6 Theory of Fields, I: Classical

electromagnetic ﬁelds based on the gauge group SU

(3) × SU

(2) × U

(1).

This theory is usually referred to as the standard model of strong, weak,

and electromagnetic interactions or SM for short. We discuss a model of the

electroweak theory and the related Higgs mechanism of symmetry-breaking

in Chapter 8, where a brief account of the standard model will also be given.

Our current knowledge of the fundamental forces and their carrier particles

is summarized in Table 6.2.

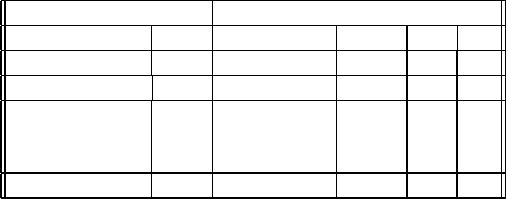
Tab le 6. 2 Fundamental forces and carrier particles

Force Carrier Particles

Name Range Name Symbol Mass Spin

gravitation ∞ graviton Γ 0 2

electromagetism ∞ photon γ 0 1

weak W

80 1

weak 10

−16

intermediate W

−

80 1

vector bosons Z

91 1

strong 10

−14

gluons G

0 1

From the experimental point of view the main diﬃculty in studying strong

interaction is that the energy required for the creation and detection of the

gluons is very high. The Large Hadron Collider (LHC) at CERN, which has

recently gone on line, is expected to bring protons into head-on collision

at extremely high energies. Now the well-known de Broglie wave-particle

duality principle tells us that particles are also waves. This principle is used

in electron microscopes, which exploit the short wavelength of an electron to

reveal details unseen with visible light. The higher the energy, the greater

is the probability for creating more massive particles out of a collision and

the shorter the wavelength corresponding to these particles. This will allow

scientists to penetrate still further into the ﬁne structure of matter. When

fully operational, the LHC is expected to re-create conditions prevailing in

the very early universe (about 10

−10

seconds after the so-called “Big Bang”)

causing nuclear matter to transform into quark-gluon plasma. Studies of such

a state of matter are expected to shed new light on a number of unexplained

phenomena such as quark conﬁnement and the masses of elementary particles.

From the theoretical side, there are several other proposals beyond the

standard model for a uniﬁed treatment of electromagnetic, weak, and strong

interactions. The most extensively studied models are those of the grand

uniﬁed theories (also known as GUTs), the technicolor models, string and

superstring theories, and supersymmetric theories. However, none of these

has been found to be completely satisfactory. The known theories of gravi-

tational forces diﬀer substantially from those of the other three forces. The

quantum theories of gravitational forces as well as uniﬁed theories of all the

6.3 Gauge Fields 179

known forces should at present be considered to be speculative at best. Work

to understand and explain all these theories in precise mathematical terms

should remain on the agenda of mathematical physics of the twenty-ﬁrst cen-

tury.

6.3 Gauge Fields

The theory of gauge ﬁelds and their associated ﬁelds, such as the Yang–Mills–

Higgs ﬁelds, was developed by physicists to explain and unify the fundamental

forces of nature. The theory of connections in a principal ﬁber bundle was

developed by mathematicians during approximately the same period, but as

we pointed out in the preface, the fact that they are closely related was not

noticed for many years. Since then substantial progress has been made in

understanding this relationship and in applying it successfully to problems

in both physics and mathematics. In physical applications one is usually

interested in a ﬁxed Lie group G called the gauge group, which represents an

internal or local symmetry of the ﬁeld. The base manifold M of the principal

bundle P (M,G) is usually the space-time manifold or its Euclidean version,

i.e., a Riemannian manifold of dimension 4. But in some physical applications,

such as superspace, Kaluza–Klein and string theories, the base manifold can

be an essentially arbitrary manifold. In this section we formulate the theory

of gauge ﬁelds on an arbitrary pseudo-Riemannian base manifold.

Let (M,g)beanm-dimensional pseudo-Riemannian manifold and G a

Lie group which we take as the gauge group of our theory. Let P (M,G)

be a principal bundle with the gauge group G as its structure group. A

connection in P is called a gauge connection. The connection 1-form ω is

called the gauge connection form or simply the gauge connection.A

global gauge or simply a gauge is a section s ∈ Γ (P ). The gauge potential

A on M in gauge s is obtained by pull-back of the gauge connection ω on P

to M by s, i.e., A = s

∗

(ω). A global gauge and hence the gauge potential on

M exists if and only if the bundle P is trivial. A local gauge is deﬁned as a

section of the bundle P (M,G) restricted to some open subset U ⊂ M .Local

gauges deﬁned for the local representations (U

,ψ

)

i∈I

of P always exist. Let

t ∈ Γ (U

,P) be a local gaugel then the 1-form t

∗

ω ∈ Λ

, g) is called the

gauge potential in the local gauge t and is denoted by A

. If the local gauge

t is given, we often denote A

by A and call it a local gauge potential.In

electromagnetism, the gauge group G = U(1), the circle group. An element

iθ

∈ U (1) is determined by the phase θ. Thus, in this case, a local gauge

over an open set V ⊂ M can be regarded as a choice of a phase in the bundle

= V ×G at each point of V . For this reason, the total space of the bundle

P is sometimes called the space of phase factors in the physics literature.

Let Ω = d

ω be the curvature 2-form of ω with values in the Lie algebra

g.WecallΩ the gauge ﬁeld on P . Although this terminology is fairly

180 6 Theory of Fields, I: Classical

standard, we would like to warn the reader that sometimes, in the physics

literature, our gauge potential is called the gauge ﬁeld and our gauge ﬁeld is

called the ﬁeld strength tensor. As we have seen in Chapter 4, there exists a

unique 2-form F

on M with values in the Lie algebra bundle ad P associated

to the curvature 2-form Ω such that

= s

. (6.7)

The 2-form F

∈ Λ

(M,ad P ) is called the gauge ﬁeld on M corresponding

to the gauge connection ω. The gauge ﬁeld F

is globally deﬁned on M ,even

though, in general, there is no corresponding globally deﬁned gauge potential

on M. If we are given a local gauge potential A

∈ Λ

, g), then on U

have the relations

∗

ω = A

and F

= d

. (6.8)

Example 6.1 (The Dirac Monopole) Let S

,U(1)) be the principal U(1)-

bundle over S

determined by the Hopf ﬁbration of S

.Letμ denote the

connection 1-form of the canonical connection on this bundle and let F

the corresponding gauge ﬁeld on S

. In this case there is no globally deﬁned

gauge potential on S

. We need at least two charts to cover S

and therefore

at least two local potentials, which give rise to a single globally deﬁned gauge

ﬁeld. This ﬁeld can be shown to be equivalent to the Dirac monopole ﬁeld.

The Dirac monopole quantization condition corresponds to the classiﬁcation

of principal U(1)-bundles over S

. These are classiﬁed by π

(U(1))

∼

In general, the principal G-bundles over S

are classiﬁed by π

(G).Thus

(SU(2)) = id implies that there is a unique SU(2)-monopole on S

and

(SO(3)) = Z

implies that there are two inequivalent SO(3)-monopoles on

(see [161, 411, 410] for further details).

Gauge potentials and gauge ﬁelds acquire physical signiﬁcance only after

one postulates the ﬁeld equations to be satisﬁed by them. These equations and

their consequences must then be subjected to suitably devised experiments

for veriﬁcation. On more than one occasion a theory was abandoned when

its predictions seemed to contradict an experimental result, but later this

experiment or its conclusions turned out to be incorrect and the abandoned

theory turned out to be correct. In any case there is no natural mathemat-

ical method for assigning ﬁeld equations to gauge ﬁelds. Thus the Riemann

curvature of a space-time manifold M is the gauge ﬁeld corresponding to

the gauge potential given by the Levi-Civita connection on the orthonormal

frame bundle of M , but it does not describe the gravitational ﬁeld until it

is subjected to Einstein’s ﬁeld equations. If instead it satisﬁes Yang–Mills

equations, then it describes a classical Yang–Mills ﬁeld. This aspect of gauge

ﬁelds is already evident in the following remark of Yang:

The electromagnetic ﬁeld is a gauge ﬁeld. Einstein’s gravitational theory

is intimately related to the concept of gauge ﬁelds, although to identify

6.3 Gauge Fields 181

the gravitational ﬁeld as a gauge ﬁeld is not an absolutely straightfor-

ward matter.

However, a study of physically interesting ﬁeld equations such as Maxwell’s

equations of electromagnetic ﬁeld and their quantization indicates some de-

sirable features for the gauge ﬁeld equations. One of these features is gauge

invariance of the ﬁeld equations. This requirement is formulated in terms of

the group of gauge transformations, which acts on the various ﬁelds involved.

In the following we give a mathematical formulation of this group.

The group Diﬀ(P ) of the diﬀeomorphisms of P is too large to serve as

a group of gauge transformations, since it mixes up the ﬁbers of P .The

requirement that ﬁbers map to ﬁbers may be expressed by the condition that

the following diagram commutes:

i.e.,

π ◦ f = f

◦ π. (6.9)

We say that the map f is ﬁber-preserving if condition (6.9) is satisﬁed.

We note that condition (6.9) does not depend on the principal bundle struc-

ture of P and hence can be imposed on any ﬁber bundle. The pair (f,f

)

satisfying the condition (6.9) is called a ﬁber bundle automorphism.In

this case we call f a projectable diﬀeomorphism or transformation of

P covering the diﬀeomorphism f

. The projectable diﬀeomorphisms form a

group Diﬀ

(P ), deﬁned by

Diﬀ

(P ):={f ∈ Diﬀ(P ) | f is projectable}.

Let φ

be a one-parameter group of projectable transformations of P with

the associated vector ﬁeld X ∈X(P )andletφ

be the corresponding one

parameter group in Diﬀ(M) with the associated vector ﬁeld X

∈X(M).

Then X is a projectable vector ﬁeld on P , i.e., the pair of vector ﬁelds

(X, X

) satisﬁes the condition

∗

(X(u)) = X

(π(u)), ∀u ∈ P.

The set X

(P ) of projectable vector ﬁelds forms a Lie subalgebra of the

Lie algebra X(P ). The group Diﬀ

(P ) (resp., the algebra X

(P )) and its

subgroups (resp., subalgebras) arise in many applications. They are usually

obtained by requiring that the transformations occurring in them preserve

some additional structure on the ﬁber bundle. For example, condition (6.9)is

182 6 Theory of Fields, I: Classical

satisﬁed if f ∈ Diﬀ(P )isG-equivariant, i.e., f(ug)=f (u)g, ∀u ∈ P, g ∈ G.

We are thus led to deﬁne the set Aut(P )by

Aut(P ):={f ∈ Diﬀ(P ) | f is G-equivariant}. (6.10)

The set Aut(P ) is a group called the group of generalized gauge transfor-

mations. From the point of view of diﬀerential geometry, the group Aut(P )

is just the group of principal bundle automorphisms of P .Wenotethat

the ﬁber-preserving property of the generalized gauge transformation f com-

pletely determines the diﬀeomorphism f

. We deﬁne the group of gauge

transformations G(P ) to be the subgroup Aut

(P ) of the group Aut(P)of

generalized gauge transformations. Thus

G(P ):=Aut

(P )={f ∈ Aut(P ) | f

= id

}. (6.11)

Then G(P ) (also denoted simply by G) is a normal subgroup of Aut(P ).

From deﬁnition (6.11) it is clear that f ∈Gif and only if it is a smooth

ﬁber-preserving map of P into itself commuting with the action of the gauge

group G on P , i.e., f satisﬁes the conditions

π ◦ f = π (6.12)

and

f(p ·g)=f(p) · g, ∀p ∈ P, ∀g ∈ G. (6.13)

From deﬁnitions (6.10)and(6.11) we obtain the following exact sequence

of groups:

−→Aut(P )

−→ Diﬀ(M),

where i denotes the inclusion map and j is deﬁned by

j(f)=f

, ∀f ∈ Aut(P ).

Note that the exactness means here that Im(i)=Ker(j). The map i is injec-

tive, so by adding the identity at the beginning of the above sequence we get

a 4-term exact sequence. However, the map j is not, in general, surjective.

Thus, the 4-term exact sequence cannot be extended to a 5-term or short

exact sequence. The following example

illustrates this.

Example 6.2 Recall that the principal U (1) bundles over S

are classiﬁed

by the integers. For each n ∈ Z there exists a unique equivalence class P

U(1) bundles over S

. As we observed in Example 6.1, this corresponds to

Dirac’s monopole quantization condition. The Hopf ﬁbration of S

discussed

there is in the class P

.Nowletα : S

→ S

be the antipode map, i.e.,

α(x)=−x, ∀x ∈ S

. Then the pull-back bundle of the Hopf ﬁbration α

∗

)

This example was suggested by Stefan Wagner, a doctoral student of Prof. Neeb at TU

Darmstadt.

6.3 Gauge Fields 183

is also a principal U (1) bundle. But this bundle is in the class P

−1

.Thusα

cannot be lifted to an automorphism of the Hopf ﬁbration.

This example leads to the following proposition.

Proposition 6.1 Let Diﬀ

(M) denote the subgroup of Diﬀ(M) deﬁned by

Diﬀ

(M):={α ∈ Diﬀ(M) | α

∗

(P ) ≡ P }.

Then we have the short exact sequence

1 −→ G

−→ Aut(P )

−→ Diﬀ

(M) −→ 1.

In several applications one is interested in splitting the above exact se-

quence or in ﬁnding conditions that imply the equality Diﬀ

(M)=Diﬀ(M)

so that one may try to construct an extension of Diﬀ(M)byG. Additional

geometric structures may also be involved in this process. For example, if

P = L(M), the bundle of frames of M , then it is a principal bundle but

carries the additional structure given by the soldering form θ and we have

the following proposition.

Proposition 6.2 Let M be a manifold with a linear connection. Then there

exists a natural lift λ :Diﬀ(M ) → Diﬀ(L(M)), which splits the exact sequence

of groups

1 −→ G

−→ Diﬀ

(L(M))

−→ Diﬀ(M) −→ 1.

Furthermore, the map f ∈ Diﬀ(L(M )) is the natural lift of a diﬀeomorphism

∈ Diﬀ(M), i.e., f = λ(f

) if and only if f leaves the soldering form

invariant (i.e., f

∗

θ = θ).

When M is a 4-dimensional Lorentz manifold, connections on the frame

bundle L(M) play the role of gravitational potentials. Action functionals

involving connections and metrics on M form the starting point of gauge

theories of gravitation.

A physical interpretation of a gauge transformation f ∈Gis that f is a

local (i.e., pointwise) change of gauge For this reason, G is sometimes

called a local symmetry group and G is called the local gauge group; but

we will not use this terminology. Let t be a local gauge over U.Thent is a

section of the bundle P

, i.e., for x ∈ U , t(x)isinP

= π

−1

(x), the ﬁber of

P over x and f(t(x)) is also in P

. Therefore, there exists a unique element

f(x) ∈ G such that

f(t(x)) = (t(x)).(

f(x)), ∀x ∈ U.

The map

f : U → G is a local representation of the gauge transformation f .

If the bundle P is trivial, then we can take U = M and in this case a gauge

transformation can be identiﬁed with a map of M to G.

184 6 Theory of Fields, I: Classical

We now consider two alternative deﬁnitions of the group of gauge transfor-

mations. Note ﬁrst that the space F(P, G) of all smooth functions f : P → G

with pointwise multiplication is a group. Let F

(P, G) denote the subset of

all G-equivariant functions (with respect to the adjoint action), i.e.,

(P, G):={f : P → G | f(uα)=α

−1

f(u)α, ∀u ∈ P, ∀α ∈ G}.

Then it is easy to verify that F

(P, G) is a group. Let Ad(P )denotethe

bundle (P ×

G)overM associated to P by the adjoint action of G on itself.

It is a bundle of Lie groups with ﬁber G.ThesetΓ (Ad(P )) := Γ (P ×

of sections of the associated bundle Ad(P ) with pointwise multiplication is

a group. The relation between these groups is established in the following

theorem.

Theorem 6.3 There exists an isomorphism between each pair of the follow-

ing three groups:

1. the group of gauge transformations G;

2. the group F

(P, G) of all functions f : P → G such that f is G-

equivariant, with respect to the adjoint action of G on itself;

3. the group Γ (Ad(P )) of sections of the associated bundle Ad(P ) over M .

Proof :Forg ∈Gwe deﬁne ¯g : P → G by

¯g(u)=a,

where a ∈ G is the unique element such that g(u)=ua.Itcanbeveriﬁed

that the map T : g → ¯g is a one-to-one correspondence from G to F

(P, G)

with inverse given by the map from F

(P, G)toG such that f → g

where

(u)=uf(u). Using the deﬁnition of T and of the G action it is easy to

verify that

T (gh)=

gh =¯g

h = T (g)T (h).

It follows that T is an isomorphism of groups.

The correspondence between F

(P, G)andΓ (P ×

G) is a special case of

the correspondence between F

(P, F )andΓ (E(M, F,r,P)) (see Chapter 4)

with F = G and r = Ad, the adjoint action of G on itself. Thus, f ∈F

(P, G)

corresponds to a section s

∈ Γ (P ×

G) deﬁned by

(x)=f(u),u∈ π

−1

(x).

We note that s

is well deﬁned in view of the G-equivariance of f.Onthe

other hand a section s ∈ Γ (P ×

G) deﬁnes an element f

∈F

(P, G)by

(u)=s(x),u∈ π

−1

(x).

One can verify that the map S deﬁned by S : f → s

is an isomorphism of

the group F

(P, G) with the group Γ (P ×

G). 

6.4 The Space of Gauge Potentials 185

In view of the above theorem we use any one of the three representations

above for the group of gauge transformations as needed. For example, re-

garding G as the space of sections of (P ×

G), the bundle of groups (not

a principal G-bundle), we can show that a suitable Sobolev completion (see

Appendix D) of G (also denoted by G) is a Hilbert Lie group (i.e., G is a

Hilbert manifold with smooth group operations). Let ad denote the adjoint

action of the Lie group G on its Lie algebra g.LetE(M, g, ad,P)bethe

associated vector bundle with ﬁber type g and action ad, the adjoint action

of G on g. Recall that this bundle is a bundle of Lie algebras denoted by

P ×

g or ad P .WedenoteΓ (ad P )byLG; it is a Lie algebra under the

pointwise bracket operation. The algebra LG is called the gauge algebra of

P . It can be shown that a suitable Sobolev completion of LG is a Banach Lie

algebra with well-deﬁned exponential map to G.ItistheLiealgebraofthe

inﬁnite-dimensional Banach Lie group G. An alternative characterization of

the gauge algebra is given by the following theorem.

Theorem 6.4 The set F

(P, g) of all G-equivariant (with respect to the ad-

joint action of G on its Lie algebra g) functions with the pointwise bracket

operation is a Lie algebra isomorphic to the gauge algebra LG.

6.4 The Space of Gauge Potentials

Without any assumption of compactness for M or G it can be shown that G is

a Schwartz Lie group (i.e., a Lie group modeled on a Schwartz space) with Lie

algebra consisting of sections of ad P of compact support. While this approach

has the advantage of working in full generality, the technical diﬃculties of

working with spaces modelled on an arbitrary locally convex vector space

can be avoided by considering Sobolev completions of the relevant objects as

follows. In this section we consider a ﬁxed principal bundle P (M,G)overa

compact, connected, oriented, m-dimensional Riemannian base manifold M

with compact, semisimple gauge group G. These assumptions are satisﬁed

by most Euclidean gauge theories that arise in physical or mathematical

applications. The base manifold is typically a sphere S

or a torus T

their products such as S

×T

.Thus,forn = 4 one frequently considers as

abaseS

×S

,orS

×S

. With appropriate boundary conditions on

gauge ﬁelds one may also include non-compact bases such as R

or R

×S

The gauge group G is generally one of the following: U (n), SU(n), O(n),

SO(n), or one of their products. For example, the gauge group of electroweak

theory is SU(2) ×U(1). As we discussed above, the gauge connections (gauge

potentials) and the gauge ﬁelds acquire physical signiﬁcance only after ﬁeld

equations, to be satisﬁed by them, are postulated. However, the topology and

geometry of the space of gauge connections has signiﬁcance for all physical

theories and especially for the problem of quantization of gauge theories.

They are also fundamental in studying low-dimensional topology. Various

186 6 Theory of Fields, I: Classical

aspects of the topology and geometry of the space of gauge connections and

its orbit spaces have been studied in [22, 153, 233, 234]. We denote by A(P )

the space of gauge potentials or connections on P deﬁned by

A(P ):={ω ∈ Λ

(P, g) | ω is a connection on P }. (6.14)

If P is ﬁxed we will denote A(P )simplybyA and a similar notation will be

followed for other related spaces. From the deﬁnition of connection it follows

that ω

,ω

∈Aimplies that ω

−ω

is horizontal and of type (rmad, g) and,

therefore, deﬁnes a unique 1-form on M with values in the associated bundle

ad P := P ×

g. Then we have that, for a ﬁxed connection α,

∼

{α + π

∗

A | A ∈ Λ

(M,ad P )}. (6.15)

From the above isomorphism it follows that the space A is an aﬃne space

with the underlying vector space Λ

(M,ad P ). Thus, the tangent space T

is isomorphic to Λ

(M,ad P ) and we identify these two spaces. If  , 

is a

G-invariant inner product on g, we have a natural inner product deﬁned on

A as follows. First, for A, B ∈ T

A, we deﬁne

A, B∈F(M)byA, B := g

A



where g

are the components of the metric tensor g on M with respect to

the base {dx

} of T

∗

M and A = A

(x)dx

,B= B

(x)dx

.Wenotethat

(x),B

(x) are elements of the ﬁber of the Lie algebra bundle ad P over

x ∈ M. Then we deﬁne the inner product A, B

,orsimplyA, B,by

A, B



A



, ∀A, B ∈ T

A . (6.16)

The map α →A, B

deﬁnes a weak Riemannian metric or the L

Riemannian metric on A.

We observe that an invariant inner product always exists for semisimple

Lie algebras and is given by a multiple of the Killing form K on g deﬁned by

K(X, Y )=Tr(adX ad Y ).

The inner product deﬁned in (6.16) can be extended to Λ

(M,ad P ). A con-

nection ω on P deﬁnes a covariant derivative

∇

: Λ

(M,ad P ) → Λ

(M,ad P )

which is compatible with the metric on ad P , i.e.,

∇

ψ, φ+ ∇

φ, ψ = X(φ, ψ),

6.4 The Space of Gauge Potentials 187

for all φ, ψ ∈ Λ

(M,ad P )andX ∈X(M ). The covariant derivative has a

natural extension to (ad P )-valued tensors that is also denoted by ∇

.The

corresponding covariant exterior derivative is denoted by d

.Wenowgive

deﬁnitions of several terms that occur frequently in physical applications.

Deﬁnition 6.1 Let (M, g) be a compact, connected, m-dimensional, Rie-

mannian manifold and let P (M, G) be a principal bundle over M with com-

pact, semisimple, n-dimensional gauge group G.Let , 

be a G-invariant

inner product on its Lie algebra g.Forx ∈ M,themetricg

induces inner

products on the tensor spaces and spaces of diﬀerential forms that we also

denote by g

.Let{e

(x)}

1≤i≤n

be a basis for the ﬁber (ad P )

of the Lie al-

gebra bundle ad P .Letα, β ∈ Λ

(M,ad P ) and γ ∈ Λ

(M,ad P ).Locally,we

can write

α(x)=α

(x) ⊗ e

(x), where α

(x) ∈ Λ

(M) and e

(x) ∈ (ad P )

, ∀i,

with similar expressions for β and γ. Then we have the following deﬁnitions:

1. The product α, β∈F(M) is deﬁned by x →α, β

,where

α, β

:= g

(α

(x),β

(x))e

(x),e

(x)

The corresponding local norm |α|∈F(M) is deﬁned by

x →|α|



α, α

, ∀x ∈ M.

2. The inner product α, β ∈ R and the corresponding norm are deﬁned

α, β :=



α, βdv

and α :=



α, α.

3. The formal adjoint of d

: Λ

(M,ad P ) → Λ

p+1

(M,ad P ), denoted by δ

is deﬁned by

d

α, σ = α, δ

σ, ∀σ ∈ Λ

p+1

(M,ad P ).

4. The product α

∧γ ∈ Λ

p+q

(M) is deﬁned by

x → (α

∧γ)

=(α

(x)∧γ

(x))e

(x),e

(x)

∈ Λ

p+q

(M).

5. The bracket [α, γ]

∧

,orsimply[α, γ] of bundle-valued forms, is deﬁned

x → [α, γ]

=(α

(x)∧γ

(x))[e

(x),e

(x)] ∈ Λ

p+q

(M,ad P ).

We note that this product is also denoted by α∧γ.

The group G acts on the space of gauge connections A(P ). We can describe

this action in two diﬀerent ways. Let f ∈G. Then the ﬁrst is the right action

−1 obtained by pulling back the connection form, i.e.,