Lin S.D. Water and Wastewater Calculations Manual

Подождите немного. Документ загружается.

5-day BOD or BOD

are practiced. The BOD progressive curve is shown

in Fig. 1.2.

8.1 First-order reaction

Phelps law states that the rate of biochemical oxidation of organic mat-

ter is proportional to the remaining concentration of unoxidized sub-

stance. Phelps law can be expressed in differential form as follows

(monomolecular or unimolecular chemical reaction):

(1.11)

by integration

or (1.12)

where L

⫽ BOD remaining after time t days, mg/L

⫽ first-stage BOD, mg/L

5 L

5 10

5 e

log

520.434K

t 52k

tln

52K

d L

52K

5 K

Streams and Rivers 15

BOD

EXERTED,

g/L

Carbonaceous

1st sta

Nitrification

2nd stage

TIME

days

Figure 1.2 BOD progressive curve.

⫽ deoxygenation rate, based on e, K

⫽ 2.303k

, per day

⫽ deoxygenation rate, based on 10,

⫽ 0.4343K

⫽ 0.1 at 20⬚C), per day

e ⫽ base of natural logarithm, 2.7183

Oxygen demand exerted up to time t, y, is a first-order reaction (see

Fig. 1.2):

(1.13a)

or based on log

(1.13b)

When a delay occurs in oxygen uptake at the onset of a BOD test, a lag-

time factor t

should be included and Eqs. (1.13a) and (1.13b) become

(1.14a)

(1.14b)

For the Upper Illinois Waterway study, many of the total and NBOD

curves have an S-shaped configuration. The BOD in waters from pools

often consists primarily of high-profile second-stage or NBOD, and

the onset of the exertion of this NBOD is often delayed 1 or 2 days. The

delayed NBOD and the total BOD (TBOD) curves, dominated by the

NBOD fraction, often exhibit an S-shaped configuration. The general

mathematical formula used to simulate the S-shaped curve is (Butts

et al., 1975)

(1.15)

where m is a power factor, and the other terms are as previously defined.

Statistical results show that a power factor of 2.0 in Eq. (1.15) best

represents the S-shaped BOD curve generated in the Lockport and

Brandon Road areas of the waterway. Substituting m ⫽ 2 in Eq. (1.15)

yields

(1.15a)

Example 1: Given K

⫽ 0.25 per day, BOD

⫽ 6.85 mg/L, for a river water

sample. Find L

when t

⫽ 0 days and t

⫽ 2 days.

y 5 L

[1 2 e

st2t

]

y 5 L

[1 2 e

st2t

]

y 5 L

[1 2 10

st2t

]

y 5 L

[1 2 e

st2t

]

y 5 L

s1 2 10

y 5 L

s1 2 e

y 5 L

2 L

16 Chapter 1

solution:

Step 1.

When t

⫽ 0

Another solution using k

: Since

Step 2.

When t

⫽ 2 days, using Butts et al.’s equation (Eq. (1.15a)):

Example 2: Compute the portion of BOD remaining to the ultimate BOD

for k

⫽ 0.10 (or K

⫽ 0.23).s1 2 e

or 1 2 10

5 7.65 smg/Ld

6.85

1 2 0.105

6.85

1 2 e

22.25

6.85

1 2 e

20.25s522d

1 2 e

st2t

y 5 L

[1 2 e

st2t

]

5 9.60 smg/Ld

6.85

1 2 0.286

6.85 5 L

s1 2 10

20.10935

y 5 L

s1 2 10

5 0.4343K

5 0.4343 3 0.25 per day 5 0.109 per day

5 9.60 smg/Ld

6.85

1 2 0.286

6.85 5 L

s1 2 e

20.2535

y 5 L

s1 2 e

Streams and Rivers 17

solution: By Eq. (1.13b)

When t ⫽ 0.25 days

Similar calculations can be performed as above. The relationship between t

and is listed in Table 1.4.

8.2 Determination of deoxygenation rate

and ultimate BOD

Biological decomposition of organic matter is a complex phenomenon.

Laboratory BOD results do not necessarily fit actual stream conditions.

BOD reaction rate is influenced by immediate demand, stream or river

dynamic environment, nitrification, sludge deposit, and types and con-

centrations of microbes in the water. Therefore, laboratory BOD analy-

ses and stream surveys are generally conducted for raw and treated

wastewaters and river water to determine BOD reaction rate.

Many investigators have worked on developing and refining methods

and formulas for use in evaluating the deoxygenation (K

) and reaera-

tion (K

) constants and the ultimate BOD (L

). There are several meth-

ods proposed to determine K

values. Unfortunately, K

values determined

by different methods given by the same set of data have considerable

s1 2 10

5 1 2 10

20.1030.25

5 1 2 0.944 5 0.056

5 1 2 10

t

or  1 2 e

y 5 L

s1 2 10

18 Chapter 1

TABLE 1.4 Relationship between

t and the Ultimate

BOD (1 ⴚ 10

ⴚk

or 1 ⴚ e

ⴚK

)

0.25 0.056 4.5 0.646

0.50 0.109 5.0 0.684

0.75 0.159 6.0 0.749

1.00 0.206 7.0 0.800

1.25 0.250 8.0 0.842

1.50 0.291 9.0 0.874

1.75 0.332 10.0 0.900

2.0 0.369 12.0 0.937

2.5 0.438 16.0 0.975

3.0 0.500 20.0 0.990

3.5 0.553 30.0 0.999

4.0 0.602 ⬁ 1.0

1 2 e

or 1 2 e

1 2 10

or1 2 10

variations. Reed–Theriault least-squares method published in 1927 (US

Public Health Service, 1927) gives the most consistent results, but it is

time consuming and tedious. Computation using a digital computer was

developed by Gannon and Downs (1964).

In 1936, a simplified procedure, the so-called log-difference method

of estimating the constants of the first-stage BOD curve, was presented

by Fair (1936). The method is also mathematically sound, but is also dif-

ficult to solve.

Thomas (1937) followed Fair et al. (1941a, 1941b) and developed the

“slope” method, which, for many years, was the most used procedure for

calculating the constants of the BOD curve. Later, Thomas (1950) pre-

sented a graphic method for BOD curve constants. In the same year,

Moore et al. (1950) developed the “moment” method that was simple, reli-

able, and accurate to analyze BOD data; this soon became the most

used technique for computing the BOD constants.

Researchers found that K

varied considerably for different sources

of wastewaters and questioned the accepted postulate that the 5-day

BOD is proportional to the strength of the sewage. Orford and Ingram

(1953) discussed the monomolecular equation as being inaccurate and

unscientific in its relation to BOD. They proposed that the BOD curve

could be expressed as a logarithmic function.

Tsivoglou (1958) proposed a “daily difference” method of BOD data

solved by a semigraphical solution. A “rapid ratio” method can be solved

using curves developed by Sheehy (1960). O’Connor (1966) modified the

least-squares method using BOD

This book describes Thomas’s slope method, method of moments, log-

arithmic function, and rapid methods calculating K

(or k

) and L

Slope method. The slope method (Thomas, 1937) gives the BOD con-

stants via the least-squares treatment of the basic form of the first-

order reaction equation or

(1.16)

where dy ⫽ increase in BOD per unit time at time t

⫽ deoxygenation constant, per day

⫽ first stage ultimate BOD, mg/L

y ⫽ BOD exerted in time t, mg/L

This differential equation (Eq. (1.16)) is linear between dy/dt and y. Let

y⬘⫽dy/dt to be the rate of change of BOD and n be the number of BOD

measurements minus one. Two normal equations for finding K

and L

are

(1.17)na 1 b⌺y 2 ⌺yr 5 0

5 K

2 yd 5 K

2 K

Streams and Rivers 19

and

(1.18)

Solving Eqs. (1.17) and (1.18) yields values of a and b, from which K

and L

can be determined directly by following relations:

⫽ –b (1.19)

and

⫽ –a/b (1.20)

The calculations include first determinations of y⬘, y⬘y, and y

for

each value of y. The summation of these gives the quantities of ⌺y⬘,

⌺y⬘y, and ⌺y

which are used for the two normal equations. The values

of the slopes are calculated from the given data of y and t as follows:

(1.21)

For the special case, when equal time increments t

i⫹1

– t

⫽ t

– t

⫽

–t

⫽⌬t, y⬘ becomes

(1.21a)

A minimum of six observations (n ⬎ 6) of y and t are usually required

to give consistent results.

Example 1: For equal time increments, BOD data at temperature of 20⬚C,

t and y, are shown in Table 1.5. Find K

and L

solution:

Step 1. Calculate y⬘, y⬘y, and y

Step 2. Determine a and b

Writing normal equations (Eqs. (1.17) and (1.18)), n ⫽ 9

(1)a 1 96.2b 2 9.96 5 0

9a 1 865.8b 2 89.6 5 0

na 1 b⌺y 2 ⌺yr 5 0

i11

2 y

i21

2⌬t

 or

i11

2 y

i21

i11

2 t

i21

5 yr

2 y

i21

i11

2 t

i21

b1 sy

i11

2 y

2 t

i21

i11

2 t

i11

2 t

i21

a⌺y 1 b⌺y

2 ⌺ yyr 5 0

20 Chapter 1

and

(2)

Eq. (2) – Eq. (1)

From Eq. (2)

Step 3. Calculate K

and L

with Eqs. (1.19) and (1.20)

5 115.3 smg/Ld

5259.97/s20.52d

52a/b

5 0.52 sper dayd

52b 52s20.52d

a 5 59.97

a 1 99.97s20.52d 2 8.0 5 0

b 520.52

3.77b 1 1.96 5 0

a 1 99.97b 2 8.0 5 0

865.8a 1 86,555b 2 6926 5 0

a⌺y 1 b⌺y

2 ⌺yyr 5 0

Streams and Rivers 21

TABLE 1.5 Calculations for y ⴕ, y ⴕy, and y

Values

t, day yy⬘ y⬘yy

(1) (2) (3) (4) (5)

1 56.2 37.2

∗

2090.64 3158.44

2 74.4 15.7 1168.08 5535.36

3 87.6 10.9 954.84 7673.76

4 96.2 7.4 711.88 9254.44

5 102.4 5.6 573.44 10485.76

6 107.4 4.3 461.82 11534.76

7 111.0 3.3 366.30 12321.00

8 114.0 2.8 319.20 12996.00

9 116.6 2.4 279.84 13595.56

10 118.8

⌺ 865.8

†

89.6 6926.04 86555.08

†

Sum of first nine observations.

∗

2 y

2 t

74.4 2 0

2 2 0

5 37.2

Example 2: For unequal time increments, observed BOD data, t and y are

given in Table 1.6. Find K

and L

solution:

Step 1. Calculate ⌬t, ⌬y, y⬘, yy⬘, and y

; then complete Table 1.6

From Eq. (1.21) (see Table 1.6)

s28.8da

0.6

0.4

b 1 s27.4da

0.4

0.6

0.4 1 0.6

5 61.47

s⌬y

i21

⌬t

i11

⌬t

i21

b 1 s⌬y

i11

⌬t

i21

⌬t

i11

s⌬t

i21

d 1 s⌬t

i11

∗

2 y

i21

i11

2 t

i21

b 1 sy

i11

2 y

2 t

i21

i11

2 t

i11

2 t

i21

22 Chapter 1

TABLE 1.6 Various t and y Values

t ⌬ty⌬yy⬘ yy⬘ y

0.4 28.8

0.4 28.8 61.47

∗

1770.24 829.44

0.6 27.4

1 56.2 30.90 1736.75 3158.44

0.5 9.3

1.5 65.5 19.48 1276.00 4290.25

0.7 14.5

2.2 80.0 15.48 1238.48 6400.00

0.8 7.6

3 87.6 9.10 797.16 7673.76

1 8.6

4 96.2 7.40 711.88 9254.44

1 6.2

5 102.4 5.57 570.03 10485.76

2 8.6

7 111.0 3.55 394.05 12321.00

2 5.6

9 116.6 2.43 282.95 13595.56

3 5.6

12 122.2

Sum 744.3 155.37 8777.53 68008.65

∗

See text for calculation of this value.

Step 2. Compute a and b; while n ⫽ 9

(1)

and

(2)

Eq. (2) – Eq. (1)

with Eq. (2)

Step 3. Determine K

and L

Moment method. This method requires that BOD measurements must

be a series of regularly spaced time intervals. Calculations are needed

for the sum of the BOD values, ⌺y, accumulated to the end of a series

of time intervals and the sum of the product of time and observed BOD

values, ⌺ty, accumulated to the end of the time series.

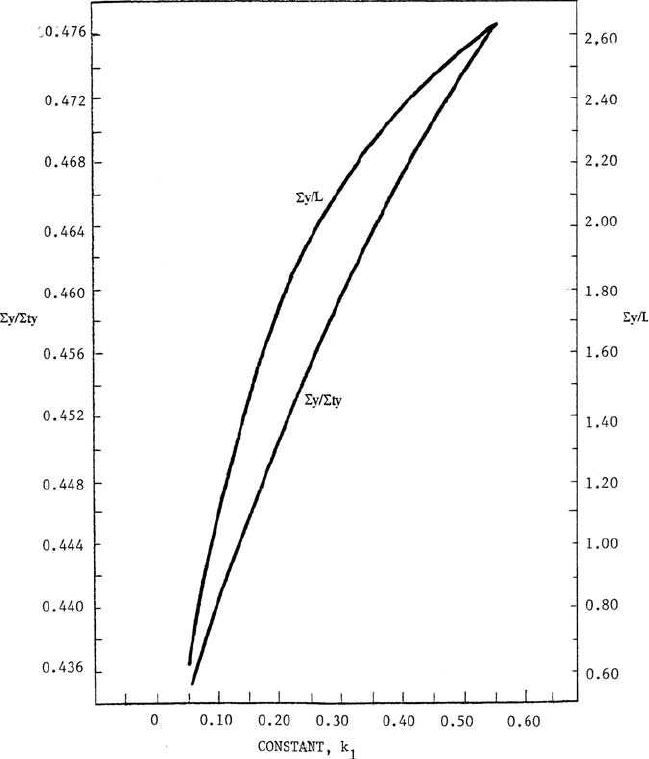
The rate constant K

and the ultimate BOD L

can then be easily

read from a prepared graph by entering values of ⌺y/⌺ty on the appro-

priate scale. Treatments of BOD data with and without lag phase will

be different. The authors (Moore et al., 1950) presented three graphs for

5 110.1 smg/Ld

5269.35/20.63

52a/b

52b 5 0.63 sper dayd

a 5 69.35

a 1 91.37s20.63d 2 11.79 5 0

b 520.63

8.67b 1 5.47 5 0

a 1 91.37b 2 11.79 5 0

744.3a 1 68,008.65b 2 8777.53 5 0

a⌺y 1 b⌺y

2 ⌺yyr 5 0

a 1 82.7b 2 17.26 5 0

9a 1 744.3b 2 155.37 5 0

na 1 b⌺y 2 ⌺yr 5 0

Streams and Rivers 23

3-, 5-, and 7-day sequences (Figs. 1.3, 1.4, and 1.5) with daily intervals

for BOD value without lag phase. There is another chart presented for

a 5-day sequence with lag phase (Fig 1.6).

Example 1: Use the BOD (without lag phase) on Example 1 of Thomas’

slope method, find K

and L

solution:

Step 1. Calculate ⌺y and ⌺ty (see Table 1.7)

24 Chapter 1

Figure 1.3 ⌺y/L and ⌺y/⌺ty for various values of k

in a 3-day sequence.