Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications

Подождите немного. Документ загружается.

frequency,

rL

and

r

1

are

the

transverse

and

longitudinal

relaxation

rates

respectively,

and

n

the

density

of

the

atoms.

Let

us

limit

our

considerations

to

fast

transverse

relaxation,

which

means

that

the

polarization

follows

the

electric

field

adiabatically

(adiabatic

elimination

method)

i.e.,

aa;ar

~

o, we

have

a=

iJ,LNE/(i.t.n-

r_L).

(7.3-2)

Substituting

Eq.(7.3-2)

into

Eq.(7.3-la),

then

the

electric

field

can

be

written

in

the

integral

form.

E(r+zjc,z)

=

E(r,O)exp[28W(r,z)(i.t.fl

+

r_L)/(.t.fl'+rL')],

(7.3-3)

where

8 =

27TnkJ,L'

and

W(r,z)

=

foz

N(r

+

z'jc,z')dz'.

(7.3-4)

Substituting

Eqs.(7.3-3)

and

(7.3-4)

into

Eq.

(7.3-lb)

and

then

integrating

over

z,

one

has

aw(r,z);ar

=-

r

1

cw

+

z/2)

-

J.L'IE(r,O)

I'

(exp[4SrLW/(.t.n'

+

rL')]

-1)/48.

(7.3-5)

Introducing

the

dimensionless

quantities

E(t,z)

=

J.L

E(t,z)jzjrLr

1

(1

+

.t.'

),

x =

tr

1

,

cp(t) =

W(t-

J;./c,L)/L,

(7.3-6)

.t.

= .t.n;rL.

Combining

Eqs.(7.3-3)

and

(7.3-5)

together

with

the

boundary

conditions

with

the

dimensionless

quantities

in

(7.3-6)

we

have

the

following

set

of

equations

which

do

not

involve

the

optical

coordinates:

€(X,O)

=

)T€

1

(X)

+

RE(x-k,O)exp(aLcf>(x))exp(i(aL.t.(cp(x)-~)-6

0

},

(7.3-7a)

dcp(x)jdx

=

-(cp(x)+~)

-

2IE(x-k,O)

I'

[exp(2aLcf>(x))-1]/aL,

(7.3-7b)

€r

=

)TE(x-k,O)exp(aLcf>(x))exp(i(aL.t.(cp(x)+~)-(6

0

+kl)

},

where

and

is

the

Er(x)

=

J.LEr(t-

l/c)/2(rLr

1

(1

+ .t.')}v.,

€

1

(X)

=

J,LE

1

(t)/2(rLr

1

(1

+

.t.

2

)

}%,

a =

2Srv(.t.fl

2

+

rL')

I

effective

absorption

coefficient,

and

344

(7.3-8)

6

0

= -

k(/~L

+

1)

+

27fM,

(where

£

1

1

51-

47fn~

2

A0/(A0

2

+

ri

2

)

is

the

linear

dielectric

constant,

and

27fM

is

the

multiple

of

27f

nearest

to

k(JE7'L+l))

being

the

mistuning

parameter

of

the

ring

cavity

and

k =

r

1

~;c

being

the

dimensionless

round

trip

time.

Eqs.(7.3-7a,b)

and

(7.3-

8)

can

be

interpreted

as

difference

-

differential

equations

and

whose

solutions

are

uniquely

determined

by

the

initial

value

~(0)

and

the

boundary

condition

E(x,O)

for

- k S x < o.

For

the

stationary

case,

we

can

set

d~(x)jdx

= o

and

E(x,O)

=constant.

By

eliminating

E(x,O)

from

Eqs.(7.3-7a,b)

and

(7.3-8),

one

obtains

the

relationship

between

the

stationary

solution

of

the

transmitted

field

and

the

incident

field:

1£

I•=

I;

I•

(exp(-ar_4)

-

R]'

I T A

,..

+

4Rexp(-a~)sin•

[6(1Eri

2

)/2]/T

2

}.

where

6(1;r1

2

)

a 6

0

-

aL

(~

+

1/2),

"'

(7.3-9)

(7.3-10)

and

~

denotes

the

stationary

solution

and

~

is

related

to

I

ET

I•

by

($

+

1/2)/[exp(-2ar_4)

-

1]

=

2l;ri

2

/TaL.

(7.3-11)

Here$

is

a

monotonic

increasing

function

of

1£~1·

from

-1/2

to

zero.

Also,

note

that

6(1~1·>

denotes

the

intensity

dependent

mistuning

parameter,

which

is

originated

from

the

nonlinear

shift

of

wavenumber

(or

frequency)

in

the

absorber.

In

the

limit

of

aLA~

0

and

6

0

~

o,

Eqs.(7.3-9)

and

(7.3-11)

reduce

to

the

absorptive

bistability

obtained

by

Bonifacio

and

Lugiato

[1978].

on

the

other

hand,

if

the

conditions

aLA<<

1,

aL

<<

1,

16

0

1

<<

1

and

l;ri•;T

<<

1

are

satisfied,

then

Eq.(7.3-9)

reduces

to

l£

1

1

2

=

ltrl•

[1

+

4R(aLAI~I·/T-

6of2)

2

/T

2

],

(7.3-12)

by

the

approximation

6(1£~1·>::::

6

0

-

2aLAIE'ri•;T.

Eq.(7.3-12)

agrees

with

the

relation

obtained

by

Gibbs

et

al

[1976]

exhibiting

the

dispersive

bistability

experimentally.

If

the

parameter

aLA

is

sufficiently

large,

the

transmitted

field

oscillates

as

a

function

of

the

incident

field

due

to

the

factor

sin•

[6(1;rl•)j2].

Consequently,

the

345

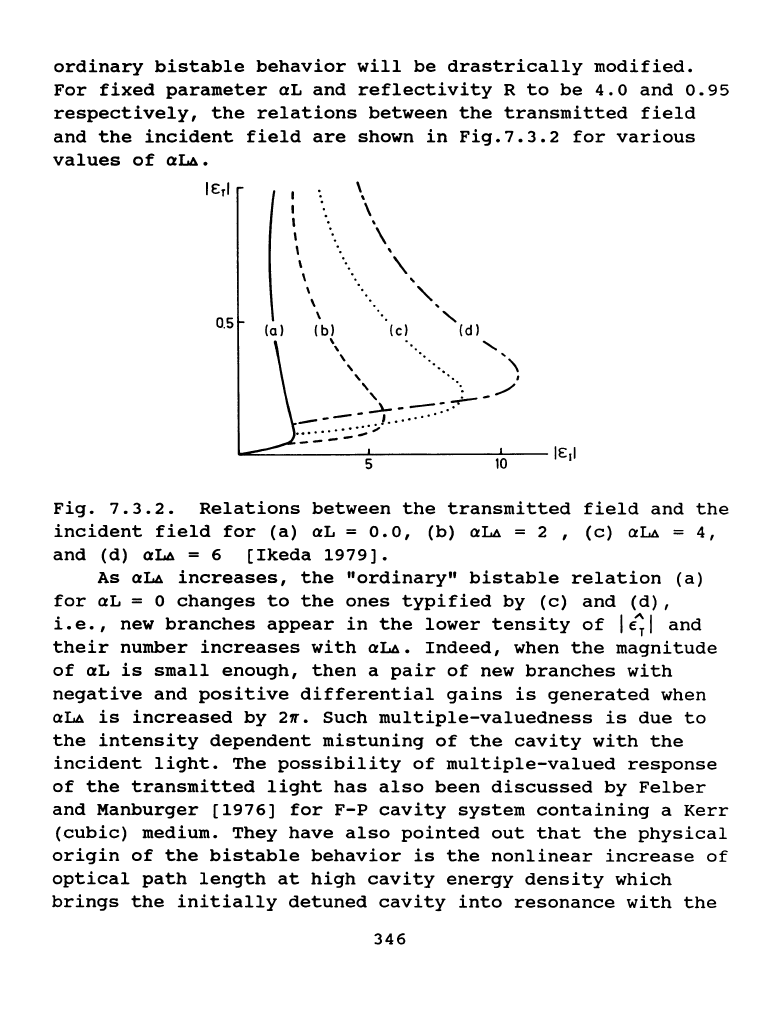
ordinary

bistable

behavior

will

be

drastrically

modified.

For

fixed

parameter

aL

and

reflectivity

R

to

be

4.0

and

0.95

respectively,

the

relations

between

the

transmitted

field

and

the

incident

field

are

shown

in

Fig.7.3.2

for

various

values

of

aLt..

I

Erl

0.5

(a)

I

I

I

I

I

I

'

\

'

\

(b)

\

'

'

\

'

\

'

\

·.

'

'

(c)

'

'

(d)

'

··

..

.......

',

·..

..

..

:

~---.~

.-:::~~-~-;}·········

Fig.

7.3.2.

Relations

between

the

transmitted

field

and

the

incident

field

for

(a)

aL

=

0.0,

(b)

aLt. = 2 ,

(c)

aLt.

4,

and

(d)

aLt.=

6

[Ikeda

1979].

As aLt.

increases,

the

"ordinary"

bistable

relation

(a)

for

aL

= 0

changes

to

the

ones

typified

by

(c)

and

(d),

i.e.,

new

branches

appear

in

the

lower

tensity

of

IE~I

and

their

number

increases

with

aLt..

Indeed,

when

the

magnitude

of

aL

is

small

enough,

then

a

pair

of

new

branches

with

negative

and

positive

differential

gains

is

generated

when

aLt.

is

increased

by

2".

Such

multiple-valuedness

is

due

to

the

intensity

dependent

mistuning

of

the

cavity

with

the

incident

light.

The

possibility

of

multiple-valued

response

of

the

transmitted

light

has

also

been

discussed

by

Felber

and

Manburger

[1976]

for

F-P

cavity

system

containing

a

Kerr

(cubic)

medium.

They

have

also

pointed

out

that

the

physical

origin

of

the

bistable

behavior

is

the

nonlinear

increase

of

optical

path

length

at

high

cavity

energy

density

which

brings

the

initially

detuned

cavity

into

resonance

with

the

346

field.

Once

the

resonance

is

achieved

the

transmissivity

is

high.

For

the

discussion

of

the

stability

of

the

multiple-valued

stationary

state,

we

shall

consider

the

limiting

case

k <<

1,

i.e.,

very

short

cavity

round

trip

time.

In

this

limit,

we may

set

d¢(x)jdx

in

Eq.(7.3-7b)

equal

to

zero.

Then

Eqs.(7.3-7a,b)

and

(7.3-8)

reduce

to

the

following

difference

equations:

eo,n

=

fTe

1

n +

Re

0

,n_

1

exp(a14>n)

exp{

i

(aLA

(¢n+~)

-

c5

0

)},

(7.3-13a)

(7.3-13b)

where

E

0

n'

ETn'

and

e

1

n

denote

e

(x

0

+nk,

0),

e

1

(x

0

+nk)

and

e

1

(x

0

+nk)

respectively,

and

¢n

relates

with

e

0

n-l

by

(¢n +

1/2)/[1-

exp(a14>n)l =

2leo,n-,1•;a

'

(7.3-14)

It

should

be

noted

that

when

aL

=

c5

0

= 0

one

can

choose

E

0

n

as

real,

thus

Eq.(7.3-13a)

can

be

solved

by

graphical

'

method.

It

can

be

shown

that

in

the

case

of

pure

absorptive

bistability,

the

branch

with

the

positive

differential

gain

is

always

stable.

The

stability

problem

in

general

has

not

been

properly

analyzed

as

yet.

Note

that

as

we

have

discussed

earlier

in

Chapter

6,

in

particular,

the

last

section,

the

proper

mathematical

setting

and

their

techniques

are

readily

available.

See

elsewhere

in

these

Notes

and

references

therein.

For

more

detail

discussion

of

the

Maxwell-Bloch

theory

of

ring

laser

cavity,

see,

for

instance,

Lugiato

et

al

[1987],

Risken

[1986:

1988],

Milonni

et

al

[1987].

It

suffices

to

say

that

from

Eqs.(7.3-1)

one

can

identify,

formally

and

intuitively,

that

the

Maxwell-Bloch

equations

are

closely

related

to

the

Lorenz

equations,

and

consequently

the

unstable

region

of

the

parameter

space.

Some

detailed

derivations

of

this

relationship

can

be

found

in

Milani

et

al

[1987].

The

Lorenz

model

has

been

well-studied,

and

we

shall

not

go

into

any

detail

here.

One

would

expect

that

the

projection

of

the

phase

space

trajectory

in

the

E-N-plane

will

produce

the

"butterfly"

347

figures

as

in

the

Lorenz

model.

Indeed,

one

would.

Since

the

electric

field

amplitude

is

not

directly

observable,

it

is

interesting

to

know how

to

distinguish

a

symmetry

breaking

transformation

using

traditional

measurements.

The

solution

is

the

application

of

heterodyne

techniques

using

a

stable

reference

source.

For

heterodyne

techniques

in

infrared

and

optical

frequencies,

see

for

instance,

Kingston

[1978].

It

suffices

to

say

that

the

main

differnce

between

a

symmetric

and

an

asymmetric

electric

fields

is

that

the

symmetric

one

has

a

zero

average

electric

field,

while

the

asymmetric

one

has

non-zero

average

electric

field.

Thus,

the

heterodyne

spectrum

of

a

symmetric

solution

has

symmetric

frequency

components

around

In

- n

0

l

but

with

zero

spectral

power,

while

an

asymmetric

solution

displays

a

distinguishing

signature

of

having

a

line

at

the

beat

frequency

In

- n

0

l

and

with

symmetric

sidebands.

It

should

be

noted

that

a

survey

of

the

available

experiments

indicates

that

significant

areas

of

disagreement

still

exist

between

the

theoretical

predictions

of

the

Maxwell-Bloch

model

and

the

experimental

data.

Indeed,

nobody

really

knows how

to

model

the

active

medium

with

sufficient

accuracy,

in

particular,

for

solid

state

lasers.

Even

for

gas

lasers,

which

can

operate

with

a

homogeneous

broadened

gain

and

yet

show

behavior

which

is

not

compatible

with

the

usual

descriptions.

In

fact,

Lippi

et

al

[1986]

have

shown

that

the

C0

2

laser

whose

unstable

behavior

near

threshold

is

in

striking

difference

with

the

theoretical

descriptions.

Hendow

and

Sargent

[1982;

1985]

and

Narducci

et

al

[1986]

have

discovered

a new

type

of

instability,

the

phase

instability,

and

its

dynamical

origin

can

be

attributed

by

the

loss

of

phase

stability

instead

of

its

amplitude.

The

experimental

confirmation

showed

good

qualitative

agreement

between

the

theoretical

predictions

and

the

experimental

observations

[Treducci

et

al

1986].

Experiments

have

also

shown

that

frequent

appearance

of

regular

and

chaotic

pulsations

in

inhomogeneous

broadened

lasers

[Casperson

348

1978; 1980;

1981;

1985;

Bentley

and

Abraham

1982;

Maeda

and

Abraham

1982;

Abraham

et

al

1983;

Gioggia

and

Abraham

1983;

1984].

An

important

warning

about

the

adequacy

of

the

plane-wave

approximation

came

from

the

lack

of

quantitative

agreement

between

the

predictions

of

the

plane-wave

stationary

theory

of

optical

bistability

[Bonifacio

and

Lugiato

1976;

1978;

Lugiato

1984],

and

the

failure

of

the

time-dependent

plane-wave

calculations

to

match

the

observed

pulsing

pattern

[Lugiato

et

al

1982].

Indeed,

a

growing

number

of

experimental

and

theoretical

results

support

the

view

that

transverse

effects

play

a

significant

role

and

maybe

even

more

influential

when

the

optical

resonator

contains

an

active

medium

[LeBerre

et

al

1984;

Valley

et

al

1986;

Derstine

et

al

1986;

McLaughlin

et

al

1985;

Moloney

et

al

1982;

Moloney

1984].

There

is

another

type

of

optical

instability

which

is

very

distinct

from

the

optical

chaos

mentioned

above

relating

to

the

Lorenz

model

of

a

nonlinear

dynamical

system.

This

other

type

of

optical

instability

is

due

to

nonlinear

delay

feedback,

which

is

related

to

the

iterative

maps.

In

the

following,

the

stability

results

of

linearized

equations

due

to

Ikeda

[1979]

is

presented.

The

linear

motion

of

eo,n

around

its

stationary

solution

is

characterized

by

two

eigenvalues

of

a 2x2

evolution

matrix,

and

the

stationary

solution

is

stable

only

if

each

of

the

eigenvalues

has

an

absolute

value

less

than

unity.

The

stability

has

also

been

studied

numerically.

As

expected,

the

branches

with

negative

differential

gain

dlerl/dle

1

1 < 0

are

always

unstable.

It

is

interesting

to

note

that

even

the

branch

with

positive

differential

gain

is

not

always

stable.

The

stationary

solution

becomes

stable

when

le

1

1

is

set

in

the

vicinity

of

the

supremum

or

infimum

of

the

branch,

as

illustrated

in

the

following

figure.

349

I

I

I

I

I

I I

l.

... ····i··

,......-;

I

'-....~......

I

I ··i.")

I k

,.---,··········1

..

B

.... I I

I I

....

)

c

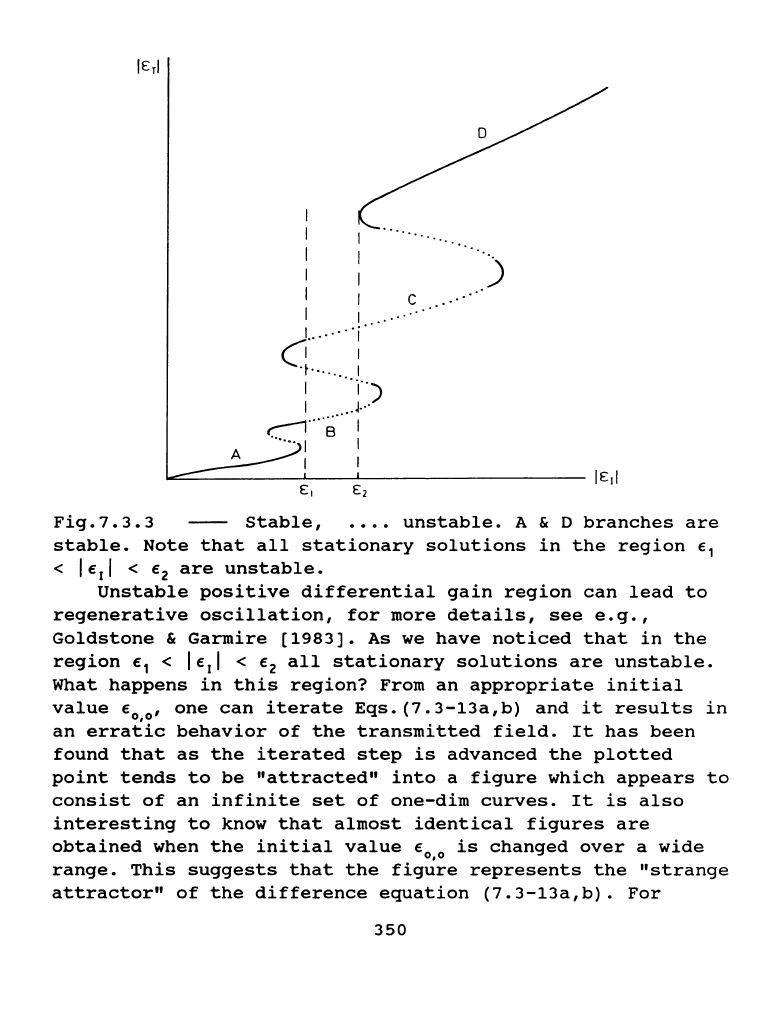
Fig.7.3.3

Stable,

unstable.

A & D

branches

are

stable.

Note

that

all

stationary

solutions

in

the

region

e

1

< le

1

1 < e

2

are

unstable.

Unstable

positive

differential

gain

region

can

lead

to

regenerative

oscillation,

for

more

details,

see

e.g.,

Goldstone

&

Garmire

(1983].

As

we

have

noticed

that

in

the

region

e

1

< le

1

l < e

2

all

stationary

solutions

are

unstable.

What

happens

in

this

region?

From

an

appropriate

initial

value

e

0

,

0

,

one

can

iterate

Eqs.

(7.

3-13a,b)

and

it

results

in

an

erratic

behavior

of

the

transmitted

field.

It

has

been

found

that

as

the

iterated

step

is

advanced

the

plotted

point

tends

to

be

"attracted"

into

a

figure

which

appears

to

consist

of

an

infinite

set

of

one-dim

curves.

It

is

also

interesting

to

know

that

almost

identical

figures

are

obtained

when

the

initial

value

E

0

,

0

is

changed

over

a

wide

range.

This

suggests

that

the

figure

represents

the

"strange

attractor"

of

the

difference

equation

(7.3-13a,b).

For

350

details,

see,

Ikeda

[1979);

Ikeda,

Daido

and

Akimoto

[1980,

1982].

For

more

general

discussion

on

iterate

maps,

see,

Collet

and

Eckmann

[1980).

For

more

detailed

discussions

and

further

references

on

optical

bistability,

see,

Bowden,

Ciftan

and

Robl

[1981);

Bowden,

Gibbs

and

McCall

[1984);

Gibbs

[1985);

Gibbs,

Mandel,

Peyghambarian

and

Smith

[1986);

Goldstone

[1985);

Lugiato

[1984);

Zhang

and

Lee

[1988].

Gibbs

[1985)

provides

the

most

comprehensive

treatment

of

optical

bistability

up

to

1984-5,

including

references.

Goldstone's

review

article

is

also

recommended.

For

recent

results,

see

those

conference

proceedings.

There

are

some

review

articles

on

optical

instabilities,

e.g.,

Boyd,

Raymer

and

Narducci

[1986);

Narducci

and

Abraham

[1988);

Orayevskiy

[1988);

Chrostowski

and

Abraham

[1986],

Milonni

et

al

[1987],

and

forthcoming

conference

proceedings.

7.4

Chemical

reaction-diffusion

equations

The

usual

study

of

chemical

processes

is

concentrated

in

that

several

chemical

reactants

are

put

together

at

a

certain

time

and

then

processes

taking

place

are

then

studied.

In

equilibrium

thermodynamics,

one

usually

compares

only

the

reactants

and

the

final

products

and

observes

the

rate

as

well

as

the

direction

of

a

process

taking

place.

Here

we

would

like

to

consider

the

following

situation

which

can

be

served

as

a

model

for

biochemical

processes.

Let

us

suppose

several

reactants

are

continuously

put

into

a

reactor

vessel

where

new

chemicals

are

continuously

produced

and

the

end

products

are

then

removed

in

such

a

way

that

they

satisfy

steady

state

conditions.

Certainly,

these

processes

can

be

maintained

for

any

finite

amount

of

time

only

under

conditions

far

from

thermal

equilibrium.

Indeed,

a

number

of

interesting

questions,

such

as,

under

what

conditions

can

we

get

certain

products

in

a

well-controlled

large

concentration?

Can

such

processes

produce

some

spatial

or

temporal

patterns?

Some

of

the

answers

may

have

some

351

bearing

on

the

formation

of

structures

in

biological

systems

and

on

the

theories

of

evolution.

Just

to

illustrate

the

applications,

in

the

following

we

shall

discuss

deterministic

reaction

equations

with

diffusion,

leaving

the

stochastic

point

of

view

as

an

"after

thought".

Before

we

get

into

any

specific

reaction

processes,

it

suffices

to

say

that

the

time

rate

of

change

of

the

concentration

of

a

given

species

can

be

related

to

the

rate

constants,

concentrations

of

other

species,

reverse

reaction

rate

constants,

losses,

and

diffusion

terms,

which

can

be

written

as

dnjdt

=

DnV

2

n +

g(n).

(7.4-1)

For

some

situations,

g(n)

may

be

derived

from

a

potential

V,

i.e.,

g(n)

=-

av;an.

(7.4-2)

When

studying

the

steady

state,

dn/dt

= o,

we

want

to

derive

a

criterium

for

the

coexistence

of

two

phases,

i.e.,

we

consider

a

situation

in

which

we

have

a

change

of

concentration

within

a

certain

layer.

To

study

the

steady

state

equation,

we

may

invoke

an

analogy

with

an

oscillator,

or

more

generally,

with

a

particle

under

the

influence

of

the

potential

V(n)

by

noting

the

following

correspondence

(just

for

one-dimension):

x

~

time,

V

~

potential,

n

~

coordinate.

Now

let

us

consider

a

reaction-diffusion

model

with

two

or

three

variables:

A ~

X,

B + X

~

Y +

D,

2X

+ Y

~

3X,

X

~

E,

between

molecules

of

the

species

A, B,

D,

E,

X,

Y.

Only

the

following

corresponding

concentrations

enter

into

the

equations

of

the

chemical

reaction,

a,

b,

n

1

,

and

n

2

for

A,

B,

X,

and

Y

respectively.

We

shall

treat

a

and

b

fixed,

whereas

n

1

and

n

2

are

treated

as

variables.

Thus

the

reaction-diffusion

equations

read

as:

an,;at

=a-

(b

+

l)n,

+

n,•n2

+

o,V>n,

(7.4-3)

an

2

;at

=

bn

1

-n

1

•

n

2

+ D

2

V

2

n

2

, (7

.4-4)

where

D

1

and

D

2

are

diffusion

coefficients.

For

simplicity,

let

us

only

consider

one

spatial

dimension.

We

shall

subject

the

concentrations

n

1

and

n

2

to

two

kinds

of

boundary

352

conditions;

either

n

1

(0,t)

= n

1

(1,t)

=a,

n

2

(0,t)

= n

2

(1,t)

=

b/a,

or

n

1

and

n

2

remain

finite

for

x

~

±

~.

(7.4-5)

(7.4-6)

One

can

easily

verifies

that

the

stationary

state

of

Eqs.(7.4-

3,4)

is

given

by

n

15

=

a,

n

25

=

bja.

(7

.4-7)

In

order

to

see

whether

any

new

solution

classes

can

occur,

i.e.,

if

any

new

spatial

andjor

temporal

structure

may

arise,

we

can

perform

a

linear

stability

analysis

of

the

reaction-diffusion

equations,

Eqs.(7.4-3,4).

Let

n

1

= n

15

+ q

1

;

n

2

= n

25

+ q

2

,

(7.4-8)

and

linearize

Eqs.(7.4-3,4)

with

respect

to

q;'s.

It

is

straight

forward

that

the

linearized

equations

are

aq,;at

=

(b

-

1)

q,

+

a•

qz

+

o,a•

q,;ax•

'

aq

2

;at

= -

bq

1

-

a•

q

2

+ D

2

a•

q

2

jax•

•

The

boundary

conditions

(7.4-5,6)

require

that:

q

1

(O,t)

= q

1

(1,t)

= q

2

(0,t)

= q

2

(1,t)

= o,

and

q;'s

remains

finite

for

x

~

±

~.

(7.4-9)

(7.4-10)

(7

.4-11)

Treating

q

as

a

column

matrix,

then

Eqs.(7.4-9,10)

can

be

written

as

aq~at

=

Lq,

where

L =

[o,a•

;ax•

+ b - 1

a•

J

-b

o

2

a•

;ax•

-

a•

(7.4-12)

(7.4-13)

One

can

satisfy

the

boundary

conditions

(7.4-11)

by

setting

q(x,t)

~exp(~

1

t)sin(l~x),

with

1 =

1,2,

•••

As

usual,

solving

the

characteristic

equation

~·

-

a~

+

f3

= o,

where

a=

(-D

1

'

+

b-

1 D

2

' -

a•),

f3

=

(-D

1

' +

b-

1)

(-D

2

' -

a•)

+

ba

2

,

and

D;'

=

D;P~·,

i =

1,

2.

(7.4-14)

(7.4-15)

One

can

easily

show

that

an

instability

occurs

if

Re(~)

>

0.

For

fixed

a

but

changing

the

concentration

b

beyond

the

critical

value

be,

one

can

find

oscillating

solutions

as

well

as

bifurcating

solutions.

The

above

equations

may

serve

as

a

model

for

a

number

of

353