Labelle P. Supersymmetry DeMYSTiFied

Подождите немного. Документ загружается.

366

Supersymmetry Demystified

Now, let us turn our attention to the quark superﬁelds. The superﬁeld containing

the left-chiral anti-up quark will be denoted as

≡

+ θ ·χ

θ ·θ F

with quantum numbers

3, 1, −4/3 (again, we are suppressing the color indices).

The superﬁeld containing the left-chiral anti-down quark is

≡

+ θ ·χ

θ ·θ F

with quantum numbers

3, 1, 2/3.

We also need a superﬁeld containing the SU(2)

left-chiral quark, which we

write as

≡





+ θ ·





θ ·θ





(15.23)

with quantum numbers 3, 2, 1/3. For the second-generation quarks, the strange and

charm, we of course use D

, U

, and L

, and so on.

Note that the superﬁelds E

, U

, and D

[which are all SU(2)

singlets] contain

the antiparticle left-chiral spinors, whereas the SU(2)

doublets L

and Q

contain

the particle left-chiral states.

Consider now the Higgs sector of the MSSM. Here, there is a twist. Recall that in

the standard model we needed the conjugate Higgs ﬁeld to generate a mass for the up

quark. On the other hand, we have seen in previous chapters that SUSY-invariant

interactions between left-chiral superﬁelds are obtained from the F term of the

superpotential, which must be holomorphic in the chiral superﬁelds! Therefore, we

will not be able to use H

†

to construct SUSY-invariant Yukawa-type interactions,

and some of the standard model fermions will remain massless even after SSB.

This is very bad, so we need to ﬁgure out a way to take care of this.

The solution is obviously to introduce a second Higgs superﬁeld, with quantum

numbers opposite to those of the ﬁrst. This means that the MSSM contains twice as

many Higgs ﬁelds as in the standard model. In the standard model, there is a single

SU(2) Higgs doublet of complex ﬁelds corresponding to four degrees of freedom.

Three of those are the Goldstone modes that get “eaten” by gauge bosons to make

the W

and Z

massive, leaving a single Higgs particle. In the MSSM, there are

eight Higgs degrees of freedom, three of which also will get eaten, leaving this time

CHAPTER 15 Introduction to the MSSM

367

ﬁve observable Higgs particles. We will study the properties of these particles in

Chapter 16.

We therefore introduce two Higgs superﬁelds. The ﬁrst one will be taken as

having the same quantum numbers as the standard model Higgs ﬁeld, namely,

1, 2, 1. We will call it H

and write its components as





+ θ ·



˜χ



θ ·θ





(15.24)

As in the standard model, the subscripts indicate the electric charge of the particle,

which can be obtained using, as usual, Q = T

+ Y/2 (with the states in the upper

position having T

= 1/2 and the ones below having T

=−1/2). The reason for

the subscript u is that this Higgs generates a mass for the up quark.

Since the second Higgs doublet superﬁeld will have to play the role that H

†

played in the standard model, we must assign to it the opposite hypercharge to H

We obviously take it to be a color singlet. We therefore assign the quantum numbers

1, 2, −1 to this second Higgs doublet and write it as



−



+ θ ·



˜χ

−



θ ·θ



−



(15.25)

The spin 1/2 superpartners of the Higgs are referred to as Higgsinos. The quantum

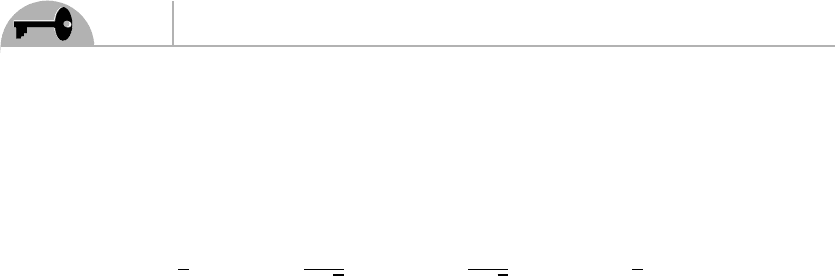
numbers of the MSSM left-chiral superﬁelds are summarized in Table 15.1.

Table 15.1 Quantum Numbers of the MSSM Left-Chiral Superﬁelds

Superﬁeld SU (3)

SU (2)

U (1)

321/3

121

12−1

31−4/3

312/3

12−1

112

368

Supersymmetry Demystified

15.5 The Gauge Vector Superfields

We will denote the hypercharge gauge superﬁeld by B. In the Wess-Zumino gauge,

it can be read off [see Eq. (13.10)] as

B ≡

θσ

θ B

√

θ ·θ

θ ·

√

θ ·

θθ · λ

−

θ ·θ

θ ·

θ D

(15.26)

For color, we introduce an octet of gauge ﬁelds G

, as in the standard model,

which means that we must introduce an octet of left-chiral fermions λ

as well as

an octet of auxiliary ﬁelds D

.WeuseG

to represent the corresponding superﬁeld,

which has the same expansion as Eq. (15.26) with λ

replaced by λ

and D

replaced

by D

(where the label c stands for color).

It is natural to use W

to represent the SU(2)

gauge superﬁeld, which should

not be confused with the superpotential W (with no index). The component ﬁelds

are W

, λ

, and D

To be honest, we will not do much with any of the component ﬁelds just given

and have deﬁned them for the sake of completeness only. So don’t sweat it if all

the notation is confusing; we will be concerned mainly with the superﬁelds.

15.6 The MSSM Lagrangian

We can organize the contributions to the MSSM lagrangian into ﬁve categories:

•

The kinetic energy terms of the leptons and quarks

•

The kinetic energy terms of the Higgs ﬁelds

•

The kinetic energy terms of the gauge bosons

•

The superpotential

•

The SUSY-breaking terms

Let us introduce each type of term in turn.

Kinetic Energy Terms of the Leptons, Quarks, and Higgs

Fields

It is straightforward to write down these terms after our work in Chapter 13. For

example, consider the kinetic energy of the ﬁrst-generation quark doublet Q

.Itis

CHAPTER 15 Introduction to the MSSM

369

given by 

†

exp(∂qV), which here takes the form

kin

= Q

†

exp





B + g

+ g W





(15.27)

where we have used Y = 1/3.

We will write the lagrangian explicitly in terms of the component ﬁelds, just

to illustrate what kind of interactions are generated. Before doing so, it proves

convenient to assign names to the SU(2)

doublets containing the component ﬁelds

in Eq. (15.23). To keep things as simple as possible, let’s just use

≡

φ +θ · χ +

θ ·θ F (15.28)

where

φ ≡

⎛

⎝

⎞

⎠

and so on.

In terms of these doublets, Eq. (15.27) gives the following lagrangian:

kin

†

φ +i χ

†

¯σ

χ + F

†

−

√



†

· χ −

√



· ¯χ −



†

−

√

†

χ ·λ

−

√

φτ

¯χ ·

−

†

φ D

−

√

†

χ ·λ

−

√

φλ

¯χ ·

−

†

φ D

(15.29)

where 

stands for the Gell-Mann matrices and D

is the gauge-covariant deriva-

tive corresponding to the quantum numbers of Q

, i.e., 3, 2, 1/3.

It is now that all our work on superspace truly pays off. We know that this

complicated lagrangian is supersymmetric by construction. Imagine how difﬁcult

it would be to construct this using the “brute force” approach we followed in

Chapters 5, 8, and 10!

370

Supersymmetry Demystified

Consider now the superﬁeld U

with quantum numbers

3, 1, −4/3. The kinetic

term of this superﬁeld is

kin

= U

†

exp



−



B + g

(λ

)

∗





= D

†

+i χ

†

¯σ

+ F

†

√



†

· χ +

√



· ¯χ +



†

−

√

†





∗

χ ·λ

−

√





∗

¯χ ·

−

†





∗

φ D

∗

(15.30)

The only interesting thing to mention here is that these terms contain the standard

model kinetic terms written in terms of the left-chiral particle and antiparticle Weyl

spinors. For example, if we focus on the SU(3)

kinetic terms for the spinors in

Eqs. (15.29) and (15.28), we have

iχ

†

¯σ



∂



+i χ

†

¯σ



∂

−ig

(λ

)

∗



+i χ

†

¯σ



∂



(15.31)

We showed in Section 10.4 that the ﬁrst two terms are equivalent to the quantum

chromodynamics (QCD) part of kinetic term for the up quark written in terms of a

Dirac spinor, i.e.,

γ



∂



 (15.32)

As for the down quark, the kinetic energy for the superﬁeld D will provide

i χ

†

¯σ



∂

−ig

(λ

)

∗



(15.33)

which, when combined with the last term of Eq. (15.31), corresponds to the QCD

part of the standard model kinetic term for the down quark. It’s clear that the

standard model kinetic terms are all contained in our supersymmetric expressions.

We won’t write down the kinetic terms for the leptons and the Higgs superﬁelds

because they are obvious to obtain after our examples above (note that Q is the

most complex case because it is not a singlet under any of the three gauge groups).

CHAPTER 15 Introduction to the MSSM

371

The Kinetic Energy Terms for the Gauge Bosons

These are simply (see Sections 13.5 and 13.7)

· F



Tr(F

· F

)



Tr(F

· F

)



(15.34)

where F

, F

, and F

are the ﬁeld-strength superﬁelds of the U (1)

, SU(2)

, and

SU(3)

gauge ﬁelds, respectively. Here, the dot product denotes the usual spinor

dot product (recall that the ﬁeld-strength superﬁelds are Weyl spinors).

The corresponding expansions in terms of component ﬁelds can be found in Eqs.

(10.8) and (10.29).

15.7 The Superpotential of the MSSM

Now we are ready to look for the superpotential of the MSSM, which is a function

of these superﬁelds of the theory, i.e., the lepton, quark, and Higgs left-chiral

superﬁelds. This will contain the Yukawa couplings of the fermion with the Higgs

ﬁeld, as a subset.

What we need is to build something that is an SU(3)

× SU(2)

×U (1)

in-

variant out of the scalar left-chiral superﬁelds. This will automatically be Lorentz-

invariant because these superﬁelds are invariant under Lorentz transformations.

And we will get a SUSY-invariant lagrangian by projecting out the F term, as

usual. So the only thing we have to worry about is to make sure that we respect

gauge invariance.

Again, note how the superﬁeld approach makes it easy to construct supersym-

metric theories! Imagine the difﬁculty we would be facing if we were working with

the component ﬁelds and trying to ensure invariance under SUSY following the

brute-force approach of Chapters 5, 8, and 10! The calculations were already long

when we were dealing with only one left-chiral and one gauge supermultiplet, so

you can imagine the nightmare if we were trying this approach with a theory as

complex as the MSSM.

Let us consider a single family for now. It will be trivial to generalize the super-

potential to three families later on.

Let’s start with the superﬁeld Q

. It transforms as 3 under the color group, so we

must pair it up with something that transforms as

3 in order to get a color singlet,

i.e., a color invariant. We have two possibilities: U

and D

Consider ﬁrst the combination U

. This has a hypercharge of 1/3 −4/3 =−1

and is a color singlet and a doublet under SU(2)

. We must pair it up with a ﬁeld

that has a hypercharge of 1 and is a weak doublet and a color singlet. Therefore,

372

Supersymmetry Demystified

we are looking for a ﬁeld with quantum numbers 1, 2, 1. The ﬁeld H

ﬁts the bill.

A technical detail we need to know is that the SU(2)-invariant way to couple two

SU(2) doublets is to sandwich between them the matrix iτ

. We have thus found

our ﬁrst candidate for the superpotential:

) ◦ H

which, of course, will have to be multiplied by an arbitrary constant. We are sup-

pressing the color indices but will put in parentheses the superﬁelds which have

color indices that are contracted.

If we now consider D

, with quantum numbers 1, 2, 1, we need to couple this

with a ﬁeld with quantum numbers 1, 2, −1. This time we have two possibilities:

and L

, so we can construct the two gauge invariants

) ◦ H

) ◦ L

However, we will see a bit below that the second term will have to be rejected

for phenomenological reasons, and this will force us to impose a new type of

symmetry. But let’s keep going with building gauge invariants. We have exhausted

all the possible terms containing Q

Consider now terms containing H

but with no Q

(to avoid listing again some

of the terms already found). In other words, we consider only pairing H

with itself

or with other ﬁelds appearing below it in the table.

Consider pairing H

with itself. H

◦ H

has quantum numbers 1, 1, 2. We have

one possibility: We can pair it with L

◦ L

◦ H

◦ L

but the F term of this expression is of dimension 5 (recall that extracting the F

term increases the dimension by 1), so we do not consider it (and is actually zero!

See below).

We also can construct invariants containing only H

and a single other ﬁeld. To

get an invariant, we need to pair up H

with a ﬁeld with quantum numbers 1, 2, −1.

We have two candidates! So it is possible to build two invariants containing only

two ﬁelds:

◦ H

◦ L

There are no other invariants that we can build out of H

(and, again, that do not

contain Q

CHAPTER 15 Introduction to the MSSM

373

Let us turn to H

. We can pair it up with itself, in which case we need to multiply

by the E

superﬁeld:

◦ H

but this vanishes identically because for any two-component vector A,wehave

A ◦ A = A

iτ

A = 0.

can be paired up with L

to form an SU(2)-invariant. But then, to get the

hypercharge equal to zero, we need to pair them up with E

,soweget

◦ H

We cannot build any new invariants containing only two of the antiquark super-

ﬁelds U

and D

because there is no way to make this invariant under SU(3)

(one

can indeed show using Young tableaux that

3 ⊗

3 = 3 ⊕

6). However, it is possible

to build a color singlet out of three

3 representations. Explicitly, the color singlet

is given by

abc

◦ D

where we showed explicitly the color indices and f

abc

are the structure constants

of QCD.

Consider now L

. Our last possible invariant is

◦ L

) E

Thus we have uncovered eight gauge-invariant terms built out of the chiral super-

ﬁelds. But we have a problem. Some of these terms violate either baryon or lepton

number conservation, and this has nasty implications in terms of phenomenology.

Lepton and baryon number–violating interactions might lead to rapid decay of the

proton (which is not observed) or to large ﬂavor-changing neutral current (such as

unobserved muon decay to an electron and a photon μ → eγ ). The undesirable

interactions contain unequal numbers of lepton and antilepton ﬁelds or unequal

numbers of quark and antiquark ﬁelds:

) ◦ L

◦ L

abc

◦ D

(15.35)

The ﬁrst three violate lepton number conservation, whereas the last one violates

baryon number conservation. The “good” interactions are

) ◦ H

◦ H

(15.36)

374

Supersymmetry Demystified

Why don’t we have such a problem in the standard model? Well, the standard

model is actually quite special in that respect. The particle content is such that there

are no gauge-invariant interactions that violate lepton or baryon number conser-

vation. Therefore, gauge invariance is what saves the day for the standard model

(although nonperurbative effects are known to violate both symmetries, but only

by extremely small amounts at the energies we can reach in particle accelerators).

Unfortunately, the situation is not as rosy for the MSSM as we just saw. Why

not simply discard the unpleasant terms, then? The problem is that in quantum

ﬁeld theory, if we set to zero some interaction even though it does not violate any

symmetry of the theory, loop corrections will necessarily reintroduce the offending

term in the lagrangian. One always could ﬁne-tune the bare coupling constants to

set the problematic interactions to zero order by order in the loop expansion, but this

just reintroduces a naturalness problem (actually, several naturalness problems!),

which is what we wanted to avoid in the ﬁrst place!

So what is the way out? We have used gauge invariance and SUSY to restrict

the types of interactions, but that’s not enough. Clearly, what we need is an extra

symmetry, one that will kill all the undesirable terms while keeping the good ones!

This symmetry preferably should be a global one so as to not require extra gauge

bosons and all the complications that would follow. It turns out that we can indeed

deﬁne a global U (1) symmetry that does exactly what we need! Admittedly, it may

sound a bit unsatisfactory to invent an extra symmetry to get rid of things we don’t

like, but the fact that it is possible at all is admirable in itself and suggests that there

must be some underlying but not yet understood principle at work. This should not

be a disappointment; after all, nobody expects a supersymmetric standard model to

be a fundamental theory.

To understand this new symmetry, let’s have a closer look at what the difference

is between the good and the bad terms. A generic left-chiral superﬁeld is given by

 = φ + θ · χ +

θ ·θ F (15.37)

The F term of a product of three left-chiral superﬁelds XYZ can be written

schematically as

XYZ φ

+ φ

· χ

+ permutations (15.38)

After eliminating the auxiliary ﬁelds, the terms proportional to F will become part

of the scalar potential. Let’s focus on the spinor terms.

The key observation is the following: The standard model fermions correspond to

the spinor component ﬁelds of the lepton and quark superﬁelds. The Higgs scalars,

correspond to the scalar ﬁeld components of the Higgs superﬁelds (by scalar ﬁeld

CHAPTER 15 Introduction to the MSSM

375

components, we obviously mean the scalar components that are not multiplied by

any Grassmann variables, not the auxiliary ﬁelds). We will refer to these particles

as standard particles because they are present in the standard model, with the only

exception that there are more Higgs scalars.

On the other hand, the superpartners of these standard particles are the scalar

components of the lepton and quark superﬁelds and the spinor component of the

Higgs superﬁelds. If we use a label ST for the standard particles and SP for their

superpartners, then the F terms of the good interactions (15.36) containing two

spinors are of the form

· χ

(15.39)

whereas the bad interactions are of the form

· χ

or χ

· χ

(15.40)

Do you see the pattern? All the unwanted terms contain a single superpartner,

whereas the good terms contain either no superpartners or two of them. We can sum-

marize this simply by saying that terms with even numbers of superpartners are al-

lowed, whereas we want to eliminate the terms with an odd number of superpartners.

Clearly, we need a symmetry that acts differently on the component ﬁelds of the

superﬁelds in order to distinguish the standard particles from their superpartners.

Since the different component ﬁelds multiply different powers of the Grassmann

variable θ, it is natural to introduce a U(1) symmetry acting the following way:

θ → θ



= e

iϕ

θ →



= e

−iϕ

θ (15.41)

where ϕ is a U (1) global phase. This transformation is often written as

⎛

⎝

⎞

⎠

→ e

iγ

⎛

⎝

⎞

⎠

(15.42)

when the Grassmann variables θ and

θ are assembled into a four-component spinor.

This symmetry is known as R-symmetry.

We now impose that left-chiral superﬁelds transform with an overall phase under

R-symmetry. If a superﬁeld  transforms with a phase  → exp(ikϕ), where k

is a real constant, then Eqs. (15.37) and (15.41) show that the component ﬁelds

transform as

φ → e

ikϕ

φχ→ e

i(k−1)ϕ

χ F → e

i(k−2)ϕ

F (15.43)