Labelle P. Supersymmetry DeMYSTiFied

Подождите немного. Документ загружается.

246

Supersymmetry Demystified

only by a sign. Note that for this to work, it is crucial that U contains both Q · θ

and

Q ·

θ! If we had only one of the two terms, U would no longer be unitary.

Now that we have an explicit expression for U in terms of the charges, we can

rewrite Eq. (11.8) as

exp(ia· P + i

ζ ·

Q +i ζ · Q) exp(ix· P + i

θ ·

Q +i θ · Q)

= exp(ix



· P +i



Q +i θ



· Q) (11.12)

We will now spend some time to calculate the left-hand side of Eq. (11.12).

Using the Baker-Campbell-Hausdorff formula, it becomes

exp



i (x + a) · P + i (

θ +

ζ)·

Q +i (θ +ζ)· Q

−



ζ ·

Q +ζ · Q,

θ ·

Q +θ · Q



+···



(11.13)

where we have used the fact that

commutes with all charges to simplify the

commutator term. This looks like a nightmare to calculate (well, an inﬁnite number

of nightmares), but luckily, we will see that the expansion terminates pretty quickly.

The commutators we need actually have been calculated in Chapter 6 using all

components with lower, undotted indices, which forced us to carry around a bunch

of σ

matrices. We can calculate the commutators much more efﬁciently now using

the notation of Chapter 3. Consider ﬁrst the commutator [ζ · Q,θ · Q] (recall that

the order in spinor dot products does not matter). Expanding, we get



ζ · Q,θ· Q





,θ



= ζ

− θ

=−ζ

, Q

}

= 0 (11.14)

where we have used that the supercharges anticommute, [Eq. (6.70)].

Similarly,



Q ·

ζ,

Q ·





˙a



=−

˙a

{

˙a

}

= 0 (11.15)

CHAPTER 11 Superspace Formalism

247

Next, we turn our attention to



ζ · Q,

Q ·







(11.16)

Let’s pause to make a comment before we proceed with the calculation of this

expression. It is frequent to see in SUSY expressions the same letter appearing

both dotted and undotted. For example, we could have written Eq. (11.16) as



ζ · Q,

Q ·





˙a



(11.17)

In such expressions, it is implicit that the index ˙a is totally unrelated to the index

a, so Eq. (11.17) is completely equivalent to Eq. (11.16). In this book we will

avoid using a letter and the dot of the same letter in any given expression only to

make equations easier to read. It is indeed easier to tell apart b and ˙c than b and

especially given that these appear as small indices.

Proceeding with the calculation, we ﬁnd that





= ζ

}

= ζ

(σ

)

using Eq. (6.72)

= ζσ

θ P

(11.18)

From this, we get [

Q ·

ζ,θ · Q] for free (or almost). We simply have to write



Q ·

ζ, θ · Q



=−



θ · Q,

Q ·



and use Eq. (11.18) with ζ and θ switched to get



Q ·

ζ, θ · Q



=−θσ

ζ P

(11.19)

The key results are the commutators (11.14), (11.15), (11.18), and (11.19). Now

we can use them to evaluate Eq. (11.13). Note that since the commutators of Q

and Q

†

yield the momentum operator P

, which commutes with everything, all

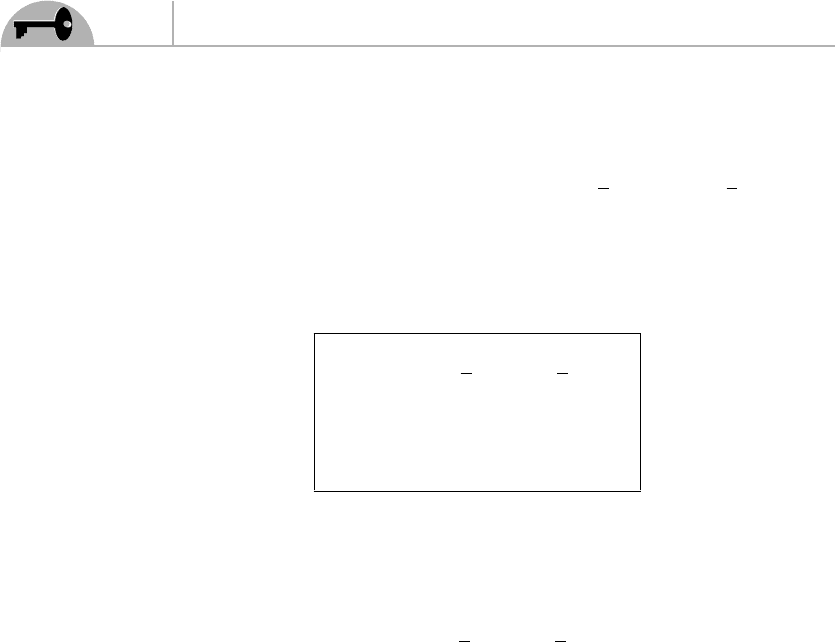
the higher-order commutators represented by the dots in Eq. (11.13) vanish! So

Eq. (11.13) is simply

exp



i (x + a) · P + i (

θ +

ζ)·

Q +i (θ +ζ)· Q −

ζσ

θ P

θσ

ζ P



(11.20)

248

Supersymmetry Demystified

Recall that this is the left-hand side of Eq. (11.12). Setting this equal to the

right-hand side of Eq. (11.12), we obtain

exp



i (x + a) · P + i (

θ +

ζ)·

Q +i (θ +ζ)· Q −

ζσ

θ P

θσ

ζ P



= exp



i θ



· Q + i



Q +ix



· P



from which we can ﬁnally read off the transformations of the superspace coordi-

nates:



= x + a +

ζσ

θ −

θσ



= θ +ζ

θ =

θ +

(11.21)

As expected, the transformation of x acquires extra terms that mix ordinary space

with the fermionic coordinates of superspace.

Using Eq. (A.50), the transformation of x also could be written as



= x + a −

θ ¯σ

ζ +

ζ ¯σ

θ (11.22)

Now we are ready to write the SUSY charges as differential operators acting in

superspace.

11.4 Introduction to Superfields

Before going any further, it is important to take a short pause to review some

important facts about the calculus of Grassmann variables. Although this may

seem a bit intimidating at ﬁrst, it turns out that Grassmann calculus is much easier

than calculus involving real or complex variables. As one might guess, this comes

from the fact that the square of any Grassmann quantity is zero.

Before discussing superﬁelds, let’s start with something simpler. Consider an

arbitrary (but commuting) function F of a single Grassmann variable, say, θ

. Let

us now look at the Taylor expansion of this function around θ

= 0. Usual Taylor

expansions (with respect to real or complex variables) contain an inﬁnite number

of terms, but here, since θ

= 0, the expansion terminates after only two terms!

F(θ

) = c

+ c

(11.23)

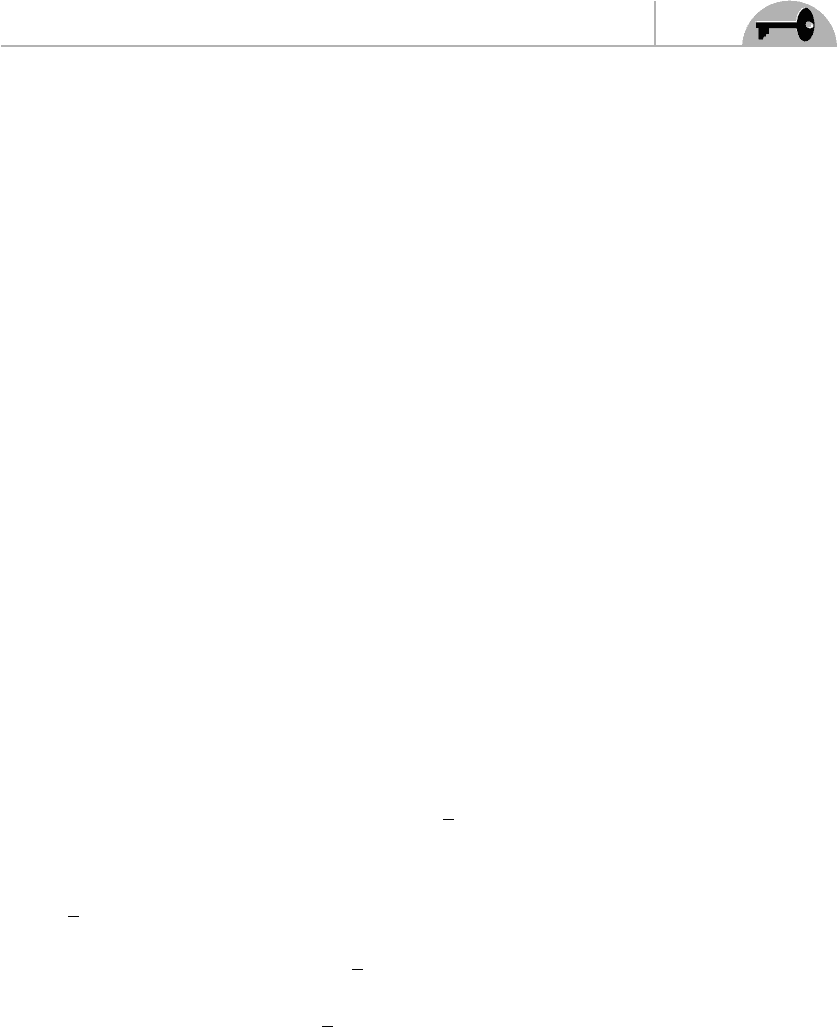
CHAPTER 11 Superspace Formalism

249

where we really mean an equality, not an approximation, and the coefﬁcients c

and

are some complex numbers. If the function depends on two Grassmann variables

and θ

, then the Taylor expansion clearly terminates after four terms:

F(θ

,θ

) = c

+ c

There is no need to write a term proportional to θ

because this term could be

combined with the term in θ

after using θ

=−θ

Now we add a twist: We make F a function of both Grassmann variables and

spacetime and call it a superﬁeld F (in this book, we will use calligraphic let-

ters to represent most superﬁelds). Then the Taylor expansion with respect to the

Grassmann variables obviously is given by

F(x,θ

,θ

) = A(x) + B(x)θ

+C(x)θ

+ D(x)θ

(11.24)

Note that in this expression, B(x) and C(x) are Grassmann functions, whereas

A(x) and D(x) are ordinary (i.e., commuting) functions. Of course, our interest is

in quantum ﬁeld theory, so we promote the functions A(x) ···D(x) to quantum

ﬁelds.

F is not the most general superﬁeld we can construct because we have chosen it

to be independent of

θ. Exercise 11.2 invites you to work out the expansion of the

most general superﬁeld, which is a function of x,θ, and

θ.

We will be mostly concerned with superﬁelds that are invariant under Lorentz

transformations, but even in that case, it is not straigthforward to infer how the

four ﬁelds appearing in Eq. (11.24) transform. It is preferable instead to write the

expansion in terms of the Weyl spinor θ, i.e.,

F(x,θ) = φ(x) + θ · χ(x) +

θ ·θ F (x) (11.25)

where the factor of 1/2 in the last term is purely conventional and chosen to make

this resemble usual Taylor expansions. As an aside, note that many authors rescale

θ →

√

2θ so that they write the expansion as

F(x,θ) = φ(x) +

√

2 θ ·χ(x) + θ · θ F(x)

This introduces various powers of

√

2 with respect to our convention.

If the ﬁeld F is a scalar under Lorentz transformations, we can tell right away

that φ(x) is a scalar ﬁeld and that χ(x) is a left-chiral spinor ﬁeld, because θ is

itself a left-chiral Weyl spinor. We also can tell that F(x) is a scalar ﬁeld because

the quantity θ · θ is invariant under Lorentz transformations. Note that the number

250

Supersymmetry Demystified

of independent ﬁelds in Eqs. (11.24) and (11.25) is the same because χ(x) is a

two-component spinor.

The ﬁelds φ(x), χ(x), and F(x) are often referred to as the component ﬁelds of

the superﬁeld F.

EXERCISE 11.1

Find the relations between the ﬁelds appearing in Eq. (11.24), and the ﬁelds

φ(x), χ

(x), χ

(x), and F(x) appearing in Eq. (11.25).

EXERCISE 11.2

Consider a general scalar superﬁeld S(x,θ,

θ). Write down the most general Taylor

expansion of this superﬁeld [not in terms of components as in Eq. (11.24) but in

the index-free notation, as in Eq. (11.25)]. Hint: You should ﬁnd nine terms. Don’t

forget that you may use σ

(but then you will not need ¯σ

; do you see why?).

11.5 Aside on Grassmann Calculus

Besides Taylor expansions, we also will need to differentiate and integrate with

respect to Grassmann variables. Consider ﬁrst taking derivatives. Of course, we

deﬁne

∂θ

= 1

Note that we treat θ

,θ

, and

as four independent variables, so the derivative

of any of those four quantities with respect to any of the other three gives zero. For

example,

∂θ

∂

∂θ

∂

∂θ

= 0

On the other hand, the variables with upper indices must be viewed as functions

of the variables with lower indices, not as independent variables. Indeed, recall that

as we saw in Chapter 3 [see Eqs. (3.13) and (3.16)],

= θ

=−θ

=−

(11.26)

CHAPTER 11 Superspace Formalism

251

This means that, for example,

∂θ

= 0

whereas

∂θ

=−1

Note that the relations (11.26) allow us to also rewrite derivatives with respect to

variables with upper indices in terms of derivatives with respect to variables with

lower indices, and vice versa. For example,

∂

∂θ

∂

∂θ

and

∂

∂θ

=−

∂

∂θ

Consider now taking the derivative of something less trivial, let’s say

∂

∂θ

(θ

)

We may apply the product rule on the condition that we keep in mind that the

derivative is a Grassmann quantity so that we pick up a minus sign when passing it

through θ

∂

∂θ

(θ

) =



∂θ



− θ



∂θ



Since θ

is independent of θ

, we simply get

−θ



∂θ



=−θ

252

Supersymmetry Demystified

Of course, we could have simply moved θ

in front of the derivative because it is a

constant as far as the derivative with respect to θ

is concerned, to get

∂

∂θ

=−θ

∂

∂θ

=−θ

Particularly useful will be the identities mentioned in the following exercise.

EXERCISE 11.3

Prove

∂

∂θ

θ ·θ = 2θ

and

∂

∂θ

θ ·θ =−2θ

These formulas will prove to be very useful in the next section.

Now let’s turn our attention to integration of Grassmann variables. Here, inte-

gration is not related to the limit of a sum and has some properties quite different

from conventional Riemann integrals. It is better thought of as an abstract algebraic

operation deﬁned in terms of its properties. As such, a different symbol than an

integral sign probably would be more appropriate. The integration over Grassmann

variables originally was deﬁned by Berezin

and is therefore sometimes referred to

as Berezin integration.

So how do we deﬁne this “integration” operation? First, we impose that it be a

linear operation:



dθ



F(θ

) + k

G(θ

)



= k



dθ

F(θ

) + k



dθ

G(θ

) (11.27)

where k

and k

are arbitrary complex numbers.

Now comes the key deﬁnition. We know that an arbitrary function of θ

has the

expansion given in Eq. (11.23). We deﬁne the integration of F(θ

) to give for result

the coefﬁcient of the term linear in θ



dθ

F(θ

) ≡ c

CHAPTER 11 Superspace Formalism

253

Using Eqs. (11.23) and (11.27), this means that



dθ

+ c



dθ

= c

which implies



dθ

= 0



dθ

= 1

Note that “integrating” F(θ

) with respect to θ

gives exactly the same result as

differentiating it with respect to θ

! This is not what we are used to in conventional

calculus (except for the function e

!).

Now consider integration with respect to two variables, θ

and θ

. We deﬁne the

“measures” to be Grassmann quantities, like the θ

, so we impose

{dθ

, dθ

}={dθ

,θ

}={θ

,θ

}=0

This means that



dθ

=−θ



dθ

for example.

As an example, consider



dθ



dθ

=−



dθ





dθ



=−



dθ

= 0

Clearly, we also have



dθ



dθ

= 0

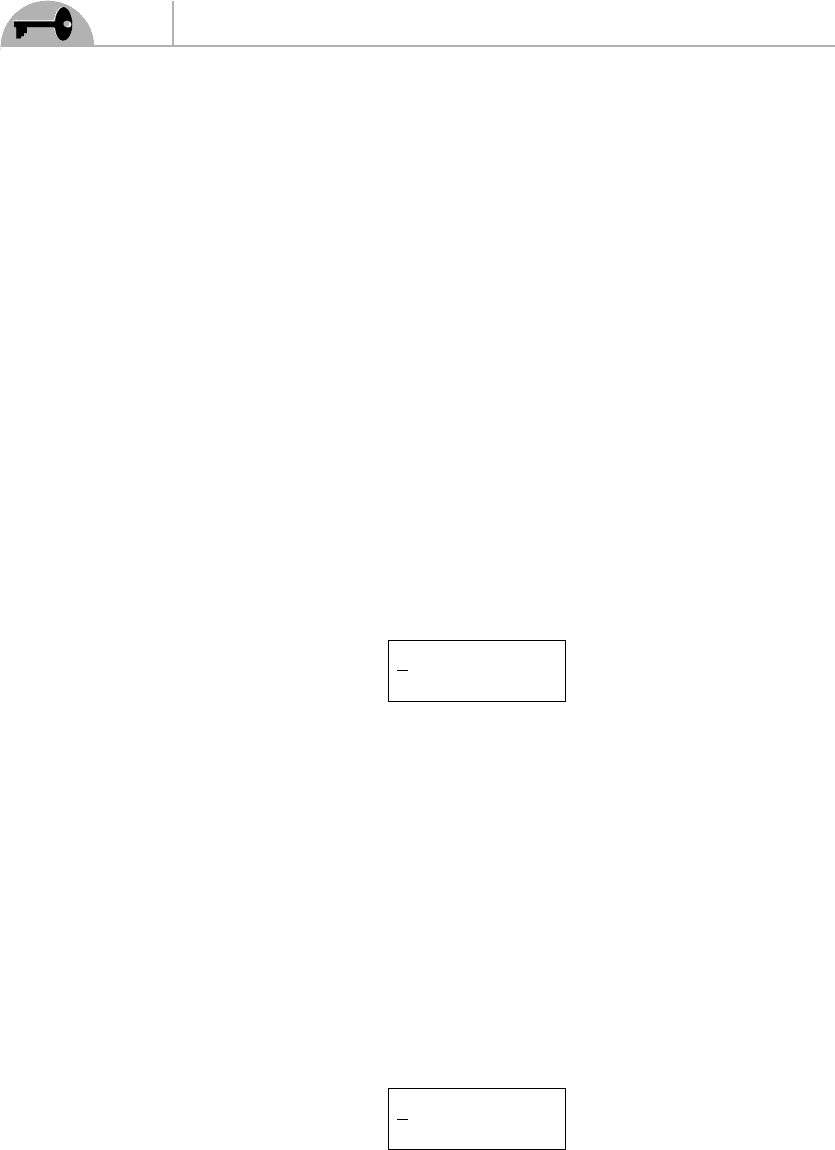
More interesting is



dθ



dθ

=−



dθ



dθ

=−1

254

Supersymmetry Demystified

Note that if we integrate the superﬁeld (11.24) over both of its Grassmann vari-

ables, we obtain



dθ

F(x,θ

,θ

) =−D(x)

so the integration projects out the component proportional to the two Grassmann

variables. The fact that we can use an integration to project out terms like this will

play a key role in the rest of this book.

Let’s deﬁne the measure d

θ to represent

θ ≡ dθ

dθ

in that order. With this deﬁnition,



θθ· θ = 2



θθ

= 2

so that



θθ· θ = 1 (11.28)

a relation we will use very often.

We obviously also can integrate over the barred variables

˙a

. All the preceding

results hold as well for those variables. However, we will deﬁne

θ ≡−d

instead. This choice is made so that



θ ·

θ = 2



= 2

and therefore,



θ ·

θ = 1

CHAPTER 11 Superspace Formalism

255

Of course, we also have



θ d

θθ· θ

θ ·

θ = 1 (11.29)

which we will also need often. Note that when we integrate any other combination

of θ and

θ, we get zero. For example, if we integrate a general superﬁeld S(x,θ,

θ),

we get



θ d

θ S(x,θ,

θ) = coefﬁcient of the term

θ ·θ

θ ·

in S(x,θ,

θ)

Similarly, Eq. (11.28) implies



θ S(x,θ,

θ) = coefﬁcient of the term

θ ·θ

in S(x,θ,

θ)

11.6 The SUSY Charges as

Differential Operators

We will keep using S to denote a general superﬁeld. The expression analogous to

Eq. (11.5) in the case of a translation plus a supersymmetric transformation is

exp



−ia

−i ζ · Q −i

ζ ·



S(x,θ,

θ) = S(x +δx,θ + δθ,

θ +δ

θ)

(11.30)

Now we just need to plug in the transformed coordinates (11.21) into the superﬁeld

and Taylor expand. This gives

S(x +δx,θ + δθ,

θ +δ

θ)

≈ S(x,θ,

θ) + δx

∂

S(x,θ,

θ) + δθ

∂

S(x,θ,

θ) + δ

˙a

∂

˙a

S(x,θ,

θ)

= S +



ζσ

θ −

θσ



∂

S + ζ

∂

S +

˙a

∂

˙a

S (11.31)

where we have suppressed the (x,θ,

θ) dependence of S to ease the notation a

bit and where the partial derivatives with respect to the Grassmann variables are