John R. Fanchi - Principles of Applied Reservoir Simulation, Second Edition
Подождите немного. Документ загружается.

182
Principles
of
Applied
Reservoir Simulation
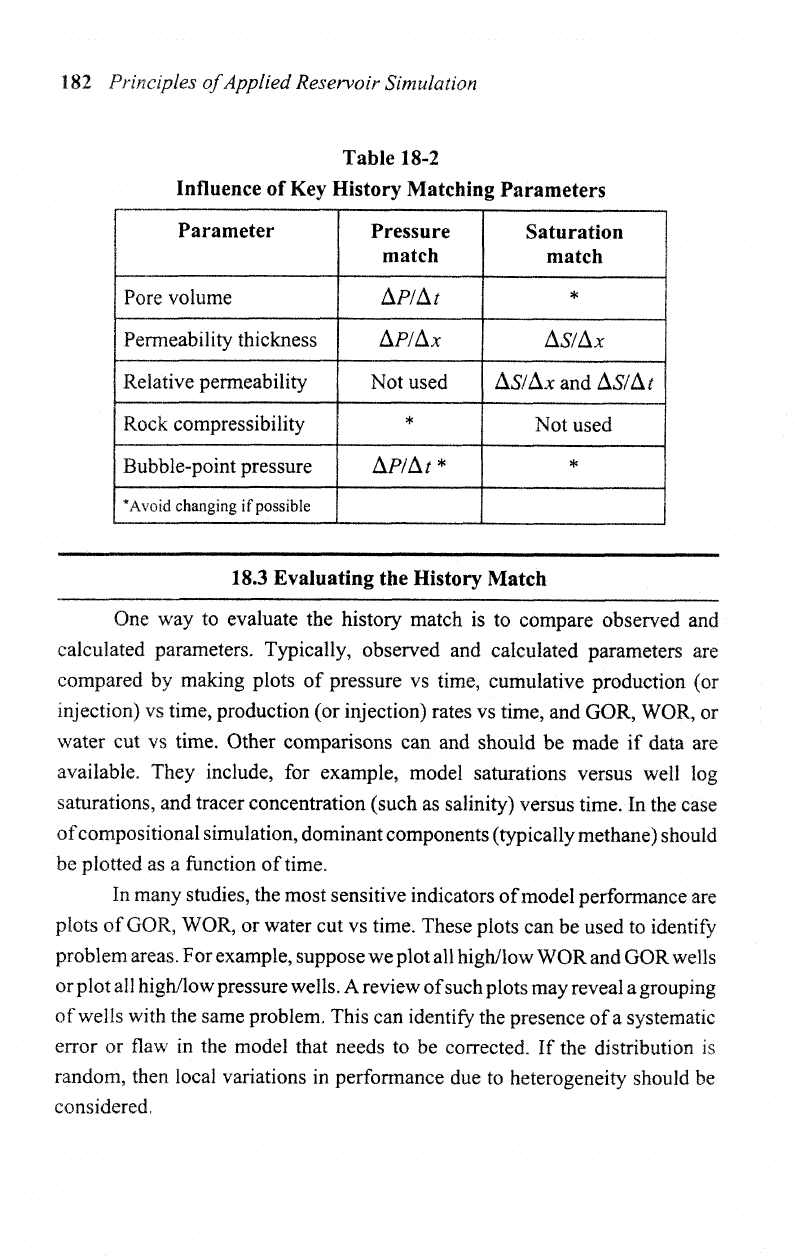
Table
18-2
Influence
of Key
History Matching Parameters
Parameter
Pore
volume
Permeability
thickness
Relative permeability
Rock
compressibility
Bubble-point pressure
*
Avoid
changing
if
possible
Pressure
match
AP/A/
AP/A*
Not
used
*
AP/A/
*
Saturation
match
*
AS/A*
AS/
A*
and
AS/A/
Not
used
*
18.3
Evaluating
the
History Match
One way to
evaluate
the
history match
is to
compare observed
and
calculated parameters. Typically, observed
and
calculated parameters
are
compared
by
making plots
of
pressure
vs
time, cumulative production
(or
injection)
vs
time, production
(or
injection) rates
vs
time,
and
GOR, WOR,
or
water
cut vs
time.
Other
comparisons
can and
should
be
made
if
data
are
available. They include,
for
example, model saturations versus well
log
saturations,
and
tracer concentration (such
as
salinity) versus time.
In the
case
of
compositional simulation, dominant components (typically methane) should
be
plotted
as a
function
of
time.
In
many studies,
the
most sensitive indicators
of
model performance
are
plots
of
GOR,
WOR,
or
water
cut vs
time. These plots
can be
used
to
identify
problem
areas.
For
example,
suppose
we
plot
all
high/low
WOR
and GOR
wells
or
plot
all
high/low
pressure wells.
A
review
of
such plots
may
reveal
a
grouping
of
wells with
the
same problem. This
can
identify
the
presence
of a
systematic
error
or
flaw
in the
model that needs
to be
corrected.
If the
distribution
is
random, then local variations
in
performance
due to
heterogeneity should
be
considered.
TEAM LinG - Live, Informative, Non-cost and Genuine!

Part
II:
Reservoir Simulation
183
18.4 Deciding
on a
Match
There
are
several ways
to
decide
if a
match
is
satisfactory.
In all
cases,
a
clear understanding
of the
study objectives should
be the
standard
for
making
the
decision.
If a
coarse study
is
being performed,
the
quality
of the
match
between
observed
and
calculated parameters does
not
need
to be as
accurate
as
it
would
need
to be for a
more
detailed
study.
For
example, pressure
may be
considered matched
if the
difference
between calculated
and
observed
pressures
is
within
±10%
draw
down.
The
tolerance
of
±10%
is
determined
by
estimating
the
uncertainty associated with measured
field
pressures
and the
required quality
of
the
study.
A
study demanding
greater
reliability
in
predictions
may
need
to
reduce
the
tolerance
to ± 5% or
even less,
but it is
unrealistic
to
seek
a
tolerance
of
less than
one
percent.
The
uncertainty applies
not to
individual well gauge
pressures, which
may be
measured
to a
precision
of
less than
one
percent,
but
to
estimates
of
average
field or
region pressure
from two or
more
well
tests.
The
latter error
is
generally much larger than
the
precision
of a
single
well
test.
In
any
event, model-calculated pressure trends should match
field or
region pres-
sure
performance.
Another sensitive indicator
of the
quality
of a
history match
is the
match
of
WOR, GOR,
or
water cut. Three factors need
to be
considered:
breakthrough
time,
the
magnitude
of the
difference
between observed
and
calculated values,
and
trends.
Adjustments
in the
model should
be
made
to
improve
the
quality
of
each
factor.
Saleri
[1993]
has
observed that
a
match
of the field is
more easily
obtained than
a
match
of
individual well performance.
Indeed,
he
notes that
matching every well
is
virtually impossible.
As a rale of
thumb,
the field
match
may
be
valid
for a
year
or
more without updating,
and we can
expect
the
well
match
to be
valid
for up to six
months without updating. Deviations
from
this
rule
will
vary widely,
and
will depend
on the
type
of
system
modeled
and the
alignment
of the
interpreted model with reality. Indeed,
gas
reservoirs without
aquifer
influx
may be
accurately
modeled
for the
life
of the field,
while
a gas
reservoir with complex lithology
and
water
influx
may
never
be
satisfactorily
matched.
TEAM LinG - Live, Informative, Non-cost and Genuine!

184
Principles
of
Applied
Reservoir Simulation
Modelers
must
resist being
drawn
into
the
"one more run"
syndrome.
This
occurs
when
a
modeler
(or
member
of the
study team)
wants
to see
"just
one
more
run"
to try an
idea
that
has not yet
been tried.
In
practice,
a final
match
is
often
declared when
the
time
or
money allotted
for the
study
is
depleted.
18.5 History Match Limitations
History
matching
may be
thought
of as an
inverse problem.
An
inverse
problem exists when
the
dependent variable
is the
best known aspect
of a
system
and
the
independent variable must
be
determined [Oreskes,
et
al,
1994].
For
example,
the
"dependent
variable"
in oil and gas
production
is the
production
performance
of the field.
Production performance depends
on
input
variables
such
as
permeability distribution
and fluid
properties.
The
goal
of the
history
match
is to find
a
set of
input variables that
can
reconstruct
field
performance.
In
the
context
of an
inverse problem,
the
problem
is
solved
by finding a
set of
reasonable reservoir parameters that minimizes
the
difference between
model performance
and
historical performance
of the field. As
usual,
we
must
remember that
we are
solving
a
non-unique problem whose solution
is
often
as
much
art as
science.
The
uniqueness
problem
arises
from
many factors. Most
notable
of
these
are
unreliable
or
limited
field
data
and
numerical
effects.
Advances
in
hardware
and
software technology have made
it
possible
to
minimize
the
effects
of
numerical problems,
or at
least estimate their
influence
on
the final
history match solution. Data limitations
are
more
difficult
to
resolve
because
the
system
is
inherently
underdetermined:
we do not
have enough data
to be
sure that
our final
solution
is
correct.
Test
of
Reasonableness
A
model
may be
considered reasonable
if it
does
not
violate
any
known
physical constraints.
In
many cases,
a
model
may be
acceptable
if it is
reason-
able.
In
other situations,
not
only must physical constraints
be
satisfied,
but
approved
processes
for
evaluating data must also
be
followed.
Thus
a
model
may
be
reasonable,
but if it is
based
on an
innovative technique
that
is
reasonable
but
not
approved,
the
model
will
be
unacceptable.
The
modeler
may use a
method
TEAM LinG - Live, Informative, Non-cost and Genuine!
Part
II:
Reservoir Simulation
185
that
is in the
literature,
but
the
commissioner
of the
study
may
have
a
philosophi-
cal
or
empirical
objection
to the
method. Window area modeling
is a
good
example
of a
method that
may be
reasonable
but not
acceptable because
failure
to
adequately
describe
flux
across window area boundaries
can
yield
poor
results.
If
someone
in a
position
of
authority
or
influence
has had a
bad
experience
with
the
modeling method, they
may
refuse
to
accept results
from
the
model.
Similarly,
the
modeler needs
to be
aware that some modeling methods
are not
universally
accepted.
At the
very least, alternative methods
may be
needed
to
corroborate
the
disputed method
as
part
of a
sensitivity
analysis
or
model
validation
exercise.
Exercises
Exercise
18.1
(A) Run
EXAM6.DAT
and
plot average reservoir pressure
as a
function
of
time.
(B)
Multiply
the
pore volume
of
data
set
EXAM6.DAT
by
0.9
and
repeat part
A. (C) How
does
the
change
in
pore volume
affect
pressure
as
a
function
of
time?
Exercise 18.2 Double
the
horizontal permeability
in
layer
K = 1 of
data
set
EXAM6.DAT.
(A)
Plot
the
average
reservoir
pressure
as a
function
of
time.
(B)
What
is the
effect
on
production,
by
layer,
at the end of two
years? File
WTEMP.WEL provides rate information
by
layer
for all
wells.
Exercise 18.3
Set the x
direction
transmissibility
to 0
between
1
= 2 and
1
= 3
for
blocks ranging
from
J = 1 to J = 4 in
layers
K = 1 and K
=
2 of
data
set
EXAM6.DAT.
This transmissibility barrier represents
a flow
barrier such
as a
sealing
fault.
How
does
the
barrier alter
flow
patterns
and the
distribution
of
reservoir pressure?
TEAM LinG - Live, Informative, Non-cost and Genuine!

Chapter
19
Predictions
The
previous
chapters have shown
how to
build
a
working model
of the
reservoir
and
establish
a
level
of
confidence
in the
validity
of
model results.
It
is
time
to
recall that modeling
was
undertaken
to
prepare
a
tool that would help
us
develop recommendations
for
a
reservoir
management program.
The
primary
reservoir management objective
is to
determine
the
optimum operating
conditions needed
to
maximize
the
economic recovery
of
hydrocarbons. This
is
accomplished,
in
principle,
by
marshaling accessible resources
to
4
optimize recovery
from a
reservoir,
and
+
minimize capital investments
and
operating expenses.
The
commercial impact
of the
simulation study
is the
preparation
of a
cash
flow
prediction
from
projected
field
performance. Thus,
the
model study
is
often
completed
by
making
field
performance
predictions
for
use in
economic analysis
of
possible operating strategies.
19.1
Prediction
Capabilities
Performance
predictions
are
valuable
for
a
variety
of
purposes.
Predictions
can be
used
to
better interpret
and
understand reservoir behavior
and
they
provide
a
means
of
determining
model sensitivity
to
changes
in
input data. This
sensitivity analysis
can
guide
the
acquisition
of
additional data
for
improving
reservoir
management.
Predictions
enable people
to
estimate project
life
by
predicting recovery
vs
time. Project
life
depends
not
only
on the flow
behavior
of the
reservoir,
but
186
TEAM LinG - Live, Informative, Non-cost and Genuine!
Part
II:
Reservoir Simulation
187
also
on
commercial issues. Models
let the
user impose
a
variety
of
economic
constraints
on
future
reservoir performance during
the
process
of
estimating
project
life.
These constraints
reflect
a
range
of
economic criteria
that
will
interest
management, shareholders,
and
prospective investors.
Commercial
interests
are
clearly important
to the
future
of a
project,
and
so are
technical issues.
It is
often
necessary
to
compare
different
recovery
processes
as
part
of a
study. Since there
is
only
one field, it is
unrealistic
to
believe
that
many
different
recovery processes
can be
evaluated
in the field,
even
as
small-scale pilot projects. Pilot projects tend
to be
substantially more
expensive
to run
than simulation studies.
In
some
cases,
however,
it
might
be
worthwhile
to
confirm
a
simulation study with
a
pilot project. This
is
especially
true
with expensive
processes
such
as
chemical
and
thermal
flooding,
Yet
another
use for
model predictions
is the
preparation
of a
reservoir
management plan. Reservoir management plans
have
been discussed
in
previous
sections. Their preparation
is
often
the
single most important motivation
for
performing
a
simulation
study.
19.2 Prediction Process
The
prediction process begins with model calibration.
It is
usually
necessary
to
ensure continuity
in
well rate when
the
modeler switches
from
rate
control during
the
history match
to
pressure
control during
the
prediction stage
of a
study.
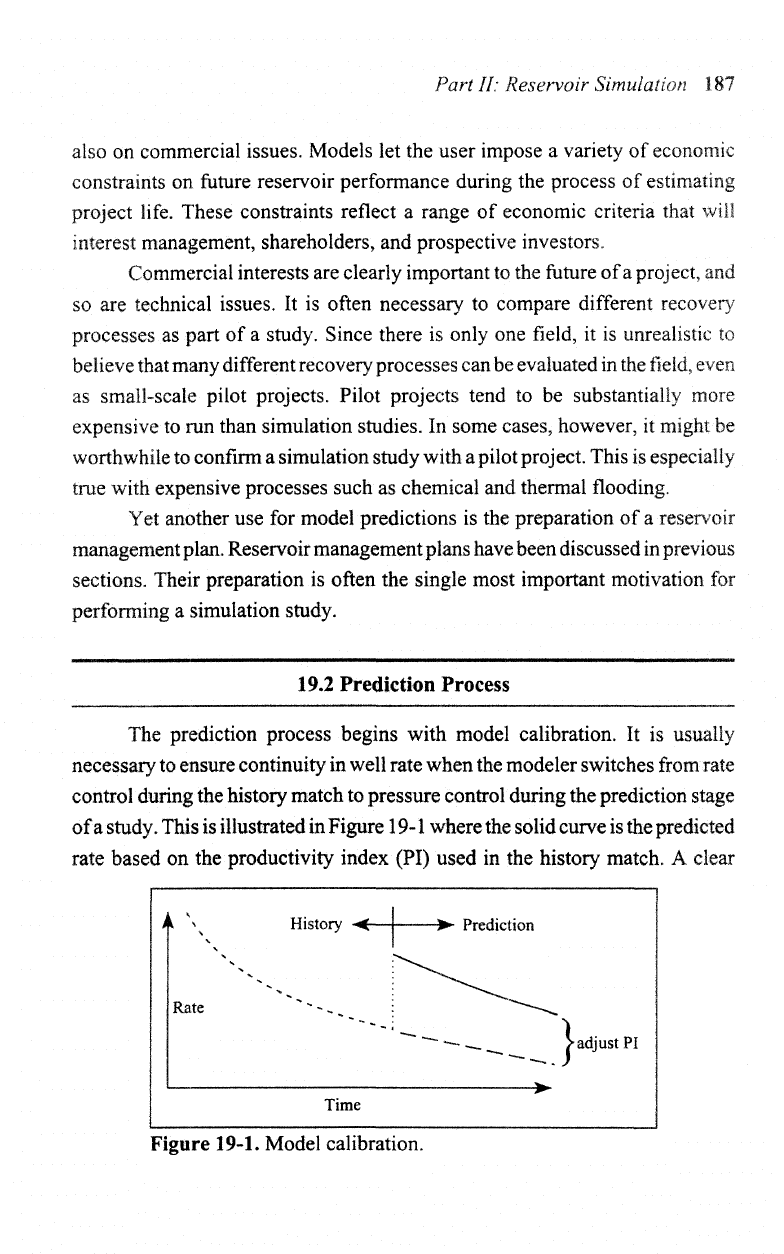
This
is
illustrated
in
Figure
19-1
where
the
solid curve
is the
predicted
rate based
on the
productivity index (PI) used
in the
history
match.
A
clear
History
-<
>•
Prediction
Rate
--,„
:
~"~~"~~
•—^
_
VadjustPI
Time
Figure
19-1. Model calibration.
TEAM LinG - Live, Informative, Non-cost and Genuine!

188
Principles
of
Applied
Reservoir Simulation
discontinuity
in
rate
is
observed between
the end of
history
and the
beginning
of
prediction.
The
rate
difference
usually arises because
the
actual
well
PI,
especially
skin
effect,
is not
accurately
modeled
by the
model
PL An
adjustment
to
model
PI
needs
to be
made
to
match
final
historical rate with
initial
predicted
rate.
The
next step
is to
prepare
a
base case prediction.
The
base case
prediction
is
a
forecast assuming existing operating conditions
apply.
For
example,
the
base
case
for a
newly developed
field
that
is
undergoing primary depletion should
be a
primary depletion
case
that extends
to a
user-specified economic limit.
By
contrast,
if the field was
being
waterflooded,
the
waterflood
should
be the
base
case
and
alternative strategies
may
include
gas
injection
and WAG
(water-
altemating-gas).
The
base case prediction establishes
a
basis
from
which
to
compare
changes
in field
performance resulting from changes
in
existing operating
conditions.
In
addition,
a
sensitivity
analysis
should
be
performed
to
provide
insight into
the
uncertainty
associated
with model predictions.
A
procedure
for
conducting
a
sensitivity analysis
is
outlined below.
19.3
Sensitivity
Analyses
Sensitivity analyses
are
often
needed
in
both
the
history matching
and
prediction stages [for example,
see
Crichlow,
1977;
Mattax
and
Dalton,
1990;
Saleri,
1993;
and
Fanchi,
et
al,
1996].
Any
method that quantifies
the
uncer-
tainty
or risk
associated
with selecting
a
particular prediction
case
may be
viewed
as a
sensitivity analysis.
An
example
of a
sensitivity analysis technique that
is
cost-effective
in
moving
a
history match forward
is
conceptual modeling.
It can
be
used
to
address very specific questions, such
as
determining
the
impact
of
fluid
contact movement
on
hydrocarbon recovery. Similarly, window models
that
study such issues
as the
behavior
of a
horizontal well
in a
fault
block
provide
useful
information
on the
sensitivity
of a
model
to
changes
in
input
parameters.
Another example
of a
sensitivity analysis technique
is
risk analysis.
Murtha
[1997]
defines risk analysis
as
"any
form
of
analysis that studies
and
hence
attempts
to
quantify
risks associated with
an
investment." Risk
in
this
TEAM LinG - Live, Informative, Non-cost and Genuine!

Pan II:
Reservoir Simulation
189
context
refers
to a
potential "change
in
assets associated
with
some chance
occurrences." Risk analysis generates probabilities associated
with
changes
of
model
input parameters.
The
parameter changes must
be
contained
within
ranges
that
are
typically determined
by the
range
of
available
data,
information
from
analogous
fields,
and the
experience
of the
modeling
team. Each model
run
using
a
complete
set of
model input parameters constitutes
a
trial.
A
large number
of
trials
can be
used
to
generate probability
distributions.
Alternatively,
the
results
of the
trials
can be
used
in a
multivariable
regression analysis
to
generate
analytical
expressions,
as
described below.
One of the
most widely used techniques
for
studying model sensitivity
to
input parameter changes
is to
modify model input parameters
in the
history
matched model.
The
following procedure combines multivariable regression
and
the
results
of
model trials
to
generate
an
analytical expression
for
quantifying
the
effect
of
changing model parameters.
Assume
a
dependent variable
F has the
form
F =
K
n
Xj
J
j*\
where
{Xj}
are n
independent variables
and K is a
proportionality constant that
depends
on the
units
of the
independent variables. Examples
of
Xj
are
well
separation, saturation
end
points,
and
aquifer strength. Taking
the
logarithm
of
the
defining
equation
for F
linearizes
the
function
F and
makes
it
suitable
for
multivariable regression analysis, thus
InF
=
InK
+ £
e
/m
X
j
7=1
A
sensitivity model
is
constructed using
the
following procedure:
4
Run a
model with
different
values
of
{Xj}
4
Obtain values
of F for
each
set of
values
of
{Xj}
The
constants
K,
{e-\
are
obtained
by
performing
a
multivariable regression
analysis using values
of F
calculated
from
the
model runs
as a
function
of
{Xj}
.
In
addition
to
quantifying behavior,
the
regression procedure provides
an
estimate
of
fractional change
of the
dependent variable
F
when
we
make
TEAM LinG - Live, Informative, Non-cost and Genuine!
1
90
Principles
of
Applied
Reservoir Simulation
fractional
changes
to the
independent variables
{Xj}
.
The
fractional change
in
F
is
given
by
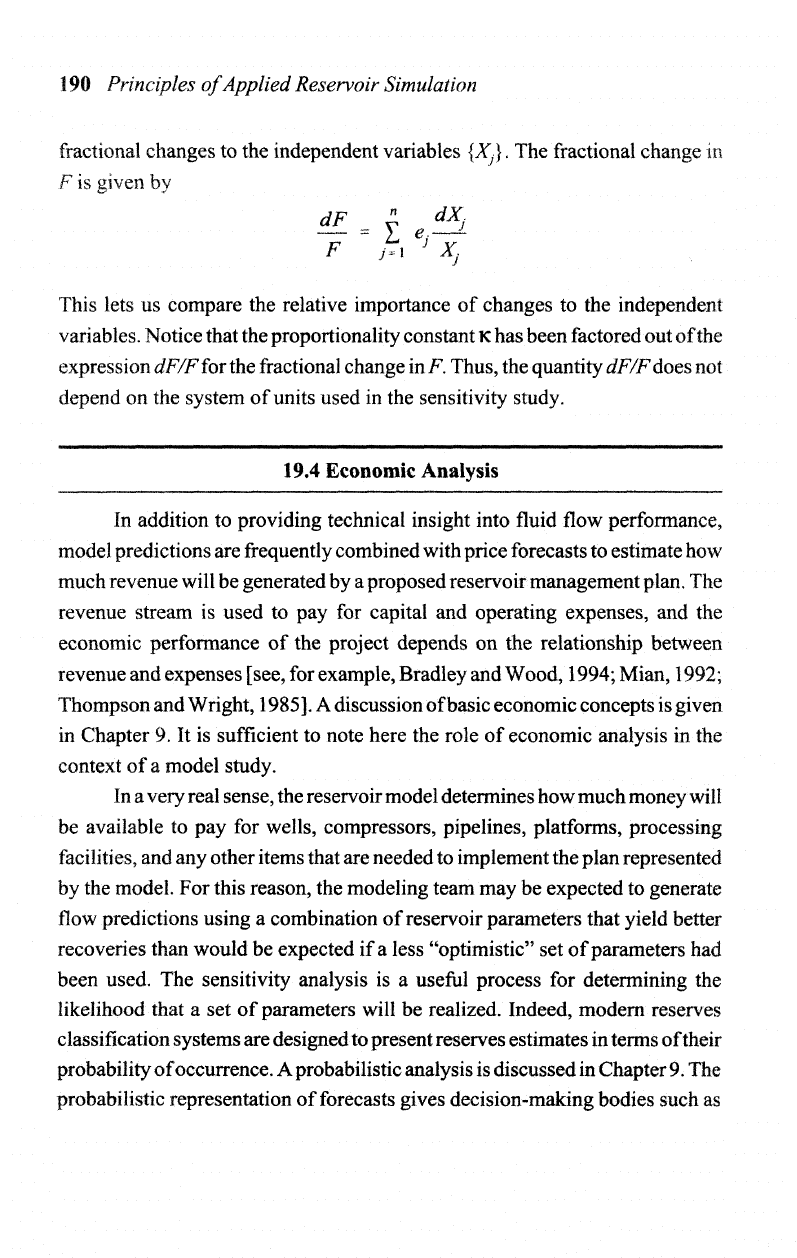
dF
dX
This
lets
us
compare
the
relative importance
of
changes
to the
independent
variables.
Notice that
the
proportionality constant
K
has
been factored
out of the
expression
dF/Ffor
the
fractional
change
in F.
Thus,
the
quantity
dF/Fdoes
not
depend
on the
system
of
units
used
in the
sensitivity
study.
19.4
Economic
Analysis
In
addition
to
providing technical insight into
fluid flow
performance,
model
predictions
are
frequently
combined
with
price forecasts
to
estimate
how
much
revenue
will
be
generated
by a
proposed
reservoir
management
plan.
The
revenue stream
is
used
to pay for
capital
and
operating expenses,
and the
economic performance
of the
project depends
on the
relationship between
revenue
and
expenses
[see,
for
example, Bradley
and
Wood,
1
994;
Mian,
1
992;
Thompson
and
Wright,
1
985].
A
discussion
of
basic
economic concepts
is
given
in
Chapter
9. It is
sufficient
to
note here
the
role
of
economic analysis
in the
context
of a
model study.
In
a
very real sense,
the
reservoir model determines
how
much money will
be
available
to pay for
wells, compressors, pipelines, platforms, processing
facilities,
and any
other items that
are
needed
to
implement
the
plan represented
by
the
model.
For
this reason,
the
modeling team
may be
expected
to
generate
flow
predictions using
a
combination
of
reservoir parameters that yield better
recoveries than would
be
expected
if a
less "optimistic"
set of
parameters
had
been
used.
The
sensitivity analysis
is a
useful
process
for
determining
the
likelihood
that
a set of
parameters will
be
realized. Indeed, modern reserves
classification
systems
are
designed
to
present reserves estimates
in
terms
of
their
probability
of
occurrence.
A
probabilistic analysis
is
discussed
in
Chapter
9. The
probabilistic
representation
of
forecasts gives decision-making bodies such
as
TEAM LinG - Live, Informative, Non-cost and Genuine!
Part
II:
Reservoir Simulation
191
corporate
managements
and
financial
institutions
the
information
they
need
to
make
informed
decisions.
19.5 Validity
of
Model
Predictions
The
validity
of
model predictions
was
studied
by
Saleri [1993]
who
compared
actual
field
performance with predicted performance.
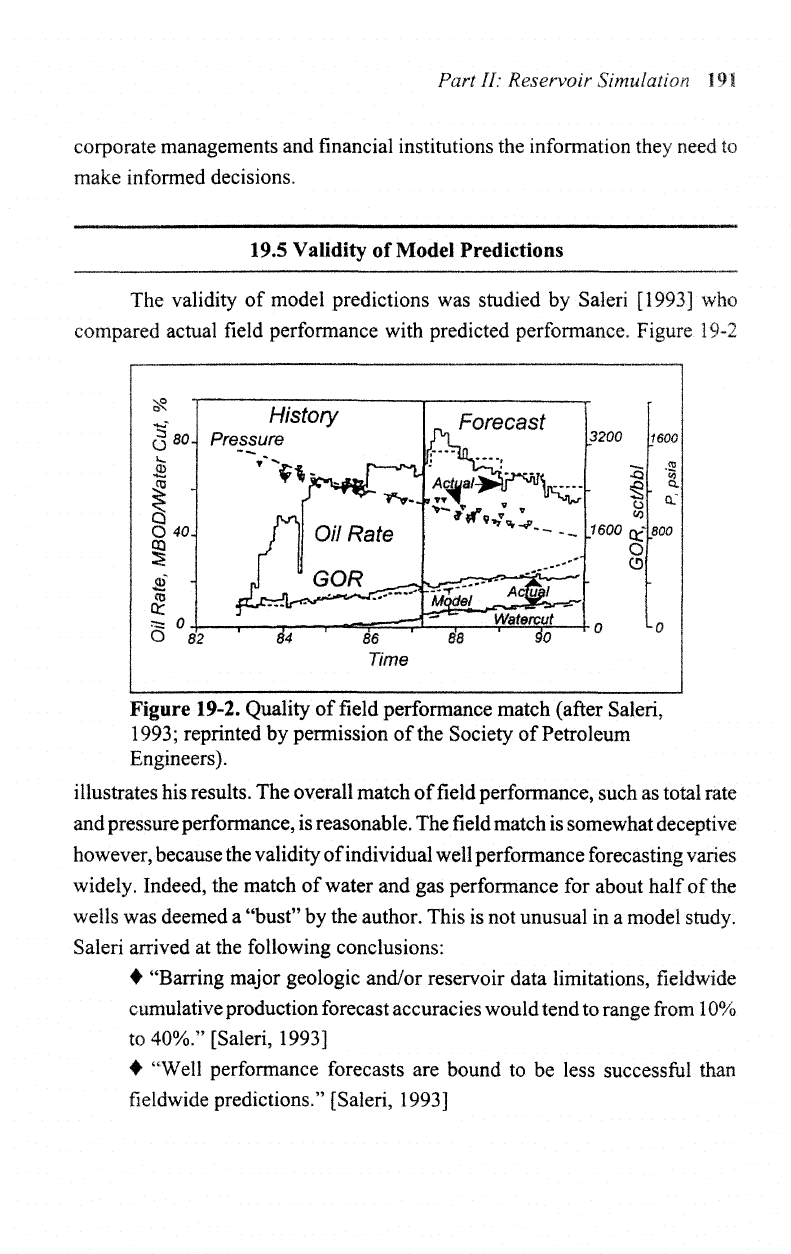
Figure
19-2
sp
~l
'
ts"'
o
80
-
&
^
"
Q
O 40-
,g>
-
2
0
History
Pressure
*
^1
j\n.
J\
J
_J
.vJt
_
XtyrCrdlr'
O 82
$4
'k-^teT"^
1
f^^^
w..
O/7
Rate
GOR_,
_
-x^K^v^r-**
Forecast
Art
aJSSp"^
»
"H
3
^"^
^^"
**f"9^
*
"-
--
"^r^^ii
-*
Watercut
3200
5
O
to
_7600
Q;
0
7600
•2
o:
800
OO
80
£rv
7/me
Figure 19-2. Quality
of field
performance match (after
Saleri,
1993;
reprinted
by
permission
of the
Society
of
Petroleum
Engineers).
illustrates
his
results.
The
overall match
of field
performance, such
as
total
rate
and
pressure performance,
is
reasonable.
The field
match
is
somewhat deceptive
however, because
the
validity
of
individual well performance forecasting varies
widely. Indeed,
the
match
of
water
and gas
performance
for
about half
of the
wells
was
deemed
a
"bust"
by the
author. This
is not
unusual
in a
model study,
Saleri arrived
at the
following
conclusions:
4
"Barring
major
geologic
and/or
reservoir data limitations,
fieldwide
cumulative
production forecast accuracies would tend
to
range
from
10%
to
40%."
[Saleri, 1993]
4*
"Well performance forecasts
are
bound
to be
less successful than
fieldwide
predictions." [Saleri, 1993]
TEAM LinG - Live, Informative, Non-cost and Genuine!
