Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.


38
THEORY AND APPLICATIONS
OF
SPECIAL FUNCTIONS
Dividing out common factors and letting
k
-+
oo
gives
C
=
0.
We can
now conclude that
G(w)
r
0,
or that is,
We complete the proof with a simple argument that gives
g
(qm)
=
0,
m
=
0,1,
.
.
. .
If
G(w)
=
0
then
Letting
k
-+
0
gives
g(1)
=
0.
Then dividing
by
q2k
and again letting
k
-+
oo
gives
g(q)
=
0.
Continuing this process we have
g
(qm)
r
0
and
the proof of the theorem is complete.
0
References
Abreu, L. D., Bustoz, J., and Cardoso, J.
L.
(2003). The roots of the third Jackson
q-Bessel function. Internat.
J.
Math. Math. Sci., 67:4241-4248.
Boas, R. P. and Pollard, H. (1947). Complete sets of Bessel and Legendre functions.
Ann. of Math., 48:366-384.
Dalzell, D. P. (1945). On the completeness of a series of normal orthogonal functions.
J.
Lond. Math. Soc., 20:87-93.
Exton, H. (1983). q-Hypergeometric Functions and Applications. Ellis Horwood, Chich-
ester.
Higgins, J. R. (1977). Completeness and basis properties of sets of special functions.
Cambridge University Press, London, New York, Melbourne.
Ismail, M. E.
H.
(1982). The zeros of basic Bessel functions, the functions J,+,,(x),
and associated orthogonal polynomials.
J.
Math. Anal. Appl., 86:l-19.
Ismail,
M.
E.
H.
(2003). Some properties of jackson's third q-bessel function. Preprint.
Jackson,
F.
H. (1904). On generalized functions of Legendre and Bessel. Transactions
of the Royal Society of Edinburgh, 41:l-28.
Koelink,
H.
T. (1999). Some basic Lommel polynomials. Journal of Approxzmation
Theory, 96:345-365.
Koelink,
H.
T.
and Swarttouw, R.
F.
(1994). On the zeros of the Hahn-Exton q-Bessel
function and associated q-Lommel polynomials.
J.
Math. Anal. Appl., 186:690-710.
Kvitsinsky, A.
A.
(1995). Spectral zeta functions for q-Bessel equations.
J.
Phys. A:
Math. Gen., 28:1753-1764.
Levin, B. Y. (1980). Distribution of zeros of Entire Functions. American Mathematical
Society, Providence, RI.
Swartouw, R.
F.
(1992). The Hahn-Exton q-Bessel Function. PhD thesis, Technische
Universiteit Delft.
a-GAUSSIAN POLYNOMIALS AND FINITE
ROGERS-RAMANUJAN IDENTITIES
George
E.
Andrews*
Department
of
Mathematics
The Pennsylvania State University
University Park, PA
16802
andrews@math.psu.edu
Abstract
Classical Gaussian polynomials are generalized to two variable poly-
nomials. The first half of the paper is devoted to a full account of
this extension and its inherent properties. The final part of the paper
considers the role of these polynomials in finite identities of the Rogers-
Ramanujan type.
Keywords:
Rogers-Ramanujan identities, Gaussian polynomials
1.
Introduction
Our object in this paper is to better understand certain classical gen-
eralizations of the Rogers-Ramanujan identities (Andrews,
1976,
p.
104):
and
where
Iq1
<
1
and
and
w
'Partially supported
by
National Science Foundation Grant DMS9206993.
O 2005
Springer Science+Business Media, Inc.
40
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
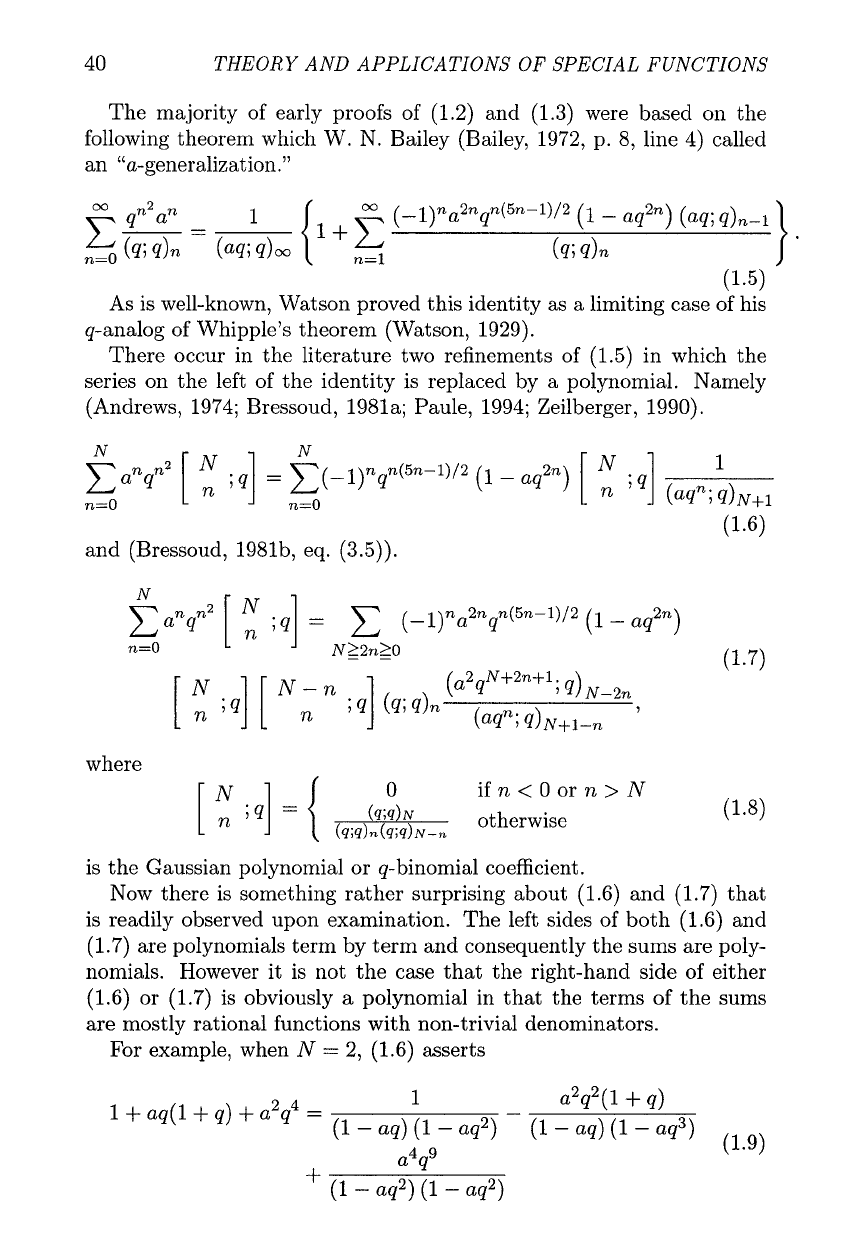
The majority of early proofs of (1.2) and (1.3) were based on the
following theorem which W. N. Bailey (Bailey, 1972, p. 8, line 4) called
an "a-generalization."
00
(-1)"
2n
n(5n-1)/2
1
aq
(
-
aq2n) (aq; q)n-1
(4; q)n
(1.5)
As is well-known, Watson proved this identity
as
a limiting case of his
q-analog of Whipple's theorem (Watson, 1929).
There occur in the literature two refinements of (1.5) in which the
series on the left of the identity is replaced by a polynomial. Namely
(Andrews, 1974; Bressoud, 1981a; Paule, 1994; Zeilberger, 1990).
and (Bressoud, 1981b, eq. (3.5)).
n=O
where
is the Gaussian polynomial or q-binomial coefficient.
Now there is something rather surprising about (1.6) and (1.7) that
is readily observed upon examination. The left sides of both (1.6) and
(1.7) are polynomials term by term and consequently the sums are poly-
nomials. However it is not the case that the right-hand side of either
(1.6) or (1.7) is obviously a polynomial in that the terms of the sums
are mostly rational functions with non-trivial denominators.
For example, when
N
=
2,
(1.6) asserts
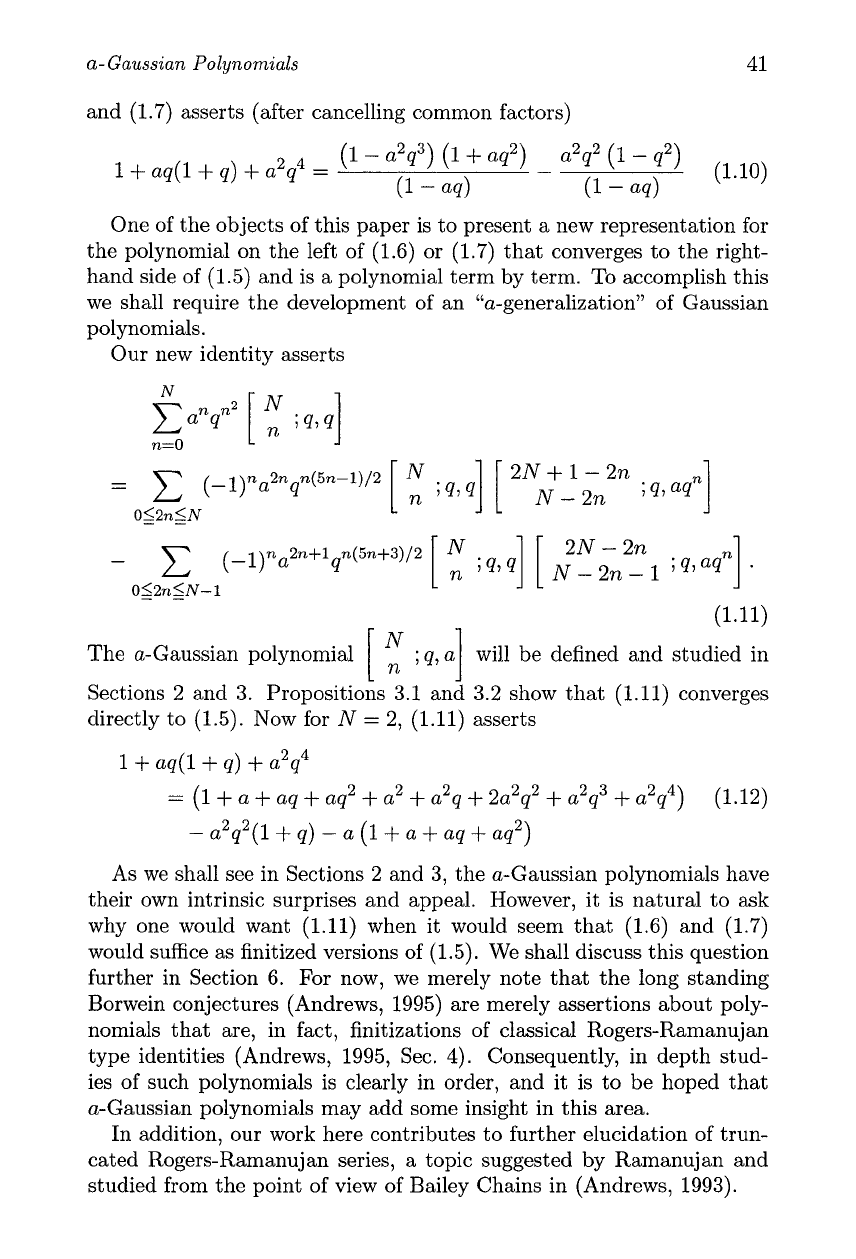
a-
Gaussian Polynomials
41
and (1.7) asserts (after cancelling common factors)
One of the objects of this paper is to present a new representation for
the polynomial on the left of (1.6) or (1.7) that converges to the right-
hand side of (1.5) and is a polynomial term by term. To accomplish this
we shall require the development of an "a-generalization" of Gaussian
polynomials.
Our new identity asserts
The a-Gaussian polynomial
1
;
;
q, a] will be defined and studied in
L
J
Sections 2 and 3. Propositions 3.1 and 3.2 show that (1.11) converges
directly to (1.5). Now for
N
=
2, (1.11) asserts
As we shall see in Sections 2 and 3, the a-Gaussian polynomials have
their own intrinsic surprises and appeal. However, it is natural to ask
why one would want
(1.11)
when it would seem that (1.6) and (1.7)
would suffice as finitized versions of (1.5). We shall discuss this question
further in Section 6. For now, we merely note that the long standing
Borwein conjectures (Andrews, 1995) are merely assertions about poly-
nomials that are, in fact, finitizations of classical Rogers-Ramanujan
type identities (Andrews, 1995, Sec.
4).
Consequently, in depth stud-
ies of such polynomials is clearly in order, and it is to be hoped that
a-Gaussian polynomials may add some insight in this area.
In addition, our work here contributes to further elucidation of trun-
cated Rogers-Ramanujan series, a topic suggested by Ramanujan and
studied from the point of view of Bailey Chains in (Andrews, 1993).
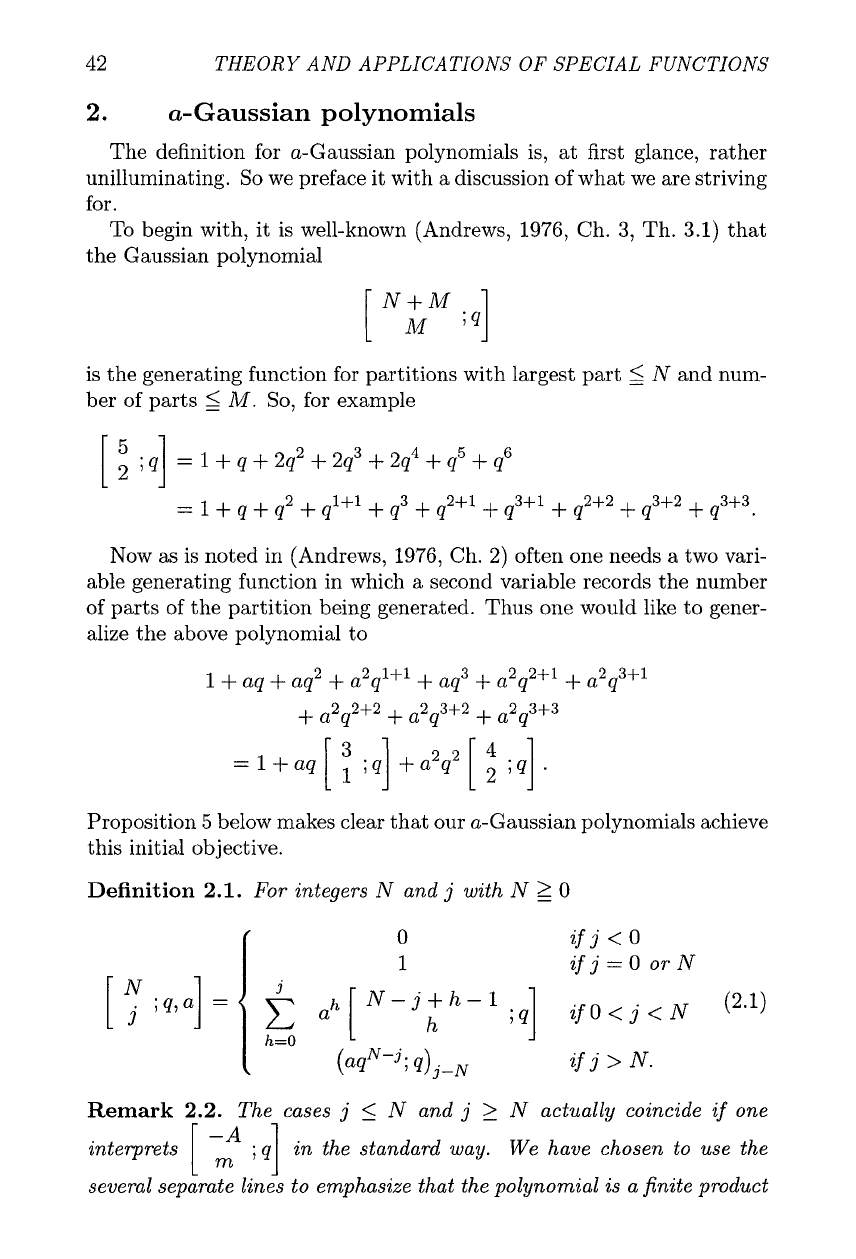
42
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
2.
a-Gaussian polynomials
The definition for a-Gaussian polynomials is, at first glance, rather
unilluminating. So we preface it with a discussion of what we are striving
for.
To begin with, it is well-known (Andrews, 1976, Ch. 3, Th. 3.1) that
the Gaussian polynomial
is the generating function for partitions with largest part
5
N
and num-
ber of parts
5
M.
So, for example
Now as is noted in (Andrews, 1976, Ch.
2)
often one needs a two vari-
able generating function in which a second variable records the number
of parts of the partition being generated. Thus one would like to gener-
alize the above polynomial to
Proposition
5
below makes clear that our a-Gaussian polynomials achieve
this initial objective.
Definition
2.1.
For integers
N
and
j
with
N
2
0
Remark
2.2.
The cases
j
5
N
and
j
2
N
actually coincide
if
one
interprets
1
2
;
q]
in the standard way. We have chosen to use the
L
J
several separate lines to emphasize that the polynomial is a finite product

a-
Gaussian Polynomials
43
when
j
>
N.
The more succinct representation would have sacrificed
clarity.
We shall now prove seven propositions about a-Gaussian polynomi-
als. The first one establishes that we have truly generalized the classical
Gaussian polynomials. Propositions
2.4-2.6
are the natural extensions
of the Pascal triangle recurrences for Gaussian polynomials. Proposi-
tion
2.7
establishes the connection with partitions that we described at
the beginning of this section. Proposition
2.8
is a naturally terminating
representation of a-Gaussian polynomials. Proposition
2.9
is the nat-
ural extension of the finite geometric series summation to a-Gaussian
polynomials.
Proposition
2.3.
For integers
N
and
j
with
N
2
0,
[
7
;
q, q]
=
[
7
;q~.
Proof.
Clearly both sides are identically
0
if
j
<
0
or
j
>
N.
Also both
sides equal
1
when
j
=
0
or
N.
Finally, for
0
<
j
<
N
by (Andrews,
1976,
p.
37,
eq.
(3.3.9)).
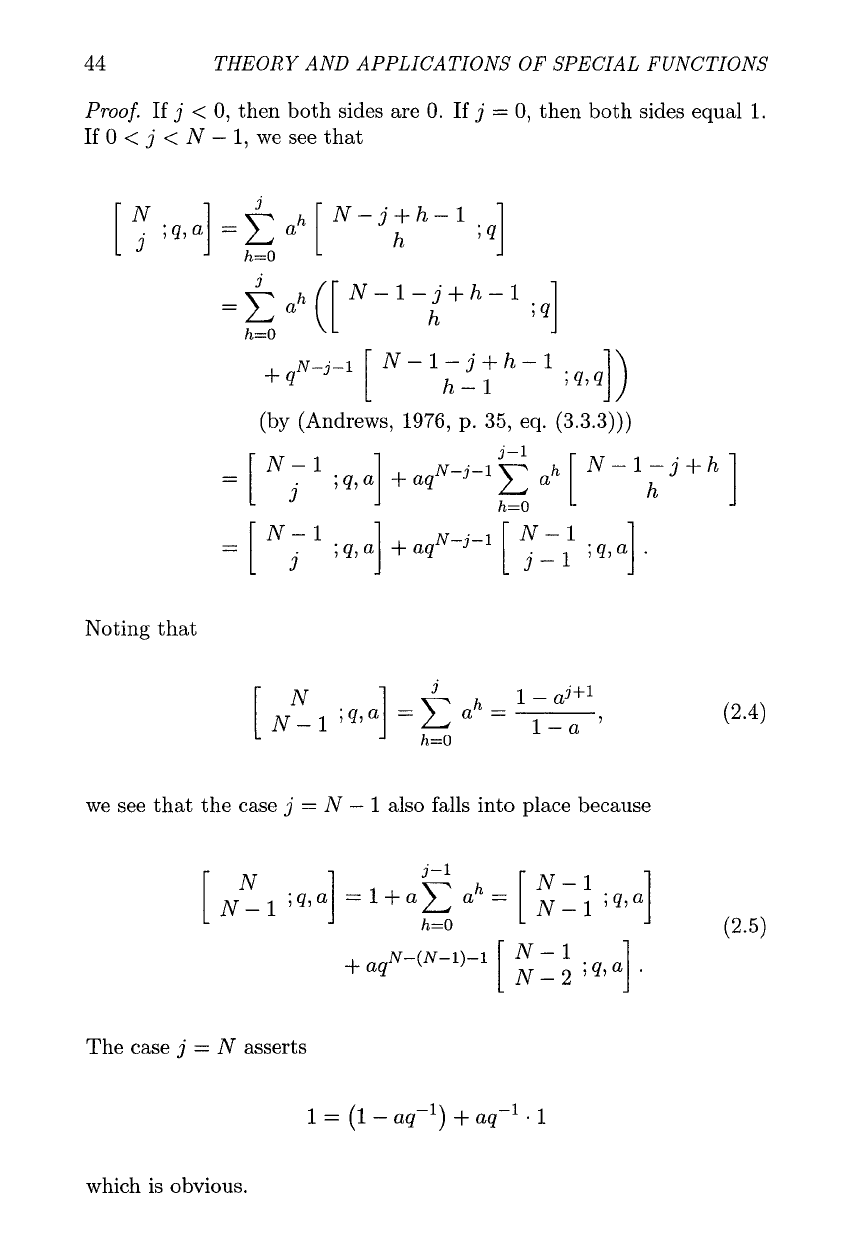
Proposition
2.4.
For integers
N
and
j
with
N
2
1,
44
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Proof.
If
j
<
0, then both sides are
0.
If
j
=
0, then both sides equal 1.
If
0
<
j
<
N
-
1, we see that
(by (Andrews, 1976, p.
35,
eq.
(3.3.3)))
Noting that
we see that the case
j
=
N
-
1
also falls into place because
The case
j
=
N
asserts
1
=
(I
-
aq-l)
+
aqF1
. I
which is obvious.

a-
Gaussian
Polynomials
Finally, if
j
>
N
Thus Proposition
2.4
is established.
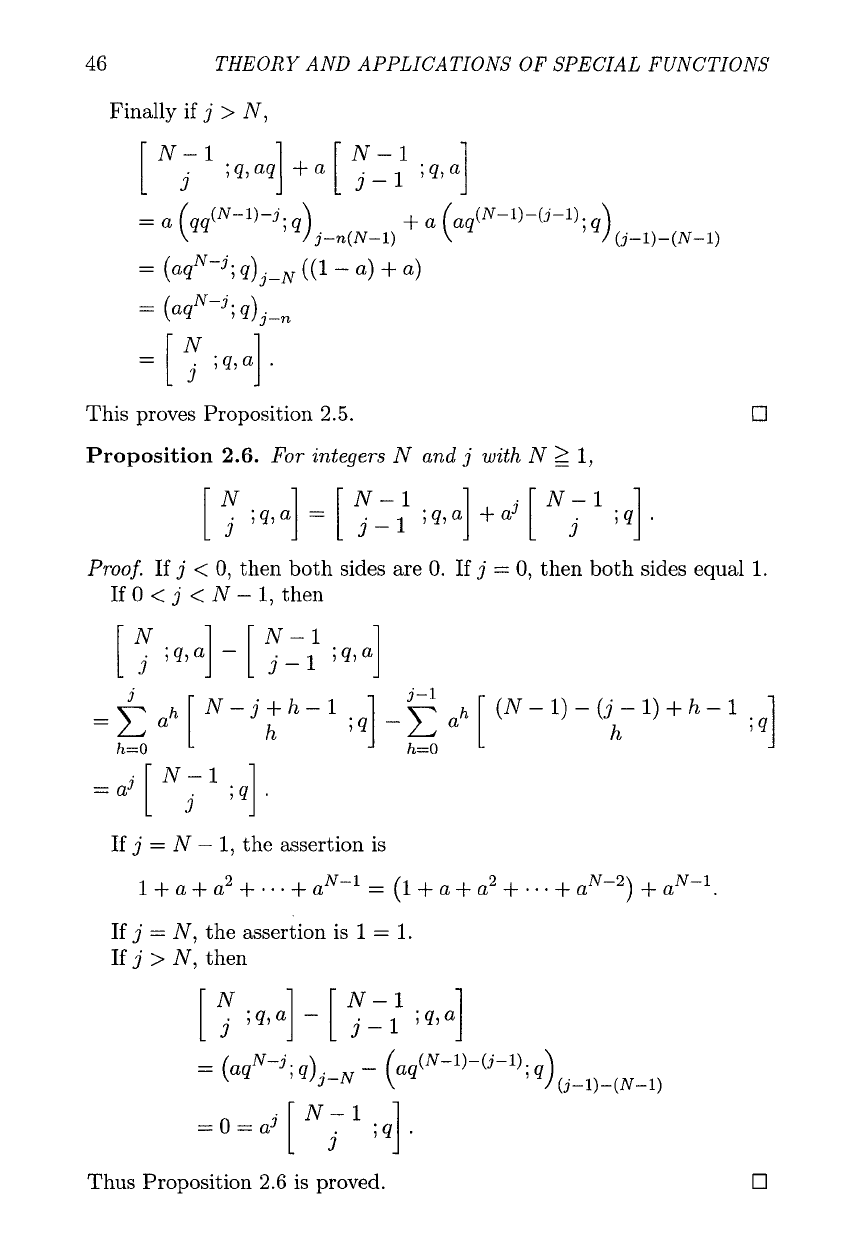
Proposition
2.5.
For integers
N
and
j
with
N
2
1,
Proof.
If
j
<
0, then both sides of this equation are identically 0. If
j
=
0, then both sides equal
1.
If
0
<
j
<
N
-
1,
then
If
j
=
N
-
1, the assertion is
which is immediate.
If
j
=
N, the assertion is
which is obvious.
46
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Finally if
j
>
N,
This proves Proposition
2.5.
Proposition
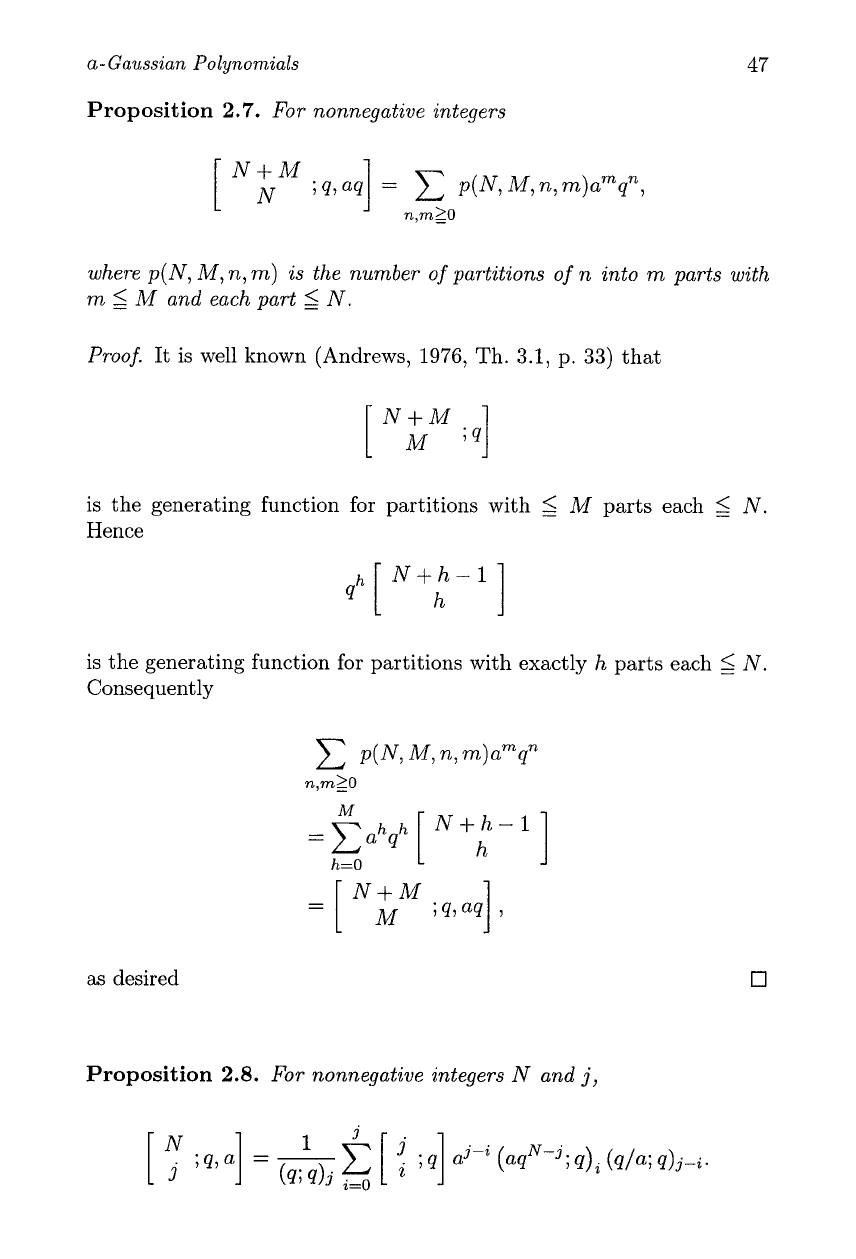
2.6.
For integers
N
and
j
with
N
2
1,
Proof.
If
j
<
0, then both sides are 0. If
j
=
0, then both sides equal 1.
IfO<j<N-1,then
If
j
=
N
-
1,
the assertion is
1
+a+a2
+
..
.
+aN-'
=
(1
+a+a2
+
-.-+aNv2) +aN-'.
If
j
=
N, the assertion is
1
=
1.
If
j
>
N, then
Thus Proposition
2.6
is proved.
a-Gaussian Polynomials
Proposition
2.7.
FOI
where p(N, M, n, m) is
nonnegative integers
the number of partitions of
n
into m parts with
m
5
M and each part
5
N.
Proof. It is well known (Andrews, 1976, Th. 3.1, p. 33) that
is the generating function for partitions with
5
M
parts each
5
N.
Hence
is the generating function for partitions with exactly
h
parts each
5
N.
Consequently
as
desired
Proposition
2.8.
For nonnegative integers N and
j,
