Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.

474
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
know that the condition
a
2
>
is necessary for nonnegative linearization
to hold. Also if
a
=
,O
then the condition
a
2
-;
is necessary (see
(Askey, 1975)). Let
a
>
P.
By Proposition 5.4 the sequence
should be nondecreasing, which holds only if
a
+
,O
2
0. Hence the
conditions on
a
and
p
are necessary for (SNLP).
Now we are going to show that the conditions on the parameters are
also sufficient for (SNLP). Assume first that
a
=
P
>
-112. Let
J?'"
(x)
Rn
(x)
=
J?@'
(1)
'
Then by (Koekeok and Swarttouw, 1998, (1.8.1)) (1.8.3)) the polynomi-
als satisfy
Hence by Corollary 5.2 the polynomials satisfy (SNLP).
Assume now that
a
>
,8
>
-1
and
a
+
p
>
0. Let pn(x) denote the
monic version of Jacobi polynomials, i.e., let
By (Askey, 1970) the polynomials pn satisfy the assumptions of Corollary
5.2 if
a
+
P
2
1. Hence they satisfy (SNLP).
We have to consider the remaining case when
a
>
P
>
-1
and
0
5
a
+
,!3
<
1.
By (6.1) we have
These numbers satisfy the assumptions of Corollary 5.3 for
a
2
/3
and
0
5
a
+
,B
5
1.
Indeed, observe that for
n
>
0 we have
Strong nonnegative linearization of orthogonal polynomials
and
These calculations are valid only for
n
>
0, because
a0
=
0 does not
coincide with
(6.2).
The formulas
(6.2)
and
(6.4)
show that
an
is non-
decreasing and
yn
is nonincreasing when
a
+
P
5
1.
Both sequences
tend to
i.
This gives the conditions (ii) and (iv) of Corollary
5.3.
The
formula
(6.5)
shows that
an
+
yn
is nondecreasing for
n
>
0, regardless
the sign of
ap.
This and the fact that
an
is nondecreasing imply
Thus the condition (iii) of Corollary
5.3
is satisfied for
0
<
m
<
n
-
1.
It remains to show the condition (iii) for
m
=
0,
i.e.
By
(6.2)
and
(6.4)
the above inequality is equivalent to the following.
Observe that the left hand side of
(6.6)
is a decreasing function of
a
-
P.
Therefore we can assume that
a
-
P
attains the maximal possible value,
i.e.,
p
=
-1.
Let
/3
=
-1
and
x
=
2n+a+P+1.
Then
x
2
2+a+P+1>
3.
The left hand side of
(6.6)
can be now written as follows.
-
(a+
1)2
+
(a+
1)2
-
1
(a
-
1)2
-
1
(a-
1I2
-
1
-
x+l x+2 x
-
2
+
x-1
- -
4
-
(a
+
q2
-
(a
-
1)2
(x-2)(x+2) (x+l)(x+2) (x-l)(x-2)
-
4
-
4 4
-
(a
+
1)2
(a
-
1)2
+
(x-2)(x+2) (x+l)(x+2) (x+l)(x+2) (x-l)(x-2).
476
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
The first two terms of the last expression give a positive contribution to
the sum because x
>
2.
Hence it suffices to show that
Note that
a
-
1
2
0
(as
/3
=
-1).
Thus
a
+
1
2
2
and 4
-
(a
+
1)2
5
0.
Hence the left hand side of (6.7) is a nondecreasing function of
x.
Therefore we can verify (6.7) only for the smallest value of x, that is for
x
=
2
+
a
+
,G'
+
1
=
2
+
a.
Under substitution x
=
2
+
a
the inequality
(6.7) takes the form
After simple transformations it reduces to
which is true because
a
is nonnegative. Summarizing, Corollary
5.3
yields that for
a
>
P
and
0
5
a
+
P
5
1
we get (SNLP).
0
References
Askey, R. (1970). Linearization of the product of orthogonal polynomials. In Gun-
ning, R., editor, Problems in Analysis, pages 223-228. Princeton University Press,
Princeton, NJ.
Askey,
R.
(1975). Orthogonal polynomials and special functions, volume 21 of Re-
gional Conference Series in Applied Mathematics. Society for Industrial and Ap-
plied Mathematics, Philadelphia, PA.
Gasper,
G.
(1970a). Linearization of the product of Jacobi polynomials, I. Canad.
J.
Math., 22:171-175.
Gasper, G. (1970b). Linearization of the product of Jacobi polynomials,
11.
Canad.
J.
Math., 22:582-593.
Gasper, G. (1983). A convolution structure and positivity of a generalized transla-
tion operator for the continuous q-Jacobi polynomials. In Conference on harmonic
analysis in honor of Antoni Zygmund, Vol.
I,
11
(Chicago, Ill., 1981), Wadsworth
Math. Ser., pages 44-59. Wadsworth, Belmont, CA.
Gasper, G. and Rahman, M. (1983). Nonnegative kernels in product formulas for
q-racah polynomials
I.
J.
Math. Anal. Appl., 95:304-318.
Hylleraas,
E.
(1962). Linearization of products of Jacobi polynomials. Math. Scand.,
10:189-200.
Koekeok, R. and Swarttouw,
R.
F.
(1998). The Askey-scheme of hypergeometric or-
thogonal polynomials and its q-analogue. Faculty of Technical Mathematics and
Informatics 98-17, TU Delft.
Markett,
C.
(1994). Linearization of the product of symmetric orthogonal polynomials.
Constr. Approx., 10:317-338.

Strong nonnegative linearization of orthogonal polynomials
477
Mlotkowski,
W.
and Szwarc, R. (2001). Nonnegative linearization for polynomials
orthogonal with respect to discrete measures. Constr. Approx., 17:413-429.
Rahman, M. (1981). The linearization of the product of continuous q-Jacobi polyno-
mials. Can.
J.
Math., 33:961-987.
Rogers,
L.
J.
(1894). Second memoir on the expansion of certain infinite products.
Proc. London Math. Soc., 25:318-343.
Szwarc,
R.
(1992a). Orthogonal polynomials and a discrete boundary value problem,
I.
SIAM
J.
Math. Anal., 23:959-964.
Szwarc, R. (199213). Orthogonal polynomials and a discrete boundary value problem,
11.
SIAM
J.
Math. Anal., 23:965-969.
Szwarc, R. (1995). Nonnegative linearization and q-ultraspherical polynomials. Meth-
ods Appl. Anal., 2:399-407.
Szwarc, R. (2003).
A
necessary and sufficient condition for nonnegative linearizatiom
of orthogonal polynomials. To appear.
REMARKS ON SOME BASIC
HYPERGEOMETRIC SERIES
Changgui Zhang
Laboratoire AGAT (UMR
-
CNRS
8524)
UFR Math.
Universite' des Sciences et Technologies de Lille
Cite' Scientifique
59655
Villeneuve d'Ascq cedex
FRANCE
zhang@agat.univ-lillel.fr
Abstract
Many results in Mathematical Analysis seem to come from some "ob-
vious" computations. For a few years, we have been interested in the
analytic theory of linear q-difference equations. One of the problems we
are working on is the analytical classification of q-difference equations.
Recall that this problem was already considered by
G.
D.
Birkhoff and
some of his students ((Birkhoff, 1913), (Birkhoff and Giienther, 1941)).
An important goal of these works is to be able to derive transcendental
analytical invariants from the divergent power series solutions; that is,
to be able to define a good concept of Stokes' multiplier for divergent
q-series! Very recently, we noted ((Zhang, 2002), (Ramis et al., 2003))
that this problem can be treated in a satisfactory manner by a new
summation theory of divergent power series through the use of Jaco-
bian theta functions and some basic integral calculus. The purpose of
the present article is to explain how much 'Lobvious" this mechanism
of summation may be if one practises some elementary calculations on
q-series. It would be
a
very interesting question to understand (Di Vizio
et al., 2003) whether Ramanujan's mysterious formulas are related to
this transcendental invariant analysis.
. .
The article contains four sections. In the first section, we explain how
to use the theta function for giving a q-integral representation which
remains valid for the sum function of every convergent power series. It is
this integral representation which leads us to a new process of summation
of divergent series. Some identities then follow on the convergent power
series, in the spirit of a Stokes analysis: the convergence takes place only
in spite of the Stokes phenomenon!
O
2005
Springer Science+Business Media, Inc.

480
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
It is well known that every basic hypergeometric series
290
is formal
limit of a family of 291's. In (Zhang, 2002), we have applied the new
summation method to this divergent power series
290.
In the third
section of our present article, we prove that each sum of
290
is exactly
the limit of an associated family of
291
while the parameter tends to
infinity following a q-spiral.
In the last section, we give a remark about the Euler's
I?
function. The
best known q-analog of
I?
is certainly the Jackson's
I',
(Askey, 1978),
which satisfies a first order q-difference equation deduced from the fun-
damental equation of
I?.
This q-difference equation has a formal Laurent
series solution that is divergent everywhere in
@
(Zhang, 2001). Using
a summation formula of Ramanujan for and the above-mentioned
summation method, one gets a new q-analog of
I?
which is meromorphic
on
@*.
Some
basic
notations.
In the following,
q
denotes a real number in
the open interval (0,l) and some notations of the book (Gasper and
Rahman, 1990) will be used. For example, if
a
E
@,
we set (a; q)o
=
1,
for al,
.
.
.
,
ae
E
(C,
we set:
To each fixed
X
E
C*,
we associate its so-called q-spiral
[A;
q] by setting
XqZ
=
[A; q]
=
{Xqn
:
n
E
Z).
If
A,
p
E
@*,
the following conditions are
equivalent:
1.
How to get the sum of a power series
by
means
of
8
Let us denote by 04(x) or more shortly O(x) the theta function of
Jacobi, given by the following series:
Jacobi's triple product formula says that:

Remarks on Some Basic Hypergeometric Series
481
from this, it follows that O(x)
=
0
if and only if x
E
[-1;
q].
Recall
also that
6'
verifies the fundamental equation O(x)
=
xO(qx) or, more
generally,
6'
(qnx)
=
q-
n(n-1)/2
X
-n
6'b)
(1.1)
for any
n
E
Z.
Consider any given power series
f
=
C
anxn with complex coeffi-
n20
cients and let
X
be an arbitrary nonzero complex number.
Suppose
the radius
R
of convergence of
f
is
>
0.
It is obvious that the
product
f
(x)O(X/x) defines an analytical function in the truncated disc
0
<
1x1
<
R. From direct computations, one obtains the following iden-
where
cpf
denotes the entire function, depending upon
f,
defined
as
follows:
n20
It is useful to note that for any
B
>
1/R, there exists
C
>
0
such that
According to (Ramis, 1992), the function
cpf
is said
to have a q-exponential
growth of order (at most) one at infinity.
Let C{x) be the ring of all power series that converge near x
=
0
and
Eq;1
the set of all entire functions having at most a q-exponential growth
of order one at infinity.
Proposition
1.1.
The map f
I+
cpf
given
in
(1.3)
establishes a bijection
between
C{x)
and
IEq;1.
More precisely,
if
cp
=
cpf,
f
E
C{x)
and
if
R
>
0
is the radius of
convergence off, then the following assertions hold.
1.
For any
0
<
r
<
R,
let
C&
be the counterclockwise-oriented circle
centered at the origin and of radius r, then
2.
For any
X
E
C*,
the following q-integral

482
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
defines an analytical function which is equal to the sum of the
convergent power series
f
in
the open disc
0
<
1x1
<
R
minus the
points of the set
(-AqZ)
(i.e., the q-spiral
[-A;
q]).
Accordingly, one can obtain the sum of a convergent power series
by the following process:
Proof.
Applying Cauchy's formula to
cpf
gives the growth of its coeffi-
cients, from which we deduce that the map
f
H
cpf
is surjective (see
(Ramis, 1992)). It is obvious that this map is also injective. Note that
the q-integral representation of 1.1 is a reformulation of the identity
(1.2), while the formula (1.4) follows directly from Cauchy.
0
According to (Zhang, 2002) and (Ramis and Zhang, 2002), we shall
denote by
$;l
f
the power series
ipf
of (1.3) and by
,CF1cp
the q-integral
-.
of (1.5). It is important to remark that the convergence of
,C$F1cp
de-
pends only on the asymptotic behaviour of the function
cp(J)
as
J
goes
to
co
along the q-spiral
[A;
q]. So, let Hz1 be the set of analytic func-
tion germs at the origin that can be analytically continued to a function
having a q-exponential growth of order one at infinity in a neighborhood
of
[A;
q].
Here we call
neighborhood of
[A;
q]
any domain
V
c
@
such
that there exists a neighborhood
U
of
X
in
@*
for which the inclusion
{Jqn
:
,$
E
U,
n
E
Z)
C
V
holds. It is essential to notice that Eq;1
c
H!iql
for every
A
E
@*,
the inclusion being strict. Therefore, the process (1.6)
allows us to
sum
not only the convergent power series, but also any
power series
f^
that can be transformed by
f^
H
Bq;1f^
to be an element
of
w!:].
By definition, these power series
f^
are called [A;
q]-summable,
of sum
,C!;
o
$;l
f^
.
Here one uses the notation
"
j"
instead of
"
f"
,
be-
cause the series under consideration is not necessarily convergent: one
will have to distinguish a power series from "its sum(s)".
In (Zhang, 2002), it is shown that the summation process
f^
+
,C2F1
o
l?,;lf"
can be applied to every formal power series solution of any q-
difference equation if this equation is, in some sense, generically singu-
lar. For example, all basic hypergeometric series zcpo(a,
b;
-;
q, x) are
summable by this method. For more details, see (Zhang, 2002) and
(Ramis and Zhang, 2002).
Remarks on Some Basic Hypergeometric Series
483
2.
Identities characterizing the convergence of a
power series
Let
cp
=
cpf,
f
E
C{x) (i.e.,
f
is a convergent power series) and
suppose
R
is the radius of convergence of
f.
Since O(-1)
=
0, putting
X
=
5
and x
=
-5
in (1.2) gives the following formula:
which is valid for any
5
E
C such that
151
<
R.
The equality (2.1) can be viewed as an identity characterizing the
convergence of the power series
f
such that
cp
=
cpf. Indeed, let
cp
be
any analytical function known in a domain U of the form
U
=
(5
E
cC
:
I<(
<
1)
U
(5
E
C*
:
-a
<
arg x
<
a),
where
a
E
(0,
T).
Suppose that
cp
has a q-exponential growth of order at most one at infinity. For each
x
E
U such that
-a
<
argx
<
a
and 1x1
<
R
(for a suitable fixed real
R
>
0), we write
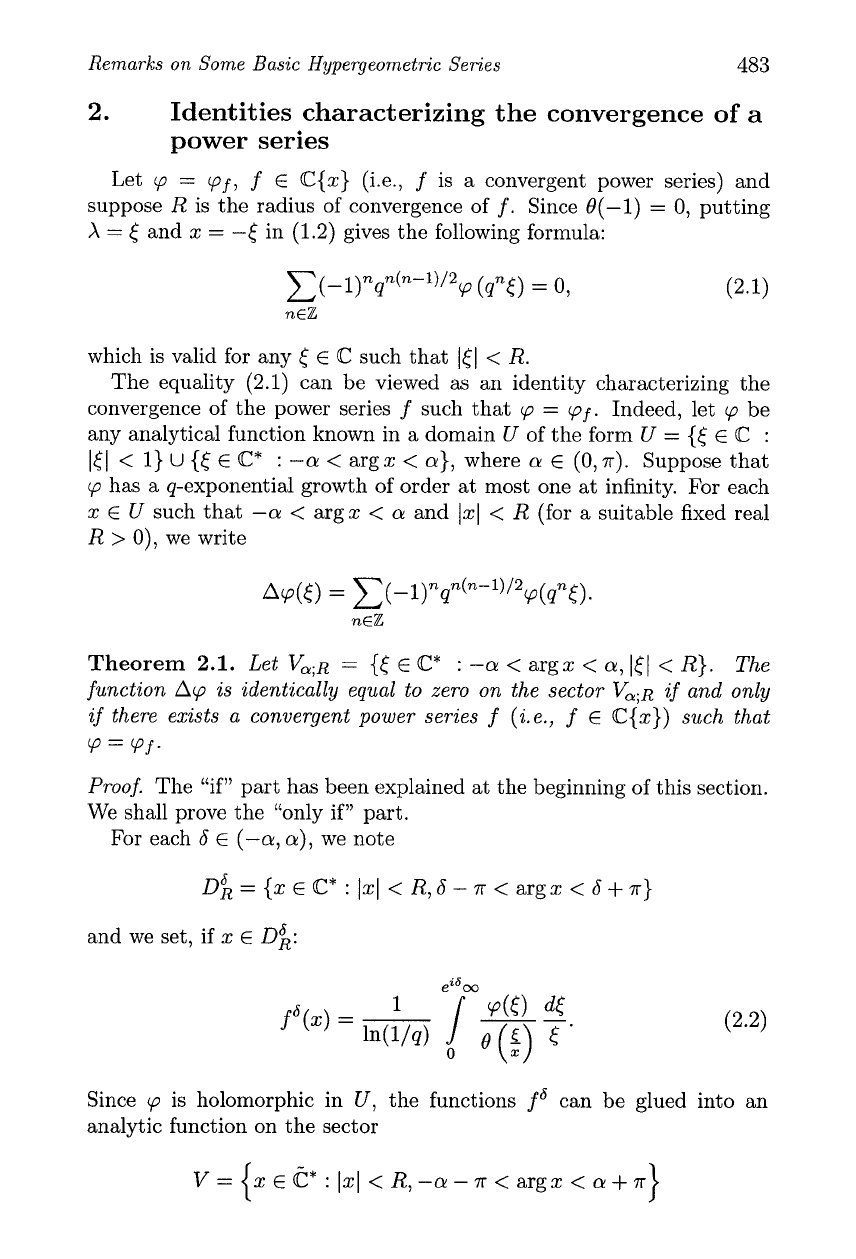
Theorem
2.1.
Let
Vff;~
=
(5
E
C*
:
-a
<
argx
<
a,
151
<
R).
The
function
Acp
is identically equal to zero on the sector
if
and only
if there exists a convergent power series f (i.e., f
E
C{x))
such that
cp
=
(Pf.
Proof.
The "if" part has been explained at the beginning of this section.
We shall prove the ('only if" part.
For each
S
E
(-a, a), we note
and we set, if
x
E
D;:
Since
cp
is holomorphic in U, the functions
fb
can be glued into an
analytic function on the sector

484
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
of the Riemann surface of the logarithm function. Let
f
be this function
just constructed on
V;
by the theorem of residues, we get:
for all
x
E
By assumption, Ap(J)
=
0 on hence, it follows
that
f
can be identified to an analytical function in the truncated disc
0
<
1x1
<
R.
We write again
f
for the latter function. By means of direct
estimations for (2.2), one can check that
f
is bounded in a neighborhood
of zero. Therefore, by Riemann's Removable Singularities Theorem the
function
f
is holomorphic at the origin, i.e.,
f
is the sum function of a
convergent power series in the disc
1x1
<
R.
It only remains to verify that the Taylor expansion of
p
at zero co-
incides with the power series pf. To do this, one can use the following
formula:
for more details, see (Zhang, 2000).
0
Now let's go back again to the formula (1.2) and let be given
X
E
C*,
p
E
(C*;
one gets:
(2.3)
Recall that for any analytic function
g
given in an open disc 0
<
1x1
<
r,
if
C
anxn
is its Laurent series expansion, then the formula (1.2) can be
nEZ
extended in the following way:
At the same time, the equality (2.3) takes the following form:
