Hibbeler R.C. Structural Analysis
Подождите немного. Документ загружается.


310 CHAPTER 8DEFLECTIONS
8
The cantilevered beam shown in Fig. 8–12a is subjected to a couple
moment at its end. Determine the equation of the elastic curve.
EI is constant.
M
0
EXAMPLE 8.4
SOLUTION
Elastic Curve. The load tends to deflect the beam as shown in
Fig. 8–9a. By inspection, the internal moment can be represented
throughout the beam using a single x coordinate.
Moment Function. From the free-body diagram, with M acting in
the positive direction, Fig. 8–12b, we have
Slope and Elastic Curve. Applying Eq. 8–4 and integrating twice
yields
(1)
(2)
(3)
Using the boundary conditions at and at
then Substituting these results into Eqs. (2) and
(3) with we get
Ans.v =
M
0
x
2
2EI
u =
M
0
x
EI
u = dv>dx,
C
1
= C
2
= 0.x = 0,
v = 0x = 0dv>dx = 0
EIv =
M
0
x
2
2
+ C
1
x + C
2
EI
dv
dx
= M
0
x + C
1
EI
d
2
v
dx
2
= M
0
M = M
0
L
x
A
M
0
(a)
x
M
M
0
(b)
Fig. 8–12

8.3 THE DOUBLE INTEGRATION METHOD 311
8
Maximum slope and displacement occur at , for which
(4)
(5)
The positive result for indicates counterclockwise rotation and the
positive result for indicates that is upward. This agrees with the
results sketched in Fig. 8–12a.
In order to obtain some idea as to the actual magnitude of the slope
and displacement at the end A, consider the beam in Fig. 8–12a to
have a length of 12 ft, support a couple moment of and be
made of steel having If this beam were designed
without a factor of safety by assuming the allowable normal stress is
equal to the yield stress then a would be
found to be adequate From Eqs. (4) and (5) we get
Since this justifies the use of Eq. 8–4, rather
than applying the more exact Eq. 8–3, for computing the deflection of
beams. Also, since this numerical application is for a cantilevered
beam, we have obtained larger values for maximum and than
would have been obtained if the beam were supported using pins,
rollers, or other supports.
vu
u
2
A
= 0.00297 rad
2
V
1,
v
A
=
15 k
#
ft112 in.>ft2112 ft2
2
112 in.>1 ft2
2
2129110
3
2 k>in
2
2116.4 in
4
2
= 3.92 in.
u
A
=
15 k
#
ft112 in.>ft2112 ft2112 in.>ft2
29110
3
2 k>in
2
116.4 in
4
2
= 0.0545 rad
1I = 16.4 in.
4
2.
W6 * 9s
allow
= 36 ksi,
E
st
= 29110
3
2 ksi.
15 k
#
ft,
v
A
v
A
u
A
v
A
=
M
0
L
2
2EI
u
A
=
M
0
L
EI
A 1x = L2

312 CHAPTER 8DEFLECTIONS
8
The beam in Fig. 8–13a is subjected to a load P at its end. Determine
the displacement at C. EI is constant.
EXAMPLE 8.5
SOLUTION
Elastic Curve. The beam deflects into the shape shown in
Fig. 8–13a. Due to the loading, two x coordinates must be considered.
Moment Functions. Using the free-body diagrams shown in
Fig. 8–13b, we have
Slope and Elastic Curve. Applying Eq. 8–4,
for x
1
,
(1)
(2)EIv
1
=-
P
12
x
3
1
+ C
1
x
1
+ C
2
EI
dv
1
dx
1
=-
P
4
x
2
1
+ C
1
EI
d
2
v
1
dx
2
1
=-
P
2
x
1
= Px
2
- 3Pa
2a … x
2
… 3a
M
2
=-
P
2
x
2
+
3P
2
1x
2
- 2a2
M
1
=-
P
2
x
1
0 … x
1
… 2a
x
2
A
P
C
B
2a
a
x
1
(a)
v
C
V
2
M
2
x
2
P
––
2
3P
___
2
2a
(b)
Fig. 8–13
x
1
P
__
2
V
1
M
1

8.3 THE DOUBLE INTEGRATION METHOD 313
8
For x
2
,
(3)
(4)
The four constants of integration are determined using three bound-
ary conditions, namely, at at and
at and one continuity equation. Here the continuity of slope at
the roller requires at (Note that
continuity of displacement at B has been indirectly considered in the
boundary conditions, since at ) Applying
these four conditions yields
Solving, we obtain
Substituting and into Eq. (4) gives
The displacement at C is determined by setting We get
Ans.v
C
=-
Pa
3
EI
x
2
= 3a.
v
2
=
P
6EI
x
3
2
-
3
2
Pa
EI
x
2
2
+
10Pa
2
3EI
x
2
-
2Pa
3
EI
C
4
C
3
C
1
=
Pa
2
3
C
2
= 0
C
3
=
10
3
Pa
2
C
4
=-2Pa
3
-
P
4
12a2
2
+ C
1
=
P
2
12a2
2
- 3Pa12a2+ C
3
dv
1
12a2
dx
1
=
dv
2
12a2
dx
2
;
0 =
P
6
12a2
3
-
3
2
Pa12a2
2
+ C
3
12a2+ C
4
v
2
= 0 at x
2
= 2a;
0 =-
P
12
12a2
3
+ C
1
12a2+ C
2
v
1
= 0 at x
1
= 2a;
0 = 0 + 0 + C
2
v
1
= 0 at x
1
= 0;
x
1
= x
2
= 2a.v
1
= v
2
= 0
x
1
= x
2
= 2a.dv
1
>dx
1
= dv
2
>dx
2
x
2
= 2a,
v
2
= 0x
1
= 2a,x
1
= 0, v
1
= 0v
1
= 0
EIv
2
=
P
6
x
3
2
-
3
2
Pax
2
2
+ C
3
x
2
+ C
4
EI
dv
2
dx
2
=
P
2
x
2
2
- 3Pax
2
+ C
3
EI
d
2
v
2
dx
2
2
= Px
2
- 3Pa

314 CHAPTER 8DEFLECTIONS
8
F8–4. Determine the equation of the elastic curve for the
beam using the x coordinate that is valid for EI
is constant.
0 6 x 6 L.
FUNDAMENTAL PROBLEMS
F8–7. Determine the equation of the elastic curve for the
beam using the x coordinate that is valid for EI
is constant.
0 6 x 6 L.
F8–5. Determine the equation of the elastic curve for the
beam using the x coordinate that is valid for EI
is constant.
0 6 x 6 L.
F8–8. Determine the equation of the elastic curve for the
beam using the x coordinate that is valid for EI
is constant.
0 6 x 6 L.
F8–6. Determine the equation of the elastic curve for the
beam using the x coordinate that is valid for EI
is constant.
0 6 x 6 L.
F8–9. Determine the equation of the elastic curve for the
beam using the x coordinate that is valid for EI
is constant.
0 6 x 6 L.
L
__
2
L
__
2
x
AB
P
L
__
2
L
__
2
x
AB
M
0
A
L
B
M
0
x
L
x
P
L
x
w
0
F8–4
F8–5
F8–6
F8–7
F8–8
F8–9
w
L
x

8.3 THE DOUBLE INTEGRATION METHOD 315
8
8–2. The bar is supported by a roller constraint at B, which
allows vertical displacement but resists axial load and moment.
If the bar is subjected to the loading shown, determine the
slope at A and the deflection at C. EI is constant.
8–3. Determine the deflection at B of the bar in Prob. 8–2.
8–7. Determine the elastic curve for the simply supported
beam using the x coordinate Also, determine
the slope at A and the maximum deflection of the beam.
EI is constant.
0 … x … L>2.
*8–4. Determine the equations of the elastic curve using the
coordinates and , specify the slope and deflection at B.
EI is constant.
8–5. Determine the equations of the elastic curve using the
coordinates and , and specify the slope and deflection
at point B. EI is constant.
x
3
x
1
x
2
x
1
*8–8. Determine the equations of the elastic curve using
the coordinates and , and specify the slope at C and
displacement at B. EI is constant.
8–9. Determine the equations of the elastic curve using
the coordinates and , and specify the slope at B and
deflection at C. EI is constant.
x
3
x
1
x
2
x
1
AB
PP
L
x
2
x
1
aa
w
A
B
LL
x
1
x
2
C
L
x
A
B
w
0
L
A
B
a
w
x
1
x
2
x
3
C
B
A
a
x
1
x
3
x
2
a
w
C
P
A
C
B
L
2
L
2
8–1. Determine the equations of the elastic curve for the
beam using the and coordinates. Specify the slope at
A and the maximum deflection. EI is constant.
x
2
x
1
8–6. Determine the maximum deflection between the
supports A and B. EI is constant. Use the method of
integration.
PROBLEMS
Prob. 8–1
Probs. 8–2/8–3
Probs. 8–4/8–5
Prob. 8–7
Prob. 8–6
Probs. 8–8/8–9

316 CHAPTER 8DEFLECTIONS
8
8.4 Moment-Area Theorems
The initial ideas for the two moment-area theorems were developed by
Otto Mohr and later stated formally by Charles E. Greene in 1873.These
theorems provide a semigraphical technique for determining the slope of
the elastic curve and its deflection due to bending. They are particularly
advantageous when used to solve problems involving beams, especially
those subjected to a series of concentrated loadings or having segments
with different moments of inertia.
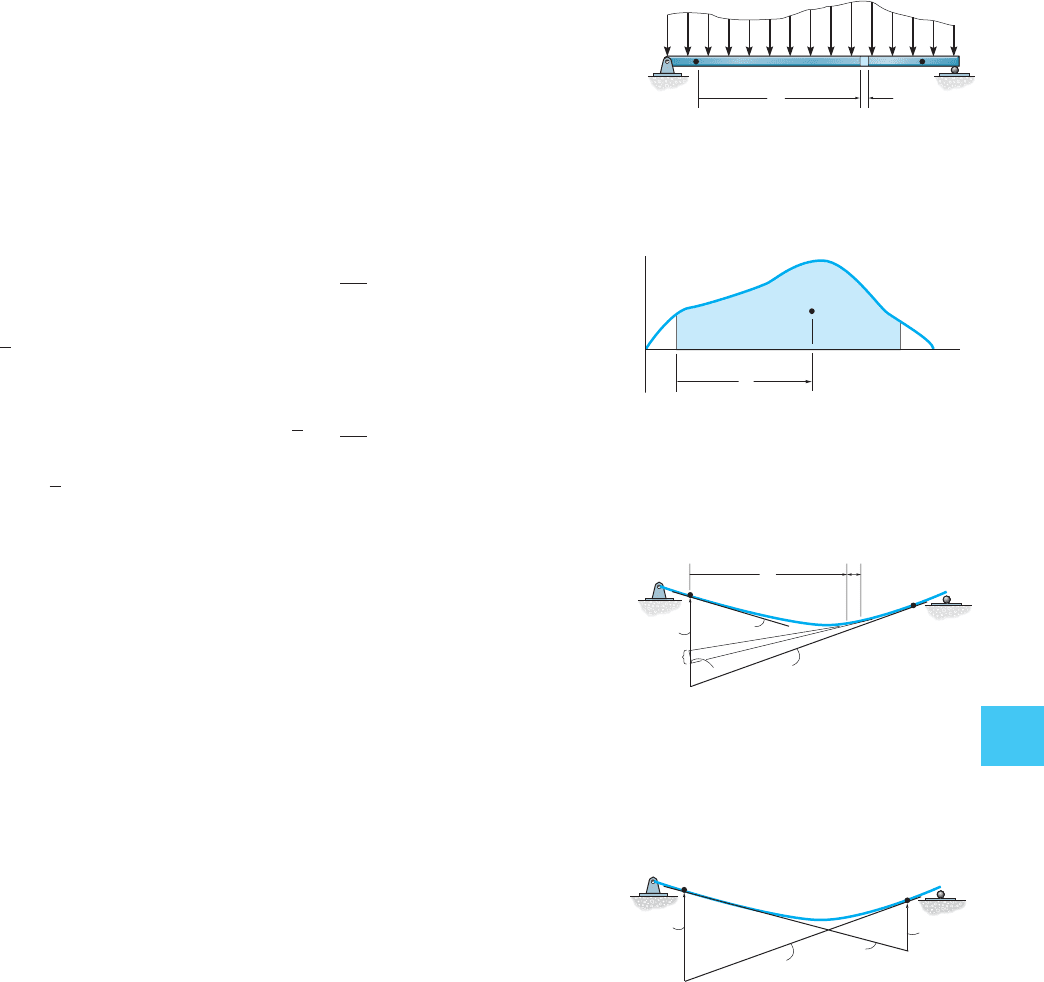
To develop the theorems, reference is made to the beam in Fig. 8–14a.
If we draw the moment diagram for the beam and then divide it by the
flexural rigidity, EI, the “M/EI diagram” shown in Fig. 8–14b results. By
Eq. 8–2,
Thus it can be seen that the change in the slope of the tangents on
either side of the element dx is equal to the lighter-shaded area under the
M/EI diagram. Integrating from point A on the elastic curve to point B,
Fig. 8–14c, we have
(8–5)
This equation forms the basis for the first moment-area theorem.
Theorem 1:The change in slope between any two points on the elastic
curve equals the area of the M/EI diagram between these two points.
The notation is referred to as the angle of the tangent at B
measured with respect to the tangent at A. From the proof it should be
evident that this angle is measured counterclockwise from tangent A to
tangent B if the area of the M/EI diagram is positive, Fig. 8–14c.
Conversely, if this area is negative, or below the x axis, the angle is
measured clockwise from tangent A to tangent B. Furthermore, from the
dimensions of Eq. 8–5, is measured in radians.u
B>A
u
B>A
u
B>A
u
B>A
=
L
B
A
M
EI
dx
du
du = a
M
EI
b dx
(
a
)
A B
w
x dx
M
___
EI
M
___
EI
A
B
x dx
(
b
)
x
A
B
elastic curve
tan B
tan A
u
B/A
(c)
Fig. 8–14
8.4 MOMENT-AREA THEOREMS 317
8
The second moment-area theorem is based on the relative deviation of
tangents to the elastic curve. Shown in Fig. 8–15c is a greatly exaggerated
view of the vertical deviation dt of the tangents on each side of the
differential element dx. This deviation is measured along a vertical line
passing through point A. Since the slope of the elastic curve and its
deflection are assumed to be very small, it is satisfactory to approximate
the length of each tangent line by x and the arc by dt. Using the
circular-arc formula where r is of length x, we can write
Using Eq. 8–2, the vertical deviation of the tangent at
A with respect to the tangent at B can be found by integration, in which
case
(8–6)
Recall from statics that the centroid of an area is determined from
Since represents an area of the M/EI dia-
gram, we can also write
(8–7)
Here is the distance from the vertical axis through A to the centroid of
the area between A and B, Fig. 8–15b.
The second moment-area theorem can now be stated as follows:
Theorem 2: The vertical deviation of the tangent at a point (A) on
the elastic curve with respect to the tangent extended from another
point (B) equals the “moment” of the area under the M/EI diagram
between the two points (A and B). This moment is computed about
point A (the point on the elastic curve), where the deviation is
to be determined.
Provided the moment of a positive M/EI area from A to B is
computed, as in Fig. 8–15b, it indicates that the tangent at point A is above
the tangent to the curve extended from point B, Fig. 8–15c. Similarly,
negative M/EI areas indicate that the tangent at A is below the tangent
extended from B. Note that in general is not equal to which is
shown in Fig. 8–15d. Specifically, the moment of the area under the M/EI
diagram between A and B is computed about point A to determine
F
ig. 8–15b, and it is computed about point B to determine
It is important to realize that the moment-area theorems can only be
used to determine the angles or deviations between two tangents on the
beam’s elastic curve. In general, they do not give a direct solution for the
slope or displacement at a point on the beam.These unknowns must first
be related to the angles or vertical deviations of tangents at points on
the elastic curve. Usually the tangents at the supports are drawn in this
regard since these points do not undergo displacement and/or have zero
slope. Specific cases for establishing these geometric relationships are
given in the example problems.
t
B>A
.
t
A>B
,
t
B>A
,t
A>B
t
A>B
x
t
A>B
= x
L
B
A
M
EI
dx
1
M>EI dxx
1
dA =
1
x dA.
t
A>B
=
L
B
A
x
M
EI
dx
du = 1M>EI2 dx,
dt = x du.s = ur,
ds¿
(
a
)
A
B
w
x dx
AB
_
x
(
b
)
x
M
___
EI
elastic curve
x
dx
A
B
tan A
ds¿
dt
tan B
(c)
t
A/B
elastic curve
A
B
tan A
t
A/B
t
B/A
tan B
(d)
Fig. 8–15

318 CHAPTER 8DEFLECTIONS
8
Procedure for Analysis
The following procedure provides a method that may be used to determine the
displacement and slope at a point on the elastic curve of a beam using the
moment-area theorems.
M
/
EI
Diagram
• Determine the support reactions and draw the beam’s M/EI diagram.
• If the beam is loaded with concentrated forces, the M/EI diagram will consist of a
series of straight line segments, and the areas and their moments required for the
moment-area theorems will be relatively easy to compute.
• If the loading consists of a series of concentrated forces and distributed loads, it may
be simpler to compute the required M/EI areas and their moments by drawing the
M/EI diagram in parts, using the method of superposition as discussed in Sec. 4–5.
In any case, the M/EI diagram will consist of parabolic or perhaps higher-order
curves, and it is suggested that the table on the inside back cover be used to locate
the area and centroid under each curve.
Elastic Curve
• Draw an exaggerated view of the beam’s elastic curve. Recall that points of zero
slope occur at fixed supports and zero displacement occurs at all fixed, pin, and
roller supports.
• If it becomes difficult to draw the general shape of the elastic curve, use the
moment (or M/EI) diagram. Realize that when the beam is subjected to a positive
moment the beam bends concave up, whereas negative moment bends the beam
concave down. Furthermore, an inflection point or change in curvature occurs
where the moment in the beam (or M/EI) is zero.
• The displacement and slope to be determined should be indicated on the curve.
Since the moment-area theorems apply only between two tangents, attention
should be given as to which tangents should be constructed so that the angles or
deviations between them will lead to the solution of the problem. In this regard,
the tangents at the points of unknown slope and displacement and at the supports
should be considered, since the beam usually has zero displacement and/or zero
slope at the supports.
Moment-Area Theorems
• Apply Theorem 1 to determine the angle between two tangents, and Theorem 2
to determine vertical deviations between these tangents.
• Realize that Theorem 2 in general will not yield the displacement of a point on the
elastic curve. When applied properly, it will only give the vertical distance or
deviation of a tangent at point A on the elastic curve from the tangent at B.
• After applying either Theorem 1 or Theorem 2, the algebraic sign of the answer
can be verified from the angle or deviation as indicated on the elastic curve.

8.4 MOMENT-AREA THEOREMS 319
8
15 ft 15 ft
M
––
EI
A
B
C
x
60
__
EI
30
__
EI
(b)
tan B
A
tan C
tan A
C
B
u
B/A
u
C/A
(c)
u
B
u
C
EXAMPLE 8.6
Determine the slope at points B and C of the beam shown in Fig. 8–16a.
Take and
SOLUTION
M
/
EI
Diagram. This diagram is shown in Fig. 8–16b. It is easier to
solve the problem in terms of EI and substitute the numerical data as
a last step.
Elastic Curve. The 2-k load causes the beam to deflect as shown in
Fig. 8–16c. (The beam is deflected concave down, since M/EI is
negative.) Here the tangent at A (the support) is always horizontal.
The tangents at B and C are also indicated.We are required to find
and . By the construction, the angle between tan A and tan B, that
is, is equivalent to
Also,
Moment-Area Theorem. Applying Theorem 1, is equal to the
area under the M/EI diagram between points A and B; that is,
Substituting numerical data for E and I, and converting feet to inches,
we have
Ans.
The negative sign indicates that the angle is measured clockwise from
A, Fig. 8–16c.
In a similar manner, the area under the M/EI diagram between
points A and C equals We have
Substituting numerical values for EI, we have
Ans. =-0.00745 rad
u
C
=
-900 k
#
ft
2
1144 in
2
>ft
2
2
29110
3
2 k>in
2
1600 in
4
2
u
C
= u
C>A
=
1
2
a-
60 k
#
ft
EI
b130 ft2=-
900 k
#
ft
2
EI
u
C>A
.
=-0.00559 rad
u
B
=
-675 k
#
ft
2
1144 in
2
>1 ft
2
2
29110
3
2 k>in
2
1600 in
4
2
=-
675 k
#
ft
2
EI
u
B
= u
B>A
=-a
30 k
#
ft
EI
b115 ft2-
1
2
a
60 k
#
ft
EI
-
30 k
#
ft
EI
b115 ft2
u
B>A
u
C
= u
C>A
u
B
= u
B>A
u
B
.u
B>A
,
u
C
u
B
I = 600 in
4
.E = 29110
3
2 ksi
Fig. 8–16
2 k
A
C
B
30 ft
15 ft
(a)
