Hibbeler R.C. Structural Analysis
Подождите немного. Документ загружается.

330 CHAPTER 8DEFLECTIONS
8
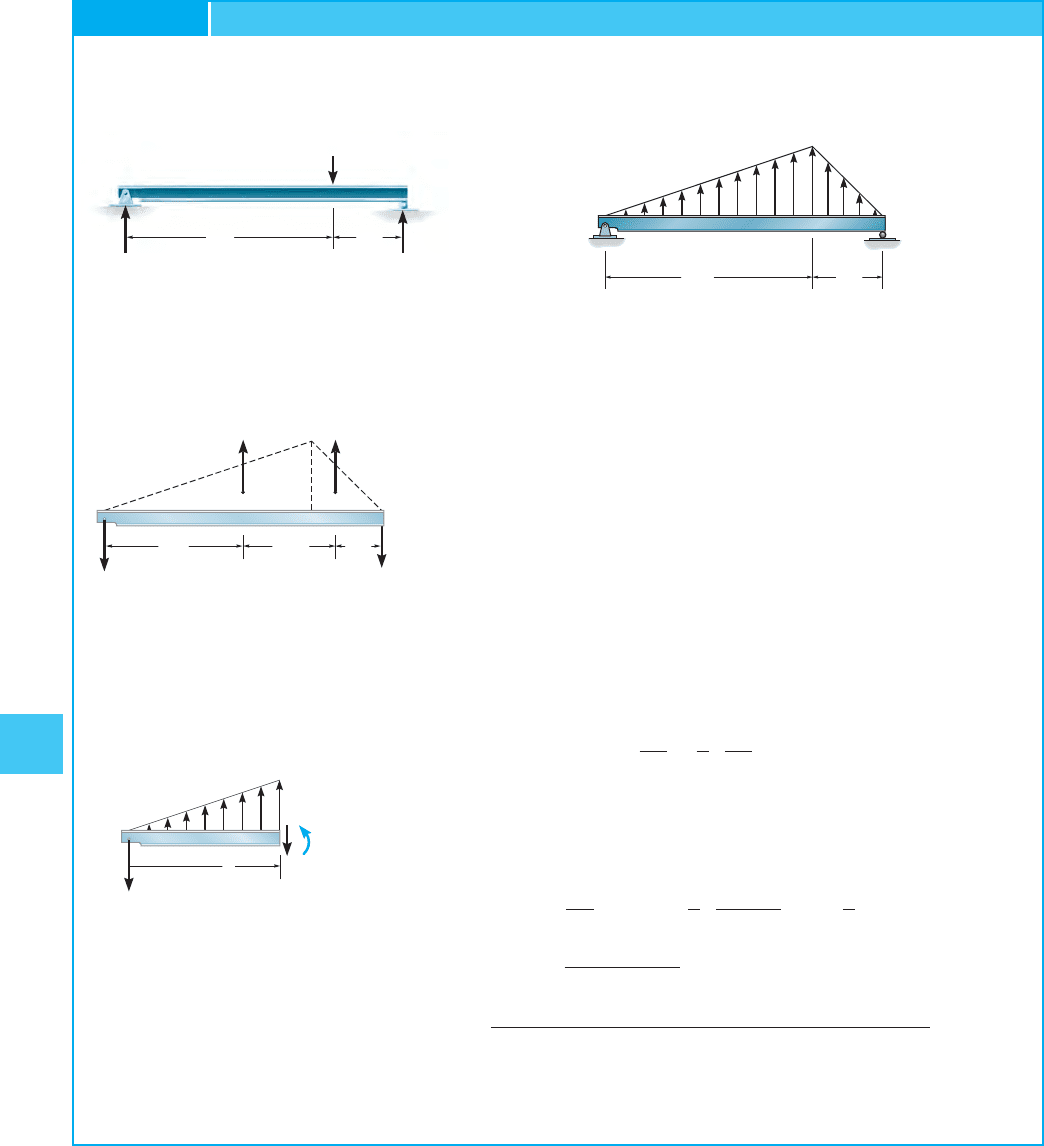
Determine the maximum deflection of the steel beam shown in
Fig. 8–26a. The reactions have been computed.
SOLUTION
Conjugate Beam. The conjugate beam loaded with the M/EI
diagram is shown in Fig. 8–26b. Since the M/EI diagram is positive, the
distributed load acts upward (away from the beam).
Equilibrium. The external reactions on the conjugate beam are
determined first and are indicated on the free-body diagram in
Fig. 8–26c. Maximum deflection of the real beam occurs at the point
where the slope of the beam is zero. This corresponds to the same
point in the conjugate beam where the shear is zero. Assuming this
point acts within the region from , we can isolate the
section shown in Fig. 8–26d. Note that the peak of the distributed
loading was determined from proportional triangles, that is,
We require so that
Using this value for x, the maximum deflection in the real beam corre-
sponds to the moment . Hence,
Ans.
The negative sign indicates the deflection is downward.
=-0.0168 m =-16.8 mm
=
-201.2 kN
#
m
3
[200110
6
2 kN>m
2
][60110
6
2 mm
4
11 m
4
>110
3
2
4
mm
4
2]
¢
max
= M¿=-
201.2 kN
#
m
3
EI
45
EI
16.712- c
1
2
a
216.712
EI
b6.71 d
1
3
16.712+ M¿=0d+©M = 0;
M¿
x = 6.71 m
10 … x … 9 m2 OK
-
45
EI
+
1
2
a
2x
EI
bx = 0+
c
©F
y
= 0;
V¿=0w>x = (18>EI)>9.
A¿0 … x … 9 m
I = 60(10
6
) mm
4
.
E = 200 GPa,
EXAMPLE 8.14
6 m
external reactions
(c)
81
__
EI
4 m 2 m
27
__
EI
45
__
EI
63
__
EI
18 x 2x
__
(
_
)
__
EI 9 EI
M¿
A
¿
x
internal reactions
(d)
45
__
EI
V¿ 0
9 m 3 m
8 kN
2 kN 6 kN
real beam
(a)
B
18
__
EI
9 m 3 m
conjugate beam
(b)
A¿
B¿
Fig. 8–26
12 18 24 36
120 120
144
M (k
ft)
x (ft)
moment diagram
(b)
8.5 CONJUGATE-BEAM METHOD 331
8
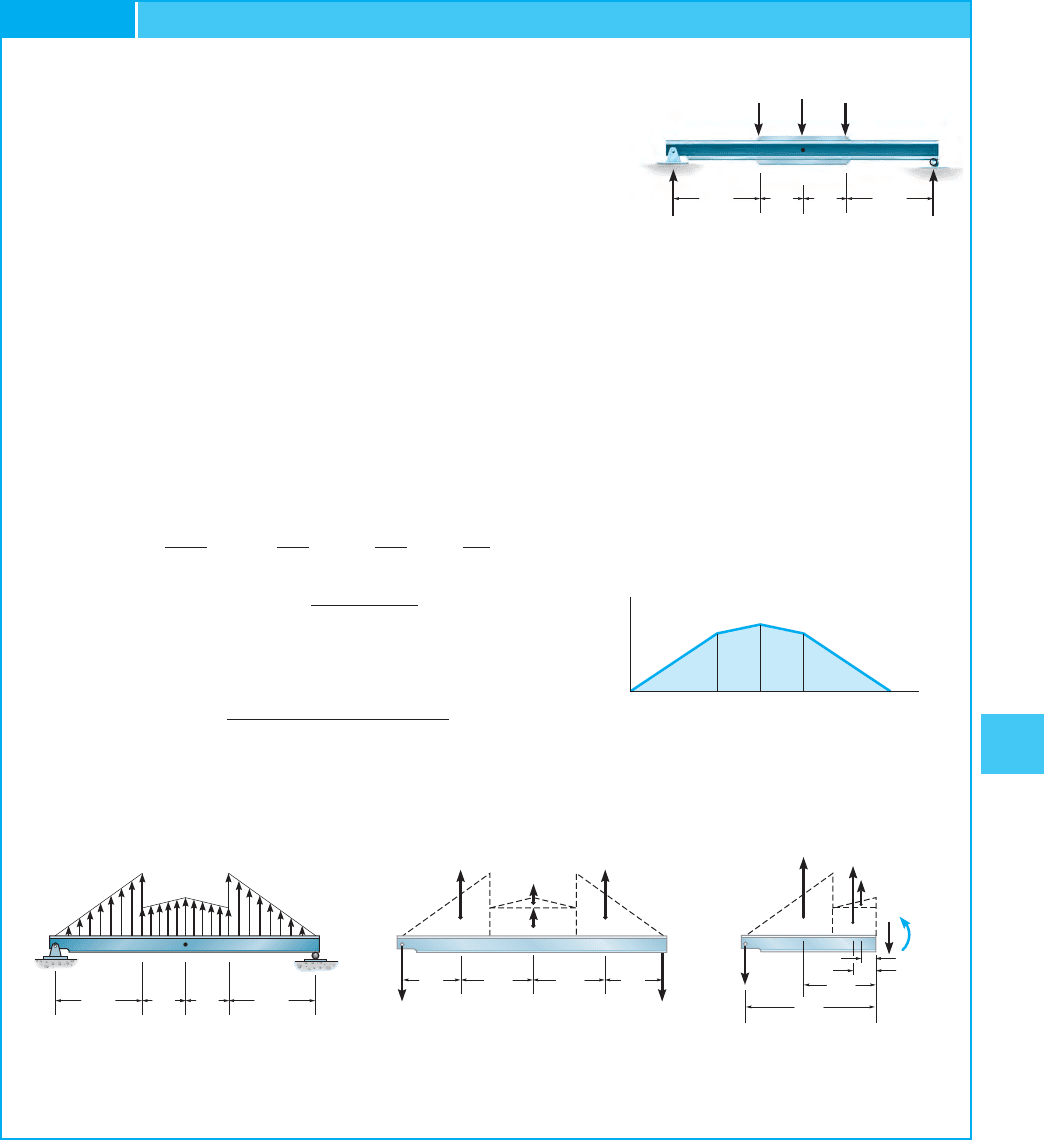
EXAMPLE 8.15
The girder in Fig. 8–27a is made from a continuous beam and reinforced
at its center with cover plates where its moment of inertia is larger.
The 12-ft end segments have a moment of inertia of and
the center portion has a moment of inertia of Determine
the deflection at the center C. Take The reactions
have been calculated.
SOLUTION
Conjugate Beam. The moment diagram for the beam is determined
first, Fig. 8–27b. Since for simplicity, we can express the load on
the conjugate beam in terms of the constant EI, as shown in Fig. 8–27c.
Equilibrium. The reactions on the conjugate beam can be calculated
by the symmetry of the loading or using the equations of equilibrium.
The results are shown in Fig. 8–27d. Since the deflection at C is to be
determined, we must compute the internal moment at . Using
the method of sections, segment is isolated and the resultants of
the distributed loads and their locations are determined, Fig. 8–27e.Thus,
Substituting the numerical data for EI and converting units, we have
Ans.
The negative sign indicates that the deflection is downward.
¢
C
= M
C¿
=-
11 736 k
#
ft
3
11728 in
3
>ft
3
2
29110
3
2 k>in
2
1450 in
4
2
=-1.55 in.
M
C¿
=-
11 736 k
#
ft
3
EI
1116
EI
1182-
720
EI
1102 -
360
EI
132 -
36
EI
122+ M
C¿
= 0d+©M
C¿
= 0;
A¿C¿
C¿
I¿=2I,
E = 29(10
3
) ksi.
I¿=900 in
4
.
I = 450 in
4
,
12 ft 12 ft6 ft 6 ft
6 k
8 k
6 k
10 k 10 k
I¿
= 900 in
4
I
= 450 in
4
I
= 450 in
4
A
B
CC
real beam
(a)
120
___
EI
60
___
EI
72
___
EI
60
___
EI
120
___
EI
12 ft 12 ft6 ft 6 ft
C¿
A¿ B¿
conjugate beam
(c)
8 ft
720
___
EI
72
___
EI
A¿ B¿
external reactions
(
d
)
8 ft10 ft10 ft
720
___
EI
720___
EI
1116
____
EI
1116
____
EI
18 ft
720
___
EI
36
___
EI
A¿
C¿
internal reactions
(e)
10 ft
1116
____
EI
360
___
EI
3 ft
2 ft
V
C¿
M
C¿
Fig. 8–27
332 CHAPTER 8DEFLECTIONS
8
Fig. 8–28
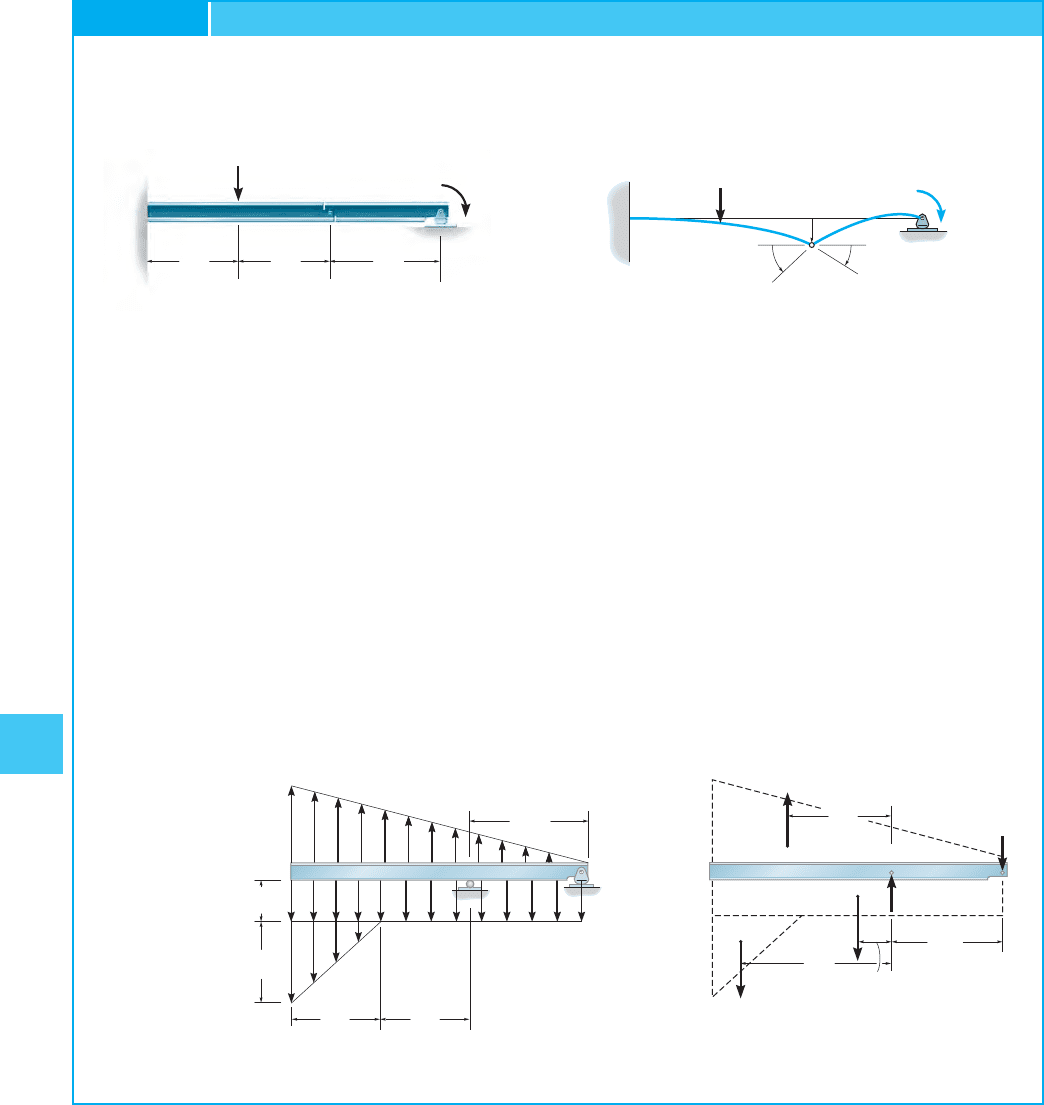
Determine the displacement of the pin at B and the slope of each
beam segment connected to the pin for the compound beam shown in
Fig. 8–28a.
SOLUTION
Conjugate Beam. The elastic curve for the beam is shown in
Fig. 8–28b in order to identify the unknown displacement and the
slopes and to the left and right of the pin. Using Table 8–2,
the conjugate beam is shown in Fig. 8–28c. For simplicity in calculation,
the M/EI diagram has been drawn in parts using the principle of
superposition as described in Sec. 4–5. In this regard, the real beam is
thought of as cantilevered from the left support, A. The moment
diagrams for the 8-k load, the reactive force and the
loading are given. Notice that negative regions of this diagram
develop a downward distributed load and positive regions have a
distributed load that acts upward.
30-k
#
ftC
y
= 2 k,
(u
B
)
R
(u
B
)
L
¢
B
E = 29(10
3
) ksi, I = 30 in
4
.
EXAMPLE 8.16
12 ft
8 k
real beam
(a)
A
B
12 ft 15 ft
C
30 kft
A
8 k
C
30 kft
elastic curve
(
b
)
B
B
(u
B
)
R
(u
B
)
L
78
–––
EI
15 ft
B¿
conjugate beam
(c)
12 ft 12 ft
A¿
C¿
30
–––
EI
96
–––
EI
B¿
external reactions
(d)
11 ft
576
___
EI
20 ft
228.6
_____
EI
1170
____
EI
15 ft
4.5 ft
3.6
___
EI
1521
____
EI
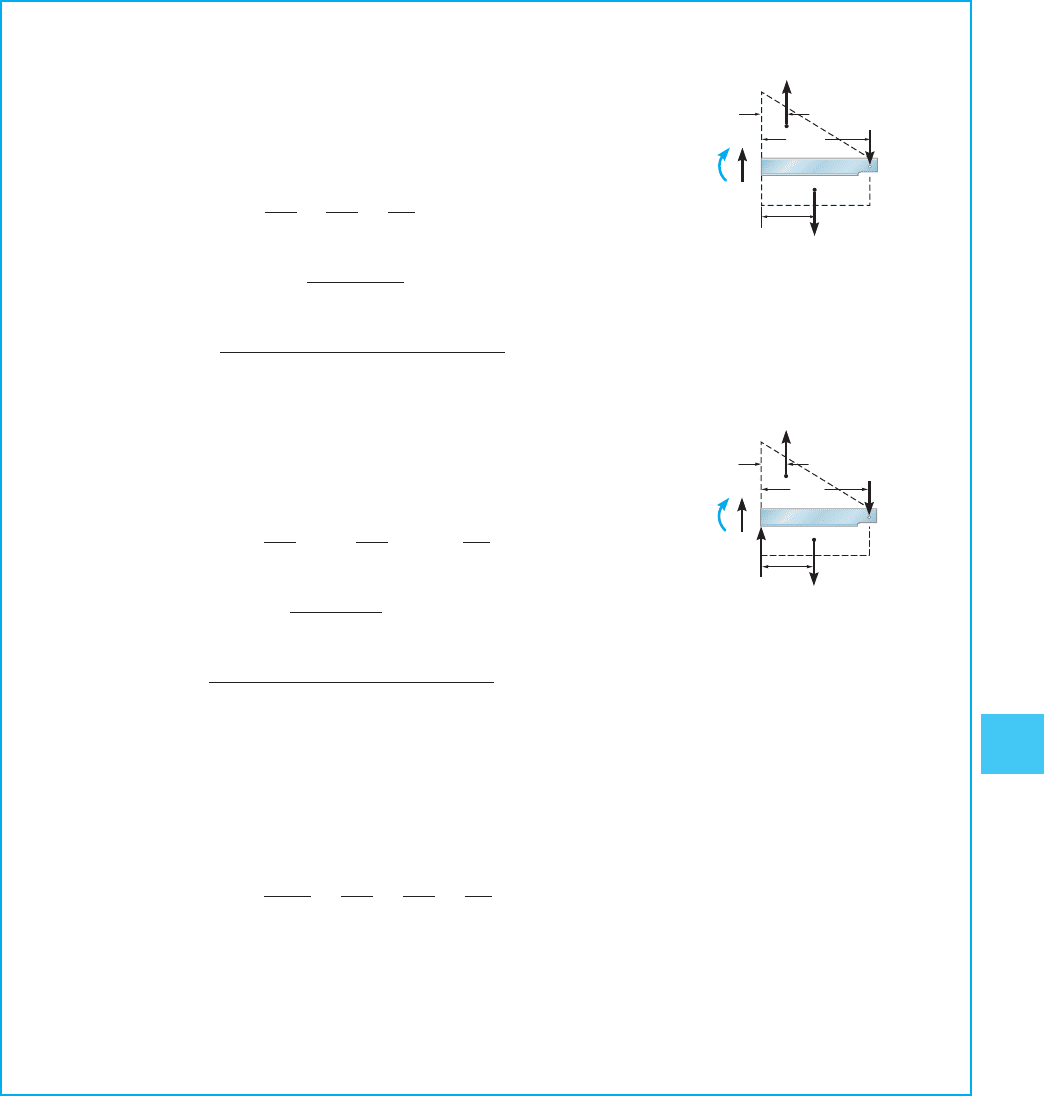
8.5 CONJUGATE-BEAM METHOD 333
8
(e)
M
B¿
(V
B¿
)
R
15 ft
7.5 ft
5 ft
3.6
___
EI
225
___
EI
450
___
EI
B¿
(
f
)
15 ft
7.5 ft
5 ft
225
___
EI
450
___
EI
M
B¿
(V
B¿
)
L
B¿
228.6
____
EI
3.6
___
EI
Equilibrium. The external reactions at and are calculated first
and the results are indicated in Fig. 8–28d. In order to determine
the conjugate beam is sectioned just to the right of and the
shear force is computed, Fig. 8–28e. Thus,
Ans.
The internal moment at yields the displacement of the pin. Thus,
Ans.
The slope can be found from a section of beam just to the left
of , Fig. 8–28f. Thus,
Ans.
Obviously, for this segment is the same as previously
calculated, since the moment arms are only slightly different in Figs. 8–28e
and 8–28f.
¢
B
= M
B¿
1u
B
2
L
= 1V
B¿
2
L
= 0
1V
B¿
2
L
+
228.6
EI
+
225
EI
-
450
EI
-
3.6
EI
= 0+
c
©F
y
= 0;
B¿
(u
B
)
L
=-0.381 ft =-4.58 in.
=
-2304 k
#
ft
3
[29110
3
211442 k>ft
2
][30>1122
4
] ft
4
¢
B
= M
B¿
=-
2304 k
#
ft
3
EI
-M
B¿
+
225
EI
152-
450
EI
17.52-
3.6
EI
1152= 0d+©M
B¿
= 0;
B¿
= 0.0378 rad
=
228.6 k
#
ft
2
[29110
3
211442 k>ft
2
][30>1122
4
] ft
4
1u
B
2
R
= 1V
B¿
2
R
=
228.6 k
#
ft
2
EI
1V
B¿
2
R
+
225
EI
-
450
EI
-
3.6
EI
= 0+
c
©F
y
= 0;
(V
B¿
)
R
B¿(u
B
)
R
,
C¿B¿
334 CHAPTER 8DEFLECTIONS
8
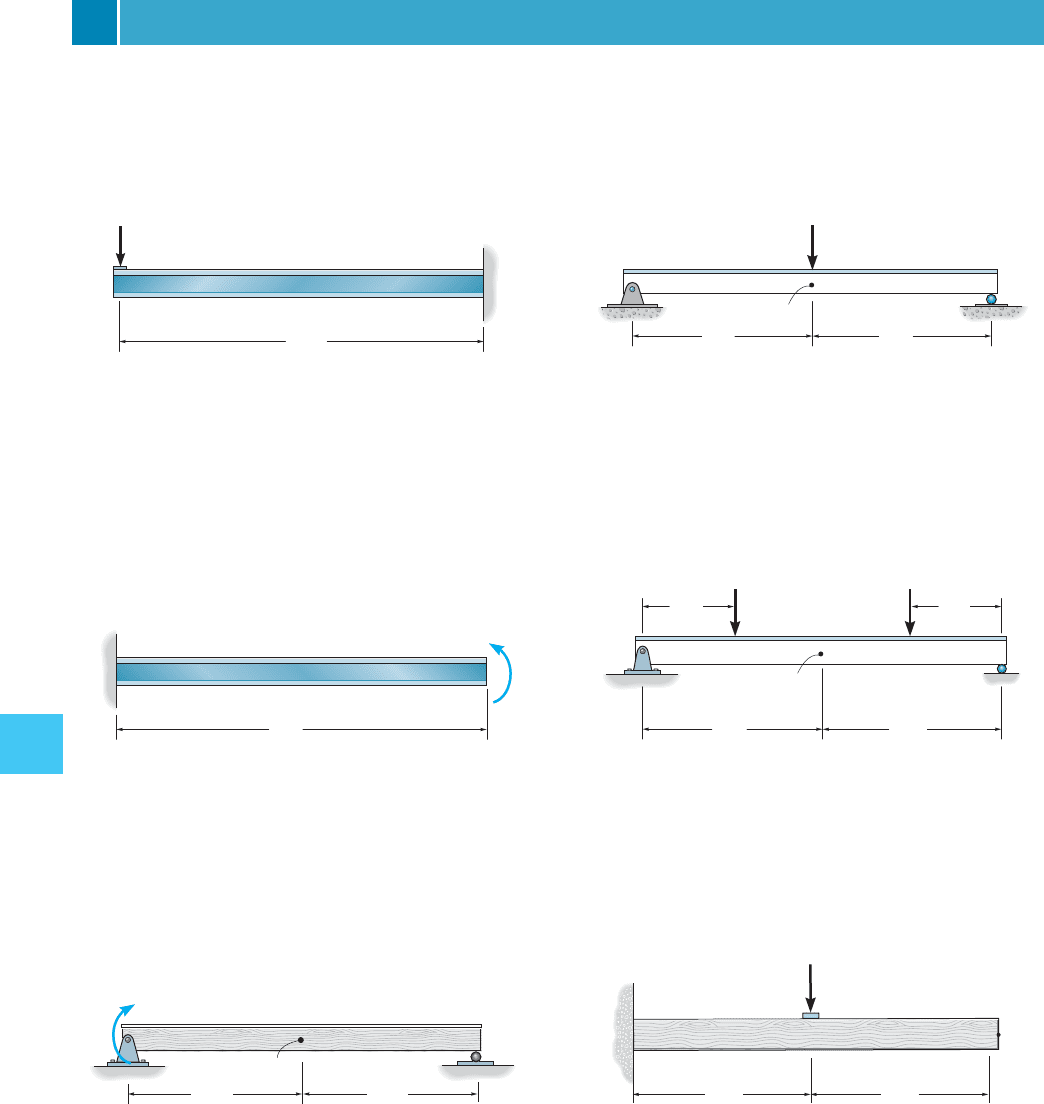
FUNDAMENTAL PROBLEMS
F8–10. Use the moment-area theorems and determine the
slope at A and deflection at A. EI is constant.
F8–11. Solve Prob. F8–10 using the conjugate beam
method.
F8–16. Use the moment-area theorems and determine the
slope at A and displacement at C. EI is constant.
F8–17. Solve Prob. F8–16 using the conjugate beam
method.
F8–12. Use the moment-area theorems and determine the
slope at B and deflection at B. EI is constant.
F8–13. Solve Prob. F8–12 using the conjugate beam
method.
F8–18. Use the moment-area theorems and determine the
slope at A and displacement at C. EI is constant.
F8–19. Solve Prob. F8–18 using the conjugate beam
method.
F8–14. Use the moment-area theorems and determine the
slope at A and displacement at C. EI is constant.
F8–15. Solve Prob. F8–14 using the conjugate beam
method.
F8–20. Use the moment-area theorems and determine the
slope at B and displacement at B. EI is constant.
F8–21. Solve Prob. F8–20 using the conjugate beam
method.
3 m
A
B
6 kN
F8–10/8–11
A
4 m
B
8 kNm
F8–12/8–13
A
C
B
5 kNm
1.5 m
1.5 m
F8–14/8–15
A
8 kN
C
3 m 3 m
B
F8–16/8–17
A
4 kN
4 kN
2 m 2 m
4 m 4 m
B
C
F8–18/8–19
A
2 m
2 m
9 kN
B
F8–20/8–21
8.5 CONJUGATE-BEAM METHOD 335
8
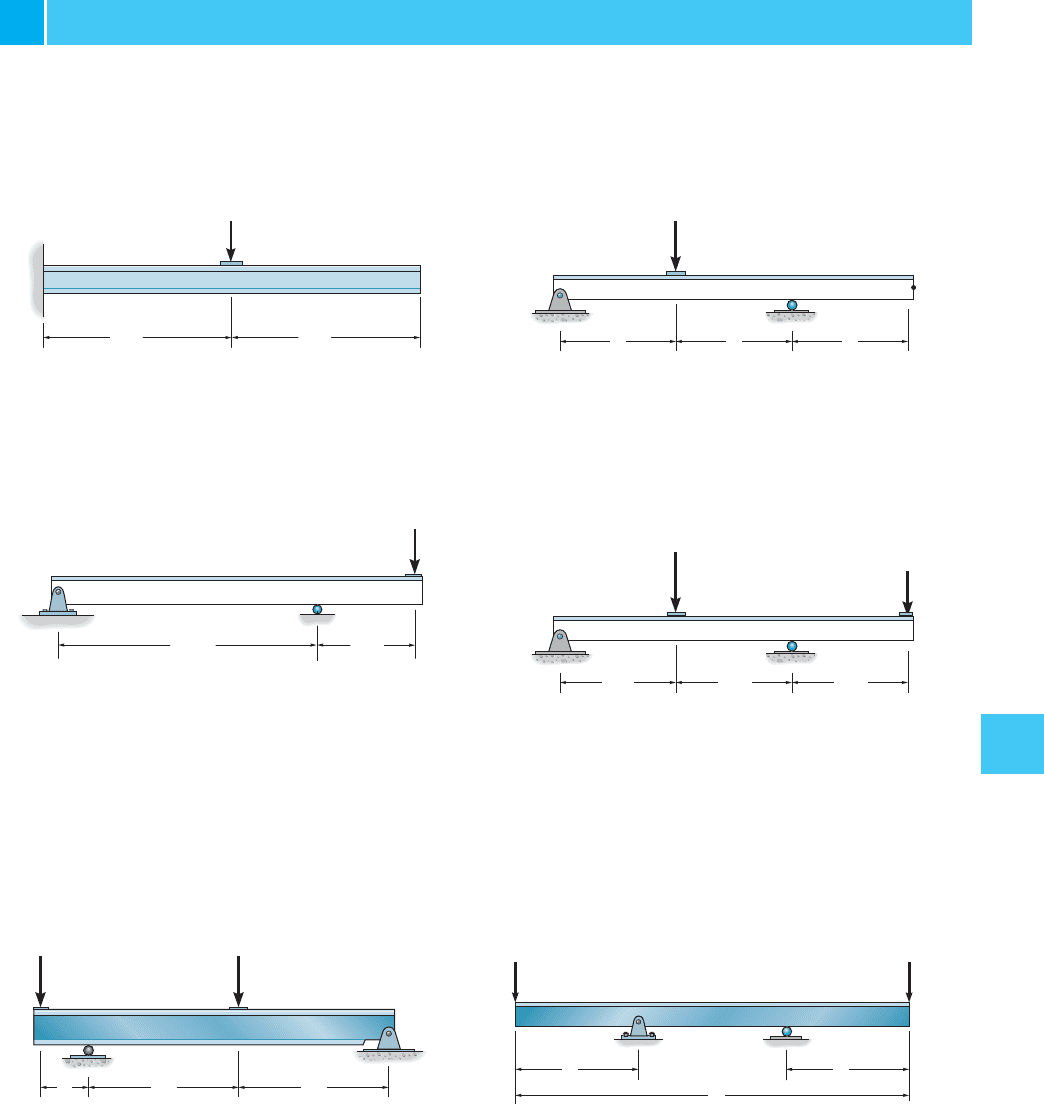
*8–12. Determine the slope and displacement at C. EI is
constant. Use the moment-area theorems.
8–13. Solve Prob. 8–12 using the conjugate-beam method.
8–10. Determine the slope at B and the maximum
displacement of the beam. Use the moment-area theorems.
Take
8–11. Solve Prob. 8–10 using the conjugate-beam method.
E = 29(10
3
) ksi, I = 500 in
4
.
8–14. Determine the value of a so that the slope at A is
equal to zero. EI is constant. Use the moment-area theorems.
8–15. Solve Prob. 8–14 using the conjugate-beam method.
*8–16. Determine the value of a so that the displacement
at C is equal to zero. EI is constant. Use the moment-area
theorems.
8–17. Solve Prob. 8–16 using the conjugate-beam method.
PROBLEMS
6 ft 6 ft
A
B
C
15 k
Probs. 8–10/8–11
A
B
C
15 ft
15 k
30 ft
Probs. 8–12/8–13
A
D
P
B
C
P
a
L
__
2
L
__
2
Probs. 8–14/8–15/8–16/8–17
a a a
B
A
C
P
Probs. 8–18/8–19
8–18. Determine the slope and the displacement at C. EI
is constant. Use the moment-area theorems.
8–19. Solve Prob. 8–18 using the conjugate-beam method.
*8–20. Determine the slope and the displacement at the
end C of the beam. Use
the moment-area theorems.
8–21. Solve Prob. 8–20 using the conjugate-beam method.
E = 200 GPa,
I = 70(10
6
) mm
4
.
B
D
A
C
3 m 3 m
8 kN
4 kN
3 m
Probs. 8–20/8–21
8–22. At what distance a should the bearing supports at A
and B be placed so that the displacement at the center of
the shaft is equal to the deflection at its ends? The bearings
exert only vertical reactions on the shaft. EI is constant. Use
the moment-area theorems.
8–23. Solve Prob. 8–22 using the conjugate-beam method.
AB
a
L
PP
a
Probs. 8–22/8–23
336 CHAPTER 8DEFLECTIONS
8
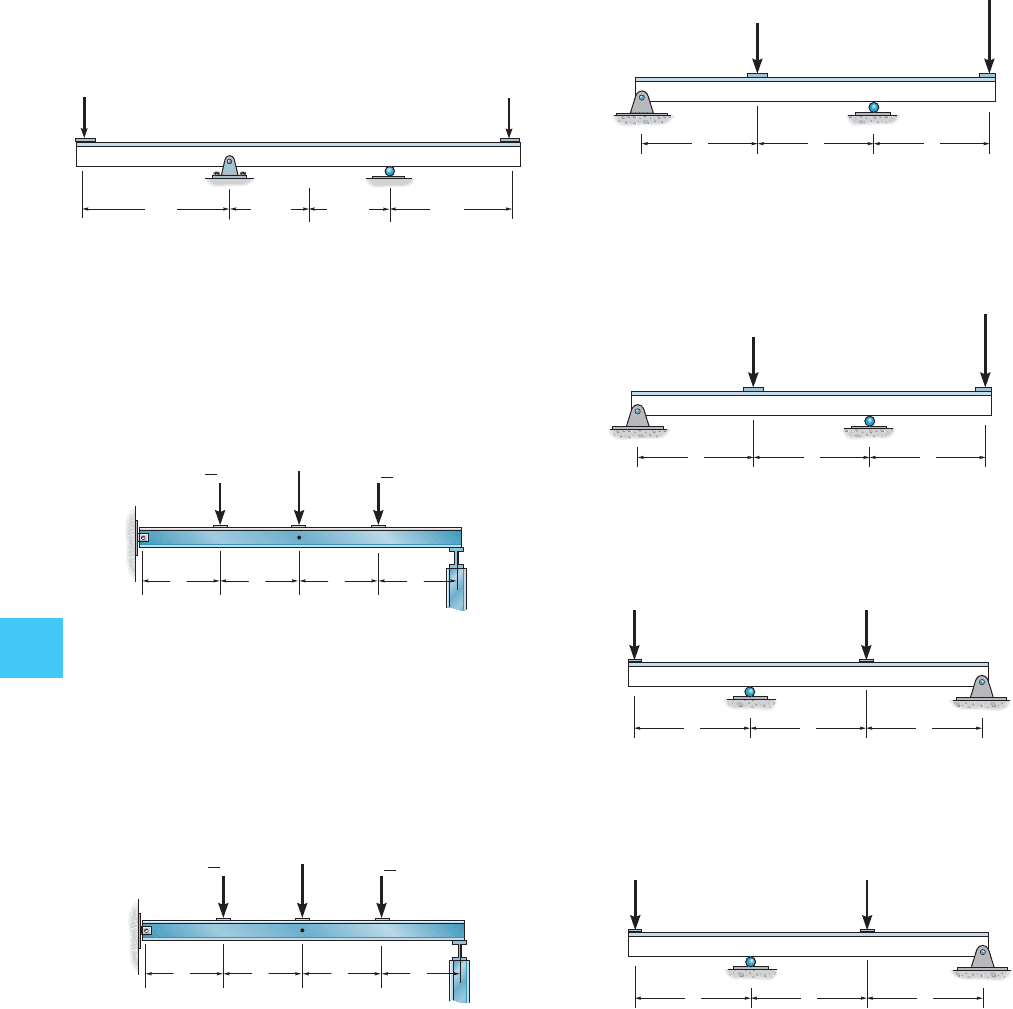
8–29. Determine the force F at the end of the beam C so
that the displacement at C is zero. EI is constant. Use the
conjugate-beam method.
8–26. Determine the displacement at C and the slope at B.
EI is constant. Use the moment-area theorems.
*8–28. Determine the force F at the end of the beam C so
that the displacement at C is zero. EI is constant. Use the
moment-area theorems.
*8–24. Determine the displacement at C and the slope
at B. EI is constant. Use the moment-area theorems.
8–25. Solve Prob. 8–24 using the conjugate-beam
method.
A
B
C
3 m
1.5 m 1.5 m
4 kN
4 kN
3 m
Probs. 8–24/8–25
A
C
B
P
P
a a a a
2
P
2
Prob. 8–26
A
C
B
P
P
a a a a
2
P
2
Prob. 8–27
a a a
B
D
A
C
P
F
Prob. 8–28
a a a
B
D
A
C
P
F
Prob. 8–29
a a a
A
C
P
P
B
Prob. 8–30
8–30. Determine the slope at B and the displacement at C.
EI is constant. Use the moment-area theorems.
a a a
A
C
P
P
B
Prob. 8–31
8–31. Determine the slope at B and the displacement at C.
EI is constant. Use the conjugate-beam method.
8–27. Determine the displacement at C and the slope at B.
EI is constant. Use the conjugate-beam method.
8.5 CONJUGATE-BEAM METHOD 337
8
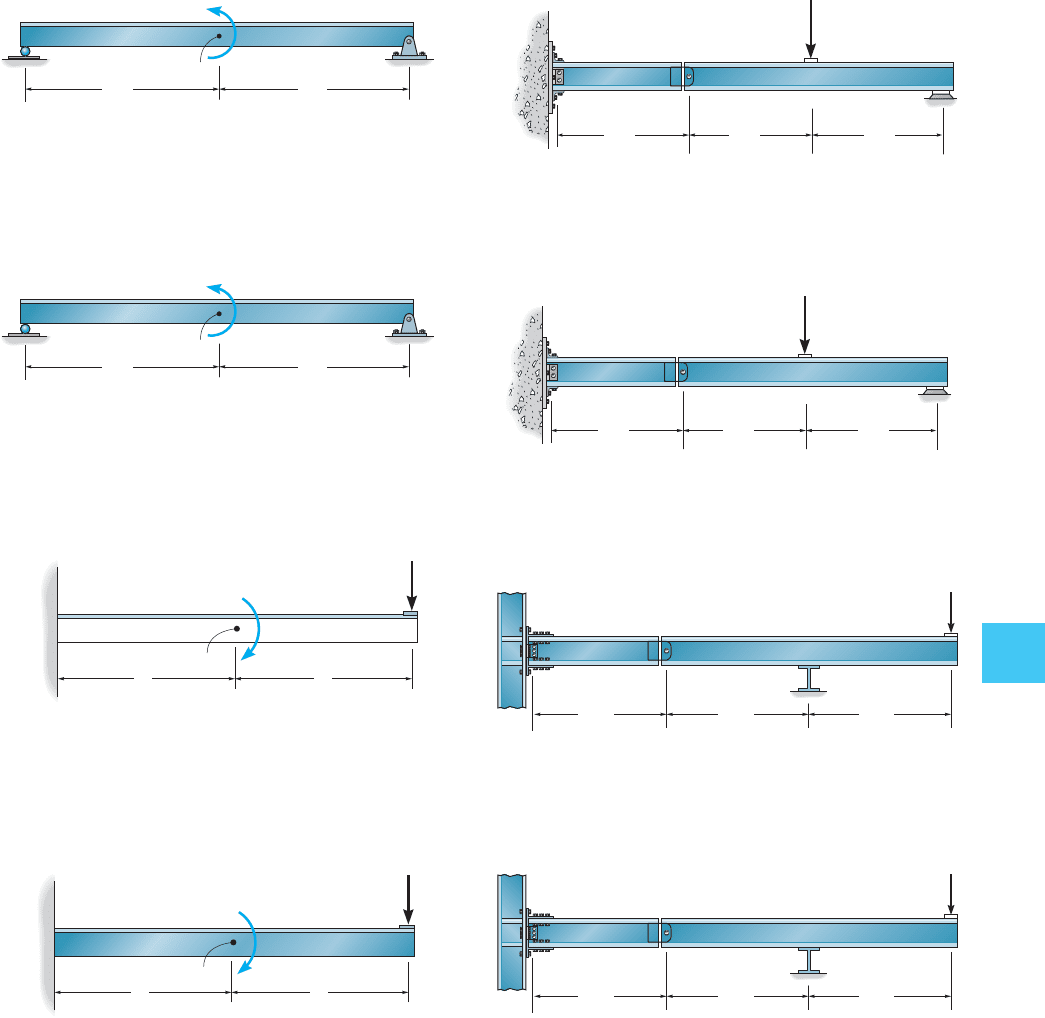
*8–32. Determine the maximum displacement and the slope
at A. EI is constant. Use the moment-area theorems.
8–38. Determine the displacement at D and the slope at
D. Assume A is a fixed support, B is a pin, and C is a roller.
Use the moment-area theorems.
A
M
0
C
B
L
__
2
L
__
2
Prob. 8–32
A
M
0
C
B
L
__
2
L
__
2
Prob. 8–33
aa
A
C
P
B
M
0
Pa
Prob. 8–35
aa
A
C
P
B
M
0
Pa
Prob. 8–34
3 m
3 m
25 kN
3 m
A
B
D
C
Prob. 8–36
3 m
3 m
25 kN
3 m
A
B
D
C
Prob. 8–37
12 ft 12 ft 12 ft
A
BC
D
6 k
Prob. 8–38
12 ft 12 ft 12 ft
A
BC
D
6 k
Prob. 8–39
8–35. Determine the slope and displacement at C. EI is
constant. Use the conjugate-beam method.
8–39. Determine the displacement at D and the slope at
D. Assume A is a fixed support, B is a pin, and C is a roller.
Use the conjugate-beam method.
*8–36. Determine the displacement at C. Assume A is a
fixed support, B is a pin, and D is a roller. EI is constant.
Use the moment-area theorems.
8–37. Determine the displacement at C. Assume A is a
fixed support, B is a pin, and D is a roller. EI is constant.
Use the conjugate-beam method.
8–33. Determine the maximum displacement at B and the
slope at A. EI is constant. Use the conjugate-beam method.
8–34. Determine the slope and displacement at C. EI is
constant. Use the moment-area theorems.
338 CHAPTER 8DEFLECTIONS
8
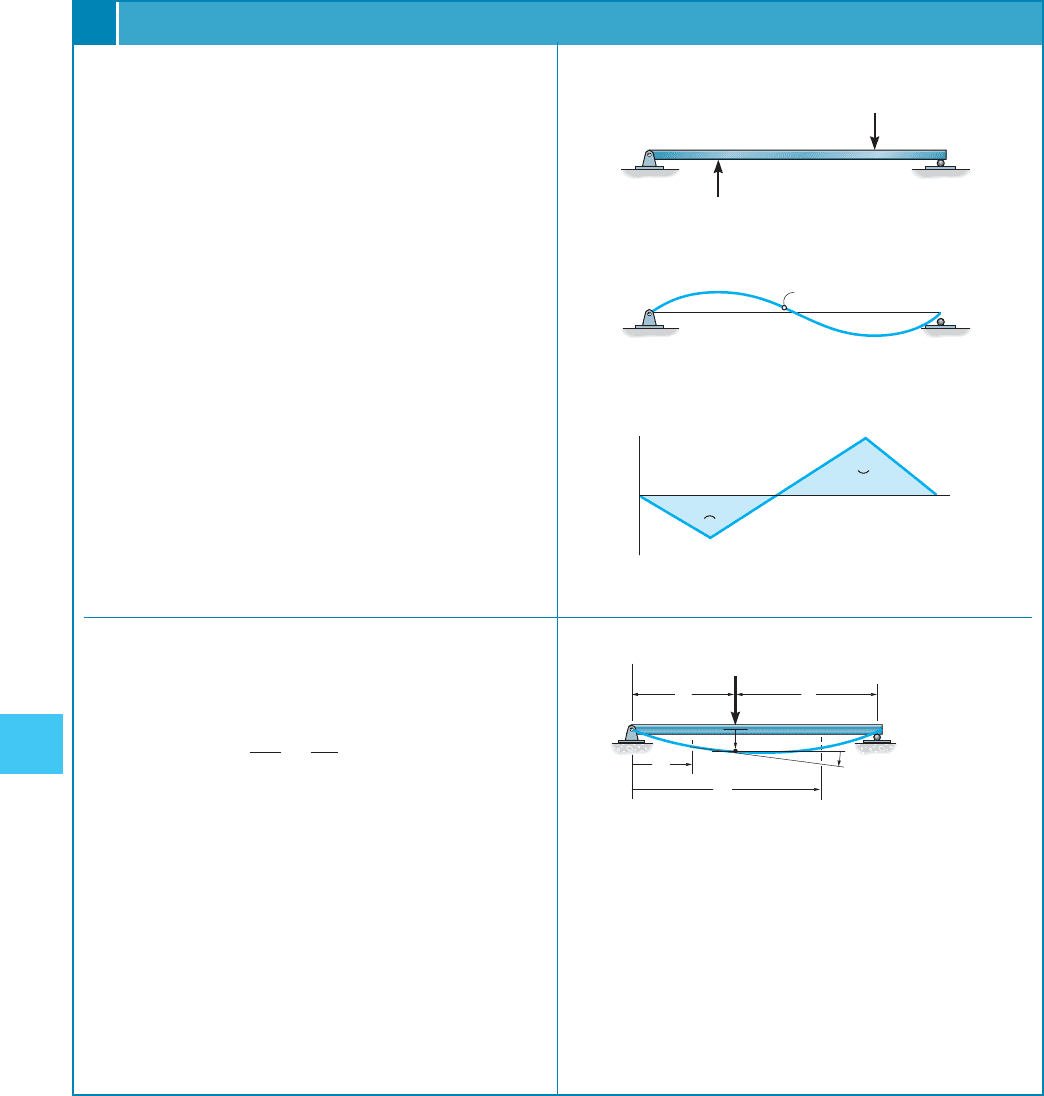
The deflection of a member (or structure) can always
be established provided the moment diagram is
known, because positive moment will tend to bend the
member concave upwards, and negative moment
will tend to bend the member concave downwards.
Likewise, the general shape of the moment diagram
can be determined if the deflection curve is known.
CHAPTER REVIEW
Deflection of a beam due to bending can be
determined by using double integration of the
equation.
Here the internal moment M must be expressed as a
function of the x coordinates that extend across the
beam. The constants of integration are obtained from
the boundary conditions, such as zero deflection at a
pin or roller support and zero deflection and slope at a
fixed support. If several x coordinates are necessary,
then the continuity of slope and deflection must be
considered, where at and
.v
1
(a) = v
2
(a)
u
1
(a) = u
2
(a)x
1
= x
2
= a,
d
2
v
dx
2
=
M
EI
beam
P
1
P
2
P
x
1
x
2
v
1
,v
2
a b
v
u
M
x
moment diagram
inflection point
deflection curve
CHAPTER REVIEW 339
8
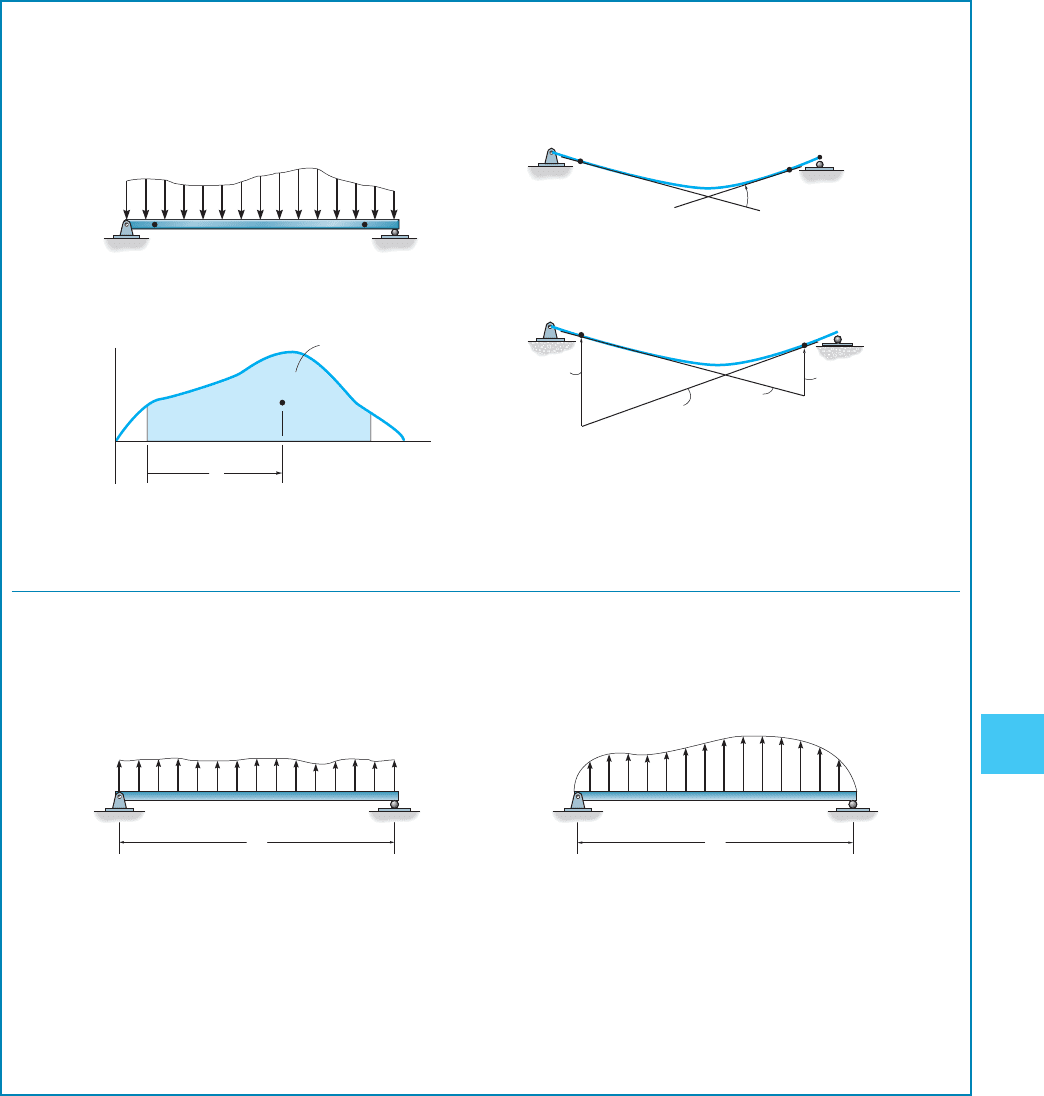
If the moment diagram has a simple shape, the moment-area theorems or the conjugate beam method can be used to
determine the deflection and slope at a point on the beam.
The moment-area theorems consider the angles and vertical deviation between the tangents at two points A and B
on the elastic curve. The change in slope is found from the area under the M/EI diagram between the two points, and the
deviation is determined from the moment of the M/EI diagram area about the point where the deviation occurs.
The conjugate beam method is very methodical and requires application of the principles of statics. Quite simply, one
establishes the conjugate beam using Table 8–2, then considers the loading as the M/EI diagram. The slope (deflection) at
a point on the real beam is then equal to the shear (moment) at the same point on the conjugate beam.
A B
w
A
B
u
B/A
Area of M/EI diagram
tan B
tan A
u
B/A
AB
_
x
Area
x
M
___
EI
A
B
tan A
t
A/B
t
B/A
tan B
t
A/B
(Area of M/EI diagram)
_
x
L
A
B
w
real beam
L
A¿
B¿
conjugate beam
M
___
EI
