Gottstein G., Shvindlerman L.S. Grain Boundary Migration in Metals: Thermodynamics, Kinetics, Applications

Подождите немного. Документ загружается.

52 1 Thermodynamics of Grain Boundaries

bulk phases, is true for any surface which is in equilibrium with the volumes

subdivided by it. At constant T and V , Eq. (1.50) can be transformed into

the relation (1.53):

= −



∂γ

∂μ



T,V,S

(1.169)

The Gibbs equation in the form of Eq. (1.169) and in its simplest form (Eq.

(1.60)) may be used to determine the grain boundary adsorption (segrega-

tion). It was implemented for the grain boundary segregation by Hondros and

Seah [36, 62, 63]: P in γ-Fe, Sn, Si and S in δ-iron. Even for a binary solution

this is not a very easy measurement. But actually the crucial point is not the

diﬃculty of experiment and calculation, but rather to understand which law

governs grain boundary segregation.

The Langmuir-Zhuchovitskii-McLean isotherm (Eqs. (1.152),(1.153)) [23]

was historically the ﬁrst isotherm of surface adsorption which was applied to

explain the concentration dependence of grain boundary segregation. Exam-

ples of systems described successfully by this isotherm are: P in α-Fe [64], In

in Ni [65].

The isotherm of multilayer adsorption (Eqs. (1.165)–(1.167)) was also used

to describe experimental data [36, 66]. This isotherm was ﬁrst put forward by

Brunauer, Emmet and Teller (BET) [67] to explain the gas adsorption at a

free surface in a close vicinity of the condensation point: C → C

;Γ→∞.

The “inﬁnite” adsorption in this case physically means condensation, i.e. the

formation of a liquid ﬁlm. Similarly, an “inﬁnite” segregation at grain bound-

aries indicates the formation of a new phase or at least the pre-precipitate

stage. The grain boundary segregation of tin, sulfur, phosphorus and anti-

mony in iron and nickel [36, 63, 66] was explained on a basis of a multilayer

grain boundary segregation.

An important feature of the adsorption isotherms, mentioned above, is that

the physical act of adsorption is independent of the degree of ﬁlling of the

adsorbed layer (of the number of atoms, which have been adsorbed before); in

other words, these isotherms do not take into account the interaction between

the adsorbed atoms. This type of isotherm was considered above (Eqs. (1.128)–

(1.133)). One of the “isotherms with interaction,” the Fowler-Guggenheim

isotherm (Eq. (1.131)), was repeatedly applied to diﬀerent systems to explain

the peculiarities of adsorption (segregation) behavior (selenium and tellurium

in α-iron [66], Bi in Cu [68], and antimony in α-iron [69]).

1.3.2 Grain Boundary Surface Tension

Unfortunately, direct experimental investigation of boundary adsorption is

only possible in a very few systems. In this case the only way to calculate the

equilibrium grain boundary segregation Γ

is to use the Gibbs equation (1.57)

1.3 Experiments 53

θθ

(c)

(d)

(f) (g)

(a)

ϕϕ

(b)

(e)

θθ

(c)

(d)

(f) (g)

(a)

ϕϕ

(b)

ϕϕ

(b)

(e)

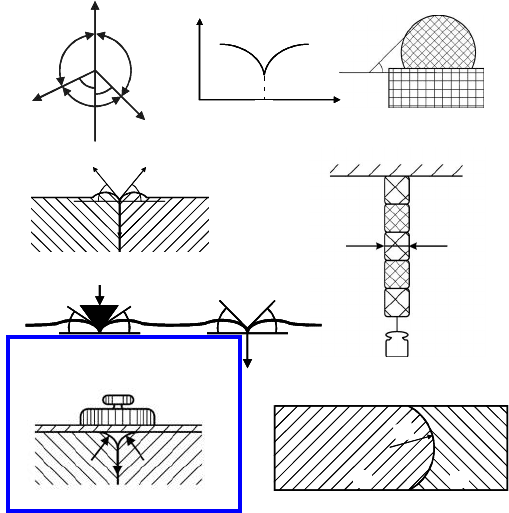
FIGURE 1.15

Methods of grain boundary surface tension measurement: (a) equilibrium an-

gles at triple function; (b) rotating ball method: sintering of small signal crys-

tal balls to single crystal substrate; (c) thermal groove method; (d) zero-creep

method; (e) method of a “ﬂoating” wedge; (f) hypothetical method of an

“equilibrium” grain boundary thermal groove; (g) balance of grain boundary

surface tension and volume diving force.

and to relate it to macroscopic parameters such as the grain boundary surface

tension γ and the chemical potentials μ

of the components in the grain. In

this case the surface tension of the grain boundary should be measured for a

number of alloys and preferably at diﬀerent temperatures. Let us consider the

— unfortunately few — experimental methods, which give us an opportunity

to measure the grain boundary surface tension. A brief summary of diﬀerent

schemes of the measurement of grain boundary surface tension is shown in

Fig. 1.15 [70].

The ﬁrst method can be called the triple junction method [71, 72] (Fig.

1.15a). If there is a mechanical equilibrium at the triple junction and the grain

boundary surface tensions do not depend on the orientation of the boundaries,

the relation between the surface tension of diﬀerent boundaries can be found

54 1 Thermodynamics of Grain Boundaries

from Young’s theorem:

sin α

(1.170)

where α

indicates the contact angles opposite to γ

. If the surface tensions

of the grain boundaries depend on their orientation, the dependency ∂γ

/∂Θ

should be taken into account where Θ is the inclination of the boundary with

regard to a reference plane [73]. These terms are associated with the forces

which tend to rotate the boundary into the position with minimum energy;

according to Herring [73]

(1 + ε

− ε

)sinα

+(ε

− ε

)cosα

(1 + ε

− ε

)sinα

+(ε

− ε

)cosα

= (1.171)

(1 + ε

− ε

)sinα

+(ε

− ε

)cosα

where ε

∂ lnγ

∂Θ

denotes the dependence of the grain boundary surface ten-

sion on grain boundary orientation. These ε

are referred to as torque terms.

The inﬂuence of the torque terms is important in the vicinity of special mis-

orientations (see Chapter 2). For random grain boundaries and far from special

misorientations the values of (∂γ

/∂Θ

) are small enough and Eq. (1.170) gives

a reasonable approximation.

This method was used in many experimental investigations [74]–[76]. It is

especially suitable for grown tricrystals, where two grain boundaries have the

same misorientation and the surface tension of the third can be calculated, or

when the observation of the change of the surface tension of the third grain

boundary is the main purpose of investigation (Fig. 1.16). Such experiments

make it possible to compare the surface tensions of diﬀerent grain bound-

aries, or grain boundaries with diﬀerent orientation in the same material. It

was shown, in particular, that the minima of the misorientation dependence

of the grain boundary surface tension correlate with special misorientations

in the coincidence site lattice theory (see Chapter 2).

Another relative method of investigation of the grain boundary surface ten-

sion was proposed by Wilson and Shewmon [77] and was applied in many

experimental studies [78]–[80]. If a number of small single-crystal balls would

be put on a single-crystal substrate of the same material at diﬀusion temper-

ature, they then would rotate during sintering to the substrate to decrease

the surface tension of the newly formed grain boundary. The main condition

for the proper conduct of the experiment is: the misorientation dependence

of the grain boundary surface tension must have singularities, sharp minima

with a discontinuity of the derivative of the surface tension with respect to

misorientation angle α, but not a usual minimum as mentioned in [35] (Fig.

1.15b). As the driving force of the rotation of the balls is proportional to the

1.3 Experiments 55

Special boundary

(b)

General boundary

Special boundary

(b)

General boundary

FIGURE 1.16

Sequential junction position during measurement of temperature dependence

of γ

/γ

derivative (∂γ/∂Θ), the driving force is kept up in the case of a singular dis-

continuity until the balls reach the minimum energy position, whereas for a

usual minimum the derivative — the driving force for rotation — tends to zero

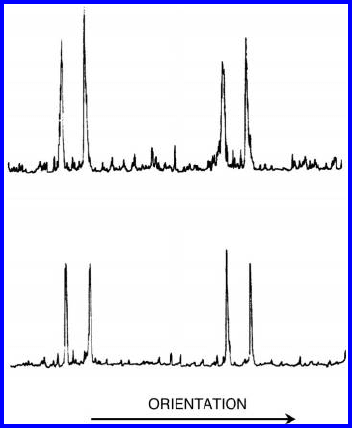
with the misorientation angle tending to the special misorientation. Fig. 1.17

[81] gives an example of the positions at special misorientations as estimated

by the method (Fig. 1.15b) discussed in [77].

To determine the absolute value of the grain boundary surface tension,

the tension of the reference surface must be known. For a known tension of

the free surface we can determine the grain boundary surface tension from

the equilibrium of the surface tensions at the root of a thermal groove (Fig.

1.15c). Unfortunately, there are only few methods to determine the tension of

afreesurfaceofthecrystal.

One of them is the method of zero creep [82] (Fig. 1.15d). Let us imagine a

wire of small diameter D or a thin foil with perimeter π · D which is loaded

with a weight P at diﬀusional creep temperatures. The free energy of the wire

(or foil) can be deﬁned as

F = P

+ γ

πDL (1.172)

where x is the coordinate of the weight P relative to the arbitrary point on

the normal to the earth surface, L is the length of the wire, γ

is the surface

tension of the free surface of the wire. The equilibrium (δF = 0) corresponds

to such changes of x and length which compensate each other. Taking into

account that δ

= − δL,weget



∂F

∂x



x=0

= P

− γ

πD = 0 (1.173)

56 1 Thermodynamics of Grain Boundaries

FIGURE 1.17

Corresponding sections through the pole ﬁgures of two specimen (1 and 2)

of the same type, demonstrating the reproducibility of the method used to

identify low energy boundaries. Both curves show similar peaks of diﬀracted

X-ray intensity at identical orientations [81].

1.3 Experiments 57

and γ

= P

/πD,whereP

is the so-called zero-creep weight.

A more correct description takes into account the area of the grain bound-

aries and their shape [83]–[85]. Evidently, the value of the free surface tension

determined in this way is averaged over a large number of crystallographic

planes.

The method of “ﬂoat wedge,” put forward and realized for the ﬁrst (and

the last) time by Shewmon, is free of the disadvantages mentioned above. The

scheme is given in Fig. 1.15e. The sharp hard wedge, which is not wetted by

the tested metal, is pressed to its surface by a known force P at diﬀusion

temperature. A groove which is similar to the thermal groove of the grain

boundary will form under the wedge. As we know the force acting on the tip

of the groove from the side of the wedge, we can calculate the surface tension

of the free surface, if the dihedral angle is measured. If, as shown in Fig. 1.15e,

a grain boundary comes to the same surface, the surface tension of the grain

boundary is equal to

γ =

P sin Θ

L sin Θ

(1.174)

where L is the length of the wedge perpendicular to the plane of Fig. 1.15e.

Admittedly, the described method has not been used and developed in the

30 years since the Shewmon paper was published. Apparently, this method is

extremely laborious, the main reason that it has fallen into oblivion.

The principle of another method to determine the absolute value of the sur-

face tension of both the free surface and a grain boundary meeting this surface

is given in Fig. 1.15f [70]. A plate of a material, which is not wetted by the

tested metal, is placed on the smooth ﬂat surface of the sample. (Graphite

is not wetted by many metals, like Cu, Au and so on.) The grain boundary

intersects the surface of the sample as well.

Under these conditions the groove will not grow unlimitedly, as would hap-

pen at the free surface. The growth of the groove will stop the sooner the

larger the load, comprised by the plate on the surface, i.e. as soon as the

excess chemical potential of the atoms at the curved surface of the groove,

which is γ

/R (Ω

is the atomic volume), becomes equal to the increase of

the chemical potential which is caused by the pressure of the plate: pΩ

A (

is the contact area under the plate). Then the surface tension of the external

surface of the crystal is γ

= pR/

A, and the grain boundary surface tension

can be determined from the dihedral angle at the root of the groove. Never-

theless, this method, which may be called the method of equilibrium groove,

has not yet been realized.

Let us use this opportunity to consider the question of how large the ther-

mal groove of the grain boundary could get under terrestrial conditions. The

development of the thermal groove will stop if the hydrostatic (capillary) pres-

sure which results from the curvature of the groove equals the gravitational

force:

/R = ρgh (1.175)

58 1 Thermodynamics of Grain Boundaries

where h is the depth of the groove, and ρ is the density of the metal.

Assuming h

∼

R,weget

∼



ρg

(1.176)

The expression under the root sign is known as the capillary constant. For val-

ues which are typical for metals, the limiting value of the radius of curvature

R, i.e. for the depth of the thermal groove h, is approximately ∼0.3 cm. Of

course, it is impossible to obtain such a groove in a reasonable time. Usually,

the depth of the thermal groove does not exceed some ten micrometers, and

this result is important for us, less from the general point of view than for

pragmatic reasons.

We discussed above that the grain boundary is a degenerate kind of in-

terphase, and if an external tensorial ﬁeld is applied to the system, the de-

generation will be removed and, from the thermodynamical standpoint, the

grain boundary can be described as an interphase. This provides a method

to measure the absolute value of the grain boundary surface tension. If an

external ﬁeld is applied to a system with a grain boundary, which is locked at

the surface of the sample, a diﬀerence in the speciﬁc free energies of the grains

will arise (g

−g

); due to this diﬀerence the initially ﬂat grain boundary will

bend like a membrane under the pressure. In this case the grain boundary

surface tension can be estimated as (Fig. 1.15g)

γ = R (g

− g

) (1.177)

The diﬀerence in the speciﬁc free energies of the grains (g

− g

) should be

large enough, otherwise the radius of grain boundary curvature R will be too

large to be measured with suﬃcient accuracy. Such a scheme, as can be seen,

provides a unique opportunity to determine the absolute value of grain bound-

ary surface tension. Firstly, this method was applied to the measurement in

polycrystalline Bi in a magnetic ﬁeld [86], and, quite recently, to the grain

boundary in bicrystals of Bi in a magnetic ﬁeld [87].

The modern techniques of determination of the chemical content of surface

layers in solids together with the thermodynamics of surfaces suggest a new

method of “experimental calculation” of the surface tension of free surfaces

of solid alloys [88]. As shown in [89], modern methods like low-energy ion-ion

scattering permits us to determine the content of a surface monolayer. Hence,

the surface tension of a free surface can be estimated by integrating the Gibbs

adsorption equation. This method, together with the determination of the

dihedral angle at the root of the grain boundary groove, holds considerable

promise for the study of grain boundary and interface phenomena in metals

and alloys.

1.3 Experiments 59

0 20406080100

[at.%]

0.6

0.5

0.4

0.3

[J/m

]

0 20406080100

[at.%]

0.6

0.5

0.4

0.3

[J/m

]

FIGURE 1.18

Dependence of grain boundary surface tension in Cu-Au on (atomic) Cu con-

centration [90].

1.3.3 Examples

In the following we will show how to determine grain boundary segregation

and how to obtain an estimation of the activity of the atoms in the boundary

solution in the event that the dependence of the grain boundary surface ten-

sion on the concentration has been measured, using the example of the binary

system Cu-Au [90]. The point is that there is very little information about

the isotherms of the surface tensions. Even if the dependence of the surface

tension on composition is known, it is only over a very narrow range of con-

centrations. Moreover, the isotherms of the activities (chemical potentials) of

the components usually are not known, and without suﬃcient evidence the

conclusion is drawn that the solutions are dilute.

There appear to be only two studies in which the surface tension of grain

boundaries has been measured for the entire range of concentration, namely

of the systems Cu-Au and Cu-Ni [24, 91], and there are isotherms of the bulk

activities of both components for these systems. For the system Cu-Au the

surface tension was determined by the zero-creep technique, while for the sys-

tem Cu-Ni the technique described above was applied using the measurement

of the chemistry of the surface monolayer and the Gibbs adsorption equa-

tion [88, 89, 91]. Because the method of thermodynamic treatment of grain

boundaries considered above (Eqs.(1.82)–(1.116)) was ﬁrst applied to the sys-

tem Cu-Au [24], the explanation of how this method works in detail will be

given using this system as an example.

The experimentally determined dependence of the grain boundary surface

tension on the atomic fraction of Cu in the system Cu-Au is depicted in Fig.

1.18. The grain boundary surface tension γ was determined at 1123 K by

60 1 Thermodynamics of Grain Boundaries

measuring the equilibrium angle at the root of a thermal groove:

cos Θ/2=γ/2γ

(1.178)

where γ

is the surface tension of the free surface was determined by the

zero-creep technique at the same temperature (Fig. 1.18). The isotherms for

the activities a

of Cu-Au components at 800 K were taken from reference

books [92, 93] and converted to 1123 K using the equation

∂ ln a

∂T

(1.179)

assuming that the partial molar enthalpy of dissolution of the i-th component

does not depend on temperature in the range from 800 to 1123 K.

We would like to reiterate once again that the model of a homogeneous grain

boundary used in Eqs.(1.82)–(1.116) and taken as a basis for the calculations

in [24, 90] coincides with the model for the surface layer of Guggenheim and

Adam [8]. The same model was used by Zhuchovitskii in his theory of sur-

face solutions [25]. Zhuchovitskii treated a surface layer as a two-dimensional

solution with its own thermodynamic properties and in equilibrium with a

volume solution. The dependence of the surface tension on composition in

such a system is deﬁned by Eqs.(1.93)–(1.105):

γ − γ

= z RT ln a

where z is the total number of adsorption centers in a unit boundary area; z

is assumed to be independent of composition. In our notation z = λρ

.The

combined solution of Eqs.(1.103)–(1.104) and the Gibbs equation in the form

z RT [C

d ln a

+(1− C

) d ln a

]=−dγ (1.180)

provides a means for obtaining the activity isotherm of each component of a

binary surface (boundary) solution: a

). But if the adsorption isotherm



is calculated, then the composition of the surface solution N

can be found

from Eq. (1.115)



= z (C

− C

)

The relation between the activities of the components in surface and volume

solutions according to Zhuchovitskii (Eq. (1.97)) is given by

exp



− γ

z RT



where, obviously, (γ

−γ

)/z means the heat of adsorption, which is a constant

in the discussed model.

Eqs.(1.103)–(1.105) can be generalized to the case of several “types of sites”

at the grain boundary [24]:

γ − γ

=RT







(1.181)

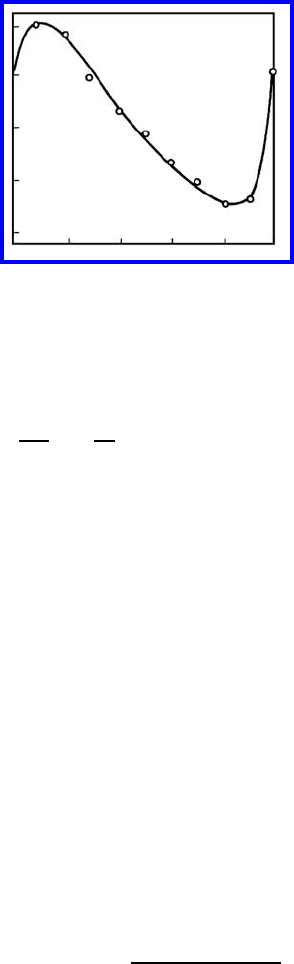
1.3 Experiments 61

0 20 40 60 80 100

-2

-4

-6

[at.%]

Γ’

[10

mol/cm

]

0 20 40 60 80 100

-2

-4

-6

[at.%]

Γ’

[10

mol/cm

]

FIGURE 1.19

Isotherm of Cu adsorption on grain boundaries in Cu-Au at 850

◦

C[24].





exp (q

/kT) (1.182)

where z

is the number of sites of the k-th type, a

and q

are the activity

and heat of adsorption of the component i in a site k of the grain boundary.

The adsorption of the i-th component is given by





− C

) (1.183)

is the atomic fraction of the component i in a site k of the grain boundary

+ C

=1).

The boundary adsorption was calculated from Eq. (1.53) due to the fact

that dμ

= RT d ln a

and Γ



+Γ



= 0. Using the Gibbs-Duhem equa-

tion the value of Γ



can be calculated from either a

)ora

). The

isotherm of the boundary adsorption of copper Γ

) is given in Fig. 1.19.

The dependence Γ



) is oscillating because of the minimum of the curve of

γ (c

The experimental data, given above, were treated thermodynamically in

[24], modifying the model until a reasonable agreement between experiment

and calculation is reached. So, for the Langmuir adsorption and a non-ideal

bulk solution (γ

= a



= q + RT lnγ

/γ

)wehave



= zc

(1 − c

)



/RT

− 1

/RT

− c

(1.184)

It is evident from the equation that Γ



has the same sign as q



.Ascan

be seen from Eq. (1.184), the dependence γ (c

) must have a maximum. Since

the experimentally determined dependence γ (N

) is quite diﬀerent, this model