Geiser J. Decomposition Methods for Differential Equations: Theory and Applications

Подождите немного. Документ загружается.

Discretization Methods 269

where the parameters are a = V

i,jk

− c

i,jk



),b = V

i,jk



,and

i,j

= V

. The linear impulse in the ﬁnite volume cell is c

i,jk



for

the concentration at the inﬂow and c

i,jk

for the concentration at the outﬂow

boundary of cell j.

The discretization with the embedded analytical mass is calculated by

n+1

i,j

− m

i,rest

= −



k∈out(j)

i,jk,out



l∈in(j)

i,lj,out

, (B.173)

where

is the retransformation of the total mass m

i,jk,out

into the partial

mass m

i,jk

. The mass in the next time-step is m

n+1

i,j

= V

n+1

,intheold

time-step it is the rest mass for the concentration i. The proof can be found

in [81].

REMARK B.19 In the embedding methods, we assume to have the

one-dimensional analytical or cheap by solving a simple ODE system. Based

on this we can decouple the discretization methods to cheap parts (e.g., the

reaction parts) and noncheap parts, the complicated spatial parts (e.g., elliptic

parts). That knowledge can save memory and computational time in the

discretization methods.

Advanced Discretization Methods, Integration of Decomposing

Methods

Often the standard methods are too general to obtain the needed accuracy.

Therefore, new modiﬁed methods were developed to gain higher-order results.

In Table B.1 we present such methods.

TABLE B.1: Modiﬁcations for the standard methods.

Discretization Modiﬁcation

Method for the Method

Finite Volume Reconstruction

Method, see [14]

Finite Element Enriched Methods, see [15], [123]

(e.g., Bubble Functions)

Discontinuous Galerkin Embedded Test Functions

(e.g., One-Dimensional Solutions), see [85]

Especially for the discretization methods for parabolic diﬀerential equa-

tions, there exist several methods, for example,

1. Characteristic methods (exact transport and reaction): Test functions

(linear or constant) are exact transported. Only approximation error

270Decomposition Methods for Diﬀerential Equations Theory and Applications

for the initial condition and splitting error in multiple dimensions, see

[177].

2. Locally improved test functions: New improved test space for the ﬁnite

volume or DG methods, locally exact solutions, see [85].

3. Locally improved trial functions to improve the discretization of critical

terms. Idea: skip the critical terms via analytical solutions, see [84].

B.3.5.5 Extended Discretization Methods : Discontinuous Galerkin

Methods

• Local mass conservation

• Higher-order methods (test functions with higher polynomials)

• Application for unstructured grids (adaptive grids)

• One grid (primary grid)

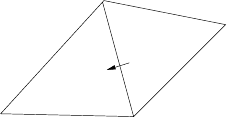
The direction of the element normal vector is given as in Figure B.8.

FIGURE B.8: Deﬁnition of the element normal vector.

• Triangulation K

for h>0 for the domain Ω

• Broken Sobolev space by

)={v ∈ L

(Ω) : v|

∈ H

(K) ∀K ∈K

}. (B.174)

Notation for Discontinuous Galerkin Methods

• Triangulation K

for h>0 for the domain Ω.

• Subdomain K ∈K

is a Lipschitz boundary.

•E

of all interior boundaries e of K

•E

of all exterior boundaries e of Γ = ∂Ω.

Discretization Methods 271

We then have the jumps across the edge e = ∂K

∩ ∂K

[v]=(v|

− (v|

. (B.175)

We also have the averages on the interfaces:

{v} =

(v|

+(v|

. (B.176)

Mixed Formulation for the Convection-Diﬀusion-Reaction

Equation

The solution is given by u(x, t) ∈ C

(Ω)×C

([0,T]) and p(x, t) ∈ (C

(Ω)×

([0,T]))

for the classical formulation, see also [85]:

∂

Ru + ∇·v u −∇·a

1/2

p + Rλ c = f,in Ω × [0,T], (B.177)

−a

1/2

∇u + p =0, in Ω × [0,T], (B.178)

u = g

, on Γ

× [0,T] ,

u − a

1/2

∇u) · n = g

, on Γ

× [0,T] ,

u(0) = u

, in Ω.

The inner product L

(S) is denoted as (·, ·)

,andforS =ΩweskiptheS.

Variational Formulation for the Mixed Equations

For the mixed methods we have to ﬁnd the unknowns

∈ L

((H

))

, [0,T]) and u ∈ L

), [0,T]) as follows:

(R∂

u, φ) −



K∈K

(u, v ·∇φ)



e∈E

conv

(u), [φ])



K∈K

1/2

p, ∇φ>



e∈E

diff

), [φ])

+(Rλu,φ)



e∈E

,φ)

+(f,φ) ,φ∈ H

), (B.179)

,χ> +



K∈K

(u, ∇·a

1/2

χ)



e∈E

diff

), [a

1/2

χ · n])



e∈E

,χ· n)

,χ∈ (H

))

. (B.180)

We have the convective ﬂuxes:

conv

v n} central diﬀerences

v n}−

|v n|

]upwind

, (B.181)

diff

)=(a

1/2

n {u

}, {a

1/2

· n})

+ C

diff

[(u

)

], (B.182)

272Decomposition Methods for Diﬀerential Equations Theory and Applications

where the ﬂux matrix C

diff

is given as

diff

⎛

⎜

⎝

0 −c

1,2

... −c

1,d+1

1,2

0 ... 0

. ...

1,d+1

0 ... 0

⎞

⎟

⎠



0 −c

c 0



where c

=(c

1,2

,...,c

1,d+1

)

and c

1,i

= c

1,i

((w

, (w

) are locally

Lipschitz.

Stability of the Mixed Form

We have the stability for the full-discrete form with the solutions u

∈ V

and p

∈ W , such that

||u

(T )||

(Ω)



) dt +



||p

(Ω))



Rλ

||u

(Ω)

≤

||u

(0)||

(Ω)



2 Rλ

||f||

(Ω)

dt, (B.183)

where Θ



e∈E

< [w

] ,C[w

] >

and

C =

⎛

⎝

1,1

−c

c 0

⎞

⎠

(B.184)

is the ﬂux matrix.

Design of New Test Functions with Local Analytical Solutions

For the adjoint problem of the convection-reaction terms, we get

−



K∈K

,v·∇φ)

+(u

,Rλφ) (B.185)



K∈K

, −v ·∇φ + Rλφ)=0,

where u

,φ ∈ V

, and we solve the adjoint local equation for the convection

reaction in space:

−v

·∇φ + Rλφ=0, (B.186)

where the initial condition is φ(0) = φ

Discretization Methods 273

Embed Local Analytical Solutions to Test Functions of the

Discontinuous Galerkin Methods

The motivation for the new test spaces came from the idea to improve the

local behavior of the test functions. Standard test functions like polynomials

do not respect the local character.

To have a local behavior of the solutions we use the ideas of the adjoint

problems. They are done in the ELLAM schemes [177]. We use these ideas

for the space terms and solve the locally adjoint problems for the spatial

dimensions.

We concentrate on the convection-reaction equation, given as

−



K∈K

,v·∇φ)

+(u

,Rλφ) (B.187)



K∈K

, −v ·∇φ + Rλφ)=0, (B.188)

where u

,φ ∈ V

, and solve the adjoint local equation for the convection

reaction in space:

−v

·∇φ + Rλφ=0, (B.189)

where the initial condition is φ(0) = φ

. We derive the local solution of the

equation (B.189) the next section.

Local Test Functions in Space for One Dimension

We derive the one-dimensional solutions for the local convection-reaction

equation, given as adjoint problem:

−v∂

φ + Rλφ=0, (B.190)

whereby φ(0) = a

is a constant.

The equation (B.190) is solved exactly and the solution is denoted with

respect to the velocity v ∈ R and v =0:

anal,i

(x)=a

⎧

⎨

⎩

exp(−β (x

i+1/2

− x)) v>0

exp(−β (x − x

i−1/2

)) v<0

, (B.191)

where β =

Rλ

|v|

new,i

= φ

anal,i

(x) , (B.192)

where x

i−1/2

<x<x

i+1/2

The local solution is applied as an analytical weight with 0 ≤ φ

anal,i

(x) ≤ 1.

For this test function we have one degree of freedom, so that we could use

only a constant initial condition.

274Decomposition Methods for Diﬀerential Equations Theory and Applications

For linear initial conditions we use the analytical test function and multiply

it with the standard test function of ﬁrst-order.

Thus, we have

new,i

stand,i

(x) φ

anal,i

(x) , (B.193)

whereby the standard test functions are given as polynomial functions

stand

(x)={1,x,x

,...}.

The test functions are used for the cases |v| >> λ ≥ 0, for the case v =0

we use the standard test functions, cf. [48].

So we improve it for constant cell impulses. To present the test functions

we have Figures B.9 and B.10 for two extreme cases: λ<<vand for λ = v.

0 2

0 4

0 6

0 8

0 2 0 4 0 6 0 8 1

0 2

0 4

0 6

0 8

0 2 0 4 0 6 0 8 1

FIGURE B.9: Local test functions constructed with analytical solution

and constant initial condition.

0 2

0 4

0 6

0 8

0 2 0 4 0 6 0 8 1

0 2

0 4

0 6

0 8

0 2 0 4 0 6 0 8 1

FIGURE B.10: Local test functions constructed with analytical solution

and linear initial condition.

Discretization Methods 275

REMARK B.20 The local discontinuous Galerkin methods can be

improved by local analytical solutions. Like the ELLAM schemes, we designed

the test functions based on the dual formulation. That investigation can save

memory and computational time in the discretization methods.

References

[1] M. Abramowitz and I.A. Stegun. Handbook of Mathematical Functions.

Dover Publication, New York, 1970.

[2] G. Akrivis, M. Crouzeis, and Ch. Makridakis. Implicit-explicit multistep

methods for quasilinear parabolic equations. Numerische Mathematik,

82:521–541, 1999.

[3] I. Alonso-Mallo, B. Cano, and J.C. Jorge. Spectral-fractional step

Runge-Kutta discretisations for initial boundary value problems with

time dependent boundary conditions. Mathematics of Computation,

73(248):1801–1825, 2004.

[4] Z. S. Alterman and A. Rotenberg. Seismic waves in a quarter plane.

Bulletin of the Seismological Society of America, 59:347–368, 1969

[5] D.N. Arnold, F. Brezzi, B. Cockburn, and L.D. Marini. Uniﬁed analysis

of discontinuous Galerkin methods for elliptic problems. SINUM Society

for Industrial and Applied Mathematics, 39(5):1749–1779, 2002.

[6] U.M. Ascher, S.J. Ruuth, and B.T.R. Wetton. Implicit-explicit meth-

ods for time-dependent partial diﬀerential equations. SIAM Journal on

Numerical Analysis, 32(3): 797–823, 1995.

[7] U.M. Ascher, S.J. Ruuth, and R.J. Spiteri. Implicit-explicit Runge-Kutta

methods for time-dependent partial diﬀerential equations. Appl. Num.

Math., 25(2–3):151–167, 1997.

[8] W. Balser and J. Mozo-Fernandez. Multisummability of formal solutions

of singular perturbation problems. J. Diﬀerential Equations, 183(2): 526–

545, 2002.

[9] W. Balser, A. Duval, and St. Malek, Summability of formal solutions for

abstract Cauchy problems and related convolution equations. Manuscript,

November 2006.

[10] W. Bangerth and R. Rannacher. Adaptive ﬁnite element methods for

diﬀerential equations. Lectures in Mathematics, ETH Z¨urich, Birkh¨auser

Verlag, Basel-Boston-Berlin, 2003.

[11] V. Barbu. Nonlinear Semigroups and Diﬀerential Equations in Banach

Spaces. Editura Academiei, Bucuresti, and Noordhoﬀ, Leyden, 1976.

277

278 References

[12] V. Barbu. Analysis and Control of Nonlinear Inﬁnite Dimensional Sys-

tems. Acad. Press, Boston, 1993.

[13] V. Barbu. Partial Diﬀerential Equations and Boundary Value Prob-

lems. Mathematics and its Applications, Kluwer Academic Publishers,

Dordrecht, Boston, London, 1998.

[14] T. Barth and M. Ohlberger. Finite volume methods: Foundation and

analysis. Encyclopedia of Computational Mechanics, editors: E. Stein,

R. Borst, T.J.R. Hughes, West Sussex, England, John Wiley and Sons,

Vol.1., chapter 15, 2004.

[15] I. Babuska, U. Banerjee, and J.E. Osborn. Survey of meshless and gen-

eralized ﬁnite element methods: A uniﬁed approach. Acta Numerica,

Cambridge University Press, 12:1–125, 2003.

[16] D.A. Barry, C.T. Miller, and P.J. Culligan-Hensley. Temporal discretiza-

tion errors in non-iterative split-operator approaches to solving chemical

reaction/groundwater transport models. Journal of Contaminant Hydrol-

ogy, 22: 1–17, 1996.

[17] P. Bastian. Parallele adaptive Mehrgitterverfahren. PhD thesis, Univer-

sity of Heidelberg, Germany, 1994.

[18] P. Bastian, K. Birken, K. Eckstein, K. Johannsen, S. Lang, N. Neuss,

and H. Rentz-Reichert. UG-a ﬂexible software toolbox for solving partial

diﬀerential equations. Computing and Visualization in Science, 1(1):27–

40, 1997.

[19] S. Bartels and A. Prohl. Convergence of an implicit ﬁnite element

method for the Landau-Lifschitz-Gilbert equation. SIAM J. Numer.

Anal., 44(4):1405–1419, 2006.

[20] K.J. Bathe. Finite Element Procedures. Prentice Hall, New Jersey, 1996.

[21] P. Bauer, S. Attinger, and W. Kinzelbach. Transport of a decay chain

in homogeneous porous media: Analytical solutions. Journal of Contam-

inant Hydrology, 49(3–4):217–239, 2001.

[22] R.M. Beam and R. F. Warming. Alternating direction implicit meth-

ods for parabolic equations with a mixed derivative. SIAM J. Sci. Stat.

Comput., 1:131–159, 1980.

[23] J. Bear. Dynamics of Fluids in Porous Media. American Elsevier, New

York, 1972.

[24] J. Bear and Y. Bachmat. Introduction to Modeling of Transport Phe-

nomena in Porous Media. Kluwer Academic Publishers, Dordrecht,

Boston, London, 1991.

References 279

[25] J. Bey. Finite-Volumen- und Mehrgitter-Verfahren f¨ur elliptische

Randwertprobleme. Advances in Numerical Mathematics, B.G. Teub-

ner Stuttgart, Leipzig, 1998 .

[26] M. Bjorhus. Operator splitting for abstract Cauchy problems. IMA Jour-

nal of Numerical Analysis, 18:419–443, 1998.

[27] D. Braess. Finite Elemente. Springer-Verlag, Berlin, Heidelberg, New

York, 1992.

[28] S.C. Brenner and C. Carstensen. Finite Element Methods. Encyclopedia

of Computational Mechanics, John Wiley and Sons, 2004.

[29] S.C. Brenner and L.R. Scott. The Mathematicla Theory of Finite Ele-

ment Methods. Texts in Applied Mathematics, Vol. 15, Springer Verlag,

Berlin, Heidelberg, 2002.

[30] J. Budd and A. Iserles. Geomertic integration: Numerical solution of

diﬀerential equations on manifolds. R.Soc.LondonPhilos.Trans.A,

357:943–1133, 1999.

[31] J. Budd and M.D. Piggott. Geometric integration and its applications.

Lecture notes available, [http://www.bath.ac.uk/ mascjb/home.html],

2002.

[32] D. Buhmann. Das Programmpaket EMOS. Ein Instrumentarium zur

Analyse der Langzeitsicherheit von Endlagern. Gesellschaft f¨ur Anlagen-

und Reaktorsicherheit (mbH), GRS-159, Braunschweig, 1999.

[33] J.C. Butcher. Implicit Runge-Kutta processes. Math. Comp., 18:50–64,

1964.

[34] J.C. Butcher. Numerical Methods for Ordinary Diﬀerential Equations.

John Wiley & Sons Ltd, Chichester, 2003.

[35] X.C. Cai. Additive Schwarz algorithms for parabolic convection-diﬀusion

equations. Numer. Math., 60(1):41–61, 1991.

[36] X.C. Cai. Multiplicative Schwarz methods for parabolic problems. SIAM

J. Sci Comput., 15(3):587–603, 1994.

[37] S. Candel. A review of numerical methods in acoustic wave propaga-

tion. in Recent Advances in Aeroacoustics, Springer, New York, 339–

410, 1986.

[38] H.S. Carlslaw and J.C. J¨ager. Conduction of Heat in Solids. Clarendon

Press, Oxford, 1959.

[39] C. Carstensen and S.A. Sauter. A posteriori error analysis for elliptic

PDEs on domains with complicated structures. Numer. Math., 96:691–

721, 2004.