Fattorini H.O., Kerber A. The Cauchy Problem

Подождите немного. Документ загружается.

7.7. The General Case 427

with

Ilull,,<e`1`-`1Ilulls

(uEE,0<s,t<T),

(7.7.11)

and let E, be the space E endowed with the norm II'II, Assume that

A(t)Ee+(1,w,E,) (0<t<T)

(7.7.12)

for some co. Then the family A(.) is stable.

Another method of constructing stable families is by bounded per-

turbations of other stable families. In this direction we have the following

result (which will not be used until next section).

7.7.4

Lemma.

Let

be stable (with constants C, w) and let B(-)

be a family of operators in (E) such that

IIB(t)ll <M (0<t<T).

(7.7.13)

Then (A(t)+B(t); 0 <t <T) is stable (with constants C,w+CM).

Proof It was proved in Theorem 5.1.2 that if A E C+(C, w) and

B E (E), then A + B E+(C, w + CIIBII) by using a direct construction (and

subsequent estimation) of R(X; A + B) as a power series in R(A; A). The

same power series development and a very similar estimation prove the

present result. Details are left to the reader.

We are now in condition to demonstrate a very general result to the

effect that the Cauchy problem for (7.7.1) is well posed. However, we are

forced once again to modify the notion of solution introduced in Section

7.1. In the following definition we assume that (A (t); 0 < t <T) is a family

of densely defined operators in E and F a subspace of E such that

FcD(A(t))(0<t<T).

and only if :

(a)

is E-continuous and u(t) E F in s < t < T.

(b) The right-sided derivative

D+u(t)= lim h-'(u(t+h)-u(t))

h-0+

exists (in the norm of E) and

D+u(t) = A(t)u(t)

ins<t<T.

7.7.5 Theorem. Let E, F be Banach spaces with F - E and F

reflexive, and let (A(t); 0 < t <T) be a family of densely defined operators in

E. Assume that: (a) is stable in E (with constants C, w). (b) F is

A(t)-admissible for 0 < t < T. (c) The family (A(t)F; 0 < t < T) is stable in F

(with constants C, Co). (d) F c D(A(t)) and A(t): F- E, belongs to (F; E)

428

The Abstract Cauchy Problem for Time-Dependent Equations

for each t. (e) If A(t) is the restriction of A(t) to F, the map t -* A(t) from

0 < t < T into (F; E) is continuous. Then the Cauchy problem for (7.7.1) is

properly posed with respect to solutions defined in (C) with D = F. If S(t, s) is

the evolution operator of (7.7.1), then S is E-strongly continuous in the triangle

0<s<t<Tand

IIS(t,s)II(E)<Ce0(t_ (0<s<t<T), (7.7.14)

where C, w are the constants in (a). For each u E F, S(t, s)u is differentiable

with respect to s in 0 < s < t and

DsS(t,s)u=-S(t,s)A(s)u (0<s<t).

(7.7.15)

Finally, S(t, s)F c F and

IIS(t,s)II(F)<Ce"(`_

(0<s<t<T), (7.7.16)

where C, Co are the constants in (c), and S(t, s) is F-weakly continuous in

0<s<t<T.

Proof.

Let An (t) be defined by

(!-Tt<!T),

(7.7.17)

k = 1,1,2,... , n, and (for the sake of completeness), An(T) = A(T). In view of

assumption (e),

IIAn(t)-A(t)II(F;E)

(7.7.18)

uniformly in 0 < t < T, where the tilde, as before, indicates restriction to F.

It is obvious that is stable in E and that A,(-)F'S stable in F, in both

cases with the same constants C, w, C, w postulated in (a) and (c), therefore

independent of n.

We examine now the equation

u'(t)=An(t)u(t).

(7.7.19)

A moment's reflection shows that if 0 < s < T and u E F, then (7.7.19) has a

solution in s < t < T with initial value

given by

UnW

(7.7.20)

where the operator s) is defined as follows. Let 0 < s < t < T and let

k, l be two integers, 1 < k, l < n, such that

T < s <

I-1

T<t< l

n n

so that k < l (clearly k = [ns/T ]+ 1, 1 = [nt/T ]+ 1, where [.1 denotes

7.7. The General Case

429

integral part). Then

_ _

r-1 _

S,,(t, S) = St -

n 1 T; A(1 n I T)){7

S(n

T; A(J

n I

T))

XS( nT- s;A(kn I T))

(7.7.21)

if k < 1 (if k = I - 1 the product between curly brackets is taken equal to the

identity operator). If k = 1,

(7.7.22)

The word "solution" in the preceding statement is understood in the sense

of Definition (C), or more precisely as follows:

is a genuine solution of

(7.7.19) in each interval

Is,nT),t7n1T,-T) (j=k+l,...,n),

and it

is continuous in s < t < T. Likewise, the function s -p S(t, s)u is

continuous in 0 < s < t, continuously differentiable in each interval

[i_±T,LT)

(j=l,...,k-1),

T,t

k<I-l

k=1T kT

k+lT... I=2T

!-IT lT

n n

n n n

...

A(knlT)

A(nT)

... A(/nzT )

A(/nlT)

...

kk=1T kT !T

A(kn

ll T)

A(nT)

k=1

kn lT

1 1 nT

A(k

I T)

FIGURE 7.7.1

430

The Abstract Cauchy Problem for Time-Dependent Equations

and satisfies there

DSSn(t, s)u = - Sn(t, s)A(s)u.

(7.7.23)

As the reader will no doubt suspect by now, the propagator S(t, s) of (7.7.1)

will be constructed as the limit of the Sn, in a way quite similar to that

employed in Example 7:1.8 (but under totally different hypotheses). Conse-

quently, the next step will be to show that, for each u e E,

lim Sn(t,s)u=S(t,s)u

(7.7.24)

n - co

exists, uniformly in 0 < s < t < T. In order to do this, we begin by noting

that it follows from stability of A(.) in E, stability of A( )F in F, A(t)-

admissibility of F, and (part of) Lemma 7.7.1 that Sn (t, s) F C F and

IISn(t1S)II(E)<Ce"(t-s)1

IISn(t1S)II(F)<Ce"(t-s) (7.7.25)

in 0 < s < t < T, n =1, 2..... Hence

(7.7.24) needs to be shown only for u in

a dense subset of E, say, for u e F. In order to do this we take profit of our

previous observations concerning Sn(t, s). If u E F and 0 < s < t < T, it is

easy to see that a -> Sn(t, a)Sm(a, s)u is continuous in s <a< t and con-

tinuously differentiable there (except perhaps at points of the form kT/n

lying in [s, t]) and

D0Sn(t, a)Sm(a, s)u = - Sn(t, a)An(a)Sm(a, r)u

+Sn(t,a)Am(a)Sm(a,s)u

= Sn(t,(Y )(Am(a)-An((Y ))SS.(a,S)u.

(7.7.26)

Integrating in s < a < t, we obtain

(Sm(t,s)-Sn(t,s))u= f tS.(t,a)(An(a)-Am(a))Sm(a,s)uda,

(7.7.27)

hence

II(Sn(t,S)-SSm(t,S))uIIE<CCe(w+W)(t-s)IIuIIIf

tlIAn(a)-Am(a)II(F;E)da,

(7.7.28)

which estimate, in view of (7.7.18), shows that Sn(t, s) u is convergent for

u E F; in view of the first inequality (7.7.25) and the denseness of F,

Sn(t, s)u is actually convergent for all u E E uniformly in the triangle

0 < s < t < T. Since each Sm is strongly continuous, S is strongly continuous

in 0 < s < t < T. We also have

S(s,s)=I.

In order to study the differentiability properties of S stated in Theorem

7.7.5, we shall make use of the following result:

7.7. The General Case

431

7.7.6

Lemma.

Let

be a family of densely defined operators in

E satisfying exactly the same assumptions as

in Theorem 7.7.7 (we

suppose that F is the same, as well as C, w, C, Ca ). Let S+( ,

) be the operator

function constructed from A

in the same way as S(-, ) was constructed

from A(-). Then

II(S+(t,s)-S(t,s))ullE<CCe(w+w)(t-S)IIuIIFf `IIA+(a)-A(a)II(F,E)do

(7.7.29)

in0<s<t<T.

To prove this, we use an argument very similar to that leading

to (7.7.28). If A is defined from A

as in (7.7.21), (7.7.22), we can

prove inequality (7.7.29) for A

and reasoning with the function

s - S (t, a )S (a, s). We make then use of the convergence properties of

S and S,, proved a moment ago and (7.7.29) results.

Inequality (7.7.29) will be put to work with the following A+(-):

given 0 < s < T, let

A+(t)=

f A(t)

(0<t<s)

(7.7.30)

A(s) (s<t<T).

The assumptions of Lemma 7.7.6 are rather trivially satisfied; there-

fore (7.7.29) holds. Since the right-hand side is o(t - s) as t - s + and (as

we see easily) S+(t, s)u = S(t - s; A(s))u (t > s), it follows that D,+S(t, s)u

exists at t = s and equals A(s)u = A(t)u.

We obtain easily from the definition of S that

(0<s<a<t<T).

(7.7.31)

Applying both sides of this equality to an arbitrary u E E and taking limits,

S(t, S) = S(t, a)S(a, s),

FIGURE 7.7.2

432 The Abstract Cauchy Problem for Time-Dependent Equations

thus

S(t+h,s)-S(t,s)=(S(t+h,t)-S(t,t))S(t,s). (7.7.32)

Therefore, the required right-sided differentiability of S(t, s) u for u E F

(and the equality D, S(t, s)u = A(t)S(t, s)u) will follow if we show that

S(t, s)F c F

for 0 < s < t < T. We do this next, and the reader will note that reflexivity of

F is used only here. If u E F, we have already noted that S (t, s) u E F; on

account of the uniform boundedness of IISfhI(F) (see the second inequality

(7.7.25)), (S,, (t, s)u) must be bounded in F and must then contain a weakly

convergent subsequence (which we denote in the same fashion). Let v be the

weak limit of (S,, (t, s)u). Since S,, (t, s)u -> S(t, s)u strongly (thus weakly)

in E and every continuous linear functional in E is a continuous linear

functional in F, it follows that S(t, s)u = v.

It is clear that (7.7.16) results from this argument and from the

second inequality (7.7.25).

To prove F-weak continuity of S, we argue in a somewhat similar

way. Let (t, s), (t,,, s,,), n =1, 2.... be points in the triangle 0 < s < t < T

such that (t,,, sn) - (t, s), and let u E F. If S(t,,, does not converge to

S(t, s)u weakly, there exists u* E F*, e> 0 and a subsequence of ((t,,,

(which we design with the same symbol) such that

E. (7.7.33)

However, in view of the reflexivity of F, we may assume (if necessary after

further thinning out of the sequence) that (S(t,,,

is weakly convergent

in F (thus in E) to some v E F. But S(t,,, S(t, s)u strongly, hence

weakly, in E. It follows that v = S(t, s)u, which contradicts (7.7.33) and

completes our argument.

It only remains to study the s-differentiability of S(t, s) u, u E F. The

fact that s - S(t, s)u has a left-sided derivative at s = t can be proved

exactly in the same way in which we show that t -* S(t, s) u has a right-sided

derivative; we use now

A (t)-

A(s)

(0<t<s)

(7.7.34)

A(t)

(s < t <T)

instead of A+(t) and we obtain that DS-S(t, s)u = - A(t)u for s = t.

0<s<t,

h-'(S(t,s-h)-S(t,s))u=S(t,s)h-'(S(s,s-h)u-u),

so that DS S(t, s) exists in 0 < s < t and

DS S(t,s)u=-S(t,s)A(s)u.

7.7. The General Case

433

A-(t)

A(t)

FIGURE 7.7.3

On the other hand, if 0 < s < t,

h-'(S(t,s+h)-S(t,s))u=S(t,s+h)h-'(u-S(s+ h,s)u)

-S(t,s)A(s)u

as h -+ 0 + in view of the right-sided differentiability of t -f S(t, s) u at t = s

and the strong continuity and uniform boundedness of S.

Finally, let be an arbitrary solution of (7.7.1) ins 5 t < Tin the

sense of Definition (C). Let t > s and write v (a) = S(t, a) u(a ), s 5 a < t.

We have

v(a+h)-v(a)=S(t,a+h)u(a+h)-S(t,a)u(a)

=S(t,a+h)(u(a+h)-u(a))

+(S(t,a+h)-S(t,a))u(a)

so that the right-sided derivative of v(.) exists in s < a < t and vanishes

there. After application of linear functionals, we deduce that v(.) is con-

stant from the following result:

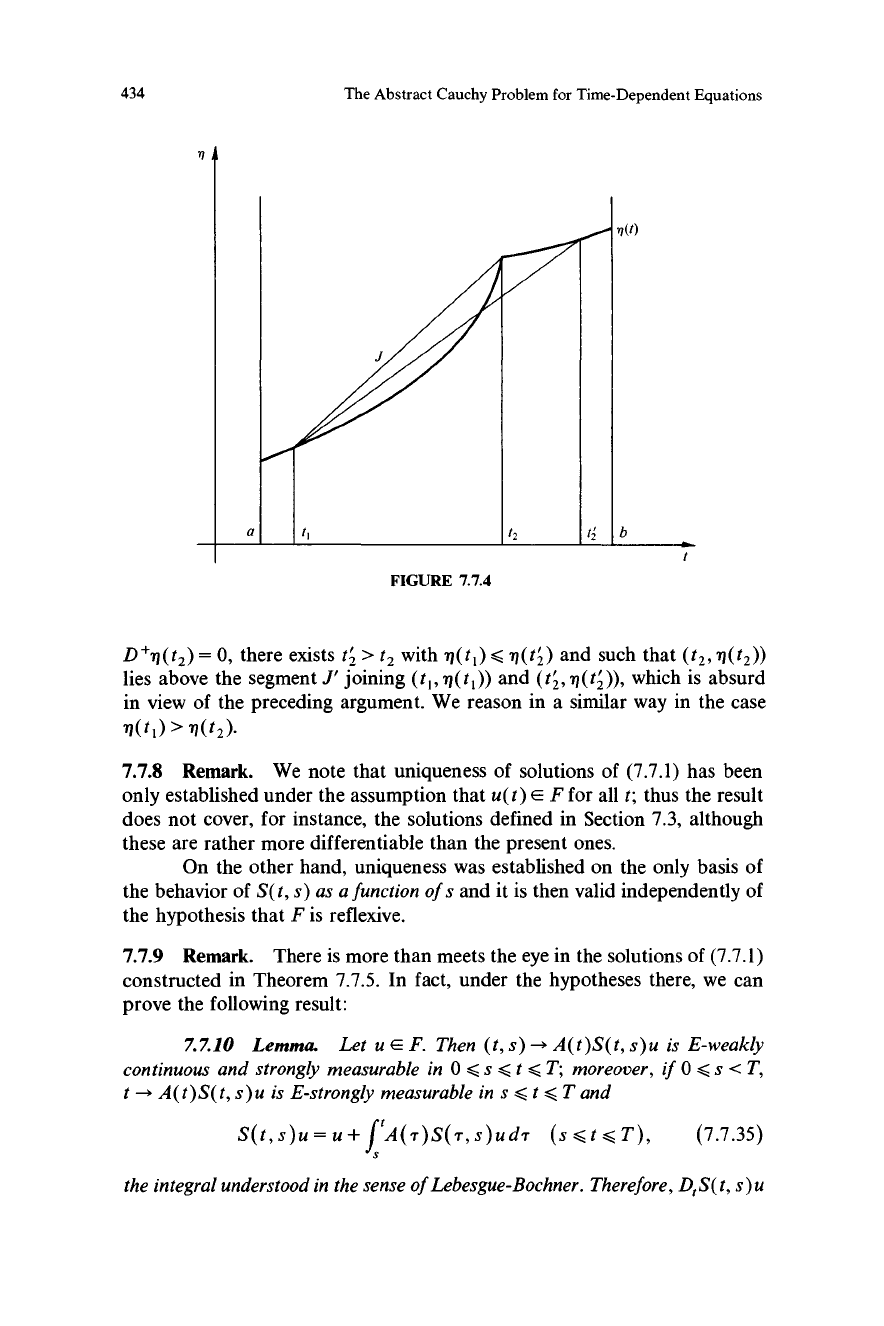
7.7.7 Lemma. Let q be a function defined and continuous in a< t

b, and such that D+,n exists and equals zero in a < t < b. Then q is constant.

In fact, since must be constant,

u(t) = v(t) = S(t, s)u(s),

which ends the uniqueness argument and thus the proof of Theorem 7.7.5.

Lemma 7.7.7 can be proved as follows. Assume q is not constant,

and let a < t, < t2 < b with, say, 'q (t,) < *t2 ). Then the graph of 71

lies

below the segment J joining (t ,q(t1)) and (t2,'Q(t2)) (otherwise, on account

of the continuity of q, it is not difficult to prove that there exists to,

t, < t0 < t2 such that (to,,q(to)) E J and the graph of q lies above J in some

interval to the right of to, thus D+rt(to) > 0). (See Figure 7.7.4.) Since

434

The Abstract Cauchy Problem for Time-Dependent Equations

FIGURE 7.7.4

D+,q(t2) = 0, there exists t; > t2 with ri(t1) < n(t2) and such that (t2, q(t2))

lies above the segment J' joining (tl,,q(tl)) and (ti, n(t2)), which is absurd

in view of the preceding argument. We reason in a similar way in the case

n(tl) > 71(t2)

7.7.8

Remark. We note that uniqueness of solutions of (7.7.1) has been

only established under the assumption that u(t) E F for all t; thus the result

does not cover, for instance, the solutions defined in Section 7.3, although

these are rather more differentiable than the present ones.

On the other hand, uniqueness was established on the only basis of

the behavior of S(t, s) as a function of s and it is then valid independently of

the hypothesis that F is reflexive.

7.7.9 Remark.

There is more than meets the eye in the solutions of (7.7.1)

constructed in Theorem 7.7.5. In fact, under the hypotheses there, we can

prove the following result:

7.7.10 Lemma.

Let u E F. Then (t, s) -* A(t)S(t, s)u is E-weakly

continuous and strongly measurable in 0 < s < t < T; moreover, if 0 < s < T,

t -* A(t)S(t, s)u is E-strongly measurable in s < t < T and

S(t,s)u=u+I A(r)S(T,s)udT (s<t<T), (7.7.35)

the integral understood in the sense of Lebesgue-Bochner. Therefore, DS(t, s) u

7.7. The General Case

435

exists in a set e = e(s) of full measure in [s, t] and

DDS(t,s)u=A(t)S(t,s)u (s<t<T)

wherever the derivative exists.

Proof. We have proved in Theorem 7.7.5 that (t, s) - S(t, s) u is

F-weakly continuous for u E F. If u* E E*, we have

(u*, A(t)S(t, s)u) = (A(t)*u*, S(t, s)u), (7.7.36)

where 4(t)*: E* . F* indicates the adjoint of A(t) (the restriction of A(t)

to F) as an operator from F to E. Hypothesis (e) plainly implies that

t - A(t)* is continuous as a (E*, F*)-valued function, thus (7.7.36) yields

our claim on E-weak continuity of A(t)S(t, s). The statements on E-strong

measurability of S(t, s) as a function of t and s as well as a function of t for

each s fixed follow from this well-known result in integration theory of

vector-valued functions:

7.7.11 Example (Hille-Phillips [1957; 1, p. 73]). Let f be a function

defined (say) in a measurable subset K of n-dimensional space with values

in E. Then, if f is weakly continuous, it is strongly measurable in K.

We note that it also follows from Example 7.7.11 that S is F-strongly

measurable, either as a function of (t, s) or in each variable separately. To

show (7.7.35), we take u* E F*, write the (obviously valid) version of this

equality for S and apply u* to both sides, obtaining

u)+

(0<s<t<T).

(7.7.37)

We have already observed in the proof of Theorem 7.7.5 that

(u(=-F)

(7.7.38)

F-weakly as n - oo. We let n -

oo in (7.7.37) and make use of the strong

convergence of S(t, s)u in E, uniformly with respect to s and t. The result is

(7.7.35), since u* can be chosen at will. It is a consequence of this equality

that DS(t, s)u must exist in a set e = e(s) of full measure in (s, T). Since

D,+S(t, s) u has been seen to exist everywhere and equals A(t)S(t, s) u, the

proof of Lemma 7.7.12 is complete.

The restrictions to the t-differentiability of S in Theorem 7.7.7 can be

(partly) eliminated if the assumptions in Theorem 7.7.5 are reinforced. A

sample result is:

7.7.12 Example (Kato [1970: 1]). Let the hypotheses of Theorem 7.7.5

hold with (c) strengthened as follows:

(c') {A(t)F; 0 < t < T) satisfies the assumptions of Example 7.7.3

with respect to a family of norms {II' II,; 0 < t < T) in F such that each of

these norms is uniformly convex.

436

The Abstract Cauchy Problem for Time-Dependent Equations

Then the conclusions of Theorem 7.7.7 hold with the following

additional statements: if 0 < s < T and u E F, t - S(t, s)u is F-strongly

right continuous in s < t < T and strongly continuous there except in a

countable set e = e(s); D1S(t, s)u exists for t (4 e(s), is continuous for

t 0- e(s) and equals A(t)S(t, s)u.

Finally, we note that the reservations to the t-differentiability of the

solutions of (7.7.1) constructed in Theorem 7.7.5 can be totally lifted in a

case that covers many of the applications of Theorem 7.7.9.

7.7.13 Theorem. Let the assumptions of Theorem 7.7.5 be satisfied

both for(A(t); 0<t<T)and for (A(t)=-A(T-t); 0<t<T). Then the

Cauchy problem for (7.7.1) is well posed in both senses of time (see Section

7.1) in 0 < t < T with respect to the following notion of solution:

(D)

is a solution of (7.7.1) in 0 < t < T if and only if:

(a) is continuously differentiable (in the sense of the norm of E)

andu(t)EFfor0<t<T.

(b) Equation (7.7.1) is ratified in 0 < s < T.

Proof.

The assumption that both

and 4(.) are stable in E

obviously implies (taking t, _ . . . = tn) that each A(t) belongs to C' (C, w).

(We may and will suppose that the stability constants for are the same

as for A(-); a similar assumption will be made for A(.) F and F.)

Accordingly, the solution operator Sn(t, s) of Equation (7.7.19) can now be

constructed in the square 0 < s, t < T using formulas (7.7.21) and (7.7.22)

when s < t and setting

Sn(t,s)=Sn(s,t)-'

(7.7.39)

when s > t, and it obeys inequalities (7.7.25) in the extended range, replac-

ing t - s by I t - s1

. Inequality (7.7.28) becomes

II(SS(t, S)-Sm(t, S))uII

<CCe(m+w)"-sIIIuIIF

f'II "n(o) -4.(o

(F, E)

(7.7.40)

valid for 0 < s, t < T and this inequality can be used to establish strong

E-convergence of S, uniformly in the whole square, and hence E-strong

continuity and the inequality

IIS(t,s)II<CeW1t-s1

(0<s,t<T).

(7.7.41)

Lemma 7.7.8 has an immediate counterpart in the present situation, inequal-

ity (7.7.29) becoming

fIIA+(o)-A(a)II(F,E)dol

(7.7.42)