Everitt B.S. The Cambridge Dictionary of Statistics

Подождите немного. Документ загружается.

Quasi-independence: A form of independence for a

contingency table

, conditional on restricting

attention to a particular part of the table only. For example, in the following table showing

the social class of sons and their fathers, it might be of interest to assess whether, once a

son has moved out of his father’s class, his destination class is independent of that of his

father. This would entail testing whether independence holds in the table after ignoring

the entries in the main diagonal.

Father’s social class Son’s social class

Upper Middle Lower

Upper 588 395 159

Middle 349 714 447

Lower 114 320 411

[The Analysis of Contingency Tables, 2nd edition, 1992, B. S. Everitt, Chapman and

Hall/CRC Press, London.]

Quasi-likelihood: A function, Q, that can be used in place of a conventional

log-likelihood

when

it is not possible (and/or desirable) to make a particular distributional assumption about

the observations, with the consequence that there is insufﬁcient information to construct

the

likelihood

proper. The function depends on assuming some relationship between the

means and variances of the random variables involved, and is given explicitly by

Q ¼

i¼1

ð

; y

where y

; y

; ...; y

represent the observations which are assumed to be independent

with means 

;

; ...;

and variances 

ð

Þ where V

(µ

) is a known function of µ

The components Q

of the function Q are given by



 t



ðtÞ

Q behaves like a log-likelihood function for 

;

; ...;

under very mild assumptions.

[GLM Chapter 8.]

Quasi-score estimating function: See estimating functions.

Quasi -symmetry: A term used to describe a pattern of frequencies or proportions that might be

displayed by a

two-dimensional contingency table

of size 3 × 3 or larger and implies that

the

odds ratios

describing the association structure are symmetric, that is for all i and j

the odds ratio (π

)/((π

) is the same for all values of k . The following is an

example of a 3 × 3 table displaying quasi-symmetry

10 5 15 30

540550

6 2 12 20

21 47 32 100

[Biometrics, 1979, 35, 417–426.]

Quetelet, Adolphe (1796^1874): Born in Ghent, Belgium, Quetelet received the ﬁrst doctorate

of science degree awarded by the new University of Ghent in 1819 for a dissertation on

the theory of conic sections. Later he studied astronomy in Paris and for much of the rest

of his life his principal work was as an astronomer and a meteorologist at the Royal

349

Observatory in Brussels. But a man of great curiosity and energy, Quetelet also built an

international reputation for his work on censuses and population such as birth rates, death

rates, etc. Introduced the concept of the ‘average man’ (‘l’homme moyen’) as a simple

way of summarizing some characteristic of a population and devised a scheme for ﬁtting

normal distributions to grouped data that was essentially equivalent to the use of

normal

probability plot

. Quetelet died on 17 February 1874 in Brussels.

Quetelet index: An index of body mass given by height divided by the square of weight. See

also body mass index .

Queuing theory: A largely mathematical theory concerned with the study of various factors

relating to queues such as the distribution of arrivals, the average time spent in the

queue, etc. Used in medical investigations of waiting times for hospital beds, for example.

[Introduction to Queuing Theory, 1990, R. B. Cooper, North Holland, New York.]

Qu ick a nddirty meth ods: A term once applied to many

distribution free methods

presumably to

highlight their general ease of computation and their imagined inferiority to the corres-

ponding parametric procedure.

Quick-size: A versatile simulation-based tool for determining an exact sample size in essentially any

hypothesis testing situation. It can also be modiﬁed for use in the estimation of

conﬁdence

intervals

. See also nQuery advisor.[The American Statistician, 1999, 53,52–5.]

Fig. 110 The quincunx used by Galton.

350

Quincunx: A device used by

Galton

to illustrate his lectures, which is shown in Fig. 110. It had a

glass face and a funnel at the top. Shot was passed through the funnel and cascaded

through an array of pins, each shot striking one pin at each level and in principle falling

left or right with equal probabilities each time a pin was hit. The shot then collected in

compartments at the bottom. The resulting outline after many shot were dropped should

resemble a normal curve.

Quintiles: The set of four variate values that divide a frequency distribution or a probability distri-

bution into ﬁ ve equal parts.

Quitting illeffect: A problem that occurs most often in studies of smoker cessation where smokers

frequently quit smoking following the onset of disease symptoms or the diagnosis of a life-

threatening disease, thereby creating an anomalous rise in, for example, lung cancer risk

following smoking cessation relative to continuing smokers. Such an increase has been

reported in many studies. [Tobacco Control, 2005, 14,99–105.]

Quota sample: A sample in which the units are not selected at random, but in terms of a certain

number of units in each of a number of categories; for example, 10 men over age 40,

25 women between ages 30 and 35, etc. Widely used in opinion polls. See also sample

survey, stratiﬁed sampling and random sample.[Elements of Sampling Theory, 1974,

V. D. Barnett, English Universities Press, London.]

351

R: An open-source software environment for statistical computing and graphics. The software provides a

wide variety of statistical and graphical techniques and its simple and effective programming

language means that it is relatively simple for users to deﬁne new functions. Full details of the

software are available at http://www.r-project.org/. Many of the ﬁgures in this dictionary have

been produced using R.[Introductory Statistics with R, 2002, P. Dalgaard, Springer, New York.]

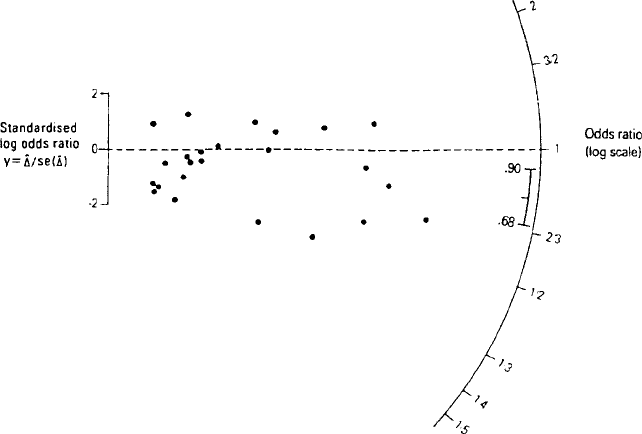
R a dialplot o fodds ra t ios: A diagram used to display the

odds ratios

calculated from a number of

different

clinical trials

of the same treatment(s). Often useful in a

meta-analysis

of the results.

The diagram consists of a plot of y ¼

=SEð

DÞ against x ¼ 1=SEð

DÞ where

D is the

logarithm of the odds ratio from a particular study. An example of such a plot is shown in

Fig. 111. Some features of this display are:

Every estimate has unit standard error in the y direction. The y scale is drawn as a 2

error bar to indicate this explicitly.

The numerical value of an odds ratio can be read off by extrapolating a line from (0,0)

through (x, y) to the circular scale drawn, the horizontal line corresponds to an odds

ratio of one. Also approximate 95% conﬁdence limits can be read off by extrapolating

lines from (0,0) through ðx; y þ2Þ; ðx; y  2Þ respectively.

Points with large SEð

DÞ fall near the origin, while points with small SEð

DÞ, that is the

more informative estimates, fall well away from the origin and naturally look more

informative.

[Statistics in Medicine, 1988, 7, 889–94.]

Radical statistics group: A national network of social scientists in the United Kingdom commit-

ted to the critique of statistics as used in the policy making process. The group is committed

to building the competence of critical citizens in areas such as health and education. [http://

www.radstats.org.uk/]

Raking adjustments: An alternative to

poststratiﬁcation adjustments

in complex surveys that

ensures that the adjusted weights of the respondents conform to each of the marginal

distributions of a number of auxiliary variables that are available. The procedure involves

an iterative adjustment of the weights using an

iterative proportional-ﬁtting algorithm

. The

method is widely used in health and other surveys when many population control totals are

available. For example, raking was used in the 1991 General Social Survey in Canada, a

random digit dialling sampling

telephone survey that concentrated on health issues. In the

survey, province, age and sex control totals were used. [International Statistical Review,

1994, 62, 333 –47.]

Ramsey, Frank Plumpton (1903^1930): Born in Cambridge, England, Ramsey was educated

at Trinity College, Cambridge. A close friend of the Austrian philosopher Ludwig

Wittgenstein, whose Tractatus he translated into English while still an undergraduate.

Ramsey worked on the foundations of probability and died at the tragically early age of

26, from the effects of jaundice.

352

Ramsey regression equation specification error test: A general speciﬁcation test for

linear regression models. Based on the idea that adding squared, cubic, quartic etc. compo-

nents of the predicted responses as covariates should not increase the coefﬁcient of deter-

mination if the model is correctly speciﬁed. [Journal of the Royal Statistical Society, Series

B, 1969, 31, 350–371.]

Rand coefficient: An index for assessing the similarity of alternative classiﬁcations of the same set

of observations. Used most often when comparing the solutions from two methods of

cluster

analysis

applied to the same set of data. The coefﬁcient is given by

R ¼



T 0:5P  0:5Q þ







where

T ¼

i¼1

j¼1

 n

P ¼

i¼1

 n

Q ¼

j¼1

 n

The quantity m

is the number of individuals in common between the ith cluster of the ﬁrst

solution, and the jth cluster of the second (the clusters in the two solutions may each be

labelled arbitrarily from 1 to g, where g is the number of clusters). The terms m

. and m

are

appropriate marginal totals of the matrix of m

values and n is the number of observations.

The coefﬁcient lies in the interval (0,1) and takes its upper limit only when there is complete

agreement between the two classiﬁcations. [MV2 Chapter 10.]

Random: Governed by chance; not completely determined by other factors. Non-deterministic.

Fig. 111 An example of a radial plot of odds ratios.

353

Random allocation: A method for forming treatment and control groups particularly in the context

of a

clinical trial

. Subjects receive the active treatment or placebo on the basis of the outcome

of a chance event, for example, tossing a coin. The method provides an impartial procedure

for allocation of treatments to individuals, free from personal biases, and ensures a ﬁrm

footing for the application of signiﬁcance tests and most of the rest of the statistical method-

ology likely to be used. Additionally the method distributes the effects of

concomitant

variables

, both observed and unobserved, in a statistically acceptable fashion. See also block

randomization, minimization and biased coin method. [SMR Chapter 5.]

Random coefficient models: See multilevel models and growth models.

Random digit dialling sampling: See telephone interview surveys.

Random dissimilarity matrix model: A model for lack of clustering structure in a

dissimilarity

matrix

. The model assumes that the elements of the lower triangle of the dissimilarity matrix

are ranked in random order, all n(n -1)/2! rankings being equally likely. [Classiﬁcation, 2nd

edition, 1999, A. D. Gordon, Chapman and Hall/CRC Press, London.]

Random effects: The effects attributable to a (usually) inﬁnite set of levels of a factor, of

which only a random sample occur in the data. For example, the investigator may want to

accommodate effects of subjects in a

longitudinal study

by introducing subject-speciﬁc

intercepts that are viewed as a random sample from a distribution of effects. See also ﬁxed

effects.

Random effects model: See multilevel models.

Random events: Events which do not have deterministic regularity (observations of them do not

necessarily yield the same outcome) but do possess some degree of statistical regularity

(indicated by the statistical stability of their frequency).

Random forests: An ensemble of classiﬁcation or regression trees (see

classiﬁcation and regression

tree technique

) that have been ﬁtted to the same n observations, but with random weights

obtained by use of the

bootstrap

. Additional randomness is supplied by selecting only a

small fraction of covariates for split point determination in each inner node of these trees.

Final predictions are then obtained by averaging the predictions obtained from each tree in

the forest. Empirical and theoretical investigations have shown that such an aggregation over

multiple tree-structured models helps to improve upon the prediction accuracy of single

trees. [Machine Learning, 2001, 45,5–32.]

R ando m mat rix theo ry: The study of stochastic linear algebra where the equations themselves are

random. Attracting interest in

regularization

of high-dimensional problems in statistics.

[Random Matrix Theory and its Applications, 2009, Z.Bai, Y.Chen and Y.-C. Liang, Eds.,

World Scientiﬁc, Singapore.]

Randomization tests: Procedures for determining statistical signiﬁcance directly from data with-

out recourse to some particular

sampling distribution

. For example, in a study involving the

comparison of two groups, the data would be divided (permuted) repeatedly between groups

and for each division (permutation) the relevant test statistic (for example, a t or F), is

calculated to determine the proportion of the data permutations that provide as large a test

statistic as that associated with the observed data. If that proportion is smaller than some

signiﬁcance level α, the results are signiﬁcant at the α level. [Randomization Tests, 1986,

E. S. Edington, Marcel Dekker, New York.]

Randomized block design: An experimental design in which the treatments in each block are

assigned to the experimental units in random order.

354

R andomized cl i n ical tr ial: See clinical trials.

Randomizedconsentdesign: A design originally introduced to overcome some of the perceived

ethical problems facing clinicians entering patients in

clinical trials

involving random

allocation. After the patient’s eligibility is established the patient is randomized to one of

two treatments A and B. Patients randomized to A are approached for patient consent. They

are asked if they are willing to receive therapy A for their illness. All potential risks, beneﬁts

and treatment options are discussed. If the patient agrees, treatment A is given. If not, the

patient receives treatment B or some other alternative treatment. Those patients randomly

assigned to group B are similarly asked about treatment B, and transferred to an alternative

treatment if consent is not given. See also Zelen’s single-consent design.[Contemperary

Clinical Trials, 2006, 27, 320–332.]

Rand om ized encourag em e nt t ria l:

Clinical trials

in which participants are encouraged to

change their behaviour in a particular way (or not, if they are allocated to the control

condition) but there is little expectation on the behalf of the trial investigators that partic-

ipants will fully comply with the request or that there will not be a substantial minority who

change their behaviour in the required way without actually being asked (by the trial

investigators, at least) to do so. An example involves randomizing expectant mothers

(who are also cigarette smokers) to receive advice to cut down on or completely stop

smoking during pregnancy. Many of the mothers will fail to reduce their smoking even

though they have received encouragement to do so. On the other hand, many will cut down

whether or not they have received the advice suggesting that they should. Randomized

encouragement to take part in cancer screening programmes is another example. Again,

adherence to the offer of screening will be far from complete, but, on the other hand, there

will always be people who will ask for the examination in any case. See also Zelen single-

consent design.[Biometrics, 1989, 4, 619–622.]

Randomized response technique: A procedure for collecting information on sensitive issues

by means of a survey, in which an element of chance is introduced as to what question a

respondent has to answer. In a survey about abortion, for example, a woman might be posed

both the questions ‘have you had an abortion’ and ‘have you never had an abortion ’, and

instructed to respond to one or the other depending on the outcome of a randomizing device

under her control. The response is now not revealing since no one except the respondent is

aware of which question has been answered. Nevertheless the data obtained can be used to

estimate quantities of interest, here, for example, the proportion of women who have had an

abortion (π); if the probability of selecting the question ‘have you had an abortion’, P,is

known and is not equal to 0.5. If y is the proportion of ‘yes’ answers to this question in a

random sample of n respondents, one estimator of π is given by

p ¼

y ð1  PÞ

2P  1

This estimator is unbiased and has variance, V

V ¼

pð1  pÞ

Pð1  PÞ

nð2P  1Þ

[Randomized Response: Theory and Techniques, 1988, A. Chaudhuri and R. Mukerjee,

Marcel Dekker, New York.]

Randomly-stopped sum: The sum of a variable number of random variables.

R ando m model: A model containing random or probabilistic elements. See also deterministic

model.

355

R ando m nu mber: See pseudorandom numbers.

Random sample: Either a set of n independent and identically distributed random variables, or a

sample of n individuals selected from a population in such a way that each sample of the

same size is equally likely.

Ra ndom vari able: A variable, the values of which occur according to some speciﬁed probability

distribution. [KA1 Chapter 1.]

Random variation: The variation in a data set unexplained by identiﬁable sources.

Random walk: The motion of a ‘particle’ that moves in discrete jumps with certain probabilities from

point to point. At its simplest, the particle would start at the point x = k on the x axis at time

t = 0 and at each subsequent time, t =1,2,..., it moves one step to the right or left with

probabilities p and 1 − p, respectively. As a concrete example, the position of the particle

might represent the size of a population of individuals and a step to the left corresponds to a

death and to the right a birth. Here the process would stop if the particle ever reached the

origin, x = 0, which is consequently termed an absorbing barrier. See also birth–death

process, Markov chain and Harris walk. [TMS Chapter 1.]

Range: The difference between the largest and smallest observations in a data set. Often used as an

easy-to-calculate measure of the dispersion in a set of observations but not recommended for

this task because of its sensitivity to

outliers

and the fact that its value increases with sample

size. [SMR Chapter 2.]

Rankadjacency statistic: A statistic used to summarize

autocorrelations

spatial data

. Given by

D ¼

 y

where y

¼ rankðx

Þ, x

is the data value for location i, and the w

are a set of weights

representing some function of distance or contact between regions i and j. The simplest

weighting option is to deﬁne

¼ 1 if regions i and j are adjacent

¼ 0 otherwise

in which case D becomes the average absolute rank difference over all pairs of adjacent

regions.The theoretical distribution of D is not known but spatial clustering (or positive

spatial autocorrelation

) in the data will be reﬂected by the tendency for adjacent data values

to have similar ranks, so that the value of D will tend to be smaller than otherwise. See also

Moran’s I. [Statistics in Medicine, 1993, 12, 1885–94.]

Rank correlation coefficients: Correlation coefﬁcients that depend only on the ranks of the

variables not on their observed values. Examples include

Kendall’s tau statistics

and

Spearman’s rho

. [SMR Chapter 11.]

Ranking: The process of sorting a set of variable values into either ascending or descending order.

[SMR Chapter 2.]

Rank of a matrix: The number of linearly independent rows or columns of a matrix.

Rank order statist ics: Statistics based only on the ranks of the sample observations, for example

Kendall’s tau statistics

Rank regression: A method of regression analysis in which the ranks of the dependent variable are

regressed on a set of explanatory variables. Since the ranks are unaffected by strictly

increasing transformations, this can be formally speciﬁed by the model

356

gðyÞ¼x

β þ

where g is any strictly increasing transformation of the dependent variable y, x is a vector of

explanatory variables and β is a vector of regression coefﬁcients. [Journal of Machine

Learning, 2007, 8, 2727–2754.]

Ranks: The relative positions of the members of a sample with respect to some characteristic. [SMR

Chapter 2.]

Rank transform method: A method consisting of replacing the observations by their ranks in the

combined sample and performing one of the standard

analysis of variance

procedures on the

ranks. [Journal of Statistical Computation and Simulation, 1986, 23, 231–40.]

Rao-Blackwell theorem: A theorem stating that for an unbiased estimator f(x) of a parameter θ,

the conditional expectation of f(x), given a

sufﬁcient statistic

T(X), is typically a better

estimator of θ and never worse. Transforming an estimator using the Rao-Blackwell theorem

is called Rao-Blackwellization.[Theory of Point Estimation, 1998, E. L. Lehmann and

G. Casella, Springer, New York.]

Rao^Hartley^Cochran method (RHC): A simple method of sampling with unequal proba-

bilities without replacement. The method is as follows:

Randomly partition the population of N units into n groups fG

g¼1

of sizes

g¼1

Draw one unit from each group with probability Z

for the gth group, where Z

= x

X, Z

j2G

; x

= some measure of the size of the jth unit and X ¼

j¼1

An unbiased estimator of



Y , the population mean, is



Y ¼

y¼1

where w

¼ f =p

, p

¼ z

, is the probability of selection of the gth sampled unit, f = n/N

is the probability of selection under simple random sampling without replacement, and y

= z

denote the values for the unit selected from the gth group. Note that by deﬁnition,

g¼1

¼ 1. An unbiased estimator of varð



Y Þ is

varð



Y Þ¼

g¼1

 N



g¼1







[Sampling Techniques, 3rd edition, 1977, W. G. Cochran, Wiley, New York.]

Rasch, Georg (1901^19 80): Born in Odense, Denmark Rasch received a degree in mathematics

from the University of Copenhagen in 1925, and then became Doctor of Science in 1930. In

the same year he visited University College in London to work with

Fisher

. On returning to

Denmark he founded the Biostatistics Department of the State Serum Institute and was the

Director from 1940 to 1956. Developed

latent trait models

including the one named after

him. He died on 19 October 1980, in Byrum, Denmark.

Rasch model: A model often used in ability testing. The model proposes that the probability that an

examinee i with ability quantiﬁed by a parameter, δ

, answers item j correctly (X

=1)is

given by

PðX

¼ 1j

;

Þ¼



=ð1 þ



357

where 

is a measure of the simplicity of the item. This probability is commonly rewritten in

the form

PðX

¼ 1j

;

Þ¼

expð

 

1 þexpð

 

where expð

Þ¼

and expð

Þ¼

. In this version, θ

is still called the ability parameter

but σ

is now termed the item difﬁculty.[Rasch Models: Foundations, Recent Developments

and Applications, 1995, G. H. Fischer and I. W. Molenaar, editors, Springer, New York.]

Ratchet scan statistic: A statistic used in investigations attempting to detect a relatively sharp

increase in disease incidence for a season superimposed on a constant incidence over the

entire year. If n

; n

; ...; n

are the total number of observations in January, February,...,

December, respectively (months are assumed to be of equal lengths), then the sum of k

consecutive months starting with month i can be written as

ðiþk1Þmod12

j¼1

The ratchet scan statistic is based on the maximum number falling within k consecutive

months and is deﬁned as

¼ max

i¼1;...;12

Under the hypothesis of a

uniform distribution

of events, i.e. the expected value of n

is N/12

for all i ¼ 1; 2; ...; 12, where N=the total number of events in the year, it can be shown that

PðT

 nÞ¼

i¼1

where the sum is taken over all the values of ðn

; n

; ...; n

Þ that yield T

 n.

Approximations for the distribution for k = 1 have been derived but not for k >1.

[Biometrics, 1992, 48, 1177–85.]

Rate: A measure of the frequency per unit time of some phenomenon of interest given by

Rate ¼

number of events in specified period

average population during the period

(The resulting value is often multiplied by some power of ten to convert it to a whole number.)