Escher J., Guidotti P., Hieber M. et al. (editors) Parabolic Problems: The Herbert Amann Festschrift

Подождите немного. Документ загружается.

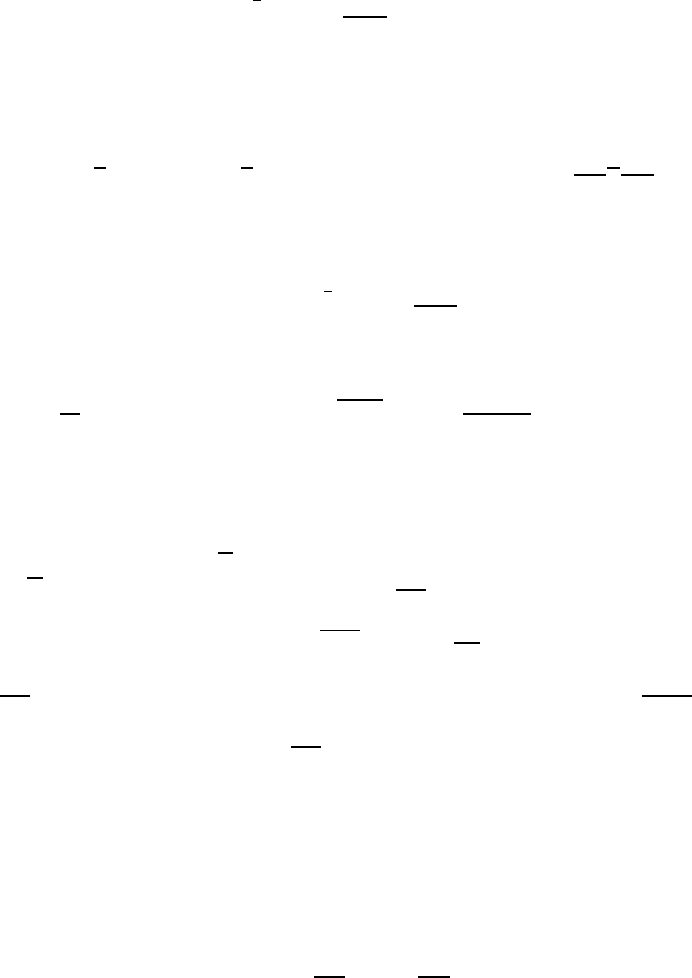
128 R. Cross, A. Favini and Y. Yakubov

Therefore, the gradient estimate reads





m(x)|u



(x)|



≤ C|λ|

−

2(2−ρ)

f

,λ∈

, |λ|≥1, (4.18)

extending the well-known result for the regular case (see [10, Theorem 3.1.3]).

This argument extends to an arbitrary bounded domain Ω ∈ R

with the

smooth boundary ∂Ω, in view of Green’s formula (and u =0on∂Ω)



mΔuudx = −



∇(mu) •∇udx = −



m|∇u|

dx −

j=1



∂m

∂x

∂u

∂x

dx,

where m satisﬁes (4.6). So, if (λm(x) − Δ+c(x))u = f ∈ L

(Ω), u =0on∂Ω(as

(4.12)–(4.13)) then, similarly to (4.18), for suﬃciently large λ ∈





m(x)|∇u(x)|



≤ C|λ|

−

2(2−ρ)

f

(Ω)

. (4.19)

As a consequence, consider the initial boundary value problem

∂

∂t

(m(x)u(x, t)) = Δu(x, t)+



m(x)

i=1

(x)

∂u(x, t)

∂x

− c(x)u(x, t)

+ f(x, t), (x, t) ∈ Ω × (0,τ], (4.20)

u(x, t)=0, (x, t) ∈ ∂Ω × (0,τ], (4.21)

m(x)u(x, 0) = v

(x),x∈ Ω. (4.22)

Suppose that m, a

∈ C

(Ω), (4.6) is satisﬁed, c is a measurable bounded function

on Ω, c(x) ≥ δ>0, f ∈ C

([0,τ]; L

(Ω)),

1−ρ

2−ρ

<σ≤ 1, and v

∈ L

(Ω). In

X := L

(Ω), consider the operator Mu := m(·)u, D(M):=X, Lu := Δu − c(·)u,

D(L):=H

(Ω) ∩ H

(Ω), L

u :=



m(·)

i=1

(·)

∂u

∂x

, D(L

):=H

(Ω). Then,

from (4.7) (which is true in

), we get condition 1 of Theorem 3.2 (with η =

2−ρ

) and, from (4.19), we get the estimate in Remark 3.3 (with σ =

2(2−ρ)

So, the conclusion of Theorem 3.2 for the operator L + L

is true. Hence, by [6,

Theorem 3.8] (with α =1,β =

2−ρ

, γ>0 big enough), problem (4.20)–(4.22)

admits a unique strict solution u(x, t), i.e., m(x)u ∈ C

((0,τ]; L

(Ω)), (L + L

)u ∈

C((0,τ]; L

(Ω)), u satisﬁes (4.20)–(4.21), and condition (4.22) is understood in the

seminorm sense m(L + L

)

−1

(mu −v

)

(Ω)

→ 0, as t → 0

Example 7. In the last example, we use the result in [3, Theorem 4.4]. Given an

unbounded domain Ω in R

, n ≥ 2, with a boundary ∂ΩofclassC

and an

admissible domain according to [3, Deﬁnition 4.1]. We consider the linear second-

order diﬀerential expression in divergence form

A(x, D

i,j=1

∂

∂x



(x)

∂

∂x



−a

(x)

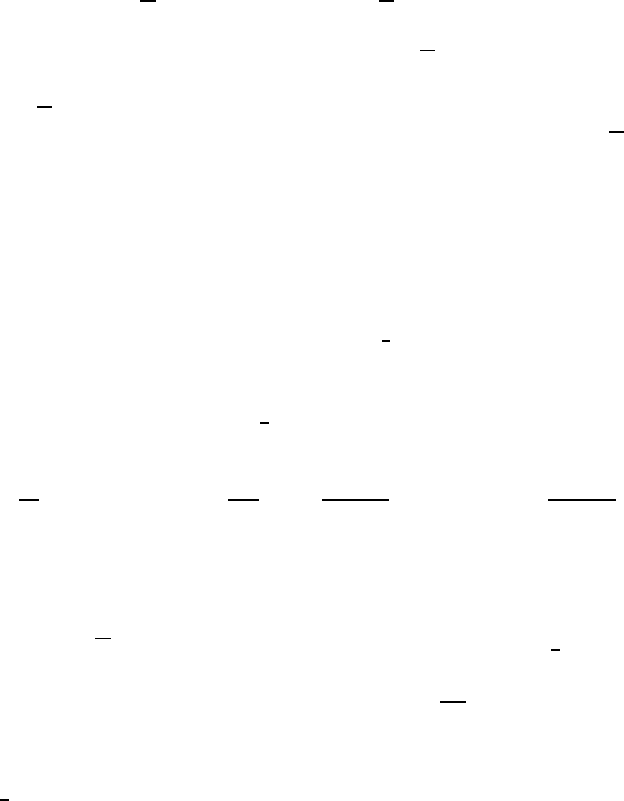
Perturbation Results for Multivalued Linear Operators 129

with a

= a

∈ C

(Ω), i, j =1,...,n, a

∈ C

(Ω), a

≥ ν

> 0, ∃ν

> 0,

|ξ|

≤

i,j=1

(x)ξ

≤ ν

|ξ|

, ∀(x, ξ) ∈ Ω ×R

, ∃ν

,ν

> 0,

m ∈ C

(Ω) is non-negative and |∇m(x)|≤Km(x), (A(x, D

),m) is an admissible

pair according to [3, Deﬁnition 4.2]. Note that m = e

,wherev ∈ C

0,1

(Ω) and

sup

x∈Ω

v(x) < +∞ satisﬁes the assumptions above.

According to the quoted [3, Theorem 4.4], for all p ∈ (1, +∞)thereexists

≥ 0 such that if λ ∈ ω

+Σ

, where again Σ

:= {λ ∈ C : eλ ≥−c(1 +

|mλ|)} for some c>0, the spectral equation λm(x)u−A(x, D

)u = f, f ∈ L

(Ω),

admits a unique solution u ∈ W

2,p

(Ω) ∩ W

1,p

(Ω) satisfying the estimates

mu

(Ω)

≤ C|λ|

−β

f

(Ω)

, (4.23)

m|∇u|

(Ω)

≤ C|λ|

−β+

f

(Ω)

, (4.24)

where

β =



1, if p ∈ (1, 2],

, if p ∈ [2, +∞).

Consider now the following initial boundary value problem

∂

∂t

(m(x)u(x, t)) =

i,j=1

∂

∂x

(x)

∂u(x, t)

∂x

)+m(x)

i=1

(x)

∂u(x, t)

∂x

− a

(x)u(x, t)+f (x, t), (x, t) ∈ Ω × (0,τ], (4.25)

u(x, t)=0, (x, t) ∈ ∂Ω × (0,τ], (4.26)

m(x)u(x, t)=v

(x),x∈ Ω, (4.27)

where a

∈ C

(Ω). Moreover, assume 1 <p<4(sothat1≥ β>

). Take X =

(Ω) and denote D(M):=X, Mu := m(·)u, D(L):=W

2,p

(Ω) ∩W

1,p

(Ω), Lu :=

A(x, D

)u, D(L

):=W

1,p

(Ω), L

u := m(·)

i=1

(·)

∂u

∂x

. Then, the inequalities

(4.23) and (4.24) provide the conclusion of Theorem 3.2 for the operator L + L

More precisely, the inequality (4.23) implies condition 1 of Theorem 3.2 (with

η = β) and the inequality (4.24) implies the estimate in Remark 3.3 (with σ =

β −

). Therefore, using [6, Theorem 3.8] (with α =1,β = β, γ = ω

), we get the

following result. For all f ∈ C

([0,τ]; L

(Ω)), 1 −β<σ≤ 1, v

∈ L

(Ω), problem

(4.25)–(4.27) has a unique strict solution u(x, t), i.e., m(x)u ∈ C

((0,τ]; L

(Ω)),

(L + L

)u ∈ C((0,τ]; L

(Ω)), u satisﬁes (4.25)–(4.26), and (4.27) is satisﬁed in the

seminorm sense m(L + L

)

−1

(mu −v

)

(Ω)

→ 0, as t → 0

References

[1] R. Cross, Multivalued Linear Operators, Marcel Dekker, Inc., 1998.

[2] H.O. Fattorini, The Cauchy Problem, Encyclopedia of Mathematics and its Appli-

cation, Addison Wesley, London, 1983.

130 R. Cross, A. Favini and Y. Yakubov

[3] A. Favaron and A. Lorenzi, Gradient estimates for solutions of parabolic diﬀerential

equations degenerating at inﬁnity, Advances Diﬀ. Eqs., (4) 12 (2007), 435–460.

[4] A. Favini, A. Lorenzi, H. Tanabe, and A. Yagi, An L

-approach to singular linear

parabolic equations in bounded domains, Osaka J. Math., 42 (2005), 385–406.

[5] A. Favini, A. Lorenzi, H. Tanabe, and A. Yagi, An L

-approach to singular lin-

ear parabolic equations with lower order terms, Discrete and Continuous Dynamical

Systems, (4) 22 (2008), 989–1008.

[6] A. Favini and A. Yagi, Degenerate Diﬀerential Equations in Banach Spaces,Marcel

Dekker, Inc., New York, USA, 1999.

[7] A. Favini and A. Yagi, Quasilinear degenerate evolution equations in Banach spaces,

J. Evol. Eqs., 4 (2004), 421–449.

[8] A. Favini and A. Yagi, Multivalued linear operators and degenerate evolution equa-

tions, Ann. Mat. Pura Appl., (4) 163 (1993), 353–384.

[9] M. Haase, The Functional Calculus for Sectorial Operators,Birkh¨auser Verlag, 2006.

[10] A. Lunardi, Analytic Semigroups and Optimal Regularity in Parabolic Problems,

Birkh¨auser Verlag, Boston, 1995.

[11] I.V. Melnikova and A. Filinkov, Abstract Cauchy Problems: Three Approaches, Chap-

man & Hall/CRC, Boca Raton, USA, 2001.

[12] S. Yakubov and Ya. Yakubov, Diﬀerential-Operator Equations. Ordinary and Partial

Diﬀerential Equations, Chapman & Hall/CRC, Boca Raton, USA, 2000.

Ronald Cross

Department of Mathematics and Applied Mathematics

University of Cape Town

Rondebosch 7700, South Africa

e-mail: ronald.c@mweb.co.za

Angelo Favini

Dipartimento di Matematica

Universit`a di Bologna

Piazza di Porta S. Donato, 5

I-40126 Bologna, Italy

e-mail: favini@dm.unibo.it

Yakov Yakubov

Raymond and Beverly Sackler School of Mathematical Sciences

Tel-Aviv University

Tel-Aviv 69978, Israel

e-mail: yakubov@post.tau.ac.il

Progress in Nonlinear Diﬀerential Equations

and Their Applications, Vol. 80, 131–166

 2011 Springer Basel AG

Semilinear Stochastic Integral Equations in L

Wolfgang Desch and Stig-Olof Londen

Dedicated to Herbert Amann on the oc casion of his 70th birthday

Abstract. We consider a semilinear parabolic stochastic integral equation

u(t, ω, x)=Aa

∗ u(t, ω, x)+

∞

k=1

G

(t, ω, u(t, ω, ·))(x)

+ a

∗ F (t, ω, u(t, ω, ·))(x)+u

(ω,x)+tu

(ω, x).

Here t ∈ [0,T], ω in a probability space Ω, x in a σ-ﬁnite measure space B with

(positive) measure Λ. The kernels a

(t) are multiples of t

μ−1

. The operator

A : D(A) ⊂ L

(B) → L

(B) is such that (−A) is a nonnegative operator.

The convolution integrals a

G

are stochastic convolutions with respect

to independent scalar Wiener processes w

. F :[0,T] × Ω ×D((−A)

) →

(B)andG :[0,T] × Ω ×D((−A)

) → L

(B,l

) are nonlinear with suitable

Lipschitz conditions.

We establish an L

-theory for this equation, including existence and

uniqueness of solutions, and regularity results in terms of fractional powers of

(−A) and fractional derivatives in time.

Mathematics Subject Classiﬁcation (2000). 60H15, 60H20, 45N05.

Keywords. Semilinear stochastic integral equations, stochastic fractional dif-

ferential equation, regularity, nonnegative operator, Volterra equation, singu-

lar kernel.

1. Introduction

Since our equation (i.e., (1.1) below) reads somewhat complicated and involved,

let us start with a casual motivation. The setting of spaces, domains and operators

will be given more precisely as soon as we state (1.1). The prototype of an equation

for modelling diﬀusion processes is the heat equation

∂

∂t

u(t, x)=Δu(t, x)+f(t, x).

132 W. Desch and S.-O. Londen

The operator Δ, as usual, denotes the Laplacian in space. Instead of the source

term f(t, x) one might consider white noise. Then the equation turns into the

stochastic heat equation

∂

∂t

u(t, x, ω)=Δu(t, ω, x)+g(t, ω, x)

Here, typically, W

is standard Brownian motion, although fractional Brownian

motion has also been considered ([2]). There is an abundance of literature on the

stochastic heat equation and its generalizations. Frequently Δ is replaced by a

general elliptic partial diﬀerential operator or even an abstract operator A (where

(−A) is a positive operator), in Hilbert space as well as (more recently) in L

general Banach spaces. The key issues in handling such equations are stochastic

integration in Banach spaces, and exploiting the regularity properties of parabolic

partial diﬀerential equations.

Diﬀusion according to the heat equation, i.e., according to Fick’s law, has the

property of exponentially decaying memory in time. However, many processes in

physics, chemistry and ﬁnance exhibit time memory decaying only at a power law

rather than exponentially. For a survey of such phenomena see [19, Chapter 8].

Such anomalous diﬀusion is frequently referred to as subdiﬀusion or superdiﬀu-

sion, depending on whether the net motion of particles happens more slowly or

quickly than random diﬀusion according to Fick’s law. Anomalous diﬀusion can

be modelled by a fractional diﬀerential equation

u(t, x)=Δu(t, x)+f(t, x).

Here, D

means the fractional derivative of order α ∈ (0, 2) with respect to time.

The case α<1 describes subdiﬀusion, while α>1 corresponds to superdiﬀu-

sion. The limiting case α = 2, of course, yields the wave equation. Deterministic

fractional diﬀerential – partial diﬀerential equations with parabolic diﬀerential op-

erators in space and fractional derivatives in time have been subject to thorough

investigations, both from theory and applications ([19], [24], [25]). Notice that the

equation may be integrated to give

u(t, x)=u

(x)+



Γ(α)

(t −s)

α−1

Δu(s, x) ds +



Γ(α)

(t −s)

α−1

f(s, x) ds.

(If α>1, we need an additional term tu

(x) to take care of the initial condition

u(0) = u

.) This equation can be considered as a parabolic abstract evolutionary

equation. An extensive theory is available to treat such equations ([29]).

Again, the source term can be replaced by a stochastic additive perturbation

of the system. The equation now turns into

u(t, ω, x)=Δu(t, ω, x)+D

β−α

g(t, ω, x)

Here W

is Brownian motion, but the introduction of the fractional derivative or

fractional integral D

β−α

allows us to model smoother (β<α) or rougher (β>α)

stochastic perturbation. To our knowledge, the ﬁrst attempt to tackle this equation

in L

with p =2wasmadein[11]andextendedin[13].

Stochastic Integral Equations 133

In this paper we go a step further and investigate state dependent forcing,

i.e., semilinear equations. The prototype of the equation to be treated here is

u(t, ω, x)=Δu(t, ω, x)+G(t, u(t, ω, x))

(ω)+F (t, u(t, ω, x)).

We treat semilinear feedback on the stochastic (volatility) term as well as on the

deterministic forcing (drift). Semilinear stochastic heat equations (with

instead

of the fractional derivative D

) in spaces more general than than Hilbert spaces

have been recently in the center of interest of several research groups, just to

mention a few, we refer to [21], as well as work based on abstract stochastic

integration in Banach spaces like [4], [27], [38] and others. To our knowledge, the

present paper is the ﬁrst attempt to deal with the fractional derivative case in L

with p =2.

One of our central tasks is to balance space and time regularity. To have as

much freedom as possible, we put again fractional integrals in front of all forcing

terms:

u(t, ω, x)=Δu(t, ω, x)+D

β−α

G(t, ω, u(t, ω, x))

+ D

γ−α

F (t, ω, u(t, ω, x)).

Regularity in space will be expressed by fractional powers of (−Δ). In integrated

form and full generality, our equation ﬁnally reads:

u(t, ω, x)=A



(t −s)u(s, ω, x) ds

∞

k=1



(t −s)G

(s, ω, u(s, ω, ·))(x) dw



(t − s)F (s, ω, u(s, ω, ·))(x) ds + u

(ω,x)+tu

(ω,x).

(1.1)

The real scalar-valued solution u(t, ω, x) depends on t ∈ [0,T], ω in a probability

space Ω, and x in a measure space B. The convolution kernels a

are deﬁned by

(t):=

Γ(μ)

μ−1

. (1.2)

We assume α ∈ (0, 2), β>

,andγ>0. While the parameter α is the order

ofthefractionaltimederivative,theparameterβ regulates the time regularity

of the stochastic semilinear feedback, and γ regulates the time regularity of the

deterministic feedback. The operator A : D(A) ⊂ L

(B; R) → L

(B; R)(with2≤

p<∞) is such that (−A) is a nonnegative linear operator (see Section 2 below).

In particular we have in mind elliptic partial diﬀerential operators on a suﬃciently

smooth (bounded or unbounded) domain B ⊂ R

, but formally we require only

that (−A) is sectorial and the state space is an L

-space on some measure space B.

The processes w

are scalar-valued, independent Wiener processes. F and G

are

nonlinear and satisfy suitable Lipschitz estimates with respect to u. The functions

and u

are given initial data. For the precise conditions, see Section 3.

134 W. Desch and S.-O. Londen

Our goal is to establish existence and uniqueness of solutions for the semilin-

ear equation (1.1) in an L

-framework with p ∈ [2, ∞). Regularity results will be

stated in terms of fractional powers of −A (for spatial regularity) and fractional

time integrals and derivatives as well as H¨older continuity (for time regularity).

Technically we rely primarily on results concerning a linear integral equation

where the forcing terms F and G are replaced by functions independent of u, i.e.,

(5.1). In recent work [13] we have developed an L

-theory for (5.1), albeit without

the deterministic part and without the u

-term. These results need, however, – for

the purpose of analyzing (1.1) – to be extended and to be made more precise.

Our linear results build on an approach due to Krylov, developed for para-

bolic stochastic partial diﬀerential equations. This approach uses the Burkholder-

Davis-Gundy inequality and estimates on the solution and on its spatial gradient.

To analyze the integral equation (5.1) we combine Krylov’s approach with trans-

formation techniques and estimates involving both fractional powers of −A,and

fractional time-derivatives (integrals) of the solution. Krylov’s approach is very

eﬃcient in obtaining maximal regularity, however, it relies on a highly nontrivial

Paley-Littlewood inequality [20]. A counterpart of this estimate can be given for

general sectorial A by straightforward estimates on the Dunford integral, when we

allow for an inﬁnitesimal loss of regularity.

We also include results for the deterministic convolution and for the u

-term.

Obviously, no originality is claimed for these results.

To obtain result on the semilinear equation (1.1) we combine our linear theory

with a standard contraction approach.

The paper is organized as follows: Before we can state our main results, we

need to collect some facts about sectorial operators and fractional diﬀerentiation

and integration in Section 2. Section 3 states the hypotheses and results for the

semilinear equation. In Section 4 we provide the tools to deﬁne a stochastic in-

tegral and a stochastic convolution in L

-spaces. The central part of this section

is an application of the Burkholder-Davis-Gundy inequality to lift scalar-valued

Ito-integrals to stochastic integrals in L

. This approach is adapted from [21]. Sec-

tion 5 deals with the linear fractional diﬀerential equation. In the beginning we give

the results on existence and regularity which are basic to obtain similar results on

the semilinear equation. We construct the solution via the resolvent operator and

a variation of parameters formula. The contribution of the initial data and of the

forcing F , which enters as a Lebesgue integral, are well known ([29], [39]). The con-

tribution of the stochastic integral containing G is handled by a recent result [13].

We collect these results in a uniﬁed way to allow a comparison of the various re-

quirements on regularity. In Section 6 we arrive at the proof of our main results on

the semilinear equation by a standard contraction procedure. In Section 7 we make

some comments on available maximal regularity results for the linear equation and

their implications for the semilinear equation. Finally, in Section 8 we compare

our results to some recent results on parabolic stochastic diﬀerential equations ob-

tained recently using an abstract theory of stochastic integration in Banach spaces.

Stochastic Integral Equations 135

2. Fractional powers and fractional derivatives

In this paper A : D(A) ⊂ L

(B; R) → L

(B; R) will be a linear operator such

that (−A) is nonnegative. Here p ∈ [2, ∞), but ﬁxed. Regularity in space will be

expressed in terms of the fractional powers (−A)

of A. Regularity in time will be

expressed in terms of fractional time derivatives D

f. In corollaries we will also

give regularity results in terms of the function spaces h

0→0

([0,T]; X), i.e., the little

H¨older-continuous functions with f(0) = 0.

In this section we summarize brieﬂy the deﬁnitions and some known results

about nonnegative operators, their fractional powers, and about fractional inte-

gration and diﬀerentiation.

Let X be a complex Banach space and let L(X) be the space of bounded

linear operators on X.LetB be a closed, linear map of D(B) ⊂ X into X.The

operator −B is said to be nonnegative if ρ(B), the resolvent set of B,contains

(0, ∞), and

sup

λ>0

λ(λI − B)

−1



L(X)

< ∞.

An operator is positive if it is nonnegative and, in addition, 0 ∈ ρ(B). For ω ∈

[0,π), we deﬁne

:= {λ ∈ C \{0}||arg λ| <ω}.

Recall that if (−B) is nonnegative, then there exists a number η ∈ (0,π) such that

ρ(B) ⊃ Σ

,and

sup

λ∈Σ

λ(λI − B)

−1



L(X)

< ∞. (2.1)

The spectral angle of (−B) is deﬁned by

(−B)

:= inf



ω ∈ (0,π] | ρ(B) ⊃ Σ

π−ω

, sup

λ∈Σ

π−ω

λ(λI − B)

−1



L(X)

< ∞



We will rely on the concept of fractional powers of (−B): Let (−B)bea

densely deﬁned nonnegative linear operator on X,andθ>0. If (−B)isposi-

tive, then (−B)

−1

is a bounded operator, and (−B)

−θ

can be deﬁned by integral

formulas [5, Ch. 3] or [22, Section 2.2.2]. As usual,

(−B)

:= ((−B)

−θ

)

−1

,θ>0. (2.2)

If (−B) is nonnegative with 0 ∈ σ(−B), we proceed as in [5, Ch. 5]: Since (−B+I)

is a positive operator if >0, its fractional power (−B + I)

is well deﬁned

according to (2.2). We deﬁne

D(((−B)

)) :=



y ∈

0<≤

D(((−B + I)

)) | lim

→0+

(−B + I)

y exists



, (2.3)

(−B)

y := lim

→0+

(−B + I)

y for y ∈D(((−B)

)). (2.4)

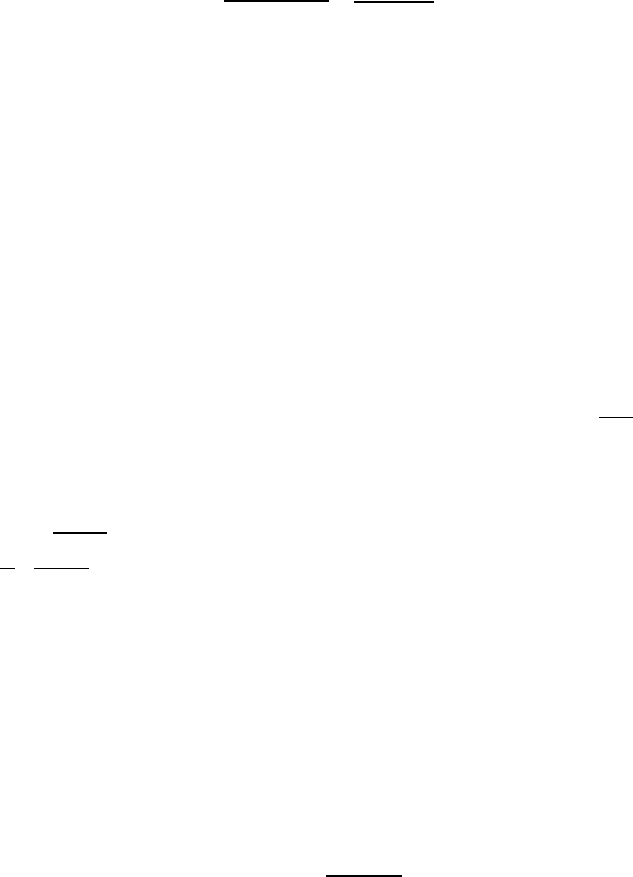
136 W. Desch and S.-O. Londen

Lemma 2.1. Let −B be a nonnegative linear operator on a Banach space X with

spectral angle φ

(−B)

,andletθ>0.

1) (−B)

is closed and D(((−B)

)) = D((−B)).

2) Assume that θφ

(−B)

<π.Then(−B)

is nonnegative and has spectral angle

θφ

(−B)

Proof. For (1) see [5, p. 109, 142], also [8, Theorem 10]. For (2) see [5, p. 123]. 

Lemma 2.2. Let −B be a nonnegative linear operator on a Banach space X with

spectral angle φ

(−B)

. Then for η ∈ [0,π− φ

(−B)

)

sup

|arg μ|≤η, μ=0

(−B)

1−θ

(μI − B)

−1



L(X)

< ∞. (2.5)

Proof. In case η = 0, see [5, Th. 6.1.1, p. 141]. The general case can be reduced to

the case μ>0, [15, p.314]. See also [13, Lemma 3.3]. 

We turn now to fractional diﬀerentiation and integration in time:

Deﬁnition 2.3. Let X be a Banach space and α ∈ (0, 1), let u ∈ L

((0,T); X)for

some T>0.

1) Fractional integration in time of order α is deﬁned by D

−α

u :=

Γ(α)

α−1

∗u.

2) We say that u has a fractional derivative of order α>0providedu = D

−α

for some f ∈ L

((0,T); X). If this is the case, we write D

u = f.

Remark 2.4. Suppose that u has a fractional derivative of order α ∈ (0, 1).

Then

Γ(1−α)

−α

∗ u is diﬀerentiable a.e. and absolutely continuous with D

u =



Γ(1−α)

−α

∗ u



For the equivalence of fractional derivatives in L

and fractional powers of

the realization of the derivative in L

, we have the following lemma.

Lemma 2.5. [9, Prop.2] Let p ∈ [1, ∞), X a Banach space and deﬁne

D(L):={u ∈ W

1,p

((0,T); X) | u(0) = 0},Lu= u



for u ∈D(L).

Then, with β ∈ (0, 1),

u = D

u, u ∈D(L

), (2.6)

where D(L

) coincides with the set of functions u having a fractional derivative

in L

, i.e.,

D(L

)={u ∈ L

((0,T); X) |

Γ(1 − β)

−β

∗ u ∈ W

1,p

((0,T); X)}.

In particular, D

is closed.

We refer to [9] for further properties of the operator D

Stochastic Integral Equations 137

3. The main result

Hypothesis 3.1. Let (B,A, Λ) be a σ-ﬁnite measure space and ﬁx 2 ≤ p<∞.Let

(−A):D(A) ⊂ L

(B; R) → L

(B; R) be a nonnegative linear operator with spec-

tral angle φ

(−A)

,andsuchthatD(A) ∩L

(B; R) ∩L

∞

(B; R)isdenseinL

(B; R).

Hypothesis 3.2. Let (Ω, F, P) be a probability space with an increasing, right

continuous ﬁltration {F

| t ≥ 0} satisfying F

⊂Ffor all t ≥ 0. Let P denote

the predictable σ-algebra on [0, ∞) ×Ω generated by {F

}, and assume that {w

k =1, 2, 3,...} is an independent family of (scalar-valued) F

-adapted Wiener

processes on (Ω, F, P).

Remark 3.3. On [0,T] ×Ω, measurability will always be understood with respect

to the predictable σ-algebra P, and the product measure of the Lebesgue measure

on [0,T]andP.

Hypothesis 3.4. For suitable θ ∈ [0, 1) and  ∈ [0, 1), the function

F :[0,T] × Ω ×D((−A)

) →D((−A)



)

satisﬁes the following assumptions:

(a) For ﬁxed u ∈D((−A)

), the function F (·, ·,u) is measurable from [0,T] × Ω

into D((−A)



(b) There exists a constant M

> 0, such that for all t ∈ [0,T], and all u

∈

D((−A)

) the following Lipschitz estimate holds

F (t, ω, u

) −F (t, ω, u

)

D((−A)



)

≤ M

u

−u



D((−A)

)

for a.e. ω ∈ Ω. (3.1)



F (t, ω, 0)

D((−A)



)

dt dP

1/p

= M

F,0

< ∞. (3.2)

Hypothesis 3.5. For the same θ ∈ [0, 1) as in Hypothesis 3.4, the function

G :[0,T] ×Ω ×D((−A)

) → L

(B; l

)

[G(t, ω, u)](x):=



(t, ω, u)(x)



∞

k=1

satisﬁes the following assumptions:

(a) For ﬁxed u ∈D((−A)

), the function G(·, ·,u) is measurable from [0,T] × Ω

into L

(B; l

(b) There exists a constant M

> 0, such that for all t ∈ [0,T], and all u

∈

D((−A)

) the following Lipschitz estimate holds:

G(t, ω, u

) −G(t, ω, u

)

(B;l

)

≤ M

u

− u



D((−A)

)

for a.e. ω ∈ Ω. (3.3)



G(t, ω, 0)

(B;l

)

dt dP

1/p

= M

G,0

< ∞. (3.4)