Dunajski M. Solitons, Instantons, and Twistors

Подождите немного. Документ загружается.

86 5: Lagrangian formalism and ﬁeld theory

The variation δq is deﬁned, for each ﬁxed t,by

δq =

s=0

The particle will follow a trajectory for which the action is stationary, that is,

δS =0.

Integration by parts shows that this condition leads to the Euler–Lagrange

equations: The variation of the action

δS =

s=0





∂ L

∂q

δq

∂ L

∂



vanishes if the Euler–Lagrange equations

∂ L

∂

−

∂ L

∂q

= 0 (5.1.3)

hold, as δq

vanishes on the boundary of [t

, t

The Euler–Lagrange equations are usually non-linear and exact solutions

are difﬁcult (or impossible) to obtain. In some cases linearization leads to

satisfactory approximate solutions. To ﬁnd these one chooses an equilibrium

position, that is, a point q

∈ X such that

∂U

∂q

q=q

=0,

and expands U around this equilibrium neglecting terms of order higher

than 2

U = const +

, where b

∂

∂q

q=q

The symmetric quadratic form b can be diagonalized, and the system under-

goes small oscillations with frequencies given by the eigenvalues of b.

Most problems treated in this book owe their interesting physical and

mathematical properties to the non-linearity of the underlying equations, and

resorting to the method of small oscillation is not appropriate. There is a less

well-known alternative: Consider a particle in R

n+1

with the Lagrangian (5.1.2)

where U : R

n+1

→ R is a potential whose minimum value is 0. The equilibrium

positions are on a subspace X ⊂ R

n+1

given by U = 0. If the kinetic energy of

the particle is small, and the initial velocity is tangent to X, the exact motion

will be approximated by a motion on X with the Lagrangian L



given by a

restriction of L to X



˙γ

, r, s =1,...,dim X. (5.1.4)

5.1 A variational principle 87

Here, the γ ’s are local coordinates on X, and the metric h = h

(γ )dγ

dγ

induced on X from the Euclidean inner product on R

n+1

. The Euler–Lagrange

equations for (5.1.4) are

¨γ

+ 

˙γ

=0, (5.1.5)

where the functions 

= 

(γ ) are the Chrisoffel symbols of the Levi-Civita

connection of h (given by (9.2.5)). If, for example, U =(1−r

)

, where r = |q|,

then the motion with small energy is approximated by the motion on the unit

sphere in R

n+1

where trajectories are great circles, that is, a circular motion at

r = 1 with constant speed. The true motion will have small oscillations in the

direction transverse to X, with the approximation becoming exact at the limit

of zero initial velocity [142].

In Arnold’s treatment [5] of constrained mechanical systems the constraints

are replaced by a potential which becomes large away from the surface of

constraints. The method leading to (5.1.4) does the converse: A slow motion

in a potential becomes a free motion on the manifold of constraints.

5.1.1 Legendre transform

Given a conﬁguration space X, and a Lagrangian L : TX−→ R, deﬁne n

functions, called conjugate momenta, by

∂ L

∂

We will assume that (q, p) can be used as coordinates in place of (q,

q). The

Hamiltonian H = H(p, q, t) is then deﬁned by the Legendre transform

H(q, p, t)=p

− L(q,

q, t) (5.1.6)

where in the above formula

q must be expressed in terms of ( p, q), and we

assume that the Lagrangian can explicitly depend on t. Comparing the two

differentials

dH =

∂ H

∂p

∂ H

∂q

∂ H

∂t

dt = d

− L(q,

q, t)

−

∂ L

∂q

−

∂ L

∂t

leads to

∂ H

∂t

= −

∂ L

∂t

, (5.1.7)

and the Hamilton canonical equations (1.1.2) where in this chapter we use

upper indices and lower indices for position and momenta, respectively.

88 5: Lagrangian formalism and ﬁeld theory

The reader will have noticed that we have abused the notation. In general

∂(H + L)/∂t = 0 despite that (5.1.7) suggests otherwise. The apparent paradox

(which Nick Woodhouse calls the second fundamental confusion of calculus

[187]) serves as a warning. The meaning of ∂/∂t in (5.1.7) depends on what

variables we hold ﬁxed. These variables are different in the Lagrangian and

Hamiltonian formulations, although the time coordinate t is unchanged by the

Legendre transform.

5.1.2 Symplectic structures

In Section 1.3 we considered Poisson structures as a general arena for the

Hamiltonian formalism. Here we shall concentrate on symplectic structures

which arise as special cases of Poisson structures.

A symplectic manifold is a smooth manifold M of dimension 2n with a

closed two-form ω ∈ 

(M) which is non-degenerate at each point, that is,

= 0. The symplectic two-form restricted to a point in M gives an isomor-

phism between the tangent and cotangent spaces given by

V −→ V

ω,

where V is a vector ﬁeld, and

denotes a contraction of a differential form

with a vector ﬁeld. Using index notation (V

ω)

= V

. In particular a

function f on M gives rise to a Hamiltonian vector ﬁeld X

given by

ω = −df. (5.1.8)

The Poisson bracket (1.3.8) of two functions f, g can be deﬁned as

{ f, g} = X

( f )=ω(X

, X

)=−{g, f },

where ω(X

, X

)=X

ω). It automatically satisﬁes the Jacobi

identity as

0=dω(X

, X

)={ f, {g, k}} + {g, {k, f }} + {k, {f, g}}.

It also satisﬁes

, X

]=−X

{ f,g}

which follows from calculating the RHS on an arbitrary function and using

the Jacobi identity.

Hamiltonian vector ﬁelds preserve the symplectic form as

Lie

(ω)=d(X

ω)+X

dω = −ddf =0,

where Lie is the Lie derivative (A3) deﬁned in the Appendix A. Conversely if

a vector ﬁeld Lie derives ω then it is always Hamiltonian provided that M is

5.1 A variational principle 89

simply connected. The one-parameter group of transformations generated by

a Hamiltonian vector ﬁeld is called a symplectomorphism.

The Darboux theorem stated in Section 1.3 implies that symplectic mani-

folds are locally isomorphic to R

with its canonical symplectic structure

ω =



i=1

∧ dq

. (5.1.9)

The formula (5.1.9) is also valid if M = T

∗

X and p

are local coordinates on

the ﬁbres of the cotangent bundle.

If ω is given in the Darboux atlas, then the Poisson bracket is given by

(1.1.1), and the Hamiltonian vector ﬁeld corresponding to the function H is



i=1

∂ H

∂p

∂

∂q

−

∂ H

∂q

∂

∂p

In general



a,b=1

∂ H

∂ξ

∂

∂ξ

where ξ

, a =1,...,2n, are local coordinates on M.

5.1.3 Solution space

Let M be a solution space of a second-order Euler–Lagrange equations (5.1.3).

We shall assume that no boundary conditions are imposed on the variation δq,

and derive the symplectic structure on M from the boundary term in (5.1.3).

Let



L(q(t),

q(t))dt

be a function on M. Consider a one-parameter family of paths q

(t). Then

s=0

∂ L

∂

δq|

because equations (5.1.3) are satisﬁed. Rewrite the last formula as

= P

− P

where

= p

is the canonical one-form on T

∗

X. The identity ddS

= 0 implies that

ω = dP

= dP

(5.1.10)

90 5: Lagrangian formalism and ﬁeld theory

is a two-form on M = T

∗

X which does not depend on the choice of points

, t

5.2 Field theory

Let R

1,D

denote a (D + 1)-dimensional Minkowski space-time with coordinates

=(x

, x

,...,x

)=(t, x),

and the ﬂat metric of signature (+ −−···−)

= η

µν

= dt

−|dx|

This metric will be used to raise and lower indices. We shall discuss a relativis-

tic ﬁeld theory of N scalar ﬁelds. Let φ : R

D+1

→ Y ⊂ R

be a scalar ﬁeld with

components φ

, a =1,...,N,onR

1,D

We assume that the Lagrangian density L = L(φ

,∂

), where ∂

= ∂/∂

depends only on ﬁelds and their ﬁrst derivatives. The action is given by

S =



×R

L d

xdt. (5.2.11)

The ﬁeld equations are derived from the least-action principle

∂L

∂φ

−

∂

∂x

∂L

∂(∂

)

=0.

The natural Lorentz-invariant Lagrangian density

L =

∂

− U(φ). (5.2.12)

leads to the second-order ﬁeld equations

∂

= −

∂U

∂φ

. (5.2.13)

Suppose that a Lie group G acts on the spaces of dependent and independent

variables in the way described in Chapter 4. Suppose that the inﬁnitesimal

group action changes the Lagrangian by a total divergence

L −→ L + ε∂

for some B

(x) (this condition must hold before the ﬁeld equations are

imposed). If G acts only on the target space Y we talk about internal sym-

metries. In a neighbourhood of the identity transformation we have

(x) −→ φ

(x)+εW

(x).

5.2 Field theory 91

We calculate the corresponding inﬁnitesimal change in the Lagrangian

density

L −→ L + ε



∂L

∂(∂

)

∂

∂L

∂φ



= L + ε∂



∂L

∂(∂

)



+ ε



∂L

∂φ

− ∂

∂L

∂(∂

)



We now use the Euler–Lagrange equations to set the last term to zero. There-

fore

∂L

∂(∂

)

− B

=(J

, J)

is the conserved current. The divergence free condition

∂ J

∂t

+ ∇·J =0

implies the conservation of the Noether charge

Q =



An application of the divergence theorem shows that this charge is independent

of time if J vanishes at spatial inﬁnity



∂ J

∂t

x = −



∇·Jd

x =0.

If the action of G on Y is trivial, and G acts on R

D+1

isometrically we talk

about space-time symmetries. The Lagrangian (5.2.12) is invariant under the

transformation

−→ x

+ εV

Inﬁnitesimally the ﬁeld and the Lagrangian density transform by the Lie deriva-

tive along the vector V = V

∂

−→ φ

+ εV

)=φ

+ εLie

and L −→ L + εLie

Assume that V

is a constant vector, so that G is the group of space-time trans-

lations. The conserved current is in this case given by the energy–momentum

tensor

∂L

∂(∂

)

∂

− η

92 5: Lagrangian formalism and ﬁeld theory

This tensor is divergence free, ∂

= 0, and the associated conserved charges

are the energy E and momentum P

E =



x and P

= −



x, i =1,...,D.

The conservation of E and P

is therefore related, by Noether’s theorem, to

the invariance of L under the time and spatial translations, respectively. In the

special case, when L is given by (5.2.12) we can deﬁne the kinetic and the

potential energy by

T =



x and V =





∇φ

·∇φ

+ U(φ)



x (5.2.14)

so that E = T + V.

5.2.1 Solution space and the geodesic approximation

A solution space S of the Euler–Lagrange equations (5.2.13) is an inﬁnite-

dimensional manifold, and formally we can equip it with a symplectic struc-

ture, which arises from the boundary term in the variational principle. Let φ

be a solution to (5.2.13). A tangent vector δφ to S at a given solution φ is the

linearization of (5.2.13) around φ

∂

δφ

= −

∂

∂φ

φ=φ

δφ

. (5.2.15)

Analysing the variation of the action along the lines leading to (5.1.10) we ﬁnd

a closed two-form  on S

(δ

φ,δ

φ)=





∂

∂t

(δ

) − δ

∂

∂t

(δ

)



where δ

φ and δ

φ are two solutions to (5.2.15) which implies that integrand

does not depend on t.

The dynamics of ﬁnite-energy solutions to (5.2.13) with small initial velocity

can be reduced to a ﬁnite-dimensional dynamical system. The idea goes back to

Manton [113], and the method is analogous to the argument leading to (5.1.4)

with R

n+1

replaced by an inﬁnite-dimensional conﬁguration space of the ﬁelds

φ, and X replaced by the the moduli space M of static ﬁnite-energy solutions

to (5.2.13).

Assume that all ﬁnite energy static solutions φ

= φ

(x,γ) to (5.2.13) are

parameterized by points in some ﬁnite-dimensional manifold M with local

coordinates γ . These solution give the absolute minimum of the potential

energy. The time-dependent solutions to (5.2.13) with small total energy (hence

small potential energy) above the absolute minimum will be approximated by

5.3 Scalar kinks 93

a sequence of static states, that is, a free motion in M. This free motion is

geodesic with respect to a natural Riemannian metric on M

(γ )=



∂φ

∂γ

∂φ

∂γ

x (5.2.16)

which arises from the kinetic energy (5.2.14) by

T =



∂φ

∂t

∂φ

∂t

x =



∂φ

∂γ

∂φ

∂γ

(γ )

5.3 Scalar kinks

In this section we shall study solitons in the context of Lagrangian ﬁeld theory.

The term ‘soliton’ here has a different meaning to that which we used in the

ﬁrst three chapters. The solitons are necessarily static and the inverse scattering

theory is not used in general.

Deﬁnition 5.3.1 Solitons are non-singular, static, ﬁnite energy solutions of the

classical ﬁeld equations.

At the quantum level solitons correspond to localized extended objects (par-

ticles): Kinks in one-dimension, Vortices or Lumps in two dimensions, and

Monopoles in three dimensions. One ﬁnds solitons by solving classical non-

linear equations exactly. Sometimes time-dependent solitons are considered

and it is required that they are non-dispersive and preserve their shape after

collisions. These are ‘rare’ in the sense that they only appear in integrable ﬁeld

theories which we studied in Chapter 2.

Consider a single scalar ﬁeld on two-dimensional space time. The

Lagrangian density (5.2.12) with D = 1 gives

L =





−

− U(φ)



dx = T − V,

where φ

= ∂

φ,φ

= ∂

φ and

T =



dx, V =





+ U(φ)



are the kinetic and the potential energies, respectively. The ﬁeld equations

(5.2.13) are

− φ

= −

dφ

. (5.3.17)

94 5: Lagrangian formalism and ﬁeld theory

U(F)

Figure 5.1 Multiple vacuum

We need U(φ) ≥ U

for a stable vacuum, and we choose the normalization

= 0. Assume that the set U

−1

(0) = {φ

,φ

, ...} is non-empty and discrete

(Figure 5.1). In perturbation theory φ undergoes small oscillations around one

of the minima, φ = φ

+ δφ. The basic perturbative excitation is a scalar boson

with a squared mass equal to the quadratic part of U when expanded about

a minimum. This is because (5.2.12) is the Lagrangian density for the Klein–

Gordon equation ( + m

)δφ =0.

The ﬁnite-energy solutions must asymptotically approach an element of

−1

(0). This element can however be different at different ends of a real line.

The simplest topological solitons are characterized by the boundary conditions

∼

as x →−∞ and φ

∼

as x →∞,

and cannot be treated within the perturbation theory. These are the Kink

solutions connecting neighbouring vacua. The static ﬁeld equation

dφ

formally resembles the Newton equations in classical mechanics. It inte-

grates to

= U(φ)+c, c = const.

The boundary conditions yield U(φ

)=U(φ

) = 0, and therefore c = 0. The

kink solution is implicitly given by

x − x

= ±





2U(

φ)

φ. (5.3.18)

The RHS diverges near a minimum of U. Here the constant x

is the location

of the kink and the sign on the RHS corresponds to the direction of the kink.

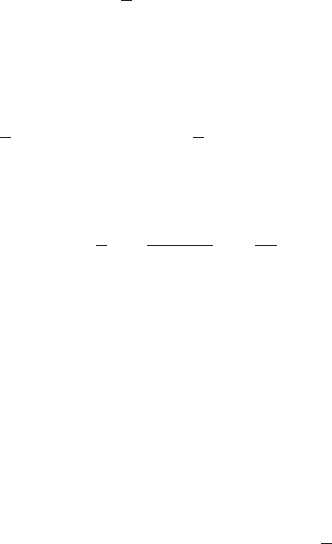
5.3 Scalar kinks 95

The potential energy of the kink is

E =





+ U



dx =



2Udx.

Example. Let U = λ

(φ

− a

)

/2. In perturbation theory this describes a

scalar boson with mass 2λa, because

U(φ)=

(φ + a)

(φ − a)

(2λa)

(φ − a)

+ O

(φ − a)

and we regard a and φ as dimensionless. The integration (5.3.18) gives

x − x

= ±



−

= ±

λa

tanh

−1

(φ/a).

Therefore

(x)=±a tanh[λa(x − x

)] (5.3.19)

which approaches ±a as x →±∞. This is a truly non-perturbative solution

as for the ﬁxed boson mass m =2λa the RHS of (5.3.19) is not analytic in

λ, and therefore it can not be obtained by starting from a solution to the

(1 + 1)-dimensional wave equation for φ and expanding in λ.

The energy of the kink is given by

E = λ



sech

[λa(x − x

)]dx =

λa

We identify E with the mass of the kink. This is because the solution has non-

zero energy density in a small region of order a

−1

. The energy density has its

maximum at x = x

which justiﬁes the interpretation of x

as the position of

the kink.

The mass of the kink is therefore much larger than the boson mass if a

large with λa ﬁxed, which is the perturbative regime of quantized theory. In

quantum theory the ﬁeld ﬂuctuations around the kink can contribute to the

kink mass. The higher order corrections are calculated in [141].

A moving kink can be obtained by a Lorentz boost of a static kink:

φ(x, t)=φ

[γ (x − vt)],γ=(1− v

)

−1/2

It solves the ﬁeld equation (5.3.17). The moving kink has conserved energy

E = T + V related to its mass by E = γ M. It also has a conserved momentum

P = −



∞

−∞

dx = γ Mv.