Drennan R.D. Statistics for Archaeologists: A Common Sense Approach

Подождите немного. Документ загружается.

18 CHAPTER 2

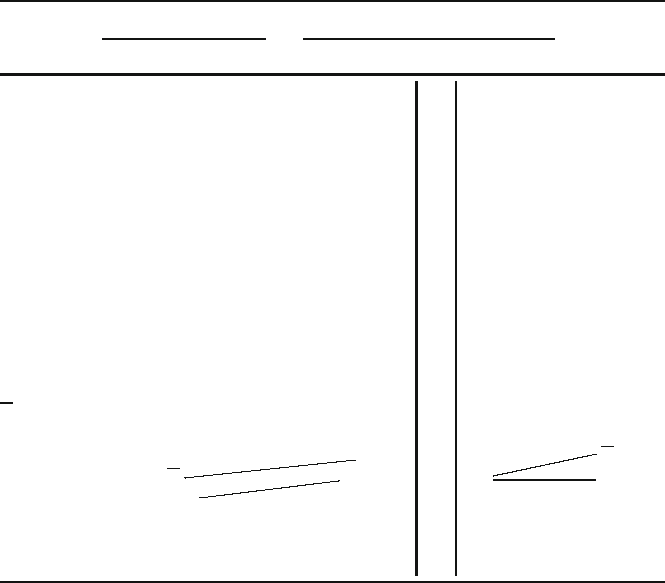
Table 2.1. Weights of Flakes Recovered from Two Bell-Shaped Pits

Flake weights (g) Back-to-back stem-and-leaf plot

Pit1 Pit2 Pit1 Pit2

9.211.3628

12.99.8 27

11.414.1 26

9.113.5 25

28.69.7 24

10.512.0 23

11.77.8 22

10.110.6 21

7.611.5 20

11.814.3 19

14.213.6 18

10.89.3 17

10.9 16

X 12.33 11.42 2 14 13

Md 11.10 11.30

13 56

12 0 X

X 874 11 35 Md

851 10 69

9 378

6 7 8

The Greek letter

∑

(capital sigma) stands for “the sum of” and is a symbol

used frequently in statistics.

∑

x simply means “the sum of all the x’s.” Formulas

with Σ may seem formidable, but, as we have just seen, Σ is simply shorthand for

a relatively simple and familiar calculation. Σ is virtually the only mathematical

symbol used in this book that is not common in basic algebra.

Table

2.1 presents some data on weights of ﬂakes recovered from two bell-shaped

storage pits in the same site. The back-to-back stem-and-leaf plot reveals that the

ﬂakes from Pit 1 bunch together between about 9 and 12 g, with one outlier at 28.6g

(to which we probably do not want to pay too much attention). The ﬂakes from Pit

2 also bunch together, although the peak is more spread out and may even have a

slight tendency to split into two. The center of the batch of ﬂakes from Pit 2 would

appear to be a little higher on the whole than for those from Pit 1. For the ﬂakes from

Pit 1, the mean (calculated by summing up all 12 weights and dividing the total by

12) is 12.33g. For Pit 2, the mean (calculated by summing up all 13 weights and

dividing the total by 13) is 11.42 g. Both means are indicated in their approximate

positions along the stem in the stem-and-leaf plot.

THE LEVEL OR CENTER OF A BATCH 19

We can be fairly happy with the mean as an index of the center for Pit 2; it does

point to something like the center of the main bunch in the batch, as seen in the stem-

and-leaf plot. When we look at Pit 1, however, we have cause for concern. The mean

seems to be well above the center of the main bunch in the batch. It is “pulled up”

quite strongly by the high outlier at 28.6 g, which has a major impact on the sum of

the weights. Since we just observed that the Pit 1 batch has a somewhat lower level

than the Pit 2 batch, it is alarming that the mean for Pit 1 is actually higher than

the mean for Pit 2. A comparison of means for these two batches would suggest

that ﬂakes from Pit 1 tended to weigh more than those from Pit 2 – a conclusion

exactly opposite to the one we arrived at by examining the stem-and-leaf plot. In this

instance, the mean is not behaving very nicely. That is, it is not providing a useful

index of the center of the Pit 1 batch for the purpose of comparing that batch to the

Pit 2 batch. There are ho hard-and-fast rules for judging when the mean is behaving

nicely enough to use as an index of center. It is ﬁnally a question of subjective

judgment that requires careful exploration of batches with stem-and-leaf plots, real

understanding of what we want an index of center to do, and practice.

THE MEDIAN

If the mean does not behave nicely because of the shape of a batch, the median may

be a more useful index of center. The median is simply the middle number in the

batch (if the batch contains an odd number of numbers) or halfway between the two

middle numbers (if it contains an even number of numbers). The stem-and-leaf plot

is useful for ﬁnding the median, because it makes it easy to count in from either

the top or the bottom to the middle number. It is especially easy to do this if the

leaves have been placed in numerical order on each line of the stem-and-leaf plot.

The alternative to the stem-and-leaf plot, the histogram, cannot be used for ﬁnding

the median because, while the histogram represents the overall shape of the batch,

it does not contain the actual numbers.

To ﬁnd the median weight of ﬂakes from Pit 1, we ﬁrst count the number of

ﬂakes. Since there are 12 (an even number), the median will be halfway between the

middle two numbers. The middle two numbers will be the sixth and seventh, count-

ing in from either the highest or lowest number. For example, counting leaves in the

stem-and-leaf plot for Pit 1 from the bottom or lowest number, we have the ﬁrst ﬁve

numbers: 7.6, 9.1, 9.2, 10.1, and 10.5; then the sixth and seventh numbers: 10.8 and

11.4. Alternatively, counting leaves from the top or highest number, we have the

ﬁrst ﬁve numbers: 28.6, 14.2, 12.9, 11.8, and 11.7; then the sixth and seventh: 11.4

and 10.8, the same as before. Halfway between 10.8 and 11.4 is 11.1. So the median

weight of ﬂakes from Pit 1 is 11.10 g (Md = 11.10g).

For Pit 2, there are 13 ﬂakes, so the median will be the middle number, or the

seventh in from either the highest or lowest. Counting leaves from the top gives us

the ﬁrst six numbers: 14.3, 14.1, 13.6, 13.5, 12.0, and 11.5; then the seventh: 11.3.

Counting leaves from the bottom gives us the ﬁrst six numbers: 7.8, 9.3, 9.7, 9.8,

20 CHAPTER 2

10.6, 10.9; then the seventh: 11.3, exactly as before. Thus the median weight of

ﬂakes from Pit 2 is 11.30g (Md = 11.30g).

Medians for both batches are indicated on the stem-and-leaf plot in Table

2.1,and

both indicate points that are visually more satisfying indications of the centers of

the two batches. Comparing the levels of the two batches according to their medians

also seems more reasonable than our attempt to use their means for this purpose.

The median weight of ﬂakes in Pit 2 is slightly higher than that for Pit 1, which is

indeed the conclusion we came to based on observation of the general pattern of the

stem-and-leaf plot.

OUTLIERS AND RESISTANCE

It might seem surprising that the mean and the median behave so differently in this

example. After all, both are fairly widely used indexes of the level of a batch. And

yet, comparing the two batches in this example by means and by medians gave

opposite conclusions about which batch had a higher center. Clearly, it is the mean

of the ﬂakes from Pit 1 that seems strange. Its peculiarly high position is attributable

entirely to the effect that the one high outlier (the ﬂake that weighs 28.6 g) has on

the calculations. While it pulls the mean up substantially, this outlier, in contrast,

has no effect whatever on the median. If instead of weighing 28.6 g, this ﬂake had

weighed 12.5 g, the median ﬂake weight for Pit 1 would not have changed at all.

The heaviest ﬂake is simply the ﬁrst number that we count past to reach the middle

of the batch, which remains in exactly the same place, irrespective of how high the

highest value is. In fact, the median does not depend at all on the actual values of the

numbers in either the upper half or the lower half of the batch. As long as there is

no change that moves a number from the upper half to the lower half or vice versa,

the median remains exactly the same.

This is one example of a general principle. The mean of a batch is strongly

affected by any outliers that may be present. The median is entirely unaffected

by them. In statistical jargon, the median is very resistant. The mean is not at all

resistant.

ELIMINATING OUTLIERS

The mean has special properties that make it a particularly useful index of the

center of a batch, but outliers can present a serious problem by making the mean

a very inaccurate index. It would be nice to eliminate outliers if we could, and,

as it turns out, often we can. In the ﬁrst place, we should always examine out-

liers carefully. Sometimes they indicate errors in data collection or recording. This

possibility was already broached in Chapter 1, where it was suggested that the

THE LEVEL OR CENTER OF A BATCH 21

extraordinarily large post hole in the example in Fig. 1.1 might have been the result

of an error in measurement or in data recording. Such an error could be corrected

by reference to photographs and drawings of the excavation, thus eliminating the

outlier.

Even if it turns out that an outlier is, indeed, a correct value, it still may be

desirable to eliminate it. As a classic example of such a situation, consider the mail

order clothing ﬁrm of L.L. Pea, Inc., specializing (of course) in the famous Pea coat.

L.L. Pea employs ten shipping clerks, nine of whom are each paid $8.00 per hour

while the tenth earns $52.00 per hour. The median wage in the L.L. Pea shipping

room, then, is $8.00 per hour, while the mean wage is $12.40 per hour. Once again,

the mean has been raised substantially by an outlier, while the median has been

entirely unaffected. A careful check of payroll records reveals that it is, indeed, true

that nine shipping clerks are paid $8.00 per hour while one earns $52.00 per hour. It

also reveals, however, that the highly paid clerk is Edelbert Pea, nephew of L.L., the

founder of the company, who spends most of his “working” hours in the company

cafeteria anyway. If our interest is in the wages of shipping clerks, there is clearly

no reason to include young Edelbert among our data. We are much better off simply

to eliminate him as not truly a case of what we want to study and use the data for

the other nine shipping clerks.

It is often sensible to eliminate outliers in just such a manner. If a good reason

can be found aside from just the aberrant number in the data (as in the instance

of Edelbert Pea), we can feel quite comfortable about eliminating outliers. In the

example batch in Table

2.1 for Pit 1, perhaps we would note that the unusually heavy

ﬂake was of a very different form from all the rest or of a very different raw material.

In this last case, we might reduce our batch to obsidian ﬂakes, say, rather than all

ﬂakes, in order to eliminate a single very heavy chert ﬂake. Even if such external

reasons cannot be found to justify it, a distant outlier can be eliminated simply on

the basis of its measurement. There are, however, other treatments that take care of

outliers without making it seem that somehow we are fudging our data by leaving

out cases we don’t like.

THE TRIMMED MEAN

The trimmed mean systematically removes extreme values from both upper and

lower ends of a batch in a balanced fashion. In considering the level of a batch,

it is the central bunch of numbers that matters most. It is not uncommon for the

highest and lowest numbers to straggle away from this bunch in an erratic man-

ner, and it is important not to be confused by such unruly behavior on the part of

a few numbers. The trimmed mean effectively avoids such confusion by simply

eliminating some proportion of the highest and lowest numbers in the batch from

consideration.

For example, we might calculate a 5% trimmed mean of the ﬂake weights from

Pit 1 in Table

2.1. For a 5% trimmed mean, we eliminate the highest 5% of the batch

22 CHAPTER 2

and the lowest 5% of the batch. There are 12 numbers in this batch, so we remove

5% of 12 numbers from each end. Since 0.05×12 = 0.60, and 0.60 rounds up to 1,

we remove one number from the top and one number from the bottom. (In deciding

how many numbers to remove for the trimmed mean we always round up.) In this

case, then, we remove the highest number (28.6) and the lowest number (7.6) from

the batch. After removing the highest and lowest numbers, we have a trimmed batch

of ten numbers (n

= 10). The trimmed mean is simply the ordinary mean of the

remaining ten numbers, once the highest and lowest have been removed. For Pit 1

the 5% trimmed mean,

, is the sum of the remaining numbers divided by n

(that

is, 10), or 11.17g. For Pit 2, a 5% trimmed mean also requires eliminating a single

number from each end of the batch (0.05 ×13 = 0.65, which rounds up to 1). The

total of the remaining numbers is divided by n

(that is, 11), for X

= 11.48g.

We can see that the trimmed mean, unlike the ordinary mean, is resistant to the

effect of outliers. In this example, the 5% trimmed means are quite similar to the

medians. They would lead us to conclude that ﬂakes in Pit 2, in general, weigh

slightly more than ﬂakes in Pit 1, just as observation of the stem-and-leaf plot makes

us know we should conclude.

In the 5% trimmed mean calculated above, 5% is the trimming fraction.The

trimming fraction can be adjusted to ﬁt the needs of a particular situation. Custom-

arily, the trimming fraction is some multiple of 5% (5%, 10%, 15%, etc.). The most

frequently used trimming fractions are probably 5% and 25%. The 25% trimmed

mean is sometimes called the midmean because it is the mean of the middle half of

the numbers (one-fourth of the numbers having been eliminated from the top of the

batch and one-fourth from the bottom).

As one ﬁnal example, a 25% trimmed mean of the ﬂake weights from Pit 1 in

Table

2.1 requires elimination of the three highest and the three lowest numbers

(0.25 ×12 = 3). The mean of the remaining six numbers is 11.05 g. For the ﬂake

weights from Pit 2, a 25% trimmed mean requires removal of four numbers from the

top and bottom (0.25×13= 3.25, which rounds up to 4). The mean of the remaining

ﬁve numbers is 11.26 g. Just as with the 5% trimmed mean, the undesirable effects

of outliers have been avoided entirely; and the comparison of means shows that Pit

2 ﬂakes are, in general, slightly heavier than Pit 1 ﬂakes.

Statpacks

Any statistics package will determine the mean and median for a batch of num-

bers. Not very many, however, provide the trimmed mean as a deﬁned option.

What you are likely to have to do to get your statpack to calculate a trimmed

mean is do the trimming yourself. You could simply omit the numbers to be

trimmed when entering the data initially or you could delete those cases (or

code them as missing data by whatever provision your statpack makes for han-

dling missing data). Then your statpack can easily calculate the mean of the

remaining numbers.

THE LEVEL OR CENTER OF A BATCH 23

It is worth noting that the median could be thought of as the ultimate in trimmed

means, the 50% trimmed mean. Removing the upper half of the batch and the lower

half of the batch leaves nothing but the midpoint, or median.

WHICH INDEX TO USE

The median, the mean, and the trimmed mean are all numerical indexes of the center

of a batch. The question thus arises, which one should we use? This question has

no simple answer. Sometimes it is better to use the mean, sometimes the median,

sometimes the trimmed mean. It depends on the characteristics of the batch in ques-

tion and on what you intend to do with the numerical index of the center once you

have it. The mean is the most familiar, and that is an advantage worth considering,

since just about anyone feels comfortable if you tell them what the mean of a batch

of numbers is. If the batch does not have outliers that make the mean a deceptive

value, then it may well be the best choice. The median is slightly less familiar, but

it is highly resistant, and so it is used fairly often for batches with outliers. The

trimmed mean is considerably less familiar to most archaeologists, but it combines

advantages of mean and median in some respects.

As we will see in later chapters, the mean has some special properties that make

it highly useful in statistics. It is thus often tempting to use the mean, even when

the batch has outliers that affect it. The trimmed mean can be put to work in at

least some of the same ways the mean can, however, without interference from

outliers. That is what makes the trimmed mean worth discussing, even though it

is more complicated to calculate than either the mean or the median and less well

known among archaeologists. The median, unfortunately, cannot be used in these

special ways. Even though it is quite straightforward and useful for the initial task

of comparing batches, then, the median will not be as important to us farther along

in this book as the mean and the trimmed mean.

BATCHES WITH TWO CENTERS

Sometimes examination of a stem-and-leaf plot makes it clear that a batch contains

two or more quite distinct bunches, as discussed in Chapter

1. We will call such

batches two-peaked or multi-peaked. (The metaphor of the peak is derived from the

histogram, where a bunch of numbers resembles a hill or peak, but it is easy enough

to think of a stem-and-leaf plot in these terms as well.)

Table

2.2 provides the areas (in square meters) of structures excavated at the

Black-Smith sites. The stem-and-leaf plot shows that these structures form two sep-

arate groups on the basis of their areas. There are large structures, mostly from about

15 to 21m

, and small structures, from about 3 to 7m

. It would make little sense to

talk about the center of this batch because it clearly has two centers. If it makes little

24 CHAPTER 2

Table 2.2. Floor Areas of Structures at the

Black-Smith Sites

Area (m

) Stem-and-leaf plot

18.3268

18.825

16.724

6.1234

5.222

21.2212

19.820

4.219

128

18.318

33789

3.617

20.016

7.515

15.314

26.8136

5.412

18.711

6.210

7.09

20.78

18.9705

19.26

1277

6.75

244689

19.14

259

23.43

4.5

16.2

5.6

17.5

5.9

6.7

4.9

17.9

15.0

13.6

5.4

5.8

sense to talk about its center, then it makes even less sense to calculate a numeri-

cal index of its center. If we tried it, the results would be nonsense. The mean, for

example, of the batch in Table

2.2 would be 12.95m

. This value falls in between

the two distinct groups, characterizing no structures at all. At 15.15m

, the median

would also fail to characterize the center of anything meaningful. We would thus

never even calculate these two values.

THE LEVEL OR CENTER OF A BATCH 25

The ﬁrst thing to do if you see a two-peaked batch in a stem-and-leaf plot is

separate it into two different batches – before calculating any indexes of center.

This is not some mysterious rule that must be memorized. It is simply the only

practice that makes sense to anyone who keeps ﬁrmly in mind what indexes of

center are doing and how they behave. In a case like this, one must think that there

are basically two different kinds of structures represented, perhaps houses and grain

bins. Other information concerning these structures could be examined for evidence

relevant to such a notion. In any event, before further quantitative analysis the batch

must be broken into two batches, and the large structures treated separately from

the small structures. We would make the break at about 10 or 11m

in the middle

of the large gap visible in the stem-and-leaf plot. The 16 small structures that are

less than 10m

have a mean area of 5.67m

(and an almost identical median area of

5.70m

). The 20 large structures have a mean area of 18.77m

(and, once again, an

almost identical median area of 18.75m

). For both small structure areas and large

structure areas, then, either the mean or the median would provide meaningful and

useful indexes of the center. (Locate them along the stem in the stem-and-leaf plot,

and you will see that they are indeed in the center of the main bunch of numbers for

each sub-batch.) Breaking a two-peaked batch into two batches has made it possible

to calculate numerical indexes of the centers of the two batches that make sense.

Batches like the one in Table

2.2 are often referred to loosely as bimodal,afterthe

term mode which refers to the single most common category in a stem-and-leaf plot

or histogram. Sometimes the mode is used as an index of the center of a batch. In

Table

2.2, the mode would be at about 5m

, where six structures fall. This, clearly, is

something like the center of the batch of small structures, but it won’t do as an index

of the center of the entire batch. There is a secondary mode at about 18m

,where

ﬁve structures fall. This is something like the center of the batch of large structures.

Only if exactly the same number of structures fell at 5m

and at 18m

would this

batch truly have two modes. Strictly speaking, it has a mode and a secondary mode

rather than two modes. Nevertheless, such multipeaked batches are often referred to

as bimodal.

PRACTICE

1. Look back at the data on scraper lengths given in Tables 1.9 and 1.10. Calculate

appropriate indexes of center to put a ﬁner point on the comparison you have

already made with a stem-and-leaf plot between Pine Ridge and the Willow Flats

scraper lengths. Try out the mean, the median, and a trimmed mean (with what-

ever trimming fraction you think is most appropriate). Which index of center

makes most sense for the comparison of scraper lengths between the two sites?

Why? (Note that comparisons of levels must be based on the same index. You

shouldn’t compare the mean for one batch to the median for another.) Summa-

rize the comparison of scraper lengths you have made between the two sites. That

is, what has all this told you about scraper lengths at the two sites?

26 CHAPTER 2

2. Using the data from Tables 1.9 and 1.10 once again, do the same for ﬂint scrapers

and chert scrapers, disregarding which site the scrapers came from. Try the mean,

the median, and the trimmed mean again. Which index makes most sense for

comparing the lengths of scrapers made of different raw materials? Why? How

would you summarize all together the comparisons you have made between ﬂint

and chert scrapers and between the Willow Flats site and Pine Ridge Cave?

Chapter 3

The Spread or Dispersion of a Batch

The Range .......................................................................................... 27

The Midspread or Interquartile Range............................................................. 28

The Variance and Standard Deviation ............................................................. 29

The Trimmed Standard Deviation ................................................................. 32

Which Index to Use ................................................................................ 34

Practice.............................................................................................. 36

Some batches of numbers are very tightly bunched together while others are much

more spread out. This property is referred to in exploratory data analysis as spread

(or in more traditional statistical terms as dispersion), and it is often an informative

characteristic of a batch to which you should pay attention. Just as it is convenient

to have a numerical index for the level or center of a batch, it is also convenient to

have a numerical index for the spread, or dispersion, of a batch. Once again there

are several different numerical indexes that behave differently and are thus used in

different circumstances.

THE RANGE

The simplest index of the spread of a batch is its range. The range in statistics

is exactly what it is in everyday conversation: the difference between the lowest

number and the highest number in the batch. Table

3.1 presents the same exam-

ple numbers we discussed in the previous chapter. The range for the weights of

ﬂakes recovered from Pit 1 is the difference between 28.6 and 7.6 g, or 21.0 g

(28.6g−7.6g = 21.0g). The range for the weights of ﬂakes recovered from Pit

2 is the difference between 14.3 and 7.8 g, or 6.5 g (14.3g−7.8g= 6.5g).

We notice immediately that the range suffers from the same problem that the

mean suffers from: it is not at all resistant. In fact, it is even less resistant than

the mean. Not only is it strongly affected by outliers, it may well depend entirely

on outliers. Examination of the stem-and-leaf diagram reveals how misleading the

range is in this instance. The two batches here have rather similar spreads, but we

would probably say that the ﬂake weights from Pit 2 are more spread out than those

R.D. Drennan, Statistics for Archaeologists, Interdisciplinary Contributions

to Archaeology, DOI 10.1007/978-1-4419-0413-3

 Springer Science+Business Media, LLC 2004, 2009