Drake G.W.F. (editor) Handbook of Atomic, Molecular, and Optical Physics

Подождите немного. Документ загружается.

1068 Part F Quantum Optics

a rotation of the Bloch vector in the V –W plane. The

angle θ is called the pulse area, deﬁned by

θ(t) ≡



−∞



Ω



) =



−∞



E (t



). (73.8)

The area under the envelope of the Rabi frequency is

thus the same as the angle through which an exactly-

resonant Bloch vector turns due to the pulse. If θ = π,

the atom is driven from the ground state exactly to the

excited state. This is “π-pulse” inversion. A “2π-pulse”

takes the atom from the ground state through the excited

state and back to the ground state. For ∆ =0, the Bloch

vector rotation angle does not depend upon the shape of

the ﬁeld pulse, only on the area of the pulse.

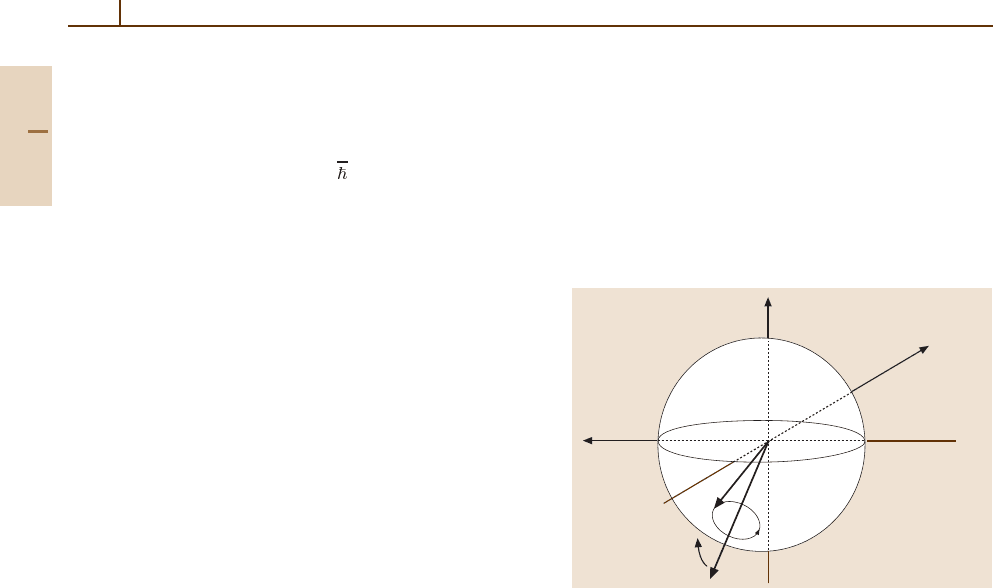
73.3.4 Adiabatic Following

The Bloch vector picture is used for semiquantitative

predictions. These are reliable even if ∆ and Ω

are

time-dependent, if the parameters change slowly (adia-

batically). For example, if Ω is moved slowly the Bloch

vector follows closely. It is possible to achieve complete

inversion smoothly in this way. If the ﬁeld is initially

tuned far below resonance so ∆  Ω

then Ω points ap-

proximately toward the south pole of the Bloch sphere.

As shown in Fig. 73.3, the Bloch vector then precesses

in a very small circle about the torque vector. If the ﬁeld

frequency is now slowly changed (chirped) so that ∆

goes from a large negative value to a large positive value

then every atomic Bloch vector will continue to precess

rapidly around the torque vector, and follow it as it pro-

ceeds from pointing straight down to pointing straight

up. In this way the population is transferred between the

two levels.

–V

–U



Fig. 73.3 In adiabatic inversion the Bloch vector of each

atom precesses in a small cone about the torque vector as

the torque vector goes from straight down to straight up

73.4 Inhomogeneous Relaxation

The fact that the various atoms in a sample may have dif-

ferent resonance frequencies produces a number of novel

phenomena. Given a distribution g(∆) of detunings in

a dilute gas of density N, the macroscopic polarization

can be written

P(t) =−Nex(t)

= Nd



g(∆) Re



(U −iV ) e

−iωt



d∆,

(73.9)

where U −iV generally depends on both t and ∆.

73.4.1 Free Induction Decay

Free induction refers to evolution of the polariza-

tion in the absence of a laser ﬁeld. For Ω

= 0,

the Bloch vector of an atom with ∆<0 precesses

counterclockwise in the U–V plane. In a macro-

scopic sample, there are many values of ∆ and about

as many are positive as negative. Thus an oriented

collection of Bloch vectors, all pointing in the V di-

rection at t = 0, will rapidly fan out in the U–V

plane due to differing precession rates, and after

a short time the net V value will be zero, as will the

net U value. This is free induction decay (FID)of

polarization.

More precisely, if all atoms are ﬁrst exposed to a θ

pulse, so that at t = 0

U(0,∆)= 0 ,

V(0,∆)=−sin θ

, (73.10)

W(0,∆)=−cos θ

then if E = 0fort > 0, an individual atom with detuning

∆ evolves according to (73.4):

U −iV = isinθ

−

(

1/T

−i∆

)

. (73.11)

The macroscopic polarization is found by summing the

individual (U −iV ) values over the detuning distribution

g(∆).

For simplicity, in this subsection we will ignore

competition from homogeneous decay (take 1/T

≈ 0)

Part F 73.4

Coherent Transients 73.5 Resonant Pulse Propagation 1069

and assume the most common inhomogeneous lineshape

(i.e., Doppler-Maxwellian):

g(∆) =

∗

√

2π

−(∆−∆

)

∗2

, (73.12)

where 1/T

∗

is here deﬁned as the width (standard devi-

tation) of the Doppler distribution and ∆

is the detuning

of the zero-velocity atoms. The collective result is

P(t)=Nd sin θ

sin ωte

i∆

exp



−

∗2



(73.13)

The detuning “inhomogeneity” in the sample leads

to dephasing of the collective dipole moment, and

the inhomogeneous relaxation time is obviously T

∗

This is illustrated by the decrease of collective align-

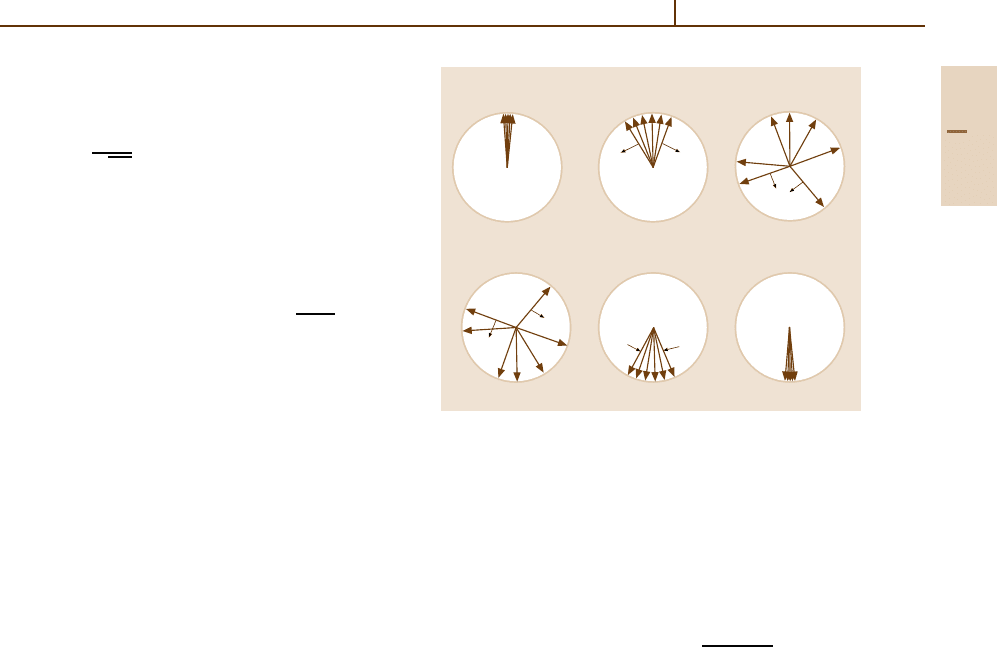
ment of Bloch vectors in the top row of Fig. 73.4.

For a typical room temperature gas a visible transi-

tion has a width given by 1/(2πT

∗

) ≈ 1.5 GHz so that

∗

≈ 10

−10

73.4.2 Photon Echoes

A photon echo is generated by pulse-induced recovery

of a nonzero P(t) after P(t) → 0 due to free induction

decay (FID). This analog of the spin echo effect is pos-

sible because each atom retains its own detuning for

a relatively long time, usually up to an average collision

time T

During FID,theU–V projection of every atom’s

Bloch vector precesses steadily clockwise or counter-

clockwise depending on the sign of its ∆. Thus the

Bloch vectors could be rephased if they could all be

forced at the same moment to reverse their relative sense

of precession. The prototypical echo scenario has FID

beginning at t = 0, with P(t) → 0fort  T

∗

, followed

by a π–pulse at the time t



,wheret



 T

∗

. The effect

of the π-pulse is to reverse the sign of V and W [re-

call (73.10)], in effect ﬂipping the equatorial plane of

the Bloch sphere upside down. Thus for t  t



we have

Bloch vectors fanning back together. The macroscopic

Free induction decay – dipoles dephase

After π pulse – dipoles rephase

t = 0 t = t– ε

t = t + ε

t = 2t

Fig. 73.4 The ensemble of dipole moments spreads due to

the distribution of resonance frequencies. The distribution

of Bloch vectors in the U–V plane is shown at various times

after the initial short pulse excitation. By the time t



,the

dipoles have spread uniformly around the unit circle. A π-

pulse then ﬂips the relative orientation of the dipoles so that

they subsequently rephase

polarization obeys

P(t) = Nd sin θ

sin ωt exp



−

(t −2t



)

∗2



−t/T

(73.14)

where the last factor recovers the effect of homogeneous

dipole damping. We require T

 t



 T

∗

for a strong

echo signal in the neighborhood of t = t



The result is illustrated in Fig. 73.4. An “echo” of

the initial excitation at t = 0 appears at the time t =



. After this, FID occurs again, and this second decay

can also be reversed by applying another π–pulse, and

so on, until t ≈ T

, at which time the inevitable and

irreversible homogeneous relaxation cannot be avoided.

The scenario of π–pulse reversal is only the most ideal,

leading to the most complete echo, and other pulse areas

will also lead to echos; a more important factor is that

the reversing pulse must be short enough that negligible

dephasing takes place during its application.

73.5 Resonant Pulse Propagation

73.5.1 Maxwell–Bloch Equations

Time-dependent atomic dipole moments created by ap-

plied ﬁelds are themselves a source of ﬁelds, another

form of coherent transient. We limit discussion to plane-

wave propagation in the z–direction. Note that we use z

rather than Z for convenience, although in the dipole ap-

proximation the coordinate entering our equations is the

Part F 73.5

1070 Part F Quantum Optics

coordinate of the center of mass of the atom rather than

the internal electron coordinate. The ﬁeld is generalized

from (73.2)to

E(t, z) =



E (t, z) e

i(Kz−ωt)

+c.c.



, (73.15)

and the macroscopic polarization is the correspondingly

generalized form of (73.9):

P(t, z) = Nd



g(∆) Re



(U −iV ) e

i(Kz−ωt)



d∆.

(73.16)

The difference between K and k =ω/c indicates that the

refractive index is nonzero. The traveling-pulse rotating

frame is also obtained by replacing ωt by ωt − Kz.

If the ﬁeld envelope E is slowly varying, its second

derivatives can be dropped when E(t, z) is substituted

into Maxwell’s wave equation. The resulting dispersive

and absorptive reduced wave equations are



−k



E = 4πk



g(∆)U(t, z,∆)d∆,

(73.17)



∂

∂z

∂

∂(ct)



E = 2πk



g(∆)V(t, z,∆)d∆.

The Bloch equations along with these reduced wave

equations form the self-consistent Maxwell–Bloch

equations that are used to treat most resonant propaga-

tion problems in quantum optics and laser theory [73.1,

2, 5, 8].

73.5.2 Index of Refraction and Beers Law

If a weak pulse of duration τ propagates in a medium

of ground-state atoms (W ≈−1), the Bloch equations

have simple quasisteady-state solutions (τ  T

)

U =

Ω

∆

+1/T

V =

−Ω

∆

+1/T

. (73.18)

When U and V are substituted back into the reduced

wave equations (73.17), the dispersive equation gives

the index of refraction n = K/k due to the ground-state

atoms:

−1 =

4πNd



∆g(∆)

∆

+1/T

d∆, (73.19)

and the absorptive equation predicts steady state ﬁeld

attenuation during propagation:

∂

∂z

E =−

E . (73.20)

The constant α

given by

4πNd



g(∆)

∆

+1/T

d∆ (73.21)

is called the extinction coefﬁcient, or the reciprocal

Beers length.

Since ﬁeld intensity I is proportional to E

,the

solution to the absorptive equation is

I(z, t) = I(0, t) e

−α

. (73.22)

This relation is called Beers Law. Both the disper-

sive and absorptive results are familiar from classical

physics [73.10], with the important distinction that

here the

-dependent oscillator strength enters naturally

rather than as an empirical parameter from Lorentzian

dielectric theory [73.8, 10].

73.5.3 The Area Theorem

and Self-Induced Transparency

A form of pulse propagation with no classical analog

arises in the short-pulse limit (τ  T

, T

,butτ  T

∗

By integration over the entire pulse, the absorptive

Maxwell equation becomes an equation for ∂θ/∂z,where

θ is the pulse area deﬁned in (73.8). In the short-

pulse limit, the relaxation terms in the OBEs can be

ignored and when substituting from them we obtain the

McCall-Hahn Area Theorem [73.1]:

∂

∂z

θ(z) =−

sin θ(z). (73.23)

This predicts the same exponential attenuation as (73.20)

in the case of a small area pulse, θ(z)  π, but in the

case of larger area pulses the behavior is quite different.

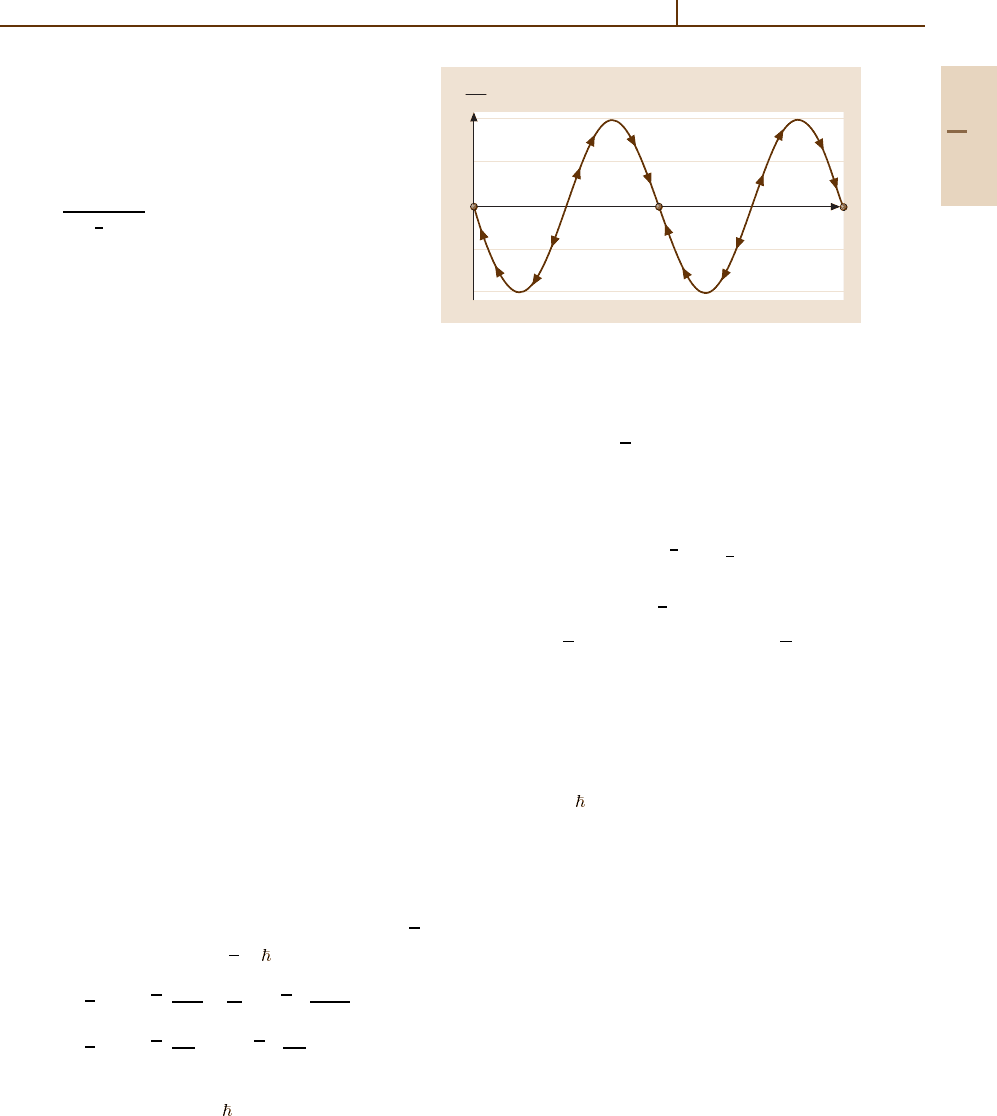
In general, the area decreases during propagation for

areas in the range 0 <θ(z)<π, but it increases for areas

π<θ(z)<2π.AsseenfromFig.73.5, this change of

area with propagation causes the pulse area to evolve to

one of the stable values 0, 2π, 4π,... .

There is one special pulse, a soliton solution with

area exactly 2π, which propagates without shape change

in the short pulse limit, given by

E (t, z) =

τd

sech



t −z/v



(73.24)

where τ is the pulse duration, which is arbitrary but must

satisfy the short-pulse inequality τ  T

, T

. The soli-

ton’s group velocity is determined by the corresponding

Part F 73.5

Coherent Transients 73.6 Multi-Level Generalizations 1071

Fig. 73.5 McCall-Hahn area theorem for an absorbing

medium. On propagation the area of the pulse will follow

the arrows toward one of the stable values, 0, 2π,4π, ...

soliton solutions to the OBE’s and the dispersive

v =

1 +

cτ

(73.25)

where α

is to be taken in the limit T

∗

 T

. The group

velocity can be slower than the speed of light by orders

of magnitude if α

cτ 1.

δθ

δz

2π 3π 4π

73.6 Multi-Level Generalizations

73.6.1 Rydberg Packets

and Intrinsic Relaxation

A short laser pulse can populate a band of excited states

whose probability amplitudes will exhibit mutual co-

herence. This single-atom coherence is transient, even

without collisions or other external perturbations to dis-

rupt it, and its decay can be called intrinsic relaxation.

The decay is basically a dephasing. The dipole moments

associated with the excited band interfere due to the wide

variety of resonance frequencies of the states in the su-

perposition. Because of the discreteness of the energy

levels of any bounded quantum system, this relaxation

has its own unique characteristics, including similarities

with both homogeneous and inhomogeneous decay.

The wave function for a coherently excited atom can

be expressed in the interaction picture in the form [73.9]

Ψ(r, t) = a(t)ψ

(r) +



(t)e

−iω

(r),(73.26)

where ψ

(r) is the ground state wave function, and n

labels the states in a band with excitation frequencies

≈ ω.If|ω

−ω|ω

, the transition frequency ω

can be expanded about the principal quantum number n

of the resonant excited state E

= ω to obtain

= ω

+(n −n )

∂ω

∂n

(n −

∂

∂n

+···

= ω

+(n −n )

2π

+(n −n )

2π

+··· . (73.27)

Thus 2π/T

is the mean frequency separating neigh-

boring levels, i. e., T

/2π = ρ(E),whereρ(E) is the

density of excited states, and 2π/T

is the mean change

in this frequency separation. T

is the same as the Ke-

pler period a classical orbit, and T

is the revival time.

Substituting the Bohr frequencies into the deﬁnitions for

and T

yields T

= nT

/3.

For times t ≤ T

the expansion can be truncated

after the third term. Then the wave function is given

approximately by

Ψ(r, t) ≈ a(t)ψ

(r) + e

−iω



n+m

(t)e

−i2πmt/T

×e

−i2πm

t/T

n+m

(r), (73.28)

where m = n −n. For high Rydberg states, n  1, the

time scales associated with the two exponentials inside

the sum are quite different. For times t  T

the individ-

ual levels are not resolved, thus the laser excites what is

effectively a continuum with a density of states ρ(E).In

that case the ground state population simply decays ex-

ponentially at the rate given by ﬁrst-order perturbation

theory, Γ = (2π/

ρ(E). At longer times t ≈ T

the ﬁrst exponential contributes, but the second does not,

giving a simple Fourier series time dependence. In this

regime the evolution of the wave function is just peri-

odic motion of a wave packet around a Kepler orbit, as

is illustrated in Fig. 73.6a.

The coherent quantum wave packet behaves like

a classical particle for many Kepler periods, gradu-

ally spreading out as the second exponential in the sum

(73.28) begins to contribute. This spreading of the wave

packet produces the intrinsic relaxation of the collective

dipole moments from the various transitions. However,

because the levels are discrete, this decay is not perma-

nent, but is reversed and leads to a spontaneous “revival”

of the original wave packet [73.6], without the need for

a π-pulse to produce an “echo.”

In its evolution toward the revival, the wave packet

passes through a number of fractional revivals in which

Part F 73.6

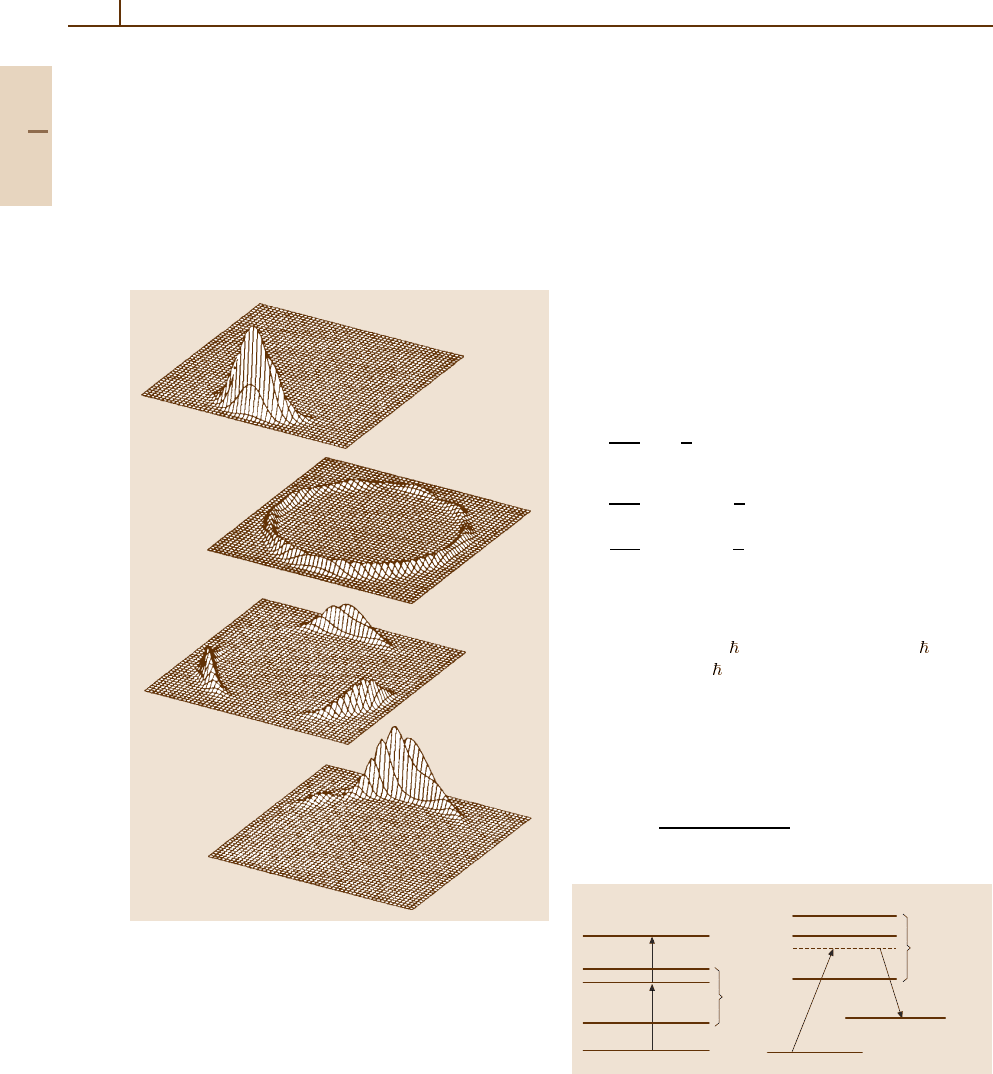
1072 Part F Quantum Optics

miniature replicas of the original wave packet are equally

spaced around the orbit, each traveling at the velocity

of a particle traveling in a corresponding classical Ke-

pler orbit. This complex time evolution arises from the

spreading of the wave packet all the way around the orbit

so that the head and tail of the packet interfere with each

other, producing interference fringes. The further evo-

lution of this fringe pattern produces the various revival

phenomena shown in Fig. 73.6.

Fig. 73.6a–d The free evolution of a Rydberg wave packet

made up of a superposition of circular-orbit states centered

about n = 360.

(a) Initially the wave packet is to good

approximation a minimum uncertainty wave packet in all

three dimensions, but after 12 orbits

(b) the packet has

spread all the way around the orbit so that the head and tail

of the wave packet overlap, producing interference fringes.

/3, the fringes have produced

the one-third fractional revival in which three miniature

replicas of the original wave packet are equally spaced

around the orbit.

(d) After 120 orbits, t = T

, the complete

wave packet revival occurs

73.6.2 Multiphoton Resonance

and Two-Photon Bloch Equations

Multiphoton transitions Fig. 73.7a introduce new coher-

ent transient phenomena. If levels |g and |e have the

same parity, two photons from the same laser ﬁeld are

sufﬁcient to excite level |e. For simplicity we regard |e

as a single state, but any number of intermediate levels

|j of opposite parity may be present.

Substituting the state vector

|Ψ(t)=a

(t)|g+



(t)e

−iωt

|j

(t)e

−i2ωt

|e , (73.29)

into the Schrödinger equation yields

=−



Ω

, (73.30)

= ∆

−



Ω

+Ω



(73.31)

= ∆

−



Ω

, (73.32)

where the ∆s are the detunings and the ΩsaretheRabi

frequencies for the dipole-allowed transitions. For ex-

ample, Ω

= d

E / ,and∆

= (E

−E

)/ −ω and

∆

= (E

− E

)/ −2ω.

If the states |j are not too close to resonance, the

oscillate rapidly and to a ﬁrst approximation average

to zero. A better approximation is to retain the small

nonzero solution for b

obtained by setting db

/dt = 0

in (73.31) to obtain

=−



Ω

+Ω



2∆

, (73.33)

a) b)

e

j

g

e

g

j

Fig. 73.7a,b Model two-photon resonances. Two photons

couple the ground state |g with an excited state |e.Many

intermediate nonresonant levels |j are present. In

(a) we

have the cascade system, and in

(b) the Λ pump–probe

system

Part F 73.6

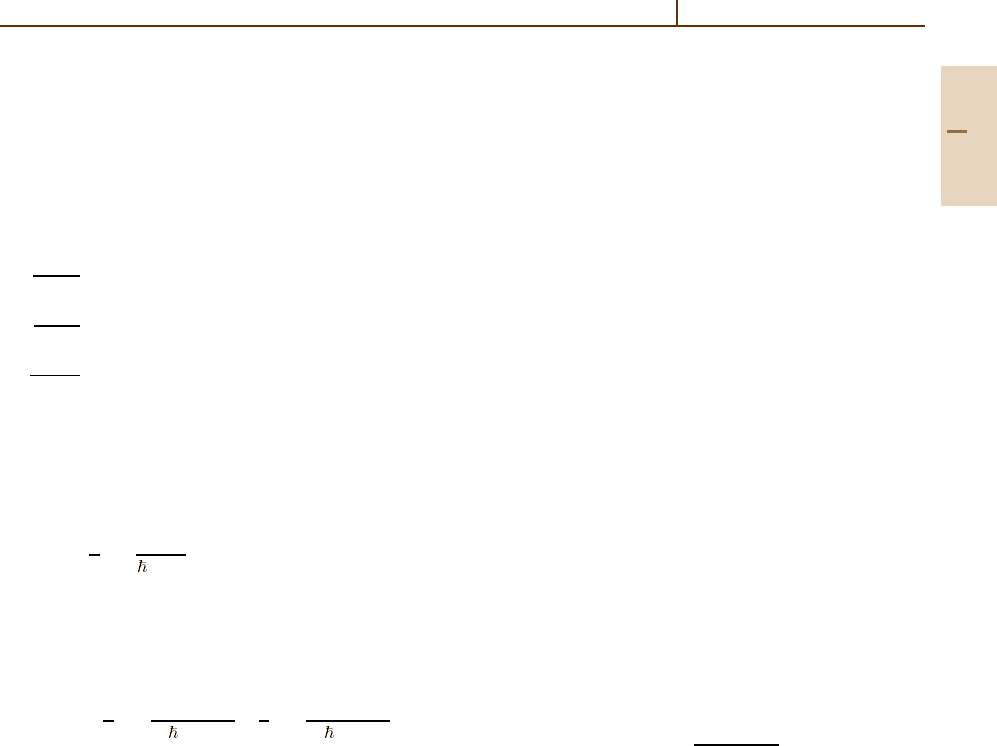
Coherent Transients 73.6 Multi-Level Generalizations 1073

which can be used to eliminate b

from the equations

for a

and a

. This is called adiabatic elimination of

dipole coherence. In this approximation, levels |g and

|e are directly coupled to each other and two-photon

coherence arises. The coupling of levels |g and |e is

similar to the two-level coupling described in Sect. 73.1

and two-photon Bloch equations analogous to (73.5)are

the result:

(2)

=−∆

(2)

= ∆

(2)

+Ω

(2)

=−Ω

(2)

, (73.34)

Here the superscript (2) indicates that the variables are

identiﬁed with the two-photon |g→|e transition.

The various coefﬁcients are similarly general-

ized [73.11]. For example, the two-photon Rabi

frequency is given by

Ω

(2)

≡



∆

. (73.35)

and the two-photon detuning ∆

(2)

incorporates the laser-

induced level shifts

∆

(2)

≡ ∆

+∆



(t)

∆



(t)

∆

(73.36)

The last two terms give the difference in the ac Stark

shifts of the upper and lower levels produced by the

laser ﬁeld.

(2)

is the inversion as before, but U

(2)

and V

(2)

are

somewhat different. They cannot be directly tied to the

expectation value of a dipole moment because levels |g

and |e have the same parity. Thus, while the quantity

(2)

−iV

(2)

is the two-photon analog of U −iV in the

original OBE’s, it cannot serve as a source term in the

Maxwell equation.

In the case of cw applied ﬁelds, the solutions to the

two-photon OBEs are formally identical to those for

a two-level atom. In the case of pulsed ﬁelds, how-

ever, the detuning ∆

(2)

(t) is automatically “chirped”

in frequency by the Stark shifts. This chirping may

signiﬁcantly modify the dynamics. Multiphoton gener-

alizations of the Bloch equations can be made for other

arrangements and numbers of levels. The two-photon

version applies as well to three-level Λ and V conﬁgu-

rations as to the cascade system shown in Fig. 73.7a, for

which they were derived.

73.6.3 Pump–Probe Resonance

and Dark States

Dark states or trapping states occur whenever a ﬁeld-

dependent linear combination of active levels is

dynamically disconnected from the other levels. This

occurs, e.g., in a pump-probe interaction, which ﬁts the

scenario of Fig. 73.7 if two lasers instead of one are used

to excite level |e from the ground level via two-photon

resonance. A strong steady laser a is applied for the

|g→|j transitions and a weak tunable “probe” laser

b for the |j→|e transitions. In the simplest format,

∆

= 0, and all the |j levels can be combined into

a single level labeled |2.

The three-level state vector can be written in terms

of ﬁeld-free states

|Ψ(t)=a

|g+b

|2+a

|e , (73.37)

or, in terms of ﬁeld-dependent dressed states

|Ψ(t)=A

|T +b

|2+A

|S , (73.38)

where Ω|T ≡Ω

|e−Ω

|g, Ω|S≡Ω

|g+Ω

|e

and

(t) ≡ Ω

−1

[Ω

+Ω

] ,

(t) ≡ Ω

−1

[Ω

−Ω

] . (73.39)

The normalizing factor is Ω ≡



Ω

+Ω

The state |T  is an eigenvector of the three-level

RWA Hamiltonian, with eigenvalue zero, and the ampli-

tude A

(t) is a constant of motion. Thus |T  is termed

a trapping state, and population in |T  is inaccessible to

the (possibly very strong) laser ﬁelds. At two-photon res-

onance this conclusion is robust, not depending strongly

on the idealized conditions assumed here. In fact, A

the trapping state amplitude, is an adiabatic invariant, re-

maining constant to ﬁrst order even under slow changes

in Ω

and Ω

. In a pump-probe experiment, this trapping

state is observed as an abrupt drop in probe absorption

as the probe frequency is tuned through two-photon res-

onance. Since only two-photon resonance is required

(both transitions can be equally detuned) this coherent

transient effect has no analog in two-level physics.

The ideal method for exciting the trapping state from

the ground state uses another coherent transient process

called counter-intuitive pulse sequencing in which pulse

b is turned on ﬁrst. The trapping state |T  is essentially

Part F 73.6

1074 Part F Quantum Optics

the ground state |g if Ω

= 0. Thus if Ω

is turned

on ﬁrst, and then Ω

turned on later, the ground state

adiabatically becomes the trapping state and all initial

probability ﬂows smoothly with it.

An essential point is the ease with which pump-

probe adiabaticity is maintained, particularly for strong

ﬁelds on resonance, in contrast to one-photon adiabatic-

ity, which is never achieved at strong-ﬁeld resonance. In

the pump-probe case one must only satisfy the inequality



dΩ

Ω

−

dΩ

Ω







Ω

+Ω



3/2

, (73.40)

which is automatically accomplished by counter-

intuitive pulse sequencing. The inequality allows one

to tolerate rapid change of Ω

while Ω

= 0. Then af-

ter Ω

has reached a high value, Ω

can also be turned

on very rapidly because the right side of (73.40)isal-

ready very large. This is “counter-intuitive” excitation

because if the population is in level |g it is “natural”

to turn on pulse Ω

ﬁrst, not Ω

. It can be dramatically

beneﬁcial to use counter-intuitive excitation when it is

important to avoid relaxation associated with level |2.

73.6.4 Three-Level Transparency

The foregoing results for three-level excitation can be

extended to resonant pulse propagation in three-level

media. The equations governing simultaneous two-pulse

evolution in the local-time coordinates cT ≡ ct −z and

ζ ≡ z are

∂E

∂ζ

= i

∗

∂E

∂ζ

= i

∗

, (73.41)

where

4πd

Nω

, etc.

(73.42)

Note that the bilinear combination 2a

∗

corresponds to

U −iV in (73.16).

Soliton-like pulses can propagate in three-level me-

dia. Both pulses must compete for interaction with level

|2. They depend only on a single variable Z ≡ ζ −uT

where u is the pulse’s constant velocity in the moving

frame. Soliton solutions are given (for µ

= µ

)forΛ

media by

= /d

= A sech KZ ,

= /d

= B tanh KZ , (73.43)

and

=−tanh KZ

−2iKu

sech KZ ,

(73.44)

sech KZ ,

where the parameters A, B, Ku are nonlinearly related

to the pulse length τ:

Ku ≡ 1/τ and



2/τ



= A

− B

. (73.45)

The moving frame velocity is given by 1/u = 2µ/A

and the expression for the lab frame velocity V is 1/V =

1/c +2µ/ A

If B →0, then E

→



2 /τd



sechKZ, which is the

exact McCall–Hahn formula for the two-level one-pulse

soliton amplitude [73.1]. No adiabatic condition was in-

voked in obtaining the soliton solutions. The physical

measure of adiabaticity comes from the pulse duration

τ.Ifτ is short, an appreciable population appears tran-

siently in level |2,butifτ is long (an adiabatic pulse),

the population skips level |2 and goes directly from |g

to |e and back again during the pulse.

Note that the sech and tanh functions are ideally

counter-intuitive, with pulse b starting inﬁnitely far

ahead of pulse a. In practice, the inﬁnite leading edge of

the tanh function plays no role and can be truncated to

several times τ without appreciable change in the char-

acter of the pulse pair.

73.7 Disentanglement and “Sudden Death” of Coherent Transients

The existence of entanglement (non-separability) of

states is the most prominent evidence that quantum me-

chanics is a truly nonlocal theory. This has consequences

for coherent transients, allowing them to exhibit non-

intuitive effects unlike any discussed up to this point.

We will choose an example that directly illustrates this

point by showing that two entangled atoms whose in-

version and coherence decay exponentially can have an

entanglement that not only does not decay exponen-

tially but which reaches its steady state long before

the atoms reach their ﬁnal states. In this sense the

nonlocal transients of the pair of atoms are not at all

Part F 73.7

Coherent Transients 73.7 Disentanglement and “Sudden Death” of Coherent Transients 1075

intuitively related to the local transients affecting the

atoms separately.

We imagine the situation sketched in Fig. 73.8,

two two-level atoms A and B of the type discussed

at length already, prepared in a partially excited en-

tangled mixed state and located remotely from each

other, without direct or indirect interaction. They each

must eventually, because of spontaneous emission, come

to their ground states, creating the ﬁnal joint state

|−

⊗|−

, which is clearly in factored form (disen-

tangled). The question is, what is the manner of evolution

by which the quantum entanglement feature evolves

toward zero.

The standard Master Equation methods [73.12]

for investigating spontaneous emission [73.13] can be

applied to each atom separately since they are not inter-

acting with each other. For any initial state ρ

(0),the

density operator at t can be expressed as

(t) =



µ=1

(t)ρ

(0)K

†

(t), (73.46)

where the so-called Kraus operators [73.14] K

(t) are

available in closed form in this case [73.15].

For illustration we will choose a partially coherent

initial state, expressed by a two-atom density matrix with

a single free parameter a:

(0) =







a 00 0

011 0

0001−a







(73.47)

Here the convention is to label the rows and columns

in the order ++, +−, −+, −−. The transient decay

of either atom’s excitation can be calculated separately

from their reduced density matrices ρ

≡Tr

{ρ

},etc.

For example:

=+

|ρ

+−

|ρ

|−





1 +a 0

02−a



(73.48)

and the upper level excitation of atom A can

be found to behave exactly as expected: ρ

(t) =

a+1

−t/τ

,whereτ

is the usual spontaneous emission

lifetime.

For nonlocal transients we will need a time-

dependent measure of entanglement, and there are

several options [73.16], all related to the joint entropy of

the two-atom system. We will use the concurrence C(t)

Atom A Atom B

Cavity A Cavity B

Fig. 73.8 Illustration of a set-up in which two partially

excited atoms A and B are located inside two spatially

separated cavities that are possibly very remote from each

other. The two atoms are assumed initially entangled, but

they have no interaction

of Wootters [73.17], which has the convenient normal-

ization 1 ≥C(t) ≥ 0, where C =1 represents completely

entangled atoms (such as in a pure Bell state, for ex-

ample) and C = 0 denotes the complete absence of

entanglement.

For the speciﬁc case a = 1 one ﬁnds for the state

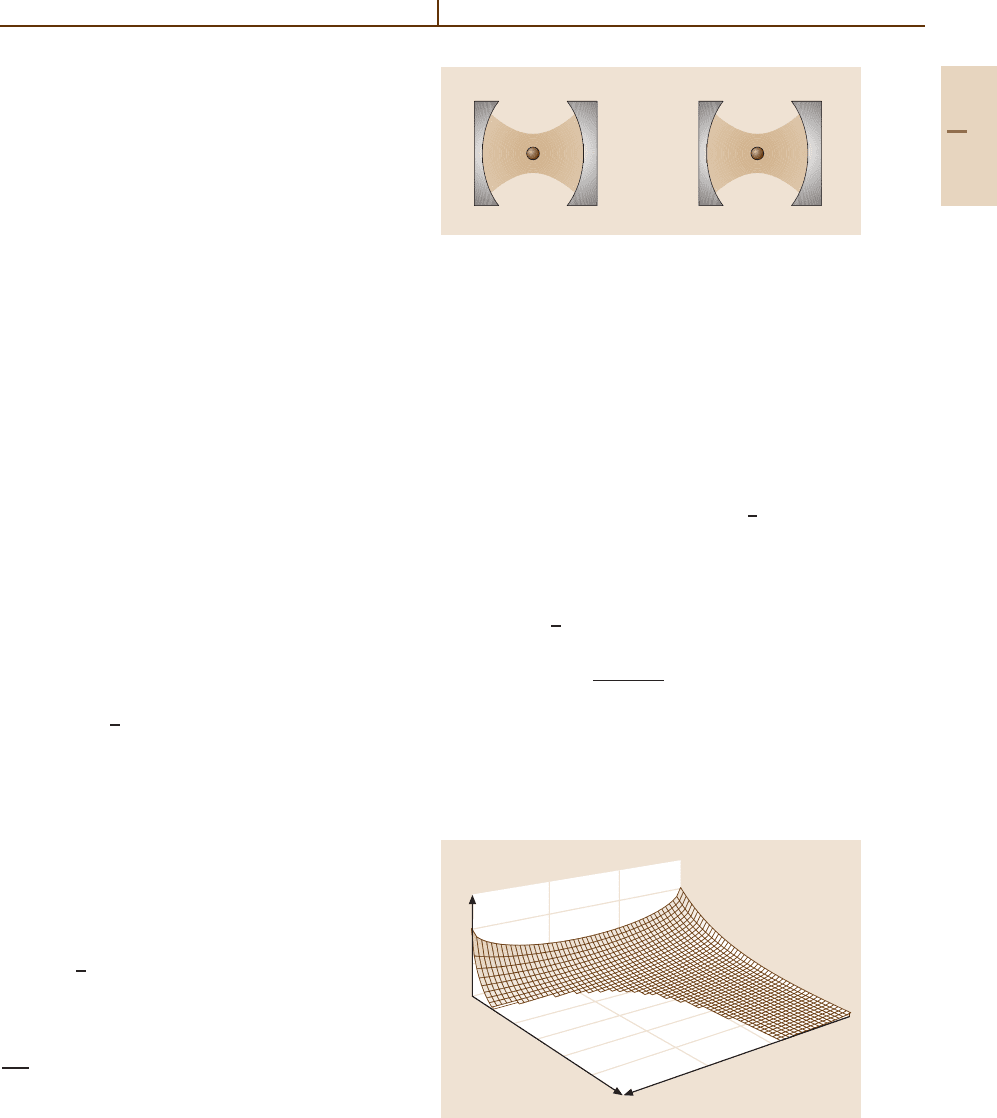
(73.47) the initial concurrence C(0) =

, indicating a

state with partial two-party coherence (incomplete en-

tanglement). At time t one then ﬁnds:

(t) =

max



0, e

−t/τ

f(t)



, (73.49)

where f(t) = 1 −

√

2ω

+ω

, and ω ≡ 1 − e

−t/τ

.The

strikingly non-intuitive consequence of this expression

is that C(t) → 0 abruptly after a short time (disentan-

glement suffers a “sudden death”) if 2ω

+ω

≥ 1. In

fact, only a minor algebraic rearrangement shows that

this strange condition must occur, and the ﬁnite sudden

⁄

0.5

2.5

⁄

C( )

Fig. 73.9 Effect of spontaneous emission on concurrence

of two two-level atoms given the initially entangled mixed

state (73.47) depending on the single parameter 1 ≥ a ≥ 0

Part F 73.7

1076 Part F Quantum Optics

death time t

is given by

≡ ln



2 +

√



≈ 0.53 .

(73.50)

The non-local coherent transient behavior of entangle-

ment for the entire range of allowed a values [73.15]is

shown in Fig. 73.9. This shows that the concurrence un-

dergoes familiar smooth and inﬁnitely long decay only

for a values in the limited range

> a ≥ 0. Otherwise

sudden death occurs sooner or later. In our example,

non-local coherence becomes zero most abruptly after

a ﬁnite time for a = 1, the case that was calculated in

(73.49). Although not yet observed experimentally, it

appears that these results are not exceptional. Sudden

termination of entanglement has also been predicted for

two-party continuum states as well as for qubit pairs ex-

periencing only T

decay, in contrast to the combined

and T

decay appropriate to spontaneous emission as

treated here.

References

73.1 L. Allen, J. H. Eberly: Optical Resonance and Two-

Level Atoms (Dover, New York 1987)

73.2 B. W. Shore: Theory of Coherent Atomic Excitation,

Vol. 1,2 (Wiley, New York 1990)

73.3 P. Meystre, M. Sargent III: Elements of Quantum

Optics (Springer, Berlin, Heidelberg 1990)

73.4 C. Cohen-Tannoudji, J. Dupont-Roc, G. Grynberg:

Atom-Photon Interactions (Wiley, New York 1992)

73.5 M. O. Scully, M. S. Zubairy: Quantum Optics (Cam-

bridge Univ. Press, Cambridge 1997)

73.6 W. P. Schleich: Quantum Optics in Phase Space

(Wiley-VCH, New York 2001)

73.7 C. C. Gerry, P. L. Knight: Introductory Quantum Op-

tics (Cambridge Univ. Press, Cambridge 2005)

73.8 P. W. Milonni, J. H. Eberly: Lasers (Wiley, New York

1988)

73.9 M. V. Fedorov: Atomic and Free Electrons in a Strong

Light Field (World Scientiﬁc, Singapore 1997)

73.10 J. D. Jackson: Classical Electrodynamics, 2nd edn.

(Wiley, New York 1975)

73.11 F. H. M. Faisal: Theory of Multiphoton Processes

(Plenum, New York 1987)

73.12 See Sect. 78.7 in this book

73.13 See Sect. 78.12 in this book

73.14 A complete discussion of Kraus operators appropri-

ate to Bloch vector evolution is given in S. Daffer,

K. W

odkiewicz, J. K. McIver: J. Mod. Optics 51,1843

(2004)

73.15 Ting Yu, J. H. Eberly: Phys. Rev. Lett. 93, 140404

(2004)

73.16 See Chapt. 81 in this book

73.17 W. K. Wootters: Phys. Rev. Lett. 80, 2245 (1998)

Part F 73

1077

Multiphoton a

74. Multiphoton and Strong-Field Processes

The excitation of atoms by intense laser pulses can

be divided into two broad regimes: the ﬁrst regime

involves relatively weak optical laser ﬁelds of long

duration, and the second involves strong ﬁelds

of short duration. In the ﬁrst case, the intensity

is presumed to be high enough for multiphoton

transitions to occur. The resulting spectroscopy is

not limited by the single-photon selection rules

for radiative transitions. However, the intensity

is still low enough for a theoretical description

based on perturbations of ﬁeld-free atomic states

to be valid, and the time dependence of the

ﬁeld amplitude does not play an essential role.

In the second case, the ﬁeld intensities are too

large to be treated by perturbation theory, and

thetimedependenceofthepulsemustbetaken

into account. A discussion on the generation of

sub-femtosecond pulses is included.

74.1 Weak Field Multiphoton Processes ........1078

74.1.1 Perturbation Theory...................1078

74.1.2 Resonant Enhanced

Multiphoton Ionization..............1078

74.1.3 Multi-Electron Effects ................ 1079

74.1.4 Autoionization..........................1079

74.1.5 Coherence and Statistics ............ 1079

74.1.6 Effects of Field Fluctuations ........1079

74.1.7 Excitation with Multiple

Laser Fields .............................. 1080

74.2 Strong-Field Multiphoton Processes ......1080

74.2.1 Nonperturbative Multiphoton

Ionization ................................1081

74.2.2 Tunneling Ionization ................. 1081

74.2.3 Multiple Ionization.................... 1081

74.2.4 Above Threshold Ionization ........ 1081

74.2.5 High Harmonic Generation ......... 1082

74.2.6 Stabilization of Atoms

in Intense Laser Fields ............... 1083

74.2.7 Molecules in Intense

Laser Fields .............................. 1084

74.2.8 Microwave Ionization

of Rydberg Atoms ......................1084

74.3 Strong-Field Calculational Techniques ... 1086

74.3.1 Floquet Theory..........................1086

74.3.2 Direct Integration of the TDSE .....1086

74.3.3 Volkov States ............................1086

74.3.4 Strong Field Approximations....... 1087

74.3.5 Phase Space Averaging Method ... 1087

References .................................................. 1088

The excitation of atoms by intense laser pulses can

be divided into two broad regimes determined by the

characteristics of the laser pulse relative to the atomic

response. The ﬁrst regime involves relatively weak op-

tical laser ﬁelds of long duration (> 1 ns), and the second

involves strong ﬁelds of short duration (< 10 ps). These

will be referred to as the weak-long (WL) and strong-

short (SS) cases respectively.

In the case of atomic excitation by WL pulses, the

intensity is presumed to be high enough for multiphoton

transitions to occur. The resulting spectroscopy of ab-

sorption to excited states is potentially much richer

than single-photon excitation because it is not lim-

ited by the single-photon selection rules for radiative

transitions. However, the intensity is still low enough

for a theoretical description based on perturbations of

ﬁeld-free atomic states to be valid, and the time depen-

dence of the ﬁeld amplitude does not play an essential

role.

The SS case is fundamentally different in that the

atomic electrons are strongly driven by ﬁelds too large

to be treated by perturbation theory, and the time depen-

dence of the pulse as it switches on and off must be taken

into account. Atoms may absorb hundreds of photons,

leading to the emission of one or more electrons, as well

as photons of both lower and higher energy. Because

the ﬂux of incident photons is high, a classical descrip-

tion of the laser ﬁeld is adequate, but the time dependent

Schrödinger equation (TDSE) must be solved directly

to obtain an accurate representation of the atom–ﬁeld

interaction.

For SS pulses of optical wavelength, it is sufﬁcient

in most cases to consider only the electric dipole (E1)

interaction term deﬁned in Chapt. 68. The atom–ﬁeld

Part F 74