De Felice F., Bini D. Classical Measurements in Curved Space-Times

Подождите немного. Документ загружается.

9.3 Measurements in Kerr space-time 203

as is known a priori, that the background geometry is that of the Kerr solution

and that the orbit of the ﬁducial particle is equatorial and spatially circular and

the frame of reference is the one described in (9.36).

As in the Schwarzschild case, consider a particle which can only move in the

θ direction in a frictionless pipe, perpendicular to the orbital plane. In order

to know what kind of motion a test particle undergoes in that direction, being

otherwise constrained to rotate rigidly with the space-ship, we impose in the

second equation of (9.37) the conditions a(U)

=0and∂

a(U)

= 0 for all

values of θ. As a consequence, recalling that the observer moves in the equatorial

plane, we obtain the equation of motion

dτ

= −G

(r; ζ,a,M)Y

, (9.45)

where

2M[ζr

− a(1 −aζ)]

+ ζ

[1 −

(1 −aζ)

− ζ

+ a

)]

, (9.46)

from (9.37).

Function G

is positive in the region of physical interest, namely for ζ ∈

(ζ

c−

,ζ

), where ζ

c,±

are the zeroes of the denominator of (9.46). This justi-

ﬁes the choice of a square, as G

,in(9.46); see Fig. 9.4. Clearly equation (9.45)

describes a harmonic motion in the

θ direction with frequency |G|, and when that

happens the direction of the oscillations ﬁxes the

θ direction of the frame and,

at the same time, the equatorial plane.

In the Schwarzschild case the frequency of the harmonic oscillations was just

the proper angular velocity of the orbital revolution, a value which |G| reduces

to when a = 0. Contrary to that case, however, the quantity |G| does not give

direct information about the angular velocity of the orbital revolution. Following

de Felice and Usseglio-Tomasset (1996) we write (9.46) in terms of the reduced

frequency y and obtain

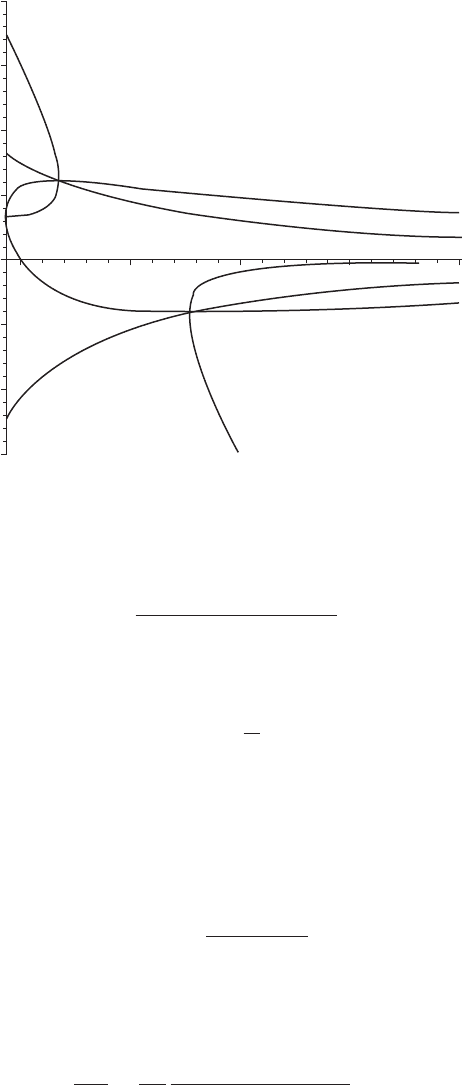
0.1

–0.1 0.1

Fig. 9.4. Behavior of G

as a function of ζ for ﬁxed values of r =4M,with

a =0.5M and M = 1. Note that when ζ = 0, with the choice of parameters

used, one has G

−10

= 0, invisible on this scale.

204 Measurements in physically relevant space-times

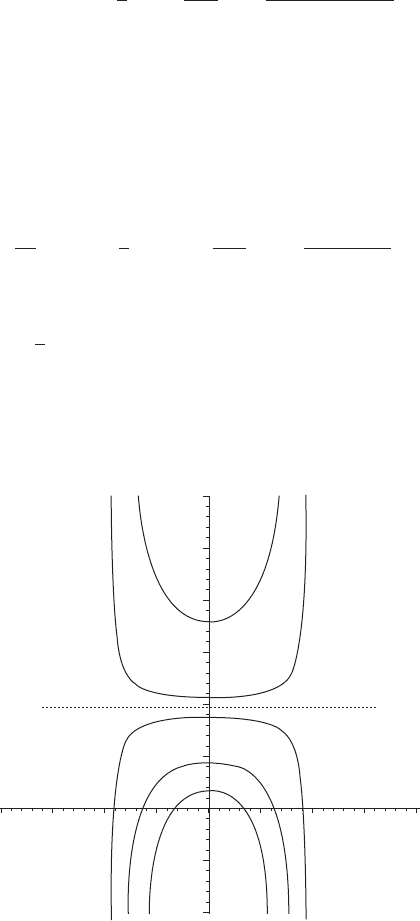
0.8

(crit)+

(crit) –

c –

K+

K–

0.6

0.4

0.2

–0.2

–0.4

–0.6

2345

r /M

Fig. 9.5. The map of the permitted equatorial circular orbits in the Kerr metric.

and ζ

c−

are the limits of permitted angular velocities. ζ

K+

and ζ

K−

are

the corotating and counter-rotating circular geodesics. ζ

(crit)−

and ζ

(crit)+

are

the counter-rotating and corotating extremely accelerated circular orbits.

2M(yr

− a)

+ y

− y)(y −y

c−

)

. (9.47)

Here y

c±

are solutions of the equation Γ

−1

= 0 with θ = π/2; from (9.35)

these are

c±

=(a ±

√

Δ)/r

. (9.48)

Spatially circular equatorial motion is then allowed in the range y

c−

<y<y

(de Felice, 1994). An intriguing implication of (9.47) is that an observer at rest

with respect to inﬁnity (ζ =0=y) would still see a harmonic oscillation in the

θ direction, with a proper frequency square

y=0

2Ma

(r − 2M)

. (9.49)

The value (9.49), however, is not the minimum which can be attained by |G|.

Diﬀerentiating (9.47) with respect to y, we obtain

∂G

∂y

2Δ

+ r

y −2Ma

− y)

(y − y

c−

)

. (9.50)

9.3 Measurements in Kerr space-time 205

This vanishes at



−1 ±



8Ma

≡

∗

. (9.51)

It is easily proven (de Felice and Usseglio-Tomasset, 1996; see Exercise 43) that

(i)

∗

−

c−

; this is disregarded, being outside the range of the permitted angu-

lar velocities for circular motion;

(ii) y

c−

≤

∗

≤ y

, the equality sign holding only on the event horizons (i.e. at

r = r

solutions of Δ = 0) and for r →∞.

From (ii) one is sure that the orbiting observer can always measure the minimum

frequency of the harmonic oscillation by varying y in the permitted range of its

values. Because

∗

> 0, the minimum of G

only occurs at a positive value of ζ;

hence the observer who brings the angular frequency of the harmonic oscillations

to a minimum would deduce that its trajectory is corotating with the black

hole. However the property of

∗

of being always positive has an intriguing

consequence. In the range 0 <y<

∗

, an increase of y causes a decrease in

|G|; hence the orbiting observer who sees |G| decrease as a consequence of a

variation of y from within his space-ship is unable to decide whether he was

decelerating the space-ship – a decrease of |y| – in which case the angular velocity

of revolution would have been in the ranges y

c−

<y<0or

∗

<y<y

or was accelerating it – an increase of |y| – meaning that y was in the range

0 <y<

∗

. Even assuming a priori a certain amount of information, e.g. being

in the Kerr metric in a spatially equatorial circular orbit and with a phase-locked

frame, a measurement of |G| leads to ambiguous information. This ambiguity

could probably be eliminated with the help of a larger set of measurements, but

this will not be considered here.

Finally, let us now compare

∗

with y

(ZAMO)

, the reduced angular velocity

corresponding to ζ

(ZAMO)

=2Mra/A (de Felice, 1994):

(ZAMO)

2Ma

r(r

+ a

)

Simple algebra shows that

∗

≥ y

(ZAMO)

for Δ ≥ 0, the equality sign hold-

ing also at the limit r →∞. In particular we deduce that as r →∞,

∗

≈ 2Ma/r

− 4M

+ ··· while y

(ZAMO)

≈ 2Ma/r

− 2Ma

+ ···,

showing that to the lowest order in a the two functions asymptotically coincide.

In slow rotation, then, an orbiting observer would detect the minimum frequency

of the harmonic oscillation about the orbital plane when the angular velocity of

the orbital revolution was equal to the gravitational drag (ζ

(ZAMO)

A behavior which appears to be induced by gravitational drag without being

completely justiﬁed by it is encountered in the eﬀect we discuss next.

206 Measurements in physically relevant space-times

9.4 Relativistic thrust anomaly

In Schwarzschild space-time, a collection of particles moving in spatially circular

orbits have an acceleration relative to the phase-locked frame (9.6) given by

a(U)

ˆa

= δ

ˆaˆr



1 −



1/2

(ζ

− ζ

)

(ζ

− ζ

sin

θ)

. (9.52)

This quantity is the speciﬁc thrust which acts on each particle to keep its orbit

spatially circular. It is directly measurable and, by convention, acts outwardly

if it is positive and inwardly if it is negative. In the plane θ = π/2thethrust

becomes independent of ζ at r =3M, where ζ

= ζ

. The behavior of the

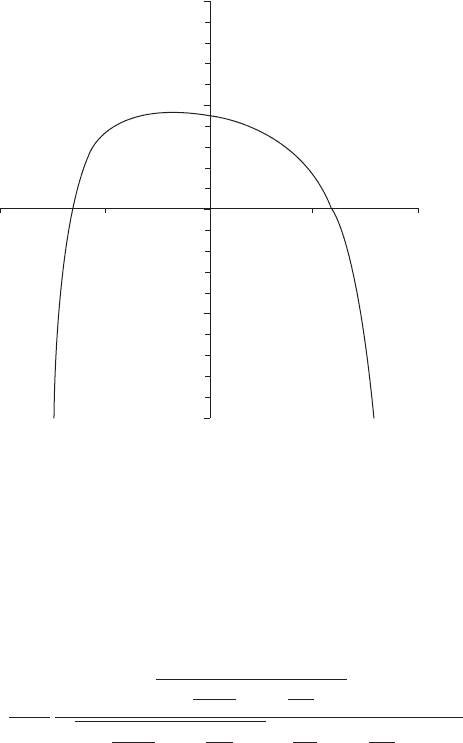
thrust as a function of ζ is shown in Fig. 9.6; clearly at any radius r =3M the

thrust takes its extreme value at ζ = 0, as is easily inferred from

∂

∂ζ

a(U)

ˆa

ˆaˆr



1 −



1/2

− ζ

(ζ

− ζ

)

, (9.53)

while at r =3M it vanishes identically for any ζ. Moreover, at that radius all

derivatives of the thrust with respect to ζ vanish; hence the thrust is constant

and equal to 1/(3

√

3M). At r<3M the thrust is always positive, meaning

that it acts outwardly for all ζ and, most peculiarly, increases with |ζ| in the

outward direction (Abramowicz and Lasota, 1974). This behavior suggests, as

already pointed out, that an increase in the angular velocity contributes to the

0.6

0.5

0.4

0.3

0.2

0.1

–0.1

–0.2

–0.4 –0.3 –0.2 –0.1 0.1 0.2 0.3

> 3M

< 3M

a (U )

0.4

Fig. 9.6. The behavior of the thrust as a function of the angular velocity of

revolution ζ at a ﬁxed radius r in Schwarzschild space-time.

9.4 Relativistic thrust anomaly 207

gravitational component of the thrust more than it does to the centrifugal one

(de Felice, 1991; Semer´ak, 1994; 1995).

As already established, there is a surprising analogy between the behavior of

the gradient of the thrust with respect to ζ and that of a gyroscopic precession.

Recalling its expression in the Schwarzschild metric (9.30), we ﬁnd that the gyro-

scopic precession is forward at r<3M, while it is backward at r>3M, being

zero at r =3M. This behavior is consistent with an increasing gravitational

strength as one approaches the horizon at r =2M; however, a more careful

analysis shows that the phenomenon is related to a form of gravitational drag

which is not induced by the rotation of the metric source. We shall refer to it as

a gravitational grip. Clearly in Schwarzschild space-time we have only gravita-

tional grip, while both types of eﬀect exist in Kerr space-time. Since the axis of

a gyroscope is Fermi-Walker transported along its own trajectory (the spatially

circular orbits in our case), the absence of precession at r =3M,thatis

ζ =0,

implies that the gravitational grip forces the phase-locked frame to become a

Fermi frame at that radius.

Let us now see how the situation changes if we consider the Kerr background.

If the metric source is a rotating black hole then the above eﬀect manifests itself

not only at small coordinate distances from the outer event-horizon on corotating

equatorial circular orbits, but also arbitrarily far away from the source on counter-

rotating circular orbits with a ﬁnite range of angular velocities which vanish at

inﬁnity.

This behavior has no Newtonian analog and its occurrence at asymptotic dis-

tances from a rotating source is a combination of grip and drag, giving rise to a

measurable new test of general relativity.

The speciﬁc thrust associated with a general non-geodesic equatorial spatially

circular orbit in the Kerr metric is given, from de Felice and Usseglio-Tomasset

(1991) and de Felice (1994), by

a(U)=

1/2

(y − y

K+

)(y − y

K−

)

(y − y

)(y − y

c−

)

,θ= π/2, (9.54)

where y is the reduced frequency (9.39), while y

K±

and y

c±

are given by Eqs.

(9.41) and (9.48) respectively. The response of the thrust to a change in the

reduced angular velocity y ataﬁxedr (and a) is illustrated by the function

∂a(U)

∂y

= −

2aΔ

1/2

(y −y

(crit)+

)(y − y

(crit)−

)

(y − y

)

(y − y

c−

)

, (9.55)

where

(crit)±

= −

⎡

⎣

1 −

∓





1 −



−

4Ma

⎤

⎦

(9.56)

identify the orbits with extreme acceleration. Clearly y

(crit)−

is always negative

and vanishes at inﬁnity as ∼r

−3

, remaining larger than y

K−

, which vanishes

208 Measurements in physically relevant space-times

0.2

0.1

–0.1

–0.2

–0.2 –0.1 0.1 0.2

K–

K+

a (U )

(crit)–

Fig. 9.7. The behavior of the thrust as a function of the angular velocity of

revolution ζ in equatorial spatially circular orbits at r =4M in the Kerr metric

with a =0.5M.

asymptotically as ∼r

−3/2

. In Fig. 9.5 we show the behavior of ζ

c±

,ζ

K±

,and

(crit)±

(equivalently, of y

c±

K±

(crit)±

) as functions of r.From(9.54), (9.55),

and (9.56) we deduce how the speciﬁc thrust a(U ) behaves with y at a ﬁxed r.

This is shown in Fig. 9.7. Setting y = y

(crit)−

, the maximum of a(U) takes the

value

a(U

(crit)−

1/2



1 −

4Ma



1 −



−2

− 1



1 −

4Ma



1 −



−2

−



1 −



−1

− 1

. (9.57)

As we see, when the metric source is rotating, the maximum of the thrust at

asymptotic distances is displaced to negative values of y; hence its anomalous

behavior occurs in the small interval (y

(crit)−

, 0). In fact, an increase of |y| from

0to|y

(crit)−

| implies, contrary to intuition, an increase of the thrust outwardly

(being a(U) > 0). It appears that an increase of |y| in the above range causes a

loss of energy, which would let the orbit plunge into the source unless a larger

radial thrust were applied outwardly. The behavior of the thrust appears to

be a response to both gravitational drag and grip, since the same behavior is

met in corotating circular orbits in the Kerr metric (de Felice, 1994) and in the

Schwarzschild metric, where no drag exists at all.

In both the rotating and the non-rotating cases the condition of extreme accel-

eration corresponds to the vanishing of the precession of a gyroscope. From de

Felice (1994) we deduce the angular velocity of precession of a gyroscopic when

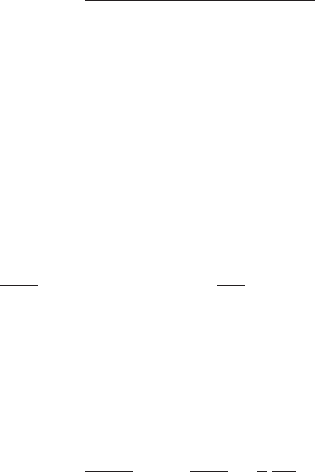
9.4 Relativistic thrust anomaly 209

the latter moves in equatorial spatially circular orbits. In the Kerr metric this is

given by

ζ =

a(y −y

(crit)+

)(y − y

(crit)−

)

(y − y

)(y − y

c−

)

. (9.58)

At each value of the radius r, then, a Fermi frame counter-rotating with the

metric source and with angular velocity of revolution equal to |y

(crit)−

| is made

to coincide to a phase-locked frame. This eﬀect can in principle be tested, as we

shall see.

Let us suppose that the rotating source is the Earth. Neglecting deviations

from sphericity, its space-time is described by the Lense-Thirring metric (Lense

and Thirring, 1918; de Felice, 1968). The latter coincides with the Kerr metric

in the weak ﬁeld limit M/r  1anda/r  1. At the Earth’s surface we have

⊕

≈ 6.9 × 10

−10

⊕

≈ 5.4 × 10

−7

, (9.59)

where R

⊕

≈ 6.37103 × 10

cm, M

⊕

≡ GM

⊕

=0.443 cm, M

⊕

≈ (5.977 ±

0.004)×10

g, a

⊕

≡J

⊕

/(cM

⊕

) ≈ 3.4×10

cm, taking J

⊕

≈ 5.9×10

gcm

−1

In conventional units (cm s

−2

), the speciﬁc thrust is given by

f ≡ c

a(U); its

extreme values are, from (9.57),

(crit)−

≈

⊕



⊕

+ ···



, (9.60)

where r

⊕

= αR

⊕

, with α>1, is the radius of the orbit of the space-ship. Let

this orbit be a counter-rotating equatorial spatially circular geodesic with radius

⊕

. We then consider the device described in Section 8.2, namely a rigid box free

to slide with negligible friction on rails which run across the ship tangentially

to the orbit. We require that the center of the box follows the trajectory of the

baricenter of the ship, moving therefore on a geodesic. A test point-like mass

is held at the center of the box by sensors which trigger small thrusters. As

stated, the test mass moves initially on a geodesic; therefore the sensors exert

no net acceleration on the mass. Now let the box be set in motion within the

space-ship, along the rails in the direction opposite to the ship’s motion. The

latter being counter-rotating, the test mass will acquire with respect to inﬁnity

an angular velocity

ζ in magnitude smaller than that allowed for a geodesic. As

a consequence, the test mass will be acted upon by a thrust which keeps it to the

center of the box on the same orbital radius. If we decrease the value of |

ζ| of the

test mass by increasing the velocity of the box within the space-ship, the thrust

will ultimately reach a maximum value at

ζ = ζ

(crit)−

. In the non-rotating case

(a = 0) the maximum occurs when

ζ = 0, i.e. when the velocity of the test mass,

as seen from inﬁnity, is equal in magnitude but opposite to that of the ship. If

rotational eﬀects are taken into account (a = 0), then the maximum occurs when

the moving test mass has an angular velocity with respect to inﬁnity equal to

210 Measurements in physically relevant space-times

cζ

(crit)−

≈−c

⊕

≈ 1.75 × 10

−14

−3

−1

, (9.61)

from (9.56) and to the lowest order in the relativistic corrections.

While the observer comoving with the ship is able to recognize when the thrust

acting on the test mass reaches a maximum, he cannot distinguish whether that

occurs when

ζ = 0 or when

ζ = ζ

(crit)−

. The latter case would signal a new

relativistic eﬀect. To detect the anomalous behavior of the thrust with |ζ|,one

has to measure, at the maximum of the thrust, the (linear) velocity of the test

mass relative to the space-ship.

Consider an observer who is comoving with the space-ship. If the thrust acting

on the test mass in the box is zero, then he knows that the angular velocity of

the ship with respect to inﬁnity is, from (9.54) and (9.39),

−

≈−



⊕





⊕



⊕





. (9.62)

If the test mass moves within the ship as indicated above, then it acquires an

angular velocity

ζ with respect to inﬁnity, which is smaller in magnitude than

the ship’s ζ

−

. Therefore it will have, relative to the latter, a velocity ν and a

Lorentz factor

γ ≡ γ(U

−

,u)=(1− ν(U

−

,u)

)

−1/2

= −g

αβ

−

, (9.63)

where

−

=Γ(ζ

K−

)(δ

+ ζ

K−

),u

=Γ(

ζ)(δ

ζδ

) (9.64)

are the 4-velocities of the ship and test mass, respectively, with their normaliza-

tion factors given in general by

Γ(ζ)=



1 −

(1 −aζ)

− ζ

+ a

)



−1/2

. (9.65)

To lowest order in the parameters a and M and in the equatorial plane, the

weak-ﬁeld limit of the Kerr metric is given by

≈−



1 −



−

4Ma

dφdt + r



2Ma



dφ



−



. (9.66)

From (9.62), (9.64), and (9.66), the Lorentz factor is, to the lowest order in a/r,

γ ≈ Γ(ζ

K−

)Γ(

ζ)



1 −

− r



2Ma



K−

2Ma

(ζ

K−

ζ)



. (9.67)

9.4 Relativistic thrust anomaly 211

On the Earth we have 2(M/r)(a

) ∼ 10

−23

and therefore we shall neglect

that term. From (9.62) and (9.65)wehave

Γ(ζ

K−

) ≈ 1+





3/2

. (9.68)

If the test mass were at rest with respect to inﬁnity then

ζ = 0 and therefore

Γ(0) ≈ 1+

; (9.69)

hence, from (9.67) and to the lowest order in a/r,

γ|

ζ=0

≈ 1+

−





3/2

−





5/2

. (9.70)

From the latter, and recalling the deﬁnition of the Lorentz factor, we deduce the

velocity of the test mass relative to the space-ship when it is at rest with respect

to inﬁnity:

≈ c





1 −



. (9.71)

This diﬀers from the Keplerian velocity by a fractional change

⊕

≈ α

−1

3.45 ×10

−9

, (9.72)

which is independent of rotation, and a rotationally induced correction given by

⊕



⊕



1/2

≈

3/2

1.41 ×10

−11

. (9.73)

The maximum thrust, however, occurs when the test mass has angular velocity

(9.61). In this case it would be

Γ(ζ

(crit)−

) ≈ 1+

. (9.74)

Hence we neglect the contribution from rotation and set Γ(ζ

(crit)−

) ≈ Γ(0). From

Eqs. (9.61), (9.62), and (9.67) we deduce

γ|

(crit)−

≈ 1+

−

21a





5/2

. (9.75)

As expected, the relative linear velocity of the test mass within the ship is now

lower and is given by

(crit)−

≈ c





1 −

−





3/2

. (9.76)

212 Measurements in physically relevant space-times

In this case the correction due to the Earth’s rotation is of the order of 1.02 ×

−19

, so it can be neglected. In order to detect the general relativistic anomaly in

the behavior of the thrust, the observer in the ship needs to identify the maximum

thrust acting on the test mass when the velocity of the latter is ν

(crit)−

(i.e. when

ζ = ζ

(crit)−

) and not ν

(i.e. when

ζ = 0) as would be natural in the ﬁeld of a

non-rotating source. This requires measuring linear velocities within the ship to

better than ∼10

−11

. As we can see, in fact, the fractional change of the velocity

(crit)−

relative to ν

is given by the factor (a/r)



M/r. Clearly, at this level of

precision the deviation from spherical symmetry of the Earth’s potential must

be known with an accuracy better than ∼10

−11

The obvious conclusion is that the proposed experiment is hardly feasible at

present. Summarizing, one would have to measure the velocity of the test mass

relative to the space-ship to 1 part in 10

, and measure the radial force on the

test mass with an accuracy of 1 part in 10

, in the short interval of time that

the mass could be kept in motion within the ship. Moreover, one has to be sure

that the mass moves within the ship at a constant radius relative to the Earth

and control with high precision the gravity gradients along the path of the test

mass resulting from the mass distribution of the space-ship itself.

9.5 Measurements of black-hole parameters

An important correspondence can be established between measurements per-

formed within a space-ship, as discussed above, and data which can be gathered

with observations “at inﬁnity” (de Felice and Usseglio-Tomasset, 1996; Semer´ak

and de Felice, 1997).

There is considerable observational evidence that most active galactic nuclei

(AGN) and X-ray binaries host black holes (Rees, 1988; 1998). Essential to a

relativistic modeling of these sources is knowledge of the properties of black holes

and of the matter distribution around them. An estimate of their mass has mainly

been based on the energy emission and on the time-scale of their variability

(Begelman, Blandford, and Rees, 1984). In Semer´ak, Karas, and de Felice (1999) a

method was presented for determining, from a set of observations, the parameters

of a system made of a rotating black hole and an accretion disk interacting in

such a way as to power sources with a periodic modulation of variability. In the

case of AGNs or stellar-size X-ray sources, a star orbiting freely around such

a system on a spherical and almost equatorial circular geodesic will play the

role of the test particle which was free to move in a frictionless pipe oriented

in the

θ direction but constrained to rotate rigidly around the compact source.

Such a particle was found to perform in the pipe harmonic oscillations about the

equatorial plane with “proper” frequency |G|. In the astrophysical situation, the

star in a spherical orbit will cross the disk with a frequency which is just twice

|G|. Moreover, from photometric observations one can deduce the frequency of

the orbital revolution; what is observed at inﬁnity, however, would not be the