Davim J.P. Tribology for Engineers: A Practical Guide
Подождите немного. Документ загружается.


89
Lubrication and roughness
The integrals in this solution are the same journal-bearing
integrals (Booker, 1965) as in subsection 3.5.2 (cylindrical
journal bearing).
For determining the integration constants we need a
specifi c body geometry and possible real pressure values
assigned at the boundaries. Consider here boundary
conditions as follows
meaning that a divergent region of pressures is assumed, that
the opposite boundaries have the same pressures, and that
the hydrodynamic effect vanishes on the vertical boundaries.
For the lower and upper θ values, we have
[3.35]
which represent a periodic pattern with cycles
θ
1
–
θ
u
= 2
π
k; l
and u denote the lower and upper integration limits
respectively.
Subject to the assigned boundary conditions, C
1
can be
determined applying the eqs. [3.35] to eq. [3.34], taking
the defi nite integrals between
θ
l
(X = 0) and
θ
u
(X = 1),
and assuming p(
θ
u
) – C
2
= 0 as in the case of a cylindrical
bearing. Further, substituting this expression and then
determining C
2
from the boundary condition P = 0 at Z = 0
associated with
, [3.36]
we have an expression for P(
θ
) which, after the requisite
transformations, reads
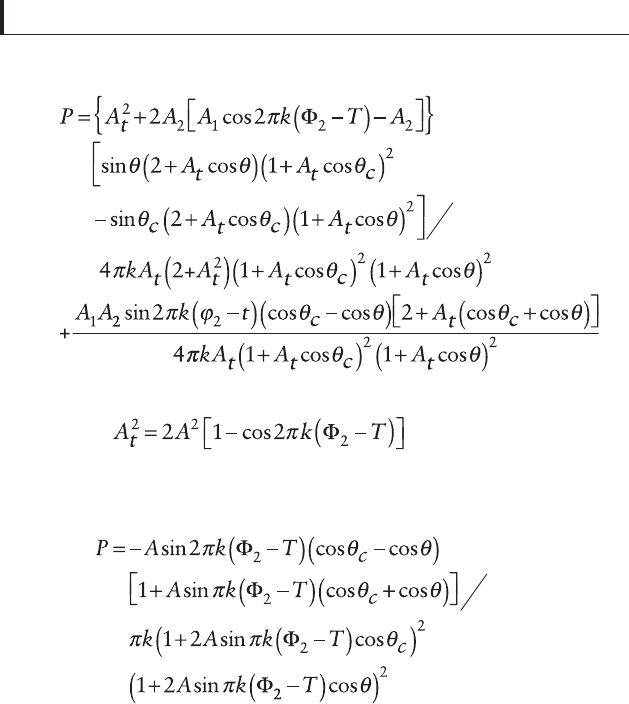
90
Tribology for Engineers
[3.37]
In the particular case of equal asperity heights A
1
= A
2
= A we
have
, the fi rst coeffi cient in
the fi rst term of eq. [3.37] vanishes and the pressure equation
reduces to
[3.38]
Eqs. [3.37] and [3.38] can be reconverted from the variable
θ to the original ones – X, Z and T – by substitution of
expressions [3.30] and [3.36] for
θ
and
θ
C
; the fi nal expression
is very long and is not given here (available in Burstein,
2010); the P,
θ
, and
θ
C
expressions constitute a complete
algebraic set of equations which suffi ces for routine arithmetic
calculations. Typical X, Z – and X, T – pressure distributions
for different roughnesses were calculated with the aid of
these equations and are given in Fig. 3.11(a) and (b). As
the pressure distribution is periodical, its examination for
one wavelength yields complete information about the
surface behaviour as a whole.
91
Lubrication and roughness
Solution for unequal wave numbers
The above relations can be extended to the case of unequal
wave numbers along axes X and Z, wave number ratios
. In this case the gap equation has the form
[3.28] with A
t
as per eq. [3.29] and
θ
given by a relation in
which does not equal 1:
[3.39]
Reynolds’ equation for this case reads
[3.40]
Integrating this equation successively as in the preceding
case, and determining the integration constants from the
same boundary conditions, we easily obtain an analogue of
eq. [3.37] that is valid for the case of different asperity
heights and different wave numbers in the X- and Z-directions
Figure 3.11
Typical pressure distribution in lubricating fi lm
between sinusoidal surfaces with wave
number 2, wave ratio 1, and asperity height of
lower surface 0.5 and upper surface 0.25, at
time 1/(2k) (a) and at coordinate y = 1/(2k) (b).
P
P
Z
X
T
X

92
Tribology for Engineers
[3.41]
with
θ
as per eq. [3.39] and
θ
c
defi ned by
[3.42]
Eq. [3.41] is more general than eq. [3.37], being valid for
both = 1 and ≠ 1.
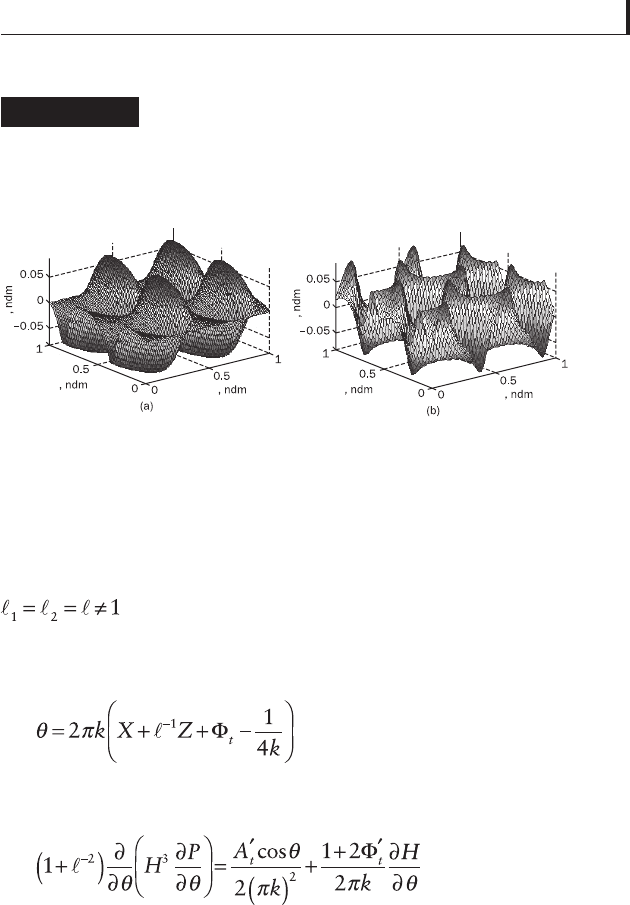
Typical pressure-coordinate and pressure-coordinate-time
distributions are presented in Fig. 3.12.
It is seen from Fig. 3.12(a), wave ratio 0.5, that the wave
number in the X-direction is 2 and in the Z-direction 4. The
waves along Z = 0 are partly truncated due to displacement
Figure 3.12
Typical pressure distribution in lubricating fi lm
between rough surfaces with wave number 2 at
wave ratio 0.5 with asperity height of lower
surface 0.5 and of upper surface 0.25 along:
(a) entire rough surface at time 1/(2k); (b) X, T
coordinates at Z = 1/(4k)
Z
X
T
X
P
P

93
Lubrication and roughness
of the moving-surface origin by a phase angle 1/(4k). The
time dependence, Fig. 3.12(b), has a more complicated form,
but it should be borne in mind that the plot refers to a single
Z-point and animated pictures are needed for studying the
overall situation. A feature common to the coordinate and
time pressure distributions is asymmetry about the X,Z –
and X,T – planes. This fact and the relevant theoretical
relations were verifi ed by numerical calculations and are
described in the next section.
Effect of asperity height and intra-surface wave
ratio on hydrodynamic pressure
The effect of sinusoidal roughness on the pressure distribution
and normal load support was studied with data presented in
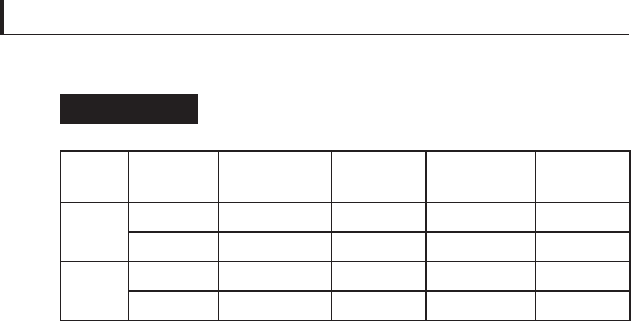
Table 3.1. The rather large reference values of the viscosity
were adopted for a better hydrodynamic effect on the resulting
pressure data.
Parameter Notation Reference value Dimensionality
Sliding velocity u 8.9 m/s
Fluid viscosity
μ
3, 0.3 Pa
.
s
Clearance Δ 6
μ
m
Surface sizes L
x
, L
z
10 mm
Asperity height
of lower surface
R
a
1
1.5 . . . 6
μ
m
Asperity height
of upper surface
R
a
2
3
μ
m
Relative gap
H
0
= Δ + 2R
a
2
12
μ
m
Inner-surface
wave ratio
1, 2 dimensionless
Number of profi le
waves
k 100 . . . 35,000 units
Reference values applied in calculations
Table 3.1
94
Tribology for Engineers
As it is obviously impossible to study all instantaneous
pressures, the maximum value is usually taken for this
purpose. It has a strong infl uence on normal fi lm support
and on such an undesirable effect as cavitation. The latter
occurs when the hydrodynamic pressure reaches, or drops
below, its normal level and is characterized by decomposition
of lube into fractions, bubbling and formation of cavities on
the surface. The adopted reference values of the dynamic
viscosity were those of motor oils SAE 30–50 at 100 °C. The
dimensionles cavitation pressure threshold P
cav
equals
0.91·10
–5
at viscosity 3 Pa·s, and 9.1·10
–3
at 0.3 Pa·s.
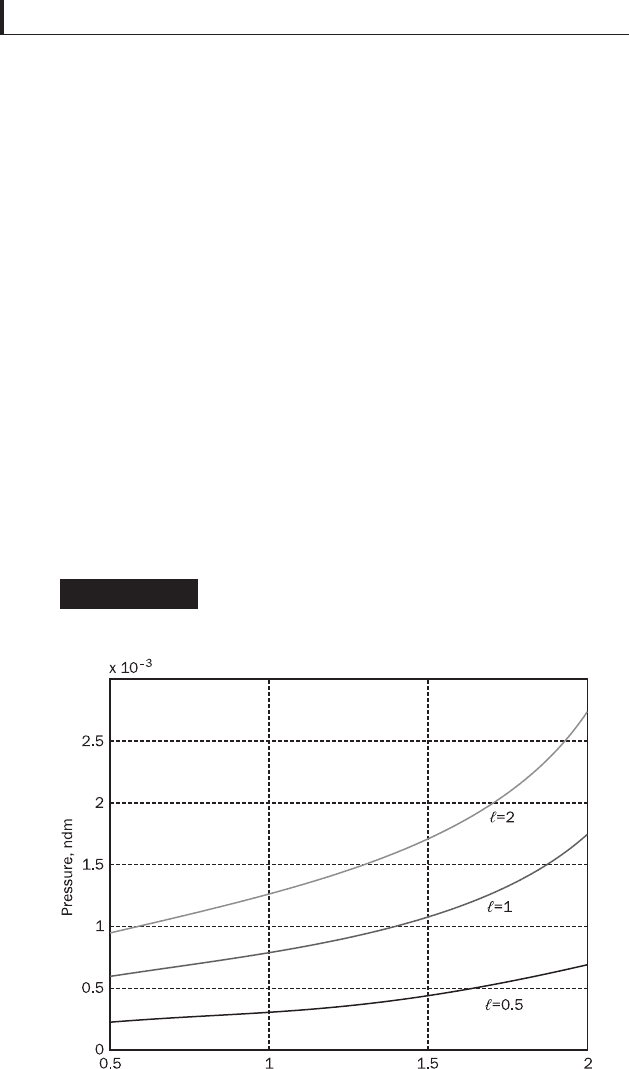
Figure 3.13 shows the dependence of the maximal positive
hydrodynamic pressure for the asperity height ratio range
0.5–2 and for wave ratios 0.5, 1 and 2. Evidently, the pressure
is lower for lower wave ratios; besides, as could be expected,
Figure 3.13
Maximal pressures versus asperity height ratio
at different wave ratios with reference wave
number k
x
= 100
Asperity height ratio A
1
/A
2
, ndm
95
Lubrication and roughness
if the wave number in the X-direction (that of motion) is
higher than in the Z-direction (wave ratio 0.5), the maximal
pressure is double and more than in the opposite case
(wave ratio 2). As can be seen, the maximal support effect is
reached at larger values of the roughness height ratio A
1
/A
2
,
that is, the roughness of the moving surface affects
the hydrodynamic pressure more strongly than that of the
stationary one. Also, maximal pressures are reached at the
largest values of wave ratio , which means the wave
number in the X-direction outweighs that in the Z-direction.
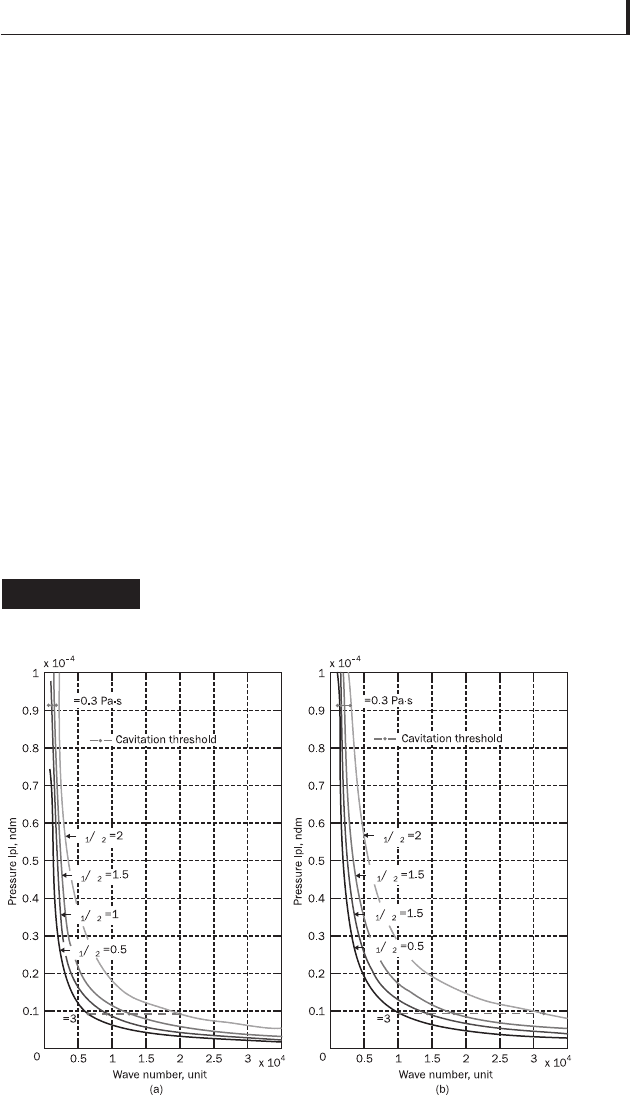
Figure 3.14 shows the dependence of the maximal pressure
on the wave number for asperity height ratio range 0.5–2
at = 1 and = 2. Numerical results are also presented in
Table 3.2. As can be expected, higher pressures are reached at
smaller wave numbers. Thus it is preferable to produce surfaces
Figure 3.14
Maximal and cavitation pressures at different
numbers of waves and roughness values at
wave ratio 1 (a) and 2 (b)
A
m
m
m
m
A
A
AA
A
A
A
AA
AA
AA
AA
96
Tribology for Engineers
with fewer waves, but unfortunately unrealistic, as in practice
the surfaces of machine parts have thousands of roughness
waves.
The horizontal dotted lines in Fig. 3.14(a) and (b) represent
the two cavitation thresholds for two viscosity values – 3 (lower
threshold line) and 0.3 Pa·s. Below these lines the positive
hydrodynamic effect did not exist at the respective viscosities.
As can be seen, for wave ratio 1 (Fig. 3.14(a)) and viscosity
3 Pa·s the hydrodynamic effect vanished at wave numbers
above 6.5·10
3
at the lower roughness height ratio 0.5 and
about 19.5·10
3
at the higher ratio 2. For the lower viscosity
0.3 Pa·s the effect vanished at wave numbers about ten times
lower – from above 0.63·10
3
and 1.8·10
3
for the two ratios
respectively. For the unequal wave numbers, in the higher
case (Fig. 14(b) – wave ratio 2), the cavitation threshold was
reached at higher wave numbers, which means that the surface
apparently can contain more waves and survive under this
maximal pressure. The hydrodynamic effect vanished at
higher wave numbers; specifi cally, for viscosity 3 Pa·s it was
above 10.3·10
3
at asperity height ratio 0.5 and above 31.0·10
3
at asperity height ratio 2; for viscosity 0.3 Pa·s, cavitation set
in when the surface had about 1.1·10
3
waves at ratio 0.5 and
3.0·10
3
waves at asperity ratio 2.
Thus we can say in general that a positive effect takes place
only at wave numbers below 600 at all studied roughness
Cavitation wave number at studied values of
viscosity, wave and asperity height ratios
Table 3.2
Wave
ratio
Viscosity,
Pa·s
A
1
/A
2
= 0.5 A
1
/A
2
= 1 A
1
/A
2
= 1.5 A
1
/A
2
= 2
1
0.3 628 919 1302 1824
3.0 6545 8571 11754 19534
2
0.3 1143 1496 1847 3012
3.0 10297 13824 19020 30964

97
Lubrication and roughness
ratios. As in most real oils the viscosities are ten and more
times lower than the discussed values, and the cavitation
threshold can set in with two-digit or even one-digit numbers
waves. This explains why real mechanical parts do not
exhibit any positive hydrodynamic effect. Most such parts
are suffi ciently large to accommodate tens and hundreds of
thousands of wavelengths, so that a sinusoidal profi le is
irrelevant to them, but for small ones, such as micro- or
nano-scale lubricated surfaces, it can be very effective.
The discussed results were computed by a special program
written in Matlab. The algorithm and other information
about this program are available in Burstein (2009).
Summarizing this analysis, the main results are:
■
The maximal support effect is reached at larger values of
the roughness height ratio A
1
/A
2
, indicating that the
roughness of the stationary surface has a stronger effect
on the hydrodynamic pressure than that of the moving
one; this is of practical interest in designing opposite
surfaces with different roughnesses.
■
In the case of equal wave numbers in the X- and Z-directions,
for the higher viscosity 3 Pa·s the hydrodynamic effect
vanishes at a wave number above 6.5·10
3
at roughness
height ratio 0.5 and at about 19.5·10
3
at the higher
ratio 2. For the lower viscosity 0.3 Pa·s the hydrodynamic
effect vanishes at wave numbers about ten times lower –
from above 0.63·10
3
to 1.8·10
3
for asperity ratios 0.5 and 2
respectively.
■
In the case of unequal wave numbers, the hydrodynamic
effect vanishes for 3Pa·s at above 10.3·10
3
, roughness ratio
0.5, and at above 31.0·10
3
, roughness height ratio 2. For 0.3
Pa·s it vanishes when the surface has about 1.1·10
3
waves at
roughness height ratio 0.5 and 3.0·10
3
waves at roughness
height ratio 2.

98
Tribology for Engineers
■
In general, the maximal pressures are reached at larger
wave ratios, indicating that the wave number in the
X-direction outweighs that in the Z-direction.
The results in this section were obtained for the case of equal
wave numbers on the surfaces. The ‘unequal’ case was, until
quite recently, considered intractable because of the serious
diffi culties in integrating Reynolds’ equation (see, e.g., Letallear
et al., 2002). This obstacle was removed on introduction of the
triangular roughness pattern.
3.6.3 Hydrodynamic lubrication for
triangular roughness
Take for analysis a control cell – an element on the surfaces
such that integer numbers of waves are located along the
coordinate axes. By this means the behaviour of the whole
surface can be studied on the basis of a small part. Thus a
surface of, say, 10 sq. cm. with asperity width 50 micron
(roughness standard numbers N8–N12) has approximately
630 asperities in each of the X- and Z-directions, but one can
make do with a cell with say 5 asperities only, and apply the
results to all 126 such cells that can be accommodated on the
surface; in parallel, the opposite surface may have a different
asperity width, but again such that the wave numbers in both
directions are integers; in this example, for say 3 waves, the
asperity width must be about 83micron. It is also clear that in
reality the number of asperities in the control cell should be
varied over a relatively narrow range, with the wave numbers
on the two surfaces varying by a factor of 2 or 3.
The X-plane of the profi le geometry and the 3D view for a
control cell with unequal wave numbers and unequal asperity
heights, R
a
, are shown in Fig. 3.15 for triangular waves, as
an example.
