Daniel W.W. Biostatistics: A Foundation for Analysis in the Health Sciences

Подождите немного. Документ загружается.

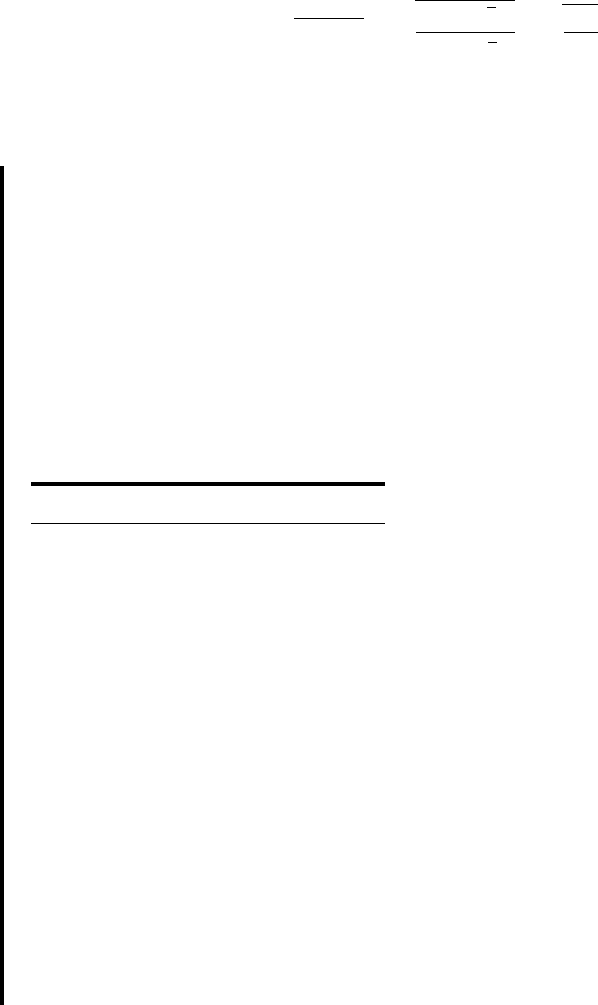
The multiple correlation coefﬁcient is the square root of the coefﬁcient of multi-

ple determination and, consequently, the sample value may be computed by taking the

square root of Equation 10.4.2. That is,

(10.6.2)

To illustrate the concepts and techniques of multiple correlation analysis, let us

consider an example.

EXAMPLE 10.6.1

Wang et al. (A-4), using cadaveric human femurs from subjects ages 16 to 19 years, inves-

tigated toughness properties of the bone and measures of the collagen network within

the bone. Two variables measuring the collagen network are porosity (P, expressed as

a percent) and a measure of collagen network tensile strength The measure of

toughness (W, Newtons), is the force required for bone fracture. The 29 cadaveric

femurs used in the study were free from bone-related pathologies. We wish to analyze

the nature and strength of the relationship among the three variables. The measure-

ments are shown in the following table.

1S2.

y.12 . . . k

= 2R

y.12 . . . k

g1y

- y2

g1y

- y2

SSR

SST

10.6 THE MULTIPLE CORRELATION MODEL 507

TABLE 10.6.1 Bone Toughness and

Collagen Network Properties for

29 Femurs

WPS

193.6 6.24 30.1

137.5 8.03 22.2

145.4 11.62 25.7

117.0 7.68 28.9

105.4 10.72 27.3

99.9 9.28 33.4

74.0 6.23 26.4

74.4 8.67 17.2

112.8 6.91 15.9

125.4 7.51 12.2

126.5 10.01 30.0

115.9 8.70 24.0

98.8 5.87 22.6

94.3 7.96 18.2

99.9 12.27 11.5

83.3 7.33 23.9

72.8 11.17 11.2

83.5 6.03 15.6

59.0 7.90 10.6

(

Continued

)

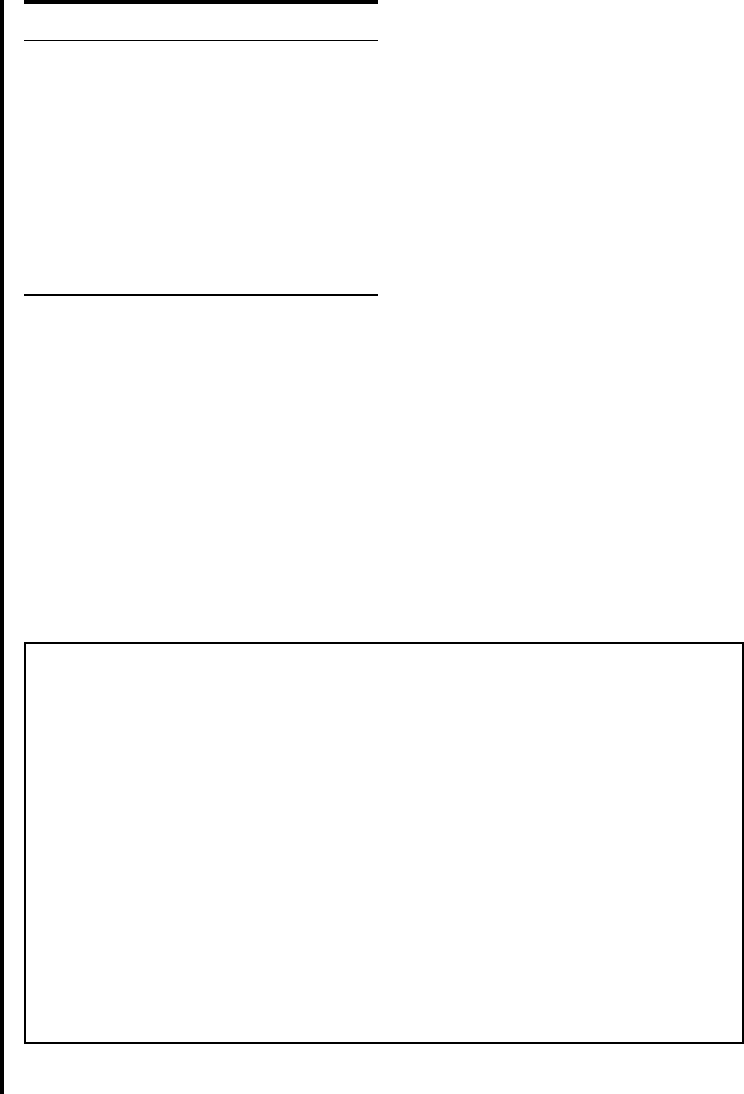
Solution: We use MINITAB to perform the analysis of our data. Readers interested

in the derivation of the underlying formulas and the arithmetic procedures

involved may consult the texts listed at the end of this chapter and Chap-

ter 9, as well as previous editions of this text. If a least-squares prediction

equation and multiple correlation coefﬁcient are desired as part of the analy-

sis, we may obtain them by using the previously described MINITAB mul-

tiple regression procedure. When we do this with the sample values of

and stored in Columns 1 through 3, respectively, we obtain the output

shown in Figure 10.6.1.

The least-squares equation, then, is

= 35.61 + 1.451x

+ 2.3960x

Y, X

508

CHAPTER 10 MULTIPLE REGRESSION AND CORRELATION

The regression equation is

Y = 35.6 + 1.45 X1 + 2.40 X2

Predictor Coef SE Coef T P

Constant 35.61 29.13 1.22 0.232

X1 1.451 2.763 0.53 0.604

X2 2.3960 0.7301 3.28 0.003

S = 27.42 R-Sq = 29.4% R-Sq(adj) = 24.0%

Analysis of Variance

Source DF SS MS F P

Regression 2 8151.1 4075.6 5.42 0.011

Residual Error 26 19553.5 752.1

Total 28 27704.6

FIGURE 10.6.1 Output from MINITAB multiple regression procedure for the data in

Table 10.6.1.

WPS

87.2 8.27 24.7

84.4 11.05 25.6

78.1 7.61 18.4

51.9 6.21 13.5

57.1 7.24 12.2

54.7 8.11 14.8

78.6 10.05 8.9

53.7 8.79 14.9

96.0 10.40 10.3

89.0 11.72 15.4

Source: Xiaodu Wang, Ph.D.

Used with permission.

This equation may be used for estimation and prediction purposes and may

be evaluated by the methods discussed in Section 10.4.

As we see in Figure 10.6.1, the multiple regression output also gives us

the coefﬁcient of multiple determination, which, in our present example, is

The multiple correlation coefﬁcient, therefore, is

Interpretation of

We interpret as a measure of the correlation among the variables force required to

fracture, porosity, and collagen network strength in the sample of 29 femur bones from

subjects ages 16 to 19. If our data constitute a random sample from the population of such

persons, we may use as an estimate of the true population multiple correlation

coefﬁcient. We may also interpret as the simple correlation coefﬁcient between

and

the observed and calculated values, respectively, of the “dependent” variable. Per-

fect correspondence between the observed and calculated values of Y will result in a cor-

relation coefﬁcient of 1, while a complete lack of a linear relationship between observed

and calculated values yields a correlation coefﬁcient of 0. The multiple correlation coef-

ﬁcient is always given a positive sign.

We may test the null hypothesis that by computing

(10.6.3)

The numerical value obtained from Equation 10.6.3 is compared with the tabulated value

of F with k and degrees of freedom. The reader will recall that this is iden-

tical to the test of described in Section 10.4.

For our present example let us test the null hypothesis that against the

alternative that . We compute

Since 5.41 is greater than so that we may reject the null hypothesis at the

.025 level of signiﬁcance and conclude that the force required for fracture is correlated

with porosity and the measure of collagen network strength in the sampled population.

The computed value of F for testing that the population multiple correlation

coefﬁcient is equal to zero is given in the analysis of variance table in Figure 10.6.1 and

is 5.42. The two computed values of F differ as a result of differences in rounding in

the intermediate calculations. ■

Partial Correlation The researcher may wish to have a measure of the strength

of the linear relationship between two variables when the effect of the remaining variables

has been removed. Such a measure is provided by the partial correlation coefﬁcient. For

4.27, p 6 .025,

F =

.294

1 - .294

29 - 2 - 1

= 5.41

y.12

Z 0

y.12

= 0

: b

= b

...

= b

= 0

n - k - 1

F =

y.12 . . . k

1 - R

y.12 . . . k

n - k - 1

y.12 . . . k

= 0

y.12

= 1.294 = .542

y.12

= .294

10.6 THE MULTIPLE CORRELATION MODEL 509

example, the partial sample correlation coefﬁcient is a measure of the correlation

between Y and after controlling for the effect of

The partial correlation coefﬁcients may be computed from the simple correlation

coefﬁcients. The simple correlation coefﬁcients measure the correlation between two vari-

ables when no effort has been made to control other variables. In other words, they are

the coefﬁcients for any pair of variables that would be obtained by the methods of sim-

ple correlation discussed in Chapter 9.

Suppose we have three variables, and The sample partial correlation coef-

ﬁcient measuring the correlation between Y and after controlling for for example,

is written In the subscript, the symbol to the right of the decimal point indicates

the variable whose effect is being controlled, while the two symbols to the left of the

decimal point indicate which variables are being correlated. For the three-variable case,

there are two other sample partial correlation coefﬁcients that we may compute. They

are and

The Coefﬁcient of Partial Determination The square of the partial

correlation coefﬁcient is called the coefﬁcient of partial determination. It provides use-

ful information about the interrelationships among variables. Consider for example.

Its square, tells us what proportion of the remaining variability in Y is explained by

after has explained as much of the total variability in Y as it can.

Calculating the Partial Correlation Coefﬁcients For three vari-

ables the following simple correlation coefﬁcients may be calculated:

the simple correlation between Y and

the simple correlation between and

The MINITAB correlation procedure may be used to compute these simple corre-

lation coefﬁcients as shown in Figure 10.6.2. As noted earlier, the sample observations

are stored in Columns 1 through 3. From the output in Figure 10.6.2 we see that

and

The sample partial correlation coefﬁcients that may be computed from the simple

correlation coefﬁcients in the three-variable case are:

1. The partial correlation between Y and after controlling for the effect of

(10.6.4)

2. The partial correlation between Y and after controlling for the effect of

(10.6.5)

3. The partial correlation between and after controlling for the effect of Y:

(10.6.6)r

12.y

= 1r

- r

2>211 - r

211 - r

y2.1

= 1r

- r

2>211 - r

211 - r

y1.2

= 1r

- r

2>211 - r

211 - r

= .535.r

=-.08, r

= .043,

y1.2

12.y

y2.1

y1.2

.Y, X

y.12

510 CHAPTER 10 MULTIPLE REGRESSION AND CORRELATION

EXAMPLE 10.6.2

To illustrate the calculation of sample partial correlation coefﬁcients, let us refer to Exam-

ple 10.6.1, and calculate the partial correlation coefﬁcients among the variables force to

fracture , porosity and collagen network strength

Solution: Instead of computing the partial correlation coefﬁcients from the simple cor-

relation coefﬁcients by Equations 10.6.4 through 10.6.6, we use MINITAB

to obtain them.

The MINITAB procedure for computing partial correlation coefﬁcients

is based on the fact that a given partial correlation coefﬁcient is itself the sim-

ple correlation between two sets of residuals. A set of residuals is obtained

as follows. Suppose we have measurements on two variables, X (independ-

ent) and Y (dependent). We obtain the least-squares prediction equation,

For each value of X we compute a residual, which is equal to

the difference between the observed value of Y and the predicted

value of Y associated with the X.

Now, suppose we have three variables, and Y. We want to com-

pute the partial correlation coefﬁcient between and Y while holding

constant. We regress on and compute the residuals, which we may

call residual set A. We regress Y on and compute the residuals, which

we may call residual set B. The simple correlation coefﬁcient measuring the

strength of the relationship between residual set A and residual set B is

the partial correlation coefﬁcient between and Y after controlling for the

effect of X

, X

- y

= b

+ b

2.1X

2,1Y2

10.6 THE MULTIPLE CORRELATION MODEL 511

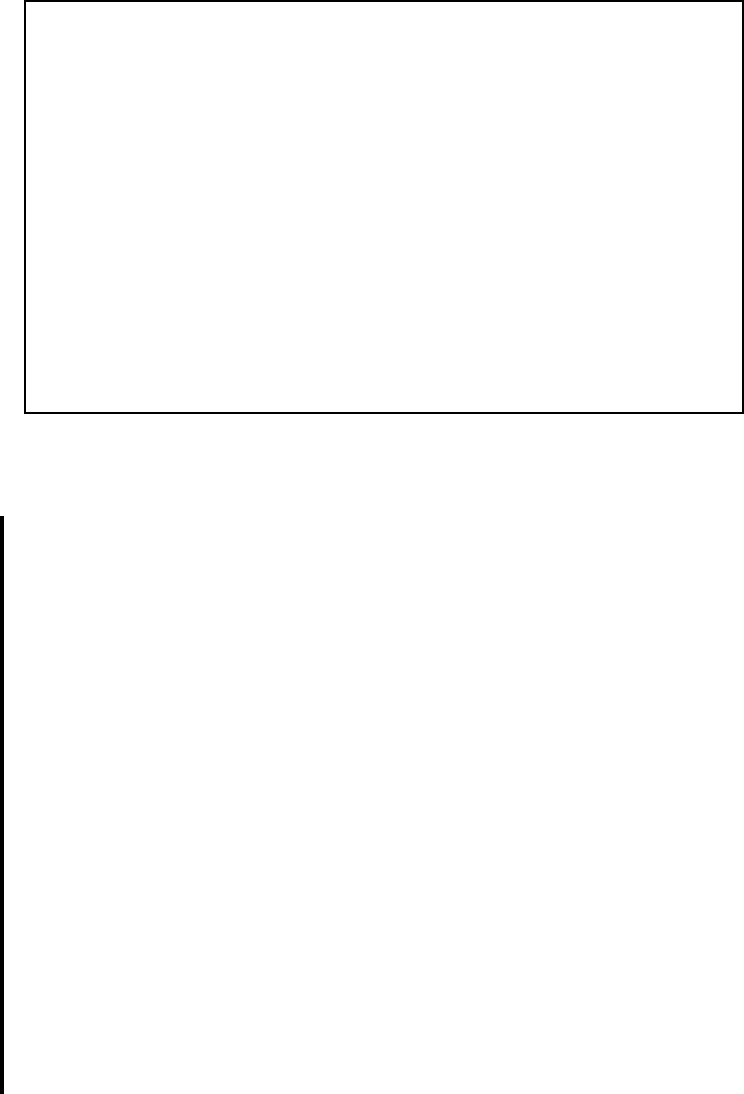
Dialog box: Session Command:

Stat ➤ Basic Statistics ➤ Correlation MTB> CORRELATION C1-C3

Type C1-C3 in Variables. Click OK.

Output:

YX1

X1 0.043

0.823

X2 0.535 -0.080

0.003 0.679

Cell Contents: Pearson correlation

P-Value

FIGURE 10.6.2 MINITAB procedure for calculating the simple correlation coefﬁcients for

the data in Table 10.6.1.

When using MINITAB we store each set of residuals in a different

column for future use in calculating the simple correlation coefficients

between them.

We use session commands rather than a dialog box to calculate the

partial correlation coefﬁcients when we use MINITAB. With the observa-

tions on and Y stored in Columns 1 through 3, respectively, the pro-

cedure for the data of Table 10.6.1 is shown in Figure 10.6.3. The output

shows that and

Partial correlations can be calculated directly using SPSS software as

seen in Figure 10.6.5. This software displays, in a succinct table, both the

partial correlation coefﬁcient and the p value associated with each partial

correlation.

■

Testing Hypotheses About Partial Correlation Coefficients

We may test the null hypothesis that any one of the population partial correlation coef-

ﬁcients is 0 by means of the t test. For example, to test we compute

(10.6.7)

which is distributed as Student’s t with degrees of freedom.

Let us illustrate the procedure for our current example by testing

against the alternative, The computed t is

Since the computed t of .523 is smaller than the tabulated t of 2.0555 for 26 degrees

of freedom and (two-sided test), we fail to reject at the .05 level of sig-

nificance and conclude that there may be no correlation between force required for

fracture and porosity after controlling for the effect of collagen network strength. Sig-

nificance tests for the other two partial correlation coefficients will be left as an exer-

cise for the reader. Note that p values for these tests are calculated by MINITAB as

shown in Figure 10.6.3.

The SPSS statistical software package for the PC provides a convenient procedure

for obtaining partial correlation coefﬁcients. To use this feature choose “Analyze” from

the menu bar, then “Correlate,” and, ﬁnally, “Partial.” Following this sequence of choices

the Partial Correlations dialog box appears on the screen. In the box labeled “Variables:,”

enter the names of the variables for which partial correlations are desired. In the box

labeled “Controlling for:” enter the names of the variable(s) for which you wish to con-

trol. Select either a two-tailed or one-tailed level of signiﬁcance. Unless the option is

deselected, actual signiﬁcance levels will be displayed. For Example 10.6.2, Figure 10.6.4

shows the SPSS computed partial correlation coefﬁcients between the other two vari-

ables when controlling, successively, for (porosity), (collagen network strength),

and Y (force required for fracture).

a = .05

t = .102

29 - 2 - 1

1 - 1.1022

= .523

: r

y1.2

Z 0.

: r

y1.2

= 0

n - k - 1

t = r

y1.2 . . . k

n - k - 1

1 - r

y1.2 . . . k

: r

y1.2 . . . k

= 0,

y2.1

= .541.r

y1.2

= .102, r

12.y

=-.122,

, X

512

CHAPTER 10 MULTIPLE REGRESSION AND CORRELATION

10.6 THE MULTIPLE CORRELATION MODEL 513

MTB > regress C1 1 C2;

SUBC> residuals C4.

MTB > regress C3 1 C2;

SUBC> residuals C5.

MTB > regress C1 1 C3;

SUBC> residuals C6.

MTB > regress C2 1 C3;

SUBC> residuals C7.

MTB > regress C2 1 C1;

SUBC> residuals C8.

MTB > regress C3 1 C1;

SUBC> residuals C9.

MTB > corr C4 C5

Correlations: C4, C5

Pearson correlation of C4 and C5 = 0.102

P-Value = 0.597

MTB > corr C6 C7

Correlations: C6, C7

Pearson correlation of C6 and C7 = -0.122

P-Value = 0.527

MTB > corr C8 C9

Correlations: C8, C9

Pearson correlation of C8 and C9 = 0.541

P-Value = 0.002

FIGURE 10.6.3 MINITAB procedure for computing partial correlation coefﬁcients from the

data of Table 10.6.1.

514 CHAPTER 10 MULTIPLE REGRESSION AND CORRELATION

Controlling for: X1

X2 Y

X2 1.0000 .5412

( 0) ( 26)

P= . P= .003

Y .5412 1.0000

( 26) ( 0)

P= .003 P= .

Controlling for: X2

YX1

Y 1.0000 .1024

( 0) ( 26)

P= . P= .604

X1 .1024 1.0000

( 26) ( 0)

P= .604 P= .

Controlling for: Y

X1 X2

X1 1.0000 -.1225

( 0) ( 26)

P= . P= .535

X2 -.1225 1.0000

( 26) ( 0)

P= .535 P= .

(Coefﬁcient / (D.F.) / 2-tailed Signiﬁcance)

“.” is printed if a coefﬁcient cannot be computed

FIGURE 10.6.4 Partial coefﬁcients obtained with SPSS for Windows, Example 10.6.2.

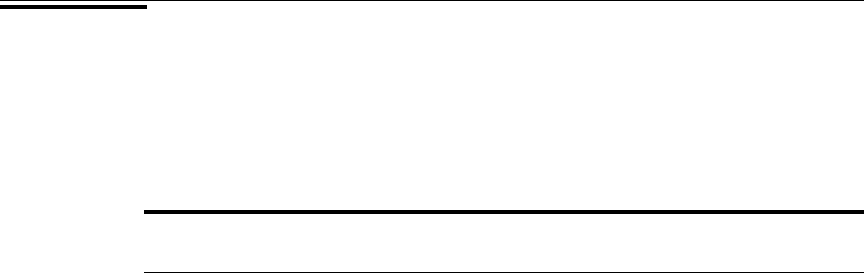
10.6 THE MULTIPLE CORRELATION MODEL 515

FIGURE 10.6.5 Partial correlation coefﬁcients for the data in Example 10.6.1. (

)

1. 2

(

)

12.

, and (

)

2.1

(a)

Correlations

Porosity Tensile

Control Variables (X1) Strength (X2)

Force to Fracture (Y) Porocity (X1) Correlation 1.000 .122

Signiﬁcance (2-tailed) . .535

df 0 26

Tensile Strength (X2) Correlation .122 1.000

Signiﬁcance (2-tailed) .535 .

df 26 0

(b)

Correlations

Tensile Force to

Control Variables Strength (X2) Fracture (Y)

Porosity (X1) Tensile Strength (X2) Correlation 1.000 .541

Signiﬁcance (2-tailed) . .003

df 0 26

Force to Fracture (Y) Correlation .541 1.000

Signiﬁcance (2-tailed) .003 .

df 26 0

(c)

Although our illustration of correlation analysis is limited to the three-variable case,

the concepts and techniques extend logically to the case of four or more variables. The

number and complexity of the calculations increase rapidly as the number of variables

increases.

Correlations

Force to

Control Variables Fracture (Y) Porosity (X1)

Tensile Strength (X2) Force to Fracture (Y) Correlation 1.000 .102

Signiﬁcance (2-tailed) . .604

df 0 26

Porosity (X1) Correlation .102 1.000

Signiﬁcance (2-tailed) .604 .

df 26 0

EXERCISES

10.6.1 The objective of a study by Anton et al. (A-5) was to investigate the correlation structure of

multiple measures of HIV burden in blood and tissue samples. They measured HIV burden four

ways. Two measurements were derived from blood samples, and two measurements were made on

rectal tissue. The two blood measures were based on HIV DNA assays and a second co-culture

assay that was a modiﬁcation of the ﬁrst measure. The third and fourth measurements were quan-

titations of HIV-1 DNA and RNA from rectal biopsy tissue. The table below gives data on HIV

levels from these measurements for 34 subjects.

HIV DNA Blood HIV Co-Culture HIV DNA Rectal HIV RNA Rectal

(Y) Blood (X

) Tissue (X

)

115 .38 899 56

86 1.65 167 158

19 .16 73 152

6 .08 146 35

23 .02 82 60

147 1.98 2483 1993

27 .15 404 30

140 .25 2438 72

345 .55 780 12

92 .22 517 5

85 .09 346 5

24 .17 82 12

109 .41 1285 5

5 .02 380 5

95 .84 628 32

46 .02 451 5

25 .64 159 5

187 .20 1335 121

5 .04 30 5

47 .02 13 30

118 .24 5 5

112 .72 625 83

79 .45 719 70

52 .23 309 167

52 .06 27 29

7 .37 199 5

13 .13 510 42

80 .24 271 15

86 .96 273 45

26 .29 534 71

53 .25 473 264

185 .28 2932 108

30 .19 658 33

9 .03 103 5

76 .21 2339 5

516 CHAPTER 10 MULTIPLE REGRESSION AND CORRELATION

(Continued)