Crank J. Free and Moving Boundary Problems

302

Analytical solution

of

seepage

problems

Izl

< 1

is

expressed as

y=

C

1

F(a,

b;

c;

z)+Cz

z

1-

C

P(a-c+

1,

b-c+

1;

2-c;

z),

(7.33c)

where it

is

assumed that

the

parameter c

is

not

an integer.

Fundamental solutions in the neighbourhood of

z = 1 (case (ii) above)

are represented by

Y3

=

P(

a,

b; a + b +

1-

c;

1-

z)

and

Y4

=(1-

z)c-a-bp(c-b,

c-

a;

c-a-b+

1;

1-

z),

(7.34)

i.e. the exponents are

p = 0 and c -

a-b.

For case (iii), in

the

neighbour-

hood of

z =

00

we have solutions

Y

s

= z-ap(a, a

-c

+ 1; 1

+a-b;

1/z),

Y

6

= z-bp(b,

b-c+

1; 1

+b-a;

liz),

(7.35)

and

the

exponents for

liz

are

(3

=

a,

b.

This information about

the

solutions of (7.31) are summarized by the Riemann P-equation

YSP{

~

l-c

b

a

1 }

o

z.

c-a-b

(7.36)

00

The

singular points of

the

equation are placed in

the

first row and

the

exponents are directly beneath them; the independent variable

is

indi-

cated in

the

fourth column.

There

is

a standard transformation that leads

to

a Riemann function which has one vanishing exponent about each

finite singular point, namely

r

~

...

a,.

00

z}

~

(z

-

.,)"·(z

-

0,)-'

...

(z

-a,.)"

P a1

a2

... a,. a

(31

(32

...

f3n

(3

{

~

a2

a,.

00

+

xP

0

a+a1+··

. + a,.

(7.37)

(31-

a

1

(32-

a

2

(3n

- a,.

(3

+ a1 +

...

+ a,.

We

now have to relate these solutions of the hypergeometric series to

seepage problems.

In

particular we need to deduce

the

exponents p at the

singular points on the real axis in the g-plane from

the

boundary

conditions which are prescribed on

the

different segments of

the

real axis.

Polubarinova-Kochina (1962) observed that the commonly occurring flow

regions are confined by straight line boundaries and free surfaces. Further-

more,

the

boundary conditions are expressed by equating linear func-

tions of the coordinates of

the

boundaries

to

zero

or

linear functions of

Polubarinova-Kochina's solution for the simple dam

303

the coordinates and the velocity potential and stream functions,

q,

and

"'.

Along each boundary, therefore, the two conditions to

be

satisfied can be

written

where

the

coefficients are constants. More conveniently we write

Im(kz+f{)=p,

Im(mz +

n{)

==

q,

(7.38)

where

k,

f,

m,

n are complex,

p,

q are real numbers.

"When

the boundaries

of the flow region are mapped on to the real axis of the

~-plane,

the

corresponding values of

~

are real.

On

introducing the functions

F==d{/d~,

Z=dz/d~,

we obtain by differentiating in (7.38)

Im(kZ

+ fF) = 0,

lm(mZ

+ nF) = 0. (7.39)

We now seek to find two functions

F, Z of

the

complex variable

~

which satisfy conditions of type (7.39) on segments of

the

real

~-axis.

Figure 7.12e shows two neighbouring segments

MIM2

and

M2M3

with

the

two conditions displayed for each segment.

In

order to examine the

behaviour of

F and Z when a singular point, say M

2

,

is

contoured we use

the

standard result that for a function

f(z)

which

is

analytic in a region of

the

upper half-plane adjacent

to

the real axis and continuous

up

to a

segment along which

1m

{f(z)}

= 0, the analytic continuation in the lower

half-plane is such that in conjugate points

the

function takes conjugate

values.

To

apply this result to the linear combinations of F and Z

associated with MIM2 (Fig. 7. 12e), a cut

is

made along segment

M2M3

and

the points

on

the

upper and lower sides of the cut are denoted by M'

and

M".

The values of F and Z at point

M'

after contouring the singular

point

M2

are written as

F*

and

Z*.

Thus we have

klZ*+flF*=klZ+llF.

mlZ*+nlF*=fiilZ+;itF.

(7.40)

With regard to

the

segment

M2M3

the conditions can

be

rewritten

(7.41)

After some manipulation to eliminate

F and Z from (7.40) and (7.41) we

can write

Z*,

F*

in

the

form

(7.42)

where

(310

(32,

'Ylo

'Y2

are constants involving the

klo

flo etc. in a

complicated way, i.e.

F and Z undergo a linear transformation when

contouring a singular point. We can interpret (7.42) to mean that

F and Z

304

Analytical solution

of

seepage problems

can

be

expressed as linear combinations

of

two functions U

and

V which

undergo

the

transformations

U* =

At

U,

V*

=

A2

V, (7.43a)

where

A10

A2

are

the

roots

of

the

characteristic equation associated with

(7.42), i.e.

I

(3t

- A

(32

I =

o.

'Yt

'Y2-

A

(7.44)

In

the

case

of

a double

root

of (7.44)

the

transformations become

U*=At

U

,

V*=(3tU+A2V.

(7.43b)

After

considerable manipulation

the

characteristic equation can

be

writ-

ten

in

terms

of

kt,

k10

.f1o

ft, k2'

k2'

etc.

and

becomes

ktA .ftA

kt

f

t

mt

A

nt

A

mt

nt

=0.

(7.45)

k2

.f2

k2

f2

m2

n2

m2

n2

H we now consider

the

behaviour

of

the

functions

(e

-

eo)'"l

and

(e

-

eoyx2

on

contouring

the

singular

point

~

=

eo,

corresponding

to

M2

in

Fig. 7.12e, through

an

angle

2'7T

and

choose

we find

In

At

at

=

2'7Ti

'

(7.46)

Thus

the

quotients

UI(~

-

eoYx"

V/(e

-

~0)"2

remain single-valued func-

tions and, having in mind

that

a10

a2 are only determined within

an

integer we can develop

Laurent

series for a regular singularity with

no

negative powers

and

write

00

U=(e-eo)Pl

L

tlJc(~_~o)k,

(7.47a)

k=O

00

V=(~-~0)P2

L

~(~-eo)k+aUln(~-~o),

(7.47b)

k=O

in

which ao, b

o

are

non-zero.

When

at-a2

is not zero

nor

an

integer,

then

we take a =

o.

Polubarinova-Kochina (1962) derived (7.45) directly

for

the

particular case

of

at

and

a2 real.

We

now seek

to

establish

the

exponents

about

singular points which

Polubarinova-Kochina's solution for

the

simple dam

305

may occur at

the

intersections of neighbouring boundary segments in the

simple dam problem, for example.

We

consider the following combina-

tions.

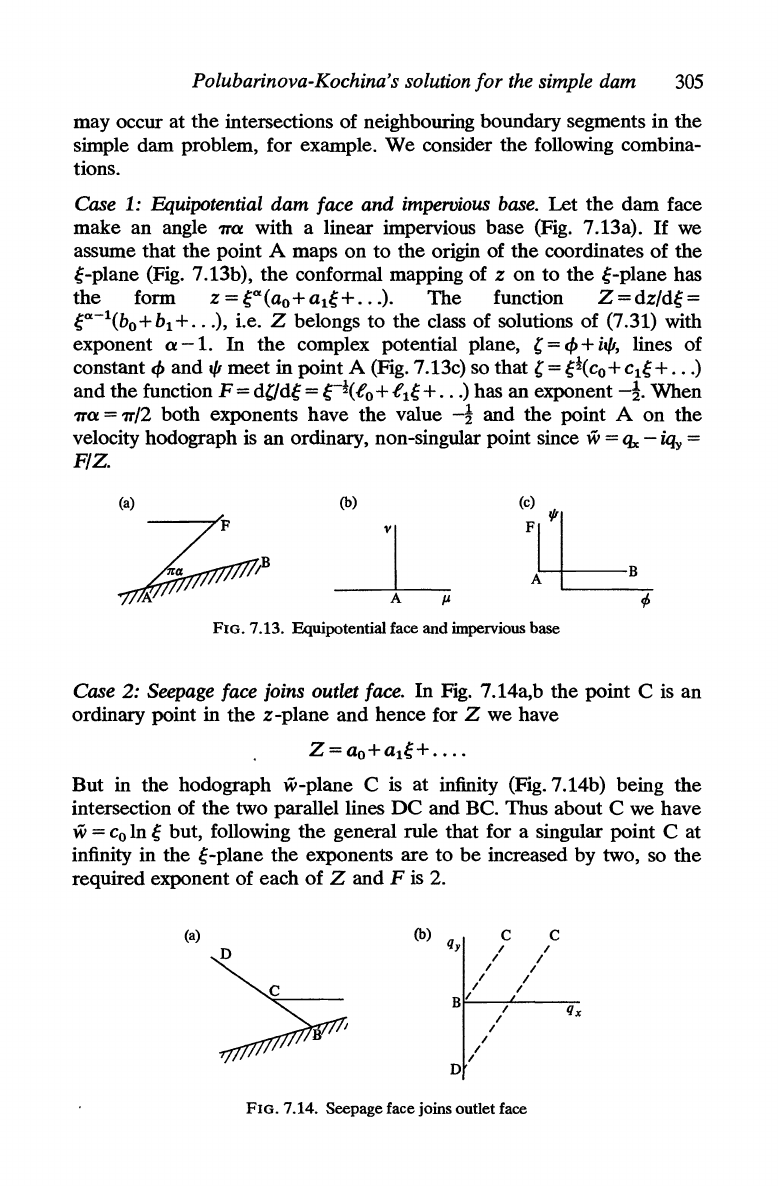
Case 1:

Equipotential dam face and impervious

base.

Let

the

dam face

make an angle

7Ta

with a linear impervious base (Fig. 7.13a).

If

we

assume that the point A maps on to the origin of the coordinates of the

~-plane

(Fig. 7.13b),

the

conformal mapping of z on to

the

~-plane

has

the

form z =

fX(aO+al~+"

.).

The

function

Z=dz/d~=

~a-l(bo+

b

l

+

...

), i.e. Z belongs to the class of solutions of (7.31) with

exponent

a

-1.

In

the

complex potential plane,

,=

q,

+

il/l,

lines of

constant

q,

and

1/1

meet in point A (Fig. 7.13c) so that

'=~!(CO+CI~+"')

and the function

F=

d"d~=

~-!(eO+el~+"')

has an exponent

-~.

When

7Ta

=

7T/2

both exponents have the value -! and the point A on the

velocity hodograph

is

an ordinary, non-singular point since w = llx -

iqy

=

F/Z.

(a)

(b)

~J

(~I

~I

A~

.p

FIG. 7.13. Equipotential face and impervious base

Case 2: Seepage face joins outlet face. In

Fig.

7.14a,b

the

point C

is

an

ordinary point in

the

z -plane and hence for Z we have

Z=aO+al~+"

..

But in the hodograph w-plane C is at infinity (Fig.7.14b) being the

intersection of

the

two parallel lines

DC

and BC. Thus about C we have

w =

Co

In

~

but, following the general rule that for a singular point C at

infinity in the

~-plane

the exponents are to

be

increased by two, so the

required exponent of each of Z and F

is

2.

(a)

(b)

qy

c c

~

/ /

/

I

/

I

/

a/

/

qx

/

D

FIG. 7.14. Seepage face joins outlet face

306 Analytical solution

of

seepage problems

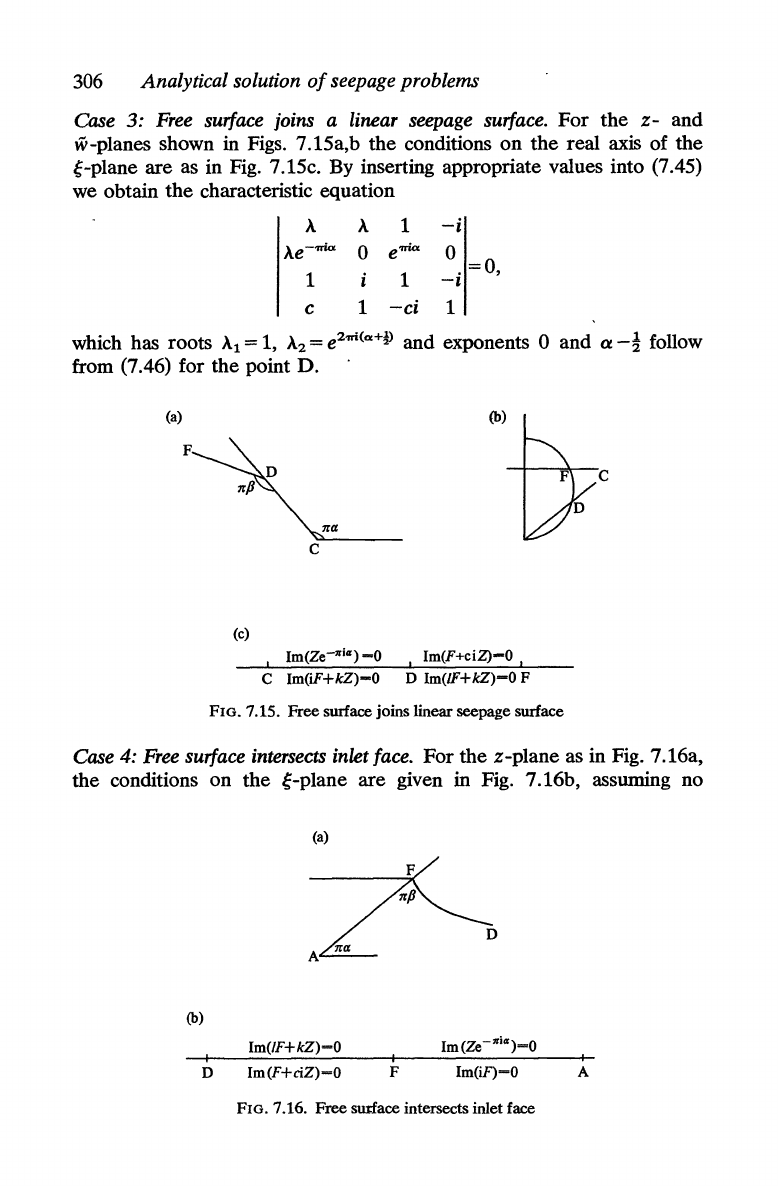
Case

3:

Free

surface joins a linear seepage surface.

For

the

z-

and

w-planes shown in Figs. 7.1Sa,b

the

conditions

on

the

real axis of the

~-plane

are

as in Fig. 7.1Sc. By inserting appropriate values into (7.45)

we obtain

the

characteristic equation

A A

1

-i

Ae-'lri<x

0

e'lri<x

0

.

=0,

1 1

-l

C 1

-ci

1

which has roots

A1

= 1,

A2

= e

2m

<a+p

and exponents ° and a

-~

follow

from (7.46) for the point

D.

(a)

(b)

c

n/%

c

(c)

I Im(F+ciZ)-O I

C Im(iF+kZ)-O D

Im(IF+kZ)-O

F

FIG. 7.15. Free surface joins linear seepage surface

Case 4:

Free

surface intersects inlet face.

For

the z-plane as in Fig. 7.16a,

the conditions

on

the

~-plane

are given in Fig. 7.16b, assuming no

(a)

D

(b)

Im(lF+kZ)-O

D

Im(F+ciZ)=O

F

Im(iF)-O

A

FIG. 7.16. Free surlace intersects inlet face

Polubarinova-Kochina's solution for the simple dam

307

infiltration.

The

characteristic equation is

A.

iA.

1

-i

0

A.

0

1

=0,

e-'Iri<>

0

e'lri<>

0

0 0

-i

with roots

A.

=

em,

e

2

'1ri<>

leading

to

exponents for the point F, -t,

-a

from

(7.46).

We are now able

to

find the exponents for

the

boundary intersections

in the simple dam problem of Fig. 7.12a and hence

to

find

the

appro-

priate functions

F and Z. Points

A(~

=

a)

and B(

~

= b) correspond to

Case 1 above with

a = t SO that these are both ordinary points.

At

point C

we have Case 2 and the exponents are 2,

2 for the point at

+00

in the

~-plane.

At

the separation point D, Case 3 applies with a =

t,

giving

exponents 0,

O.

Finally, by applying Case 4

to

point F with a

=t

we

obtain exponents

-t,

-to

Thus we see that

the

functions F and Z are different branches of the

function

Y represented by Riemann's P-equation

as

Y=p{~

-~ ~t

~!

o

-t

t t

By applying (7.37) to Y we find

y

~

(1-

g)-!(g - • )-I(g - b

)-!pU

=

YI.J{(1-~)(~-a)(~-b)}.

(7.48)

(7.49)

The

new function Y has only three singular points

at

~

= 0, 1,

00

and we

write

y=p{~

~

;

J.

o 0 t J

(7.50)

TIIUS

in (7.36) the values of the parameters corresponding to (7.50) are

a = b

=t,

c = 1 and hence

the

differential equation (7.31) takes the

particular form

d

2

y

dY

~(1-~)

de+(1-2~)

d~

-!Y=O.

(7.51)

If

we denote by U and V the linearly independent solutions of (7.51) we

308

Analytical solution

of

seepage problems

can write

AU+BV

F

.J{(1-~)(~-a)(~-b)r

Z=

CU+DV

.J{(1-~)(~-

a)(~-

b)}"

(7.52)

About

the

point

~

= 0

the

solution U

is

obtained

by

putting a = b

=!,

c = 1 in (7.33a) above

and

is

_

(1

1 ) _

(1)2

II.

3)2 2

(1.

3 .

5)2

3

U - F

2, 2,

1,

~

- 1 + 2

~

+

\2

. 4

~

+

2.

4 . 6

~

+

...

,

(7.53)

which is seen

to

differ from

the

complete elliptic integral

of

the

first kind

only by a multiplying constant.

Thus

we may write as

one

of

the

particular

solutions

of

(7.51)

J

"'12

dO

U =

K(~)

= 0

.J{1-

~

sin

2

Or

(7.54)

where

~

is

equated

to

the

square

of

the

modulus

k.

Since (7.51)

is

not

changed by substituting

1-

~

for

~

we can

take

as a

solution

V=K(1-~)=K',

(7.55)

which is regular about

~

= 1

but

has a logarithmic singularity about

~

=

o.

Thus U =

K(~)

and

V =

K(1-~)

are

linearly independent solutions about

~=o.

From

(7.35) we can find

that

U

I

=

(1/.J~)K(1/~),

VI =

(1/.J~)K(1-1/~)

(7.56)

are

linearly independent solutions

of

(7.51) convergent for

1~1>1

and

by

substituting

1-

~

for

~

we obtain

(7.57)

which converge outside a circle

of

unit radius, centre

~

= 1.

In

order

to relate these various functions

to

the

hodograph function

w =

<Ix

-

iqy

we

note

that

the

hodograph flow region

in

Fig. 7.12b is

mapped

on

to

the

upper-half

~-plane

in Fig. 7.12d by

the

relationship of

type

_

AK(~)+

BK(1-~)

w

CK(~)

+

DK(1-~)

.

Noting

that

on

the

segment

(0,1)

for

~

we have for

~

= 0, w =

ki

(AB = k),

Polubarinova-Kochina's solution for

the

simple

dam

309

for

~

= 1, W = 0, and for

~ =~,

W =

~k

+ (i/2)k,

we

find

_

K(l-~)

w = k

K(~)-

iK(l-~)

Through the relationships

we rewrite

w for the segment 1 <

~

<

00

as

and for

-00<~<0

- _ k

K(1-1/~)

w-

K(l/~)

(7.58)

(7.58a)

(7.58b)

which satisfy the necessary correspondence between the

w-

and

~-planes.

Now we return

to

expressions (7.52) for

F=a~/a~+i

a",/a~

and

Z=

ax/a~

+ i

ayla~

and insert the appropriate expressions

U1>

V1>

etc. from

(7.54-7) for each segment of

the

real axis of the

~-plane

(Fig. 7.12d)

having in mind the local boundary condition on the corresponding bound-

ary segment in the physical plane. Thus for 1

<

~

< a, which corresponds

to

the inlet face

AF

in Fig. 7.12a, where x = 0 and

~

is

constant,

we

write, using (7.56),

.

-AiK(l/~)

Z = ,

ayla~

=

.j{~(~

-1)(~

_

a)(~

-

b)}'

(7.59a)

.

-kAiK(l-1/~)

F=

,a"'la~

=

.j{~(~-1)(~-

a)(~

- b)} .

. (7.59b)

By integrating (7.59a) with respect to

~

over the range 1 <

~

< a we

obtain

f

a

K(l/~)

d~

H=A

1

J{~(~-l)(~-a)(~-b)}'

(7.60a)

and similarly (7.59b) gives the flow

Q,

where

f

a

K(l-l/~)d~

Q=kA

1

J{~(~-l)(~-a)(~-b)}'

(7.60b)

310

Analytical solution

of

seepage problems

By proceeding similarly for the segment b <

~

<

00,

which is

BC

in Fig.

7.12a,

we

find

1

00

K(1/~)

d~

h

==

A

JU(~-1)(~-a)(~-b)}'

and for a <

~

<

b,

i.e. the base

AB

in Fig. 7. 12a,

e-

Ib

K(l/~)

d~

-A

a

v'U(~-1)(~-a)(~-b)r

The length

of

the seepage surface,

CD

in Fig. 7.12a, follows by

considering the segment

-oo<~<O,

and is

1

0

K(1/{1-

~})

d~

ho==A

~

(1-~)~{(a-~)(b-~)r

The following changes of variable were introduced by Polubarinova-

Kochina (1962):

a==l/b, {3=1/a,

O~a~{3~1,

T=1/~,

C==2A1~(ab),

and

T =

a+({3-a)sin2'1'~

a

<~<b;

T={3+(1-f3)sin

2

'1',

1<g<a;

T==asin2'1',

The

expressions for

e,

H, and h become

(7.61)

(7.62)

(7.63)

By putting

1/(1-~)

==

cos

2

I{I,

a

1

=

1-

a, and

{31

==

1-

{3

in

the

integral for

ho

we obtain

1

,"/2

K(COS2

'I')sin

'I'

cos

'I'

ho

= C

J{(1-

a1 sin

2

'1')(1-

{31

sin

2

'I')} d'l'.

(7.64)

The

height of the separation point above the dam base is

hs

= h + h

o

.

Cryer (1976a) pointed to errors in

the

expressions (7.62) and (7.64) in

Polubarinova-Kochina (1962) and his corrections have been included.

To

find the equation of the free surface consider the segment 0 <

~

< 1

Polubarinova-Kochina's solution for the simple dam

311

and

observe

that

Z=

ax

+i

ay

=-A

K(~)-iK(l-~)

(7.65a)

a~

../{(l-~)(a-~)(b-~)}'

a<f>

-kAK(l-~)

F=

a~

=

../{(l-~)(a-~)(b-~)r

(7.65b)

By

separating

real

and

imaginary

parts

in (7.65a)

and

substituting

KSlD

':t"SlD':t"

~

= sin

2

'I'

we

obtain

1'i'

( . 2

'T")

•

,T,

x =

e-c

../{(l-a

sin2

'1')(1-

~

sin2'1')} d'l',

(7.66a)

(

'i'

K(COS2

'I')sin

'I'

y = h +

ho

+

C"'\J

../{(1-a sin

2

'1')(1-

~

sin

2

'I')} d'l',

(7.66b)

for

O:s;;'I':S;;1T/2.

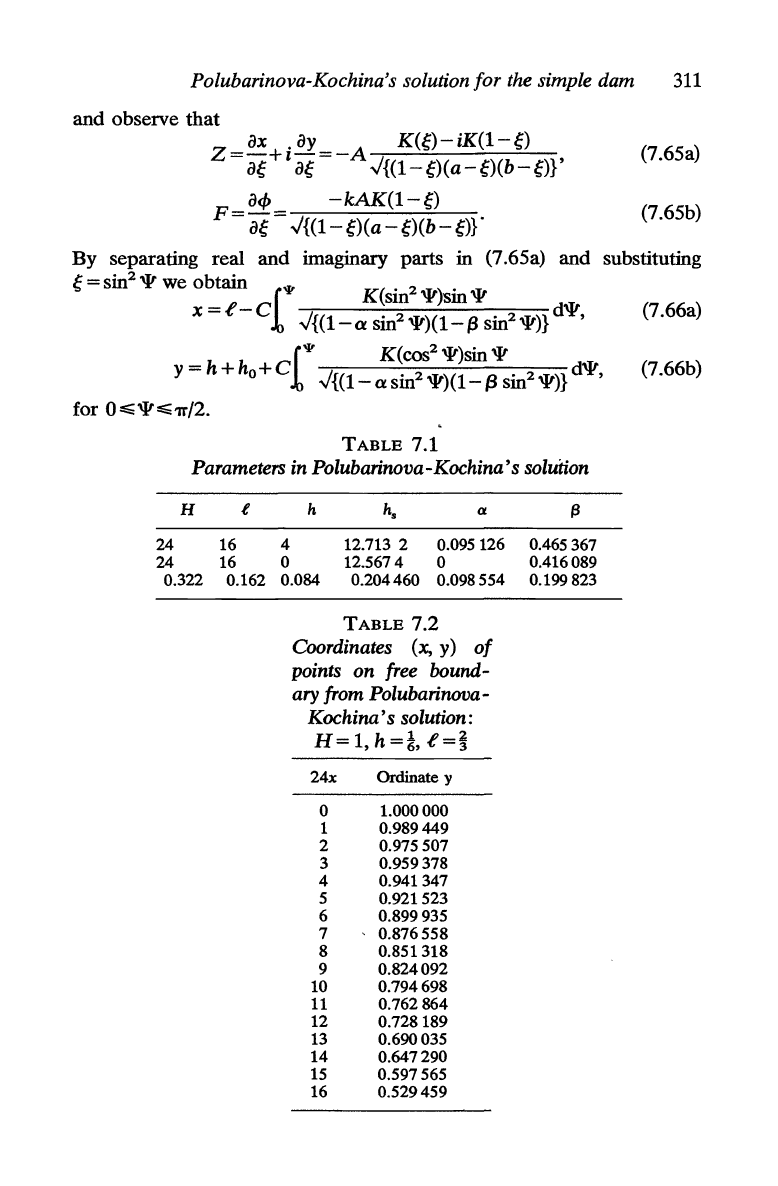
TABLE

7.1

Parameters in Polubarinova-Kochina' s soluiion

H

t h

h.

a

(3

24

16 4 12.713 2 0.095126 0.465367

24

16 0 12.5674 0

0.416089

0.322 0.162 0.084 0.204460

0.Q98554

0.199 823

TABLE

7.2

Coordinates

(x, y)

of

points on free bound-

ary from Polubarinova-

Kochina's solution:

H =

1,

h =

t,

e =

~

24x

Ordinate

y

0 1.000000

1 0.989449

2 0.975507

3 0.959378

4 0.941347

5 0.921523

6 0.899935

7 0.876558

8 0.851318

9 0.824092

10

0.794698

11

0.762864

12

0.728189

13

0.690035

14 0.647290

15

0.597565

16 0.529459

Crank J. Free and Moving Boundary Problems

Подождите немного. Документ загружается.