Crank J. Free and Moving Boundary Problems

102

Analytical solutions

The

problem is formulated by

the

system

o<x<s(t),

x>s(t),

aUt

aU2

ds

-Kt-+K

-=Lp-

ax

2

ax

dt'

x =

set),

Ut=Ut>

x=o,

t:;;:;,:o,

U2=-U

2

, x

~

00,

t:;;:;,:o,

x =

set),

t:;;:;,:o,

(3.1)

(3.2)

(3.3)

(3.4)

(3.5)

(3.6)

where

kt

=

KJ

PG,

i = 1, 2. No initial conditions are specified

at

this stage

of

Neumann's solution. They emerge later. Equations (3.1)

and

(3.4)

are

satisfied by

(3.7)

and (3.2)

and

(3.5) by

(3.8)

where A

and

B

are

constants

to

be determined. Condition (3.6) requires

(3.9)

The

two relationships (3.9) can only

be

satisfied for all t

if

s

=at~,

(3.10)

where a is a constant. By differentiating (3.7)

and

(3.8)

and

using (3.3)

and

(3.9)

the

constant a is seen

to

be

given by

the

root

of

UtKte-ot2/4k,

U

2

K

2e

--ot

2

/4/s

Lpa

(

'7Tkt)~

erf(

a/2kt)

(

'7T~)!

erfc(

a/2Iq)

=

2:

'

(3.11)

where p

is

the

density

of

both

ice

and

water

implying

that

no

volume

change occurs

on

melting.

Table 3.7 gives values of A =

a/(2ki)

computed by Churchill

and

Evans

(1971) for a wide selection of parameters. Some roots for water

and

ice

and different initial

and

surface temperatures

are

given by Carslaw

and

Jaeger (1959,

p.

286). Once a has

been

found from (3.11),

Ut

and

U2 can

Neumann's solution; generalizations; volume changes

103

be

written down from (3.7), (3,8), and (3.9). They are

U

l

X

Ul

= U

1

-

erf(a/2kt) erf 2(k

1

t)!'

(3.12)

U

2

x

~

= - U

2

+ erfc(a/2k!) erfc

2(k2t)~

.

(3.13)

It

is

now possible to see what initial conditions

are

satisfied by (3.10) and

(3.13).

At

t = 0 they give s = 0 and

~

= - U

2

,

i.e. the whole region

x>

0

is

solid

at

uniform

temperatur~

- U

2

•

The

special case in which the solid

is

initially at its melting temperature, so that U

2

= 0, is

the

single-phase

problem introduced in §1.2.1 and (3.11) reduces to

Ae

A2

erf A = U1cl/(L-rr!), (3.14)

where A =

a/(2kl).

Carslaw and Jaeger (1959, p. 287) show a graph of the

left-hand side of (3.14)

as

a function of A from which values of A can be

read for any given value

of

UlCl/(L~).

They also point

out

that for

small values of

A,

and hence of the right-hand side of (3.14), use of the

first

term

in the series expansion for erf A gives approximately

(3.15)

The

solutions (3.12), (3.13) and

the

equation (3.11) are quoted by

Carslaw and Jaeger (1959, p. 288). Their solution of

the

corresponding

solidification of a liquid in

x > 0 initially

at

a uniform temperature above

freezing

is

deducible by suitable changes of nomenclature.

Carslaw

and

Jaeger (1959, Chapter XI) also present Neumann-type

solutions for

other

physically important problems including the region

x>

0 initially liquid and x < 0 solid, the case in which melting occurs over

a temperature range, and three-phase problems. They solve the commonly

neglected problem in which motion of the liquid results from a change of

volume

on

solidification, due

to

a difference between the densities of solid

and liquid. As an example they consider the freezing of a semi-infinite

liquid in the region

x>

0 when the density of the solid,

PI'

exceeds that of

the liquid,

P2,

and both phases are incompressible. Thus

the

problem

is

defined by equation (3.1) in

the

solid phase but in the liquid phase (3.2)

is

replaced by

x>s(t),

(see equations (1.32) and (1.33».

On

the solidification boundary, x = s(t),

they take

U1

=

~

=

UM,

together with (3.3).

For

their solidification prob-

lem, instead

of

(3.4), (3.5), they have

U1

= 0, X = 0,

U2

~

U

as

x

~

00.

The

104

Analytical solutions

similarity solution based

on

s =

2A(klt)~

leads

to

the

equation for A

e-

x2

(U

-

U~Kzkte-x2""k,l""k2

AL7r~

erf'\' -

UMK

l

k!

erfc(APl

kif

1>214

Cl

U

M

'

which reduces

to

(3.11)

if

Pl =

Pz,

remembering

that

a = 2.\.kt.

Rubenstein (1971) in considering

the

crystallization of a supercooled

melt in contact with its solid phase (see Chambre 1956) also

took

density

changes into account

but

did

not

impose

the

condition of incompressibil-

ity in

the

liquid phase.

Thus

the

condition (1.32) still holds

on

the

phase-change boundary with v = v(s(t), t),

but

an equation

of

motion

av

aZv

-+v-=v-

s(t)<x<oo,

t>O

at ax ax

z

'

is

to

be

satisfied by v(x, t). Rubinstein takes

the

solid phase

to

occupy

the

space

-oo<x<s(t)

for

t>O

and

the

liquid phase

s(t)<x<oo,

coupled

with initial and boundary conditions

v=o,

v~o,

The

heat

flow equations are

auz=

kz

a

Z

U2

at ax

z

'

t=o,

x

~

00,

x

~

-00.

-oo<x<s(t),

t>o,

s(t)<x<oo,

t>o,

and conditions (3.3)

and

Ul

= U

z

= U

M

still hold

on

the

phase-change

boundary. Rubinstein derives a similarity solution based

on

s(t)=2~(vt)!,

v =

2(v/mN(1)),

Uj =

Uj(1)),

i = 1; 2

1)

=

x/{2(vt)~},

where

~,

f(1)), and

U;(1))

are

to

be

determined from

/(1)) - (4/7r!)/(1))f(1)) + 2{f(1)) +

TJ!(1))}

=

0,

ul(1))+{2ul1)

- (4/7r!)Ut!(1))}Ul(1)) = 0,

uz(1))

+

2uz1)u

z

(1))

= 0,

Uj(m =

UM,

Uj(1))

~

0;,

11)1

~

00,

i=1,2,

f(m

=

-17r~e~,

where e =

(Pz

- Pl)/Pt.

/(1))

~

f(1))

~

0,

1)

~

00;

-IL~

={Ul(1))-'Y

U

z{1))},

1)

=~,

with

Neumann's ;;olution; generalizations; volume changes

105

It

follows easily

that

where

with

and

finally

e~

exp{-(T/2-~2)}

!(T/) = -

2/7T~+

e~(erf

T/

-erf

~)exp(~2)

,

Ul(T/) = U

1

+

(UM-

U

1

)R(T/,

m,

1

+erf{T/.JO":J

U2(T/)

= U

2

+(UM-

U~

l+erf{~.JO":J·

These relationships yield

an

equation for

~.

Rubinstein pursues the

simplest case

of

0"1 = 1, which

he

considers relevant for a gas, and obtains

(2/.J7T)erfc

T/

Ul

= U

1

+

(UM-

U

1

)

{2/.J7T+

e~(erf

T/

-erf

mexp(~2)}erfc

~

and

{

_ ( _ )}

_~

{(

_ U )

exp(-~2)

'}'O"!(UM-

u~exp(-0"2~2)}

IJ.

eUM

Ul~-.J7T

UM

1

erfc~

+

l+erf(~.JO"~

.

Considerable simplification occurs

if

U

2

=

UM'

i.e.

the

solid phase

is

initially

at

the

crystallization temperature throughout.

In

a problem with several phases of different densities, Wilson (1982)

denoted

by

Sb

i = 1, 2,

...

, n

-1,

the

free boundaries separating phases of

densities Pi, i = 1, 2,

...

,

n,

together with

So

= 0 and

s"

is

the

right-hand

boundary,

not

necessarily

at

infinity.

If

the

whole slab is

of

mass M,

conservation

of

mass

is

expressed by

n-l

s,,(t) = M/p.. + L Sj(t)<Pi+l-fJj)/p..

j=1

as long as

Sn-l(t)

does

not

exceed Sn(t). When

Sn-l(t)

determined by

the

free-boundary problem becomes equal

to

s,,(t),

the

n·th phase has disap-

peared

and

a new free-boundary problem with (n

-1)

phases

is

to

be

considered. Similarly, a growing number of phases could

be

considered.

The

first local coordinate gl(X, t) is simply gl(X, t) = x,

O~x

~SI(t),

and

the

position

of

the

first moving boundary

is

denoted by f

1

(t) in

the

local

106

Analytical solutions

system so that r

1

(t) = gl(SI(t), t) = SI(t). Introducing

fLi

= pdPi+l> i =

1,2,

...

,n-1,

we further define

gz{x,t)=X-(l-ILI)rI(t)

and

rz<t)=

g2(S2(t),

t)=S2(t)-(1-ILI)rI(t)

for

SI(t)~X~S2(t).

In

general for

SI_l(t)~X~SI(t),

1-1

gl

(x, t) = x - L

(1-

ILj)rj(t),

j=1

1-1

rl(t)

=

SI(t)-

L

(1-

ILj)rj(t).

j=1

For

each

i,

gi

is

at

rest in the

ith

phase because

of

the

conservation of

mass

at

the

various interfaces.

The

position of

the

(i

-l)th

interface in

the

coordinate system

at

rest

with respect

to

the

ith

phase is given by

1-1

gi(SI-l(t), t) =

S;-I(t)-

L

(1-

ILj)rj(t)

j=1

i-2

1-1

= r

i

-

1

(t) + L

(1-

ILj)rj(t)- L

(1-

ILj)rj(t) =

fLi-l

r

i-l(t).

j=1

This can

be

made

to

include i = 1 by taking r 0 =

O.

In

order

to

retrieve x

and

SI

after computation in

the

local coordinates

we

need

1-1

X=gl(X,t)+

L

(l-ILj)r

j

(t),

j=1

i-I

Si(t) =

rj(t)

+ L

(1-

ILj)rj(t),

j=1

for i =

1,2,

...

,n

and

with r 0 =

O.

It

follows that

if

each r

j

is

propor-

tional

to

f( for some power

'Y,

then so is

Sj.

The

linear system for

the

Sj

in

terms of the r

i

may

be

inverted

to

give

j

rj =

pi

1

L

Pi(Sj

- Sj-l),

j=1

i = 1,

2,

...

, n

-1.

A problem considered by Weiner (1955)

and

for which Wilson (1978)

established

the

existence and uniqueness of similarity solutions can

be

used as an example.

The

one-dimensional

heat

flow equation

is

to

be

solved in consecutive regions of

the

half-space

x>

0 separated by phase-

change boundaries whose motions are

to

be determined.

The

constant

densities of

the

separate phases can differ from each other. As phase

changes occur

the

neighbouring regions are assumed

to

remain in contact

but

energies associated with acceleration, friction, etc. are neglected.

The

dependent variable,

u,

may undergo a finite discontinuous

jump

at

an

interface between specified, constant, limiting values

on

the

two sides

and

Neumann's solution; generalizations; volume changes

107

is also constant

at

the

fixed boundary, x =

O.

Initially all free boundaries

coincide

at

x = 0, and u =

Un

= constant for

x>

0, for t = 0 and u

~

Un

as

x

~

00,

t~O.

Here

So

= 0 and

Sn

=

00.

By using

the

nomenclature defined above

and

assuming

the

usual

Stefan condition

to

hold

on

each interface, with

~

denoting latent heat

per

unit mass of

the

ith

phase for the change from

the

ith

to

the (i +

l)th

phase,

the

problem can

be

stated as follows:

U;t

=

k;Uq;g,

U;-lfi-l(t)

<

~

<fi(t),

t > 0, i =

1,

...

,

n,

u;(t, lJ-i-lI7-l(t)) = V';-h t

>0,

i =

1,

...

,

n,

u;(t,ri(t))=U

i

+.:1j,

t>O,

i=

1,

...

,

n-1,

t>O,

i = 1,

...

,

n-1,

t~O,

fi(O)

=0,

i = 1,

...

,

n-1,

~>O,

t=O.

Here

K; denotes

heat

conductivity and

k;

heat diffusivity. Weiner's (1955)

similarity solution is expressed

by

Wilson (1982) as

U;(t,

~)

=

(Vi-l

+ a;)[erfcWv'(4k;t)}/erfc{a;-llJ-i-

l

v'(k;-l/k;)}]-a;,

t>O,

IJ-i-lfi-l(t)<~<fi(t),

i = 1,

...

,

n-1,

fi

(t) = arv'( 4k;t), i = 1,

...

, n

-1,

and for

~>rn-l(t),

t>O

In

these equations, for i = 1,

...

, n

-1,

if

k,.

=0,

k,.>0.

al

=

-Vi-l

+

(Vi-l

-

V';

-

.:1

i

)erfc{a;-llLi-lv'(k;-l/k;)}/<l>;,

<l>i

= erf(a;) -erf{a;-llJ-i-lv'(k;-l/k;)},

and

the

a;

(i

= 1,

...

, n

-1)

are

the

unique solution of

the

non-linear

equations

-(J;~k;a;v'

7T

= - K; (V';-l -

Vi

-

.:1;)exp(

-ai)/<I>lv'k;)

+ K

i

+1(Vi

-

Vi

+1

-.:1

i

+

l

)exp(-arlLrk;/k;+l)/(<I>i+lv'k;+l),

i

=1,

...

,

n-2,

108

Analytical

solutions

and

Pn-lL..-lk,,-Ia..-I-17T + K;..-I(U

n

-

2

-

U

n

-

1

-

A.

n

-

1

)exp(

-a~-I)/(<Pn-l-1k,,-l)

{

o

if

k,,=0

=

Kn

(U

n

-

1

-

Un)exp(-a~-I#L~-Ik,,-I/k,,)/(<Pn

-Ik,,)

'if

k"

1

O.

In

this last expression consistency with the previous definition

is

achieved

by taking erf(a..)

==

1 and

<Pn

= 1-erf{a..-l#Ln-l-1(k,,-I/k,,)}.

In

the defini-

tion of

<PI

the dummy parameters ao,

ko

are taken to

be

zero.

Wilson

(1978) established

the

existence and uniqueness of

the

similar-

ity solution subject

to

the following conditions:

Ie;

>0,

K;

>0,

i =

1,

...

,

n-l,

and either

k"

>0,

Kn

>0,

or

k"

=

Kn

=0;

and either

Vi-I>

Vi

+.1;,

1..;

~O,

i = 1,

...

,

n-l

and U

n

-

1

~

Un;

or

Vi-I <

Vi

+.1;,

1..;

~O,

i = 1,

...

,

n-l

and U

n

-

1

~

Un;

provided

that

if

U

n

-

1

=

Un

then L..-l

10;

and

if

k"

=Kn

=0

then U

n

-

1

=

Un

Reverting to the original frame of reference we see that

i=2,

...

,n,

and SI(t)

=fl(t)

=

2a

l-1(k

1

t).

Although a similarity solution has

been

obtained for this example,

Wilson

(1982) suggests

the

use of

the

same local coordinates in combina-

tion with finite elements

or

finite differences in

more

complicated prob-

lems.

A problem relating to

the

manufacture of glass in which volume

changes are substantial enough to

be

of

practical importance

is

reported

by Gelder and

Guy

(1975).

Carslaw and Jaeger (1959, p. 287) derive a similarity solution for

the

solidification of a supercooled liquid initially in x > 0, i.e.

the

region x > 0

contains material

at

temperature V <

Tl

which

is

in

the liquid state even

though its temperature

V

is

below

the

solidification temperature T

1

•

Solidification starts at

the

plane x = 0 and moves

to

the

right; no

heat

is

removed from

the

solidified material

and

so

it

will have the constant

temperature

Tl

throughout. In

the

supercooled liquid there will

be

a

temperature profile

of

the form

x

v = V + A erfc 2(k2t)!

and

the

usual Stefan conditions expressing

heat

conservation

on

the

boundary,

s(1)

= 2A(k

2

t)!, between

the

solid and liquid phases, leads to

the

relations V + A erfc A =

Tl

and

Ae-

A2

=

Alm!/c2'

It

follows

that

A

is

the

root of

Ae

A2

erfc A =

(T

1

-

V)c

2

/L7T!

and

Carslaw and Jaeger give a

graph of

Ae

A2

erfc

A.

Neumann's solution; generalizations; volume changes

109

The

analytical properties of more general Stefan problems, which

include superheated solids and supercooled liquids, have

been

examined

by Sherman (1970).

In

his

paper

reference is made

to

several earlier

papers of a similar nature.

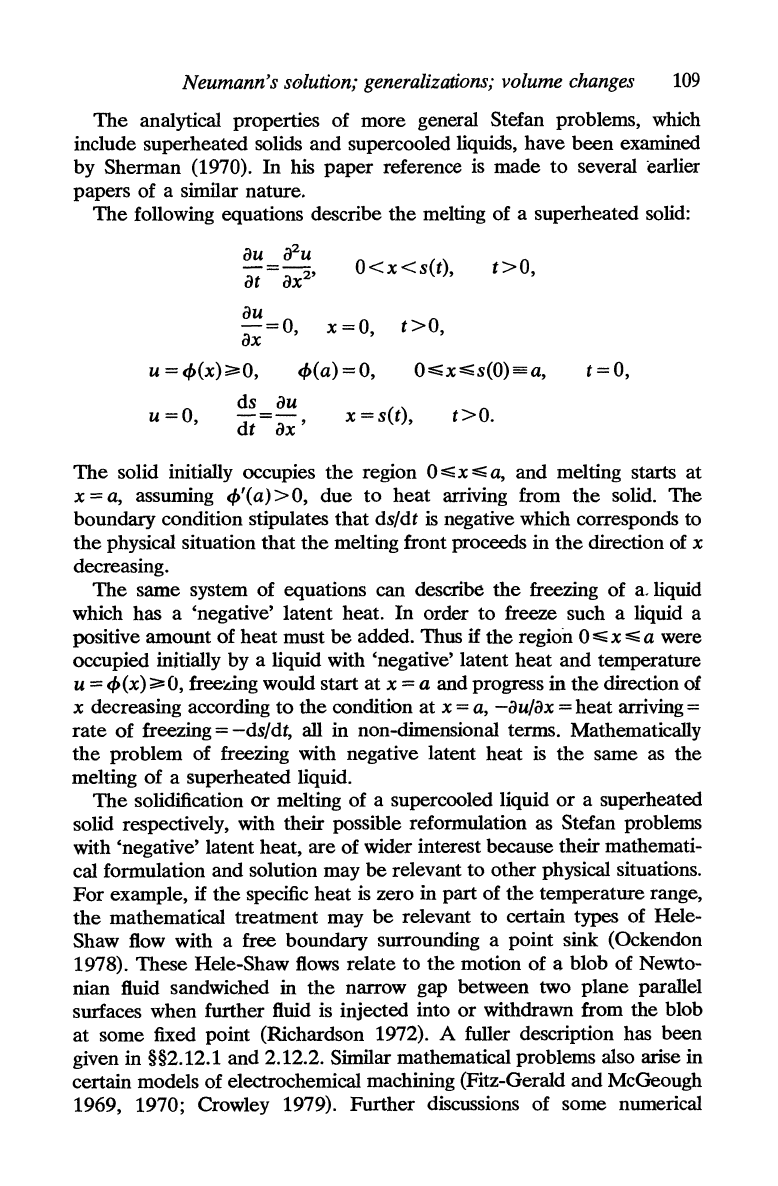
The

following equations describe

the

melting of a superheated solid:

u=O,

au

a

2

u

at=

ax

2

'

O<x<s(t),

t>O,

au

=0

0 0

ax

' x

=,

t>,

<f>(a)

=0,

O:s;;x:S;;s(O)==a,

x = s(t),

t>O.

t=O,

The

solid initially occupies

the

region

0,;;;

x

:s;;

a,

and melting starts

at

x

==

a,

assuming

<f>'(a)

>0,

due

to

heat arriving from

the

solid.

The

boundary condition stipulates

that

ds/dt

is

negative which corresponds to

the

physical situation

that

the

melting front proceeds in

the

direction of x

decreasing.

The

same system of equations can describe

the

freezing of

a.

liquid

which has a 'negative' latent heat.

In

order

to

freeze such a liquid a

positive amount

of

heat must

be

added. Thus

if

the

region

O:S;;

x

:s;;

a were

occupied initially by a liquid with 'negative' latent heat

and

temperature

u

==

<f>(x)~O,

freeL.ing

would start

at

x

==

a and progress in

the

direction of

x decreasing according

to

the

condition

at

x =

a,

-au/ax

= heat arriving

==

rate

of

freezing

==

-ds/dt,

all in non-dimensional terms. Mathematically

the

problem of freezing with negative latent heat is

the

same as the

melting

of

a superheated liquid.

The

solidification

Or

melting of a supercooled liquid

or

a superheated

solid respectively, with their possible reformulation as Stefan problems

with 'negative' latent heat, are of wider interest because their mathemati-

cal formulation and solution may

be

relevant

to

other

physical situations.

For

example,

if

the

specific heat

is

zero in part of

the

temperature range,

the

mathematical treatment may

be

relevant

to

certain types of Hele-

Shaw flow with a free boundary surrounding a point sink (Ockendon

1978). These Hele-Shaw flows relate

to

the

motion of a blob of Newto-

nian fluid sandwiched in

the

narrow gap between two plane parallel

surfaces when further fluid is injected into

or

withdrawn from the blob

at

some fixed point (Richardson 1972). A fuller description has been

given in §§2.12.1 and 2.12.2. Similar mathematical problems also arise in

certain models of electrochemical machining (Fitz-Gerald and McGeough

1969, 1970; Crowley 1979). Further discussions of some numerical

110

Analytical solutions

treatments of problems of this kind are

to

be found in §§2.12.2, 2.12.3,

and 6.2.7. Crank (1975a, Chapter 13) gives Neumann-type solutions for

several diffusion problems including

the

general approach

by

Danckwerts

(1950) which also allows for movement of

the

medium on

one

side of

the

boundary relative

to

the

other. Mikhailov (1975) obtains similarity solu-

tions of coupled equations for temperature and moisture distributions

together with

the

position of a moving evaporation front during

the

drying of a porous region x

>0,

and

he

also studied (1976)

the

corres-

ponding freezing problem. Tayler (1975) refers

to

similarity solutions

describing

the

solidification of

an

alloy as formulated in §1.3.7 for some

particular initial

and

boundary conditions.

He

also remarks

on

the

limit-

ing cases of instantaneous diffusion of

heat

or

material

and

of

no diffusion

of material. Kamin (1976) and Peletier and Gilding (1976) obtain similar-

ity solutions for a non-linear filtration problem obeying

au/at

=

if'(u

m

)/ax

2

,

m > 1.

3.3.

SimDarity solutions

in

cylindrical

and

spherical

coordinates

Similarity solutions of problems with radial symmetry,

if

they exist, can

be

expected

to

be

functions of

r/t!

only by analogy with

the

linear cases

discussed in §3.2.

Frank

(1950) presents solutions contained in a disserta-

tion by Rieck in 1924

and

quoted by

Huber

(1939),

and

applies them

to

spherical and cylindrical phase growth. Retaining Frank's nomenclature

for ease of reference, concentration (or temperature) is denoted

by

cf>

=

cf>(r,

t) =

cf>(s)

where s = r/(Dt)!, D is

the

diffusivity,

and

r

the

radial

coordinate.

Then

the

usual diffusion equation for spherical symmetry

becomes

and successive integration yields

cf>

-

cf>co

= A {s

-1

exp( -

~S2)

-

!'1T!

erfc@s)}

=

AFis)

,

(3.16)

(3.17)

where

cf>co

is

the

value

of

cf>

at

infinity.

The

constant A is related

by

Frank

(1950)

to

q,

the

amount of diflusant expelled

per

unit volume of new

phase (analagous

to

latent heat) formed as growing proceeds, and

he

deduces from

the

conservation condition on r = R

(3.18)

where S

=

R/(Dt)!

and

R is

the

outer

radius of

the

sphere.

On

the

surface

Similarity solutions and moving heat sources

111

r = R,

cf>

has

the

constant value

cf>s,

where

cf>s

= !qS3 exp(iS

2

)FiS)

+

cf>co

E qf3(S) +

cf>co.

(3.19)

For

the

corresponding radial cylindrical problem Frank (1950) obtains

the

solutions

(3.20)

where

Ei

is

the

exponential integral

and

on

the

surface of

the

growing

cylinder

the

constant

cf>s

is

cf>s

= - iqS2 exp(iS2)Ei( -

iS~

+

cf>co

==

qfZ{S) +

cf>coo

(3.21)

In

the

linear case for comparison

t1(S)

=!S

exp(iS2)F

1

(S) (3.22)

and

F

1

(s) =

7T~

erfc@s). (3.23)

Frank

(1950) tabulates

the

functions

F3(S),

tiS),

F

2

(s),

and

tz{S) and

quotes series

and

asymptotic expansions for F(s)

and

f(S)

for

both

the

spherical

and

cylindrical cases.

Carslaw

and

Jaeger (1959, p. 295) give similarity solutions in cylindri-

cal

and

spherical coordinates where

the

region r < R consists of solid

at

its melting point and r > R of supercooled liquid whose temperature

tends

to

V <

the

melting temperature

as

r

-+

00.

They also solve a cylindri-

cal problem of freezing

by

an

axial line source.

3.4.

SimDarity solutions and moving heat sources

Lightfoot (1929) developed a method suitable

if

the

thermal properties

of

the

solid

and

liquid

are

assumed

to

be

the same.

If

the

solidification

front in a one-dimensional problem

is

at

x =

s(t),

and

moves with a

velocity

s(t),

the

latent

heat

of solidification

is

liberated

on

the

front at a

rate

Lps(t). (3.24)

This liberation of heat may

be

represented by a moving source of heat at

s(t)

of

strength (3.24).

The

temperature at any point will

be

due

to

the

superposed contributions from this moving source, having

the

initial and

boundary conditions in mind.

An

integral equation for

s(t)

can be

developed which expresses

the

fact

that

the

temperature

on

the

boundary

at

x =

s(t)

is

always

the

melting temperature. In this way, Lightfoot

Crank J. Free and Moving Boundary Problems

Подождите немного. Документ загружается.