Cooper L.N., Feldman D. (Eds.) BCS: 50 Years

Подождите немного. Документ загружается.

September 17, 2010 9:51 World Scientiﬁc Review Volume - 9.75in x 6.5in ch5

Nuclear Magnetic Resonance and the BCS Theory 61

Fig. 5. Charlie Slichter, Urbana, 1950.

only electrons that could participate in such a process are those near the

Fermi energy where there are empty states into which an electron can be

scattered.

The idea that an energy gap would have a strong eﬀect on the nuclear

spin-lattice relaxation time was very exciting until I suddenly realized that

a superconductor is perfectly diamagnetic and would therefore exclude the

magnetic ﬁeld (this was before the discovery of Type II superconductors).

How could one do NMR in a substance that excluded magnetic ﬁelds? By

this time in John’s talk I had quit listening and was focused on how to do

NMR in a superconductor.

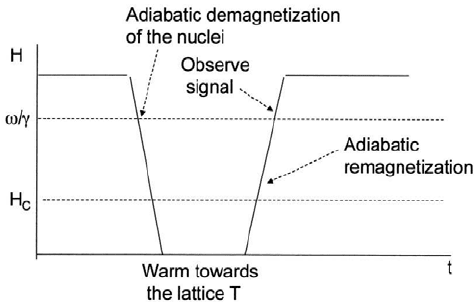
Then an idea came to me: use a time dependent “static” magnetic ﬁeld,

cycling between a strong ﬁeld that suppressed the superconductivity of the

sample and zero magnetic ﬁeld that rendered the sample superconducting

(Fig. 6). Initiate the experiment by applying a magnetic ﬁeld H

, much

stronger than the superconducting critical ﬁeld H

so that the superconduc-

tivity of the sample was suppressed, and leave it on long enough for the

nuclear magnetization to be established. Use an NMR apparatus operating

at a frequency that places the resonance at a ﬁeld H

reson

less than H

but

September 17, 2010 9:51 World Scientiﬁc Review Volume - 9.75in x 6.5in ch5

62 C. P. Slichter

Fig. 6. Schematic picture of the cycle of magnetic ﬁeld, H, versus time, t, to make

possible measurement of nuclear relaxation in a material that excludes “static”

magnetic ﬁelds when the sample is in the superconducting state. As long as H

is greater than the superconducting critical ﬁeld, H

, the sample is in the nor-

mal state, permitting static and alternating ﬁelds to penetrate the sample. The

adiabatic demagnetization leaves the nuclear spin temperature far below the lattice

temperature and the sample in the superconducting state. The spin-lattice relax-

ation process warms the nuclear spins in the superconducting state. The extent of

the warming is monitored by observing the resonance on the ﬂy as the sample is

adiabatically remagnetized.

stronger than H

. Turn the magnetic ﬁeld to zero, suﬃciently slowly to

be an adiabatic demagnetization. This step would leave the nuclear spins

at a very low spin temperature compared with the lattice and the sample

superconducting. The nuclei would then start to warm towards the lattice

temperature. After a time t

warm

, turn the magnetic ﬁeld back on to its

initial value H

, inspecting the NMR signal on the ﬂy as the ﬁeld passes

through the value H

reson

. Then study the strength of the NMR signal as a

function of t

warm

, thus deducing the nuclear spin-lattice relaxation time in

the superconducting state.

The nuclear spin-lattice relaxation time, T

, is the length of time it takes

the nuclei to establish their thermal equilibrium magnetization, M

, in an

applied magnetic ﬁeld. The ﬁrst theory of T

in a metal was actually made by

Heitler and Teller

in 1936, long before the invention of magnetic resonance,

in a paper in which they explored using the nuclear magnetization of a metal

to achieve cooling by adiabatic demagnetization of the nuclear magnetism.

The nuclear spins would then cool the solid. They predicted that the nuclear

spin lattice relaxation time in a metal would vary inversely with 1/T .

September 17, 2010 9:51 World Scientiﬁc Review Volume - 9.75in x 6.5in ch5

Nuclear Magnetic Resonance and the BCS Theory 63

In 1950, Korringa

worked out a detailed general theory of the nuclear

spin-lattice relaxation time in a metal, showing that it was closely related to

the Knight shift. Korringa had worked out the theory for metals in a strong

magnetic ﬁeld. In this case, the nuclear energy levels are just those of the

Zeeman eﬀect. But in zero ﬁeld, the Hamiltonian is just the dipolar coupling.

The eigenstates and energy levels were not known in this case. For my PhD

thesis I had used magnetic resonance to measure the T

of electron spins in

paramagnetic salts.

I had worked out a general expression describing the

relaxation of a spin system that at all times during the relaxation process

could be described by a spin temperature. I give the derivation below. Using

it, we found that we could express the quantum mechanical aspects of the

theory as a trace, making it unnecessary to solve the zero ﬁeld Hamiltonian

to determine the magnetic ﬁeld dependence of the relaxation time.

In many cases the relaxation process is described by the equation for the

time dependence of the magnetization, M:

= −

M − M

, (1)

where the thermal equilibrium magnetization, M

, obeys Curie’s law relating

magnetization of N nuclei of spin I, with gyromagnetic ratio γ

, to the lattice

temperature T

, the Boltzmann constant k

, and the Curie constant, C

, (2)

where

C = N

I(I + 1)

. (3)

Notice that if H

is zero, the thermal equilibrium magnetization is zero for

any value of the lattice temperature. In our experiment, we turn the external

magnetic ﬁeld, H

, to zero. I have called it an “adiabatic demagnetization”

since we take pains to employ a reversible process that maintains the order

of the system. From Curie’s law, we see that the magnetization is zero in

zero applied magnetic ﬁeld. In the strong ﬁeld, the order of the system is

manifested in the magnetization. Where has it gone when the magnetization

is zero in zero applied ﬁeld? Clearly, it now must reside in the alignment

of the nuclear spins along the local magnetic ﬁelds of their neighbors. Since

these local ﬁelds are randomly oriented, no net magnetization results.

In such a process, the entropy of the system, σ, remains constant during

the demagnetization process. Consequently, if the system were perfectly

isolated from the lattice, one could then turn the magnetic ﬁeld back to its

September 17, 2010 9:51 World Scientiﬁc Review Volume - 9.75in x 6.5in ch5

64 C. P. Slichter

original value, thereby recovering the initial magnetization without further

need for a T

process.

For a system of N spins, the entropy when the Zeeman energy is lower

than the thermal energy at temperature θ is given by

σ = Nk

ln[2I + 1] −

C(H

+ H

loc

)

2θ

, (4)

loc

(2I + 1)

Tr[H

]s , (5)

i,j

(γ

· I

− 3

· R

)(I

· R

)

, (6)

and H

is the Hamiltonian of the nuclear dipole-dipole coupling, and R

the distance between nuclei i and j. This relationship shows that if one starts

with a spin system in a strong magnetic ﬁeld H

, in thermal equilibrium

with the lattice at temperature θ

, lowering the applied ﬁeld to zero, cools

the spin system to a temperature, θ, of

θ = θ

loc

. (7)

To describe the spin-lattice process warming the system in zero magnetic

ﬁeld, it is simplest to talk about the temperature of the spin system since

the magnetization is zero as long as the applied ﬁeld vanishes. The concept

of spin temperature goes back to Casimir and du Pre

in the 1930s when

adiabatic demagnetization was invented as a method of obtaining low tem-

perature. Gorter utilized the spin temperature concept extensively in his

monograph on paramagnetic relaxation

with which I had become familiar

since Gorter taught a course using it while a visiting professor at Harvard in

the summer of 1947. The trace methods, involving spin temperature, that

we utilize below were ﬁrst introduced to calculations of relaxation rates by

Van Vleck in his paper on paramagnetic relaxation in Ti and Cr alums.

We then describe the relaxation process in terms of the energy of the spin

system. It is convenient to introduce the notation

β =

. (8)

For all values of applied magnetic ﬁeld, the nuclear spin system is de-

scribed by the Hamiltonian

H = H

+ H

, (9)

September 17, 2010 9:51 World Scientiﬁc Review Volume - 9.75in x 6.5in ch5

Nuclear Magnetic Resonance and the BCS Theory 65

where H

is the Zeeman energy of all N nuclear spins,

= −γ

j=1

. (10)

We label the eigenvalues of the Hamiltonian, H, as E

. Then, the mean

energy, E, of the system is given by

E =

, (11)

where p

is the probability that state n is occupied.

Using the fact that

exp(−βE

)

(2I + 1)

, (12)

we ﬁnd

E = −β







(2I + 1)







. (13)

The spin-lattice relaxation process changes the populations of the energy

states. Then, assuming that we have simple rate processes

− p

, (14)

where W

is the rate of transitions induced from state m to state n by the

external world (the thermal reservoir) so that

n,m

− p

] . (15)

If the system is described by a spin temperature as the energy change

takes place, then we also have

= −

dβ

(2I + 1)

. (16)

To guarantee that the transitions will lead to a population corresponding

to the lattice temperature, we invoke the principle of detailed balance:

= W

exp[(E

− E

)β

] . (17)

September 17, 2010 9:51 World Scientiﬁc Review Volume - 9.75in x 6.5in ch5

66 C. P. Slichter

Combining Eqs. (14)–(16), we ﬁnd

dβ

β − β

n,m

− E

)

(18)

Equation (14) is a normal modes problem with many real exponential

roots. The imposition of the assumption that the spins are described by

spin temperature at all stages of the relaxation leads to a process described

by a single exponential relaxation as described by Eq. (18). Experimentally,

we ﬁnd a single exponential, providing an experimental justiﬁcation of the

spin temperature assumption.

In a metal, the dominant source of nuclear relaxation is coupling between

the magnetic moments of the nuclei and the conduction electrons. The

process may be viewed as a scattering process in which the nuclei in an

initial state m scatter an electron from a state k, s into a ﬁnal state k

, s

while making a transition to a ﬁnal nuclear state n. (Note that these are the

eigenstates of the many-nucleus system, not those of a single nucleus.) For

many metals, the scattering interaction is the Fermi contact term between

an electron at position r

and a nucleus at position R

Fermi



8π



· I

δ(r

− R

) . (19)

Then, the probability per second of the transition from initial state m,

k, s to ﬁnal state n, k

, s

mks,nk



2π



|hmks|H

Fermi

|nk

δ(E

mks

− E

) . (20)

To have the initial state occupied and the ﬁnal state empty, satisfying

the Pauli principle, for the normal state we have

ks,ks

mks,nk

f(E

)(1 − f(E

)) , (21)

where f(E) is the Fermi function.

Assuming that the electron wave functions are products of an electron

spin function and a Bloch function ψ

) = u

ikr

, where u

has the

periodicity of the lattice, and assuming a spherical Fermi surface, we showed

September 17, 2010 9:51 World Scientiﬁc Review Volume - 9.75in x 6.5in ch5

Nuclear Magnetic Resonance and the BCS Theory 67

in the appendix of our 1958 paper that

i,j,α(=x,y,z)

hn|I

iα

|mihm|I

jα

|ni, (22)

where

64π

|u(0)

Fermi

sin

)

ρ(E

)ρ(E

)f(E

)[1 − f(E

)]dE

(23)

We have pulled the wave function out of the integral, replacing it by its

value averaged over the Fermi surface. The temperature dependence arises

from its eﬀect on the Fermi functions.

It is useful to deﬁne the temperature dependent integral Σ

(T ) for later

use. It determines the temperature variation of the spin lattice relaxation

rate, R(T ), for a metal in the normal state,

(T ) =

ρ(E

)ρ(E

)f(E

)[1 − f(E

)]dE

. (24)

Utilizing Eqs. (18) and (22), we ﬁnd that

R ≡ 1/T

= Tr{[H, I

iα

][I

jα

, H]}/2 Tr(H

) . (25)

If we keep just the on-site terms i = j, we ﬁnd for the magnetic ﬁeld

dependence of the relaxation rate

R(T ) = a

(T )

Tr H

+ 2 Tr H

Tr H

+ Tr H

. (26)

In a strong magnetic ﬁeld, this gives R(T ) = a

(T ) which is just the

Korringa result. It is easy to show that the rate then is proportional to the

absolute temperature, T .

In zero applied ﬁeld, the rate is predicted to be twice as fast as the rate

in strong applied ﬁeld. Experimentally, this is found to be true for the alkali

metals whose relaxation rates are slow enough for the ﬁeld cycling method to

work. For Al, we found that the experimental ratio is 3.35 ±0.35. Although

this diﬀered from the predicted ratio, the data ﬁt a similar mathematical

form

R(H, T )/R(∞, T ) = (H

+ A

)/(H

+ A) . (27)

We do not know why Al diﬀers from the case of the alkali metals.

Dick Norberg and Don Holcomb had both gone to college at DePauw in

Greencastle, Indiana. They told me of another very bright Illinois gradu-

ate student from there, Chuck Hebel, who was at that time working as a

September 17, 2010 9:51 World Scientiﬁc Review Volume - 9.75in x 6.5in ch5

68 C. P. Slichter

Fig. 7. Chuck Hebel in 1953.

research assistant at the Betatron and was thinking of switching ﬁelds and

urged me to try to recruit him for the experiment. I told Chuck about the

idea for the experiment and, to my delight, he decided to undertake it for

his PhD thesis. As I explain below, this experiment was quite unconven-

tional, pushing the boundaries of NMR work. It was a very challenging

project, requiring substantial building of apparatus and overcoming diﬃcult

experimental challenges.

Almost everything my students had been doing was quite novel and

unconventional. Norberg

studied the famous H-Pd system, using

H NMR

spin echoes from a short piece of Pd wire containing H. Norberg and

Holcomb’s study of the alkali metals introduced use of the boxcar integra-

tor, giving pulse techniques the ability to signal average analogous to the use

of lock-in ampliﬁers for steady state NMR. Our experiments on the alkali

metals were the ﬁrst to measure self diﬀusion in solids by NMR pulse tech-

niques. In the Overhauser eﬀect experiments, we did magnetic resonance

at magnetic ﬁelds of only a few tens of gauss, performed the ﬁrst electron-

nuclear double resonance, made the ﬁrst measurements of the spin lattice

relaxation time of conduction electrons, and made the ﬁrst demonstration of

dynamic nuclear polarization. Our experiments on static magnetic suscep-

tibility showed for the ﬁrst time how, using magnetic resonance, one could

directly measure the spin susceptibility of conduction electrons and were

September 17, 2010 9:51 World Scientiﬁc Review Volume - 9.75in x 6.5in ch5

Nuclear Magnetic Resonance and the BCS Theory 69

Table 1. Properties of some candidate metals.

207

199

115

(K) 7.19 4.13 3.40 1.17

Abundance 21% 16% 95% 100%

ν (MHz/T) 8.9 7.6 9.3 11.1

(gauss) 803 412 293 105

at 1 K (msec) ≈ 10, too fast ≈ 10, too fast ≈ 10, too fast 450

the very ﬁrst measurements of the famous Pauli electron spin susceptibil-

ity. My students generated an atmosphere of excitement, were seeking new

challenges, and eager to do things that had never been done before. What

a joy to be around them! That was the atmosphere Chuck stepped into and

rapidly enhanced.

The key issue in planning was the selection of the sample material since

its properties determined all the other experimental parameters. There were

four possible candidates, Hg, Pb, In and Al. Their properties are listed in

Table 1.

We had to be able to turn the magnet on and oﬀ in a time fast com-

pared with the nuclear spin-lattice relaxation times. Al had a spin-lattice

relaxation time of about a half second, a very favorable number, whereas

the others had expected T

’s in the millisecond time range, too short to be

workable. However, the superconducting transition temperature of Al was

only 1.17 K, a diﬃcult temperature to achieve. At that time, there were

two choices to reach such a temperature: pump on

He or adiabatic demag-

netization. This experiment was the ﬁrst one my group had attempted in

the liquid helium range, so we consulted our colleagues Dillon Mapother and

John Wheatley. It is very hard to get much below about 2 K by pumping

He because of the Rollin ﬁlm, but the alternative of cooling by adiabatic

demagnetization would have added very great complexity to what was al-

ready a challenging experiment. We decided to pump on

He using a special

design of He chamber Chuck developed based on their advice. We utilized

three Dewars. An outer liquid nitrogen Dewar enclosed a liquid He Dewar

containing liquid He at 4.2 K and an inner liquid He Dewar connected to

a oil booster pump backed by a mechanical pump. The inner Dewar con-

tained the sample that sat in a

He bath that was sealed beneath two one-

millimeter constrictions placed for the purpose of cutting down the ﬂow of the

Rollin ﬁlm.

September 17, 2010 9:51 World Scientiﬁc Review Volume - 9.75in x 6.5in ch5

70 C. P. Slichter

We needed a magnet that we could switch suﬃciently rapidly yet pro-

duced a strong enough magnetizing ﬁeld to give an observable NMR signal.

Conventional NMR used iron magnets that generated 10,000 gauss, putting

the NMR frequency at about 10 MHz. The time to turn such a magnet oﬀ

or on is measured in minutes, not fractions of a second. At lower magnetic

ﬁelds the NMR signals are weaker, but our experience from our work on

the Overhauser eﬀect where we used 50 kHz for the NMR frequency with

magnetic ﬁeld of order 30 gauss (generated by an air core solenoid) gave us

conﬁdence that we could work at magnetic ﬁelds much lower than 10,000

gauss. Since H

of Al is 105 gauss, Chuck and I decided to use a polarizing

ﬁeld, H

, of 500 gauss, and magnetic ﬁeld to detect the resonance, H

reson

of 360 gauss, putting the NMR frequency at 400 kHz. From his work at the

Betatron, Chuck knew that there were some thin sheets of magnet iron left

over from the recent construction of the Betatron, and proposed using them

to construct a magnet we could pulse oﬀ and on. It consisted of 0.014-in

thick silicon steel sheets with adjustable laminated sections near the magnet

gap as shims to obtain favorable magnetic ﬁeld homogeneity at the posi-

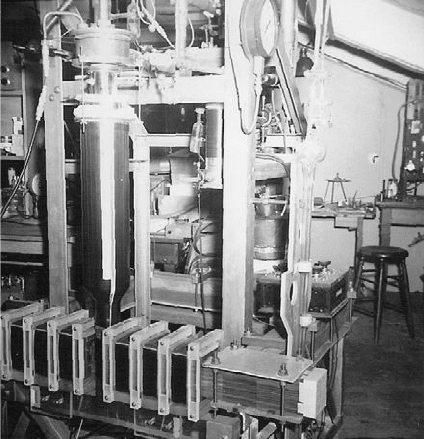
tion of the sample. Figure 8 shows the magnet and Dewar assembly Chuck

designed.

Fig. 8. Our apparatus, showing the Dewar system hanging vertically into the

magnet gap. The magnet, made of sheets of Betatron iron, and the energizing

coils are evident on either side of the bottom of the Dewar.